Solving Linear Differential Equations with an Integrating Factor (Differential Equations 16)
TLDRThe video script is an in-depth tutorial on solving linear first-order differential equations. The instructor emphasizes the importance of identifying whether an equation is linear and separable, and provides a step-by-step guide on how to transform equations to fit specific techniques. The video covers various examples, demonstrating the process of simplifying equations, applying product rules, and performing integrals to find solutions. The instructor also highlights the significance of checking work and understanding the mathematical concepts behind each step.
Takeaways
- 📚 The video discusses techniques for solving linear first-order differential equations, emphasizing the importance of recognizing whether an equation is separable or linear.
- 🔍 The speaker advises checking if the equation fits the technique being used, and if not, to modify it to fit, or choose a different technique.
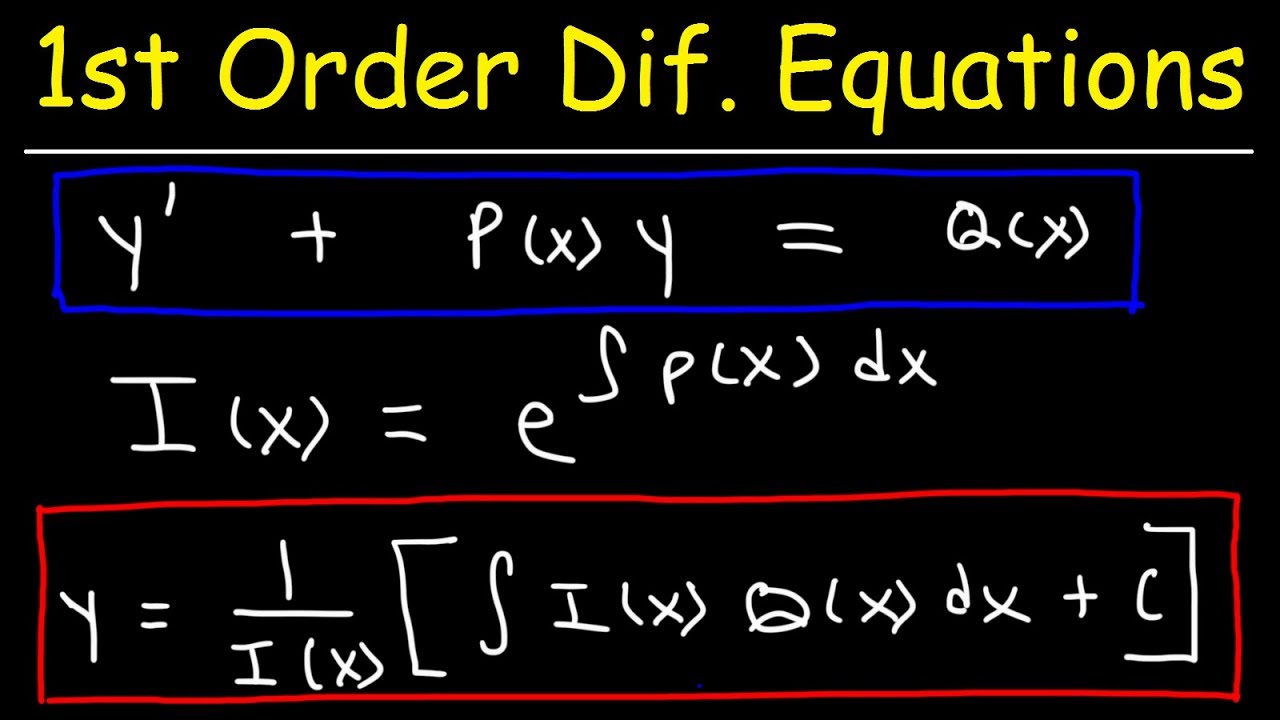
- 🎯 When dealing with linear equations, the goal is to have a first derivative of y, a function of x times y to the first power, and no other y terms.
- 🧩 For linear equations, the process involves identifying the function P(x) that multiplies y, integrating it to find the exponential function, and then multiplying all terms by this exponential to satisfy the product rule.
- 📈 The video provides a step-by-step walkthrough of solving several examples, including the integration and differentiation processes necessary to transform and solve the equations.
- 🤔 The speaker emphasizes the importance of understanding the underlying calculus concepts, such as the product rule and the fundamental theorem of calculus, which states that integrals and derivatives are inverse operations.
- 🛠️ The video script includes examples where the equation does not initially fit the linear form, requiring algebraic manipulation such as factoring or grouping terms to make it linear.
- 📌 When solving, it's crucial to check the work by ensuring the derivative of the proposed solution matches the original equation's right-hand side.
- 📋 The speaker also discusses the use of initial conditions to find the constant of integration, C, when provided in the problem.
- 🤹♂️ The video highlights the flexibility in choosing techniques, suggesting that the path of least resistance—requiring the least amount of work—should be preferred when possible.
- 🌟 The overarching goal of the video is to reinforce the method for solving linear first-order differential equations and to provide practice with various problem-solving strategies.
Q & A
What is the main focus of the video?
-The main focus of the video is to teach the technique of solving linear first-order differential equations through various examples, emphasizing the importance of fitting the equation to the appropriate technique and understanding the underlying mathematical concepts.
Why is it important to check if the equation fits the technique being used?
-It is important to check if the equation fits the technique being used because it ensures that the correct method is applied to solve the differential equation, which can prevent errors and simplify the problem-solving process.
What is the first step in solving a linear first-order differential equation?
-The first step in solving a linear first-order differential equation is to identify if the equation is separable or linear and to make sure it fits the desired technique, such as the product rule, before proceeding with the solution.
How does the video demonstrate the application of the product rule in solving differential equations?
-The video demonstrates the application of the product rule by showing how to multiply both sides of the equation by an exponential function, which is derived from the integral of the function connected to the variable y, to complete the product rule and simplify the equation for solving.
What is the significance of identifying P(x) in the process of solving the equation?
-Identifying P(x) is significant because it represents the function of x that, when integrated, gives the exponential function needed to complete the product rule and solve the differential equation.
How does the video handle equations that do not fit the linear first-order structure?
-The video suggests modifying the equation to fit the linear first-order structure by performing operations such as dividing by x or grouping terms, and then applying the appropriate technique to solve it.
What is the role of the exponential function in solving these equations?
-The exponential function plays a crucial role in solving these equations as it is used to complete the product rule. It is the function that, when integrated, gives back the original function or derivative, which is necessary for the solution process.
Why is it necessary to check the work after simplifying the equation?
-Checking the work after simplifying the equation is necessary to ensure that the derivative of the exponential function matches the original equation's right-hand side, confirming that the correct steps have been taken and the solution is accurate.
What is the final form of the solution for a linear first-order differential equation?
-The final form of the solution for a linear first-order differential equation is typically an expression for y in terms of x, which includes an arbitrary constant C that can be determined using initial conditions if provided.
How does the video address the concept of integrating with respect to X?
-The video addresses the concept of integrating with respect to X by explaining that after completing the product rule and structuring the equation properly, an integral with respect to X is performed on both sides to eliminate the derivative and solve for y.
Outlines
📘 Introduction to Differential Equations Examples
The narrator begins by emphasizing the importance of practicing solving linear first-order differential equations through examples, following a theoretical explanation in a previous video. The approach is educational, aiming to build up the viewer's understanding and proficiency step by step. The focus is on ensuring that the viewer can apply a specific technique to solve equations, checking if the equations fit the technique, or if not, finding an alternative approach or making adjustments. The segment introduces the concept of using an integrating factor, a technique to solve differential equations that do not fit the separable equations category but are linear, involving a step-by-step method of finding a solution.
🔍 Detailed Walkthrough of Example Solutions
This section delves into solving a specific type of differential equation by identifying its linear nature and determining the integrating factor. The process involves checking whether the equation fits the criteria for being solved using the linear differential equation approach, then finding the 'missing piece' or integrating factor that completes the product rule. The narrative provides a detailed explanation of the technique, illustrating the importance of identifying the structure of the equation, the role of the integrating factor (represented by an exponential function), and how to apply it to simplify and solve the equation.
📝 Techniques for Solving Complex Equations
The focus shifts to more complex examples, emphasizing the application of the integrating factor in different scenarios. The narrator illustrates how to adapt the method to equations that may not initially seem to fit the linear model by manipulating them into a suitable form. This part underscores the versatility of the integrating factor technique in solving linear differential equations by multiplying both sides of the equation by an exponential function derived from the equation itself. Through this process, equations that appeared complex or unsolvable at first glance are simplified, demonstrating the power of this mathematical tool.
✅ Applying the Method to Various Equations
This segment continues to apply the integrating factor technique to a range of differential equations, showcasing its effectiveness across different examples. The narrator methodically demonstrates the process of identifying whether an equation is linear, finding the integrating factor, and applying it to simplify and ultimately solve the equation. The detailed walkthrough includes steps like checking the equation's structure, integrating to find the exponential function needed, and multiplying through to simplify the equation into a solvable form, highlighting the method's systematic and adaptable nature.
🎓 Advanced Problem Solving and Summary
The final parts of the video present more advanced problems and summarize the techniques covered. The narrator encourages viewers to apply the integrating factor method to various equations, illustrating the broad applicability and effectiveness of the technique in solving differential equations that are linear in nature. The examples become progressively more challenging, requiring careful application of the method and understanding of the underlying mathematical principles. The video concludes by reinforcing the value of practice in mastering differential equations solving techniques.
Mindmap
Keywords
💡Linear First-Order Differential Equations
💡Product Rule
💡Integration
💡Exponential Functions
💡Separable Equations
💡Technique Application
💡Derivative
💡Solving Equations
💡Initial Conditions
💡Factoring
💡Check Your Work
Highlights
The video discusses techniques for solving linear first-order differential equations.
The presenter emphasizes the importance of understanding the structure of the equations to apply the correct technique.
The concept of 'separable' equations is introduced, and the presenter explains why they may not always fit the linear technique.
The video provides a step-by-step guide on how to identify if an equation fits the linear technique and how to modify it if necessary.
The presenter demonstrates how to use the product rule to solve differential equations, including the importance of identifying the correct P(X) function.
The video includes examples of equations that do not fit the linear technique and shows alternative methods for solving them.
The presenter explains the process of integrating both sides of a differential equation with respect to X to eliminate the derivative.
The video highlights the use of exponential functions in solving differential equations, particularly when dealing with the integral of P(X).
The presenter provides a detailed explanation of how to check work by ensuring the derivative of the exponential function matches the original equation's right side.
The video discusses the concept of 'product rule' in the context of implicit differentiation and how it relates to the structure of the differential equation.
The presenter emphasizes the importance of practice and watching all examples to gain a solid understanding of solving differential equations.
The video includes a method for dealing with equations that have a first-order derivative but are not immediately separable.
The presenter explains how to handle equations with initial conditions and how to solve for the constant C.
The video concludes with a reminder that there can be more than one technique available for solving a given differential equation, and the best approach is often the one that requires the least amount of work.
Transcripts
Browse More Related Video
First Order Linear Differential Equations

Checking Solutions in Differential Equations (Differential Equations 3)

Algebra Final Exam Review

Introduction to Linear Differential Equations and Integrating Factors (Differential Equations 15)
How To Solve Systems of Nonlinear Equations

First order, Ordinary Differential Equations.
5.0 / 5 (0 votes)
Thanks for rating: