Introduction to Linear Differential Equations and Integrating Factors (Differential Equations 15)

Professor Leonard

22 Oct 201867:15

EducationalLearning

32 Likes 10 Comments

TLDRThe video delves into solving linear first-order differential equations, emphasizing the technique of finding a 'missing piece' to make the equation solvable. It explains the concept through the lens of implicit differentiation and product rules, using the exponential function as a key to unlocking the solution. The method involves identifying the function that, when differentiated, results in the given equation, and then integrating to find the solution. The video provides a step-by-step walkthrough, complete with examples, to ensure a comprehensive understanding of the process.

Takeaways

📚 The video discusses techniques for solving linear first-order differential equations, focusing on the method that involves finding a 'missing piece'.
🔍 Linear first-order differential equations are characterized by having a single 'y' to the first power, and cannot be easily separated into functions of 'x' and 'y'.
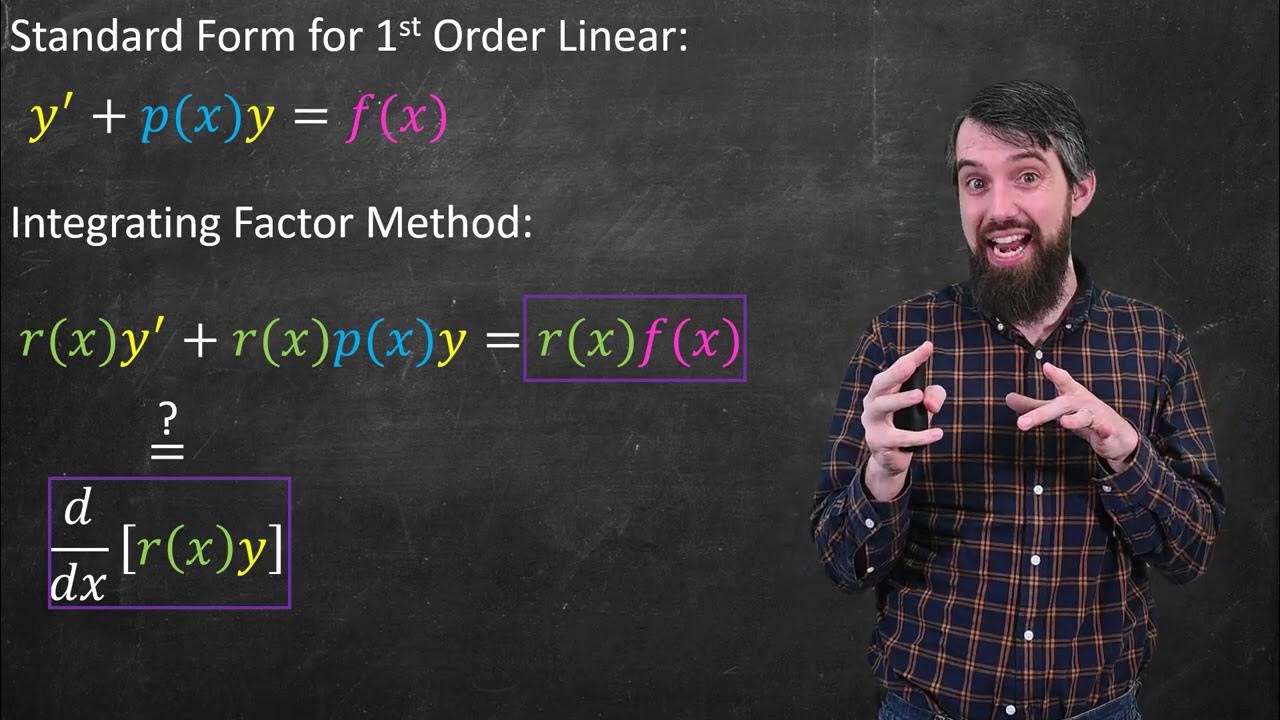
🧩 The 'missing piece' technique involves treating the left-hand side of the equation as a result of a product rule from implicit differentiation, and finding what is missing to complete the rule.
🌟 The missing piece, denoted as ρ(X), is an exponential function e^(f(X)) that repeats itself when differentiated and undifferentiated.
📈 To find ρ(X), one must ensure that its derivative gives back the original function and that P(X), the function in front of 'y', is the derivative of the exponent in ρ(X).
🤔 The process of solving the equation involves multiplying the entire equation by ρ(X) to isolate 'y' and then integrating both sides to solve for 'y'.
📝 The video provides a detailed example of solving a linear first-order differential equation using the 'missing piece' method and compares it with the separable method.
🌐 The importance of understanding the underlying concepts and the structure of the equation is emphasized for successfully applying the technique.
🎓 The video encourages viewers to not just memorize the steps, but to grasp the logic and intention behind the 'missing piece' method.
🚀 The technique is shown to be powerful and elegant, allowing for the simplification of complex equations into more manageable forms.

Q & A

What is the main topic of the video?
-The main topic of the video is solving linear first-order differential equations using a technique that involves finding a missing piece to make the equation solvable.
What is the difference between linear and separable differential equations?
-Linear differential equations involve a function of X times Y to the first power, while separable equations allow you to move all Y terms to one side and all X terms to the other, making them easier to integrate.
Why is the technique discussed in the video considered fascinating by the speaker?
-The speaker finds the technique fascinating because it involves using clever methods to solve differential equations, similar to finding the missing piece of a puzzle.
What does the speaker mean by 'finding the missing piece' in the context of solving these equations?
-Finding the missing piece refers to the process of manipulating the equation to reveal the structure of a product rule, which is a key step in solving linear first-order differential equations using this technique.
How does the speaker explain the use of e to the function of X in solving these equations?
-The speaker explains that e to the function of X is used because it repeats itself when taking or not taking the derivative, which is a requirement for the missing piece in the product rule structure of the equation.
What is the role of the function Rho of X (ρ(X)) in the explanation?
-Rho of X (ρ(X)) represents the missing piece that needs to be found and multiplied by every term in the equation to satisfy the product rule structure, which allows for the equation to be solved using integration.
How does the speaker describe the process of solving a linear first-order differential equation?
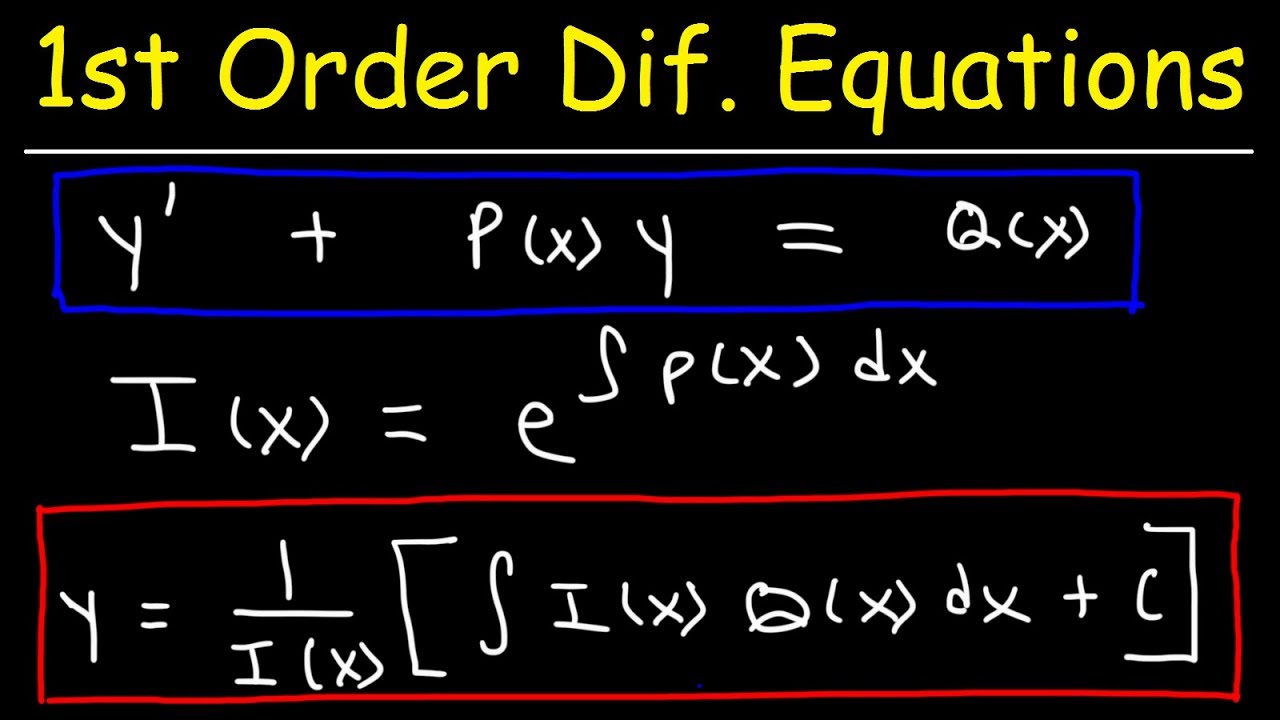
-The speaker describes the process as identifying the structure of the equation as a product rule with a missing piece, finding the missing piece (ρ(X)), multiplying every term by this missing piece, and then integrating both sides of the equation to solve for the function Y.
What is the significance of the product rule in the technique discussed?
-The product rule is significant because it provides the structure for the equation that needs to be satisfied in order to solve it. The missing piece must be found such that when multiplied, the equation fits the product rule structure, allowing for integration to solve for Y.
How does the speaker ensure the audience understands the concept?
-The speaker ensures understanding by breaking down the concept into steps, providing logical explanations, using examples, and emphasizing the reasoning behind each step rather than just the procedure.
What is the fundamental theorem of calculus mentioned in the video used for in this context?
-The fundamental theorem of calculus is used to justify the process of integrating both sides of the equation to eliminate the derivative and solve for the function Y.
What happens when you integrate the missing piece ρ(X)?
-When you integrate the missing piece ρ(X), you get back the original function that was divided away to fit the equation into the product rule structure, which is crucial for solving the equation.

Outlines

00:00

📚 Introduction to Solving Linear First-Order Differential Equations

The speaker introduces the topic of solving linear first-order differential equations, emphasizing the fascination with the techniques used. They explain that these equations involve a first-order derivative and are linear, meaning they contain only the first power of the variable y. The speaker also mentions that the method to be discussed is different from the separable equations technique and involves finding a 'missing piece' to solve the equation.

05:03

🔍 Understanding Linear Differential Equations and the 'Missing Piece'

The speaker delves deeper into the structure of linear first-order differential equations, explaining why they are not considered separable. They introduce the concept of a 'missing piece' in the equation, which is a technique used to solve these types of equations. The speaker also begins to explain the logic behind this approach, comparing it to implicit differentiation in calculus.

10:04

🧩 Finding the Missing Piece through Implicit Differentiation

The speaker continues to explain the process of finding the missing piece in the equation by using the product rule from implicit differentiation. They illustrate how the left-hand side of the equation resembles the result of a product rule and how to identify the missing piece that will complete the equation in this form. The speaker emphasizes the importance of understanding the logic behind this method.

15:05

📈 Multiplying by the Missing Piece to Solve the Equation

The speaker explains the next steps in solving the equation by multiplying both sides by the missing piece, which is a function of X. They clarify that the goal is to structure the equation to resemble the result of a product rule, with the missing piece being an exponential function. The speaker also introduces the concept of the derivative of the missing piece, which will help in finding the solution to the equation.

20:07

🌟 The Role of Exponential Functions in Solving the Equation

The speaker discusses the role of exponential functions in solving the equation. They explain that the missing piece must be an exponential function that repeats itself and that the derivative of this function must give back the original function and the missing piece itself. The speaker also emphasizes the importance of understanding the properties of derivatives and integrals in this context.

25:08

📝 Example Walkthrough: Solving a Linear Differential Equation

The speaker provides an example to demonstrate the process of solving a linear first-order differential equation. They walk through the steps of identifying the missing piece, structuring the equation, and using the product rule to find the solution. The speaker also compares this method with the separable equations technique and shows that both yield the same result.

30:09

🎓 Final Thoughts and Next Steps

The speaker concludes the discussion by reiterating the importance of understanding the underlying concepts and logic behind solving linear first-order differential equations. They encourage the audience to practice the technique and look forward to providing more examples in the next video.

Mindmap

Keywords

💡Differential Equations

Differential equations are mathematical equations that relate a function with its derivatives. In the context of the video, the focus is on ordinary differential equations (ODEs), specifically linear first-order ODEs. These equations are fundamental in many areas of science and engineering and involve finding a function that satisfies the given equation.

💡Ordinary Differential Equations (ODEs)

ODEs involve functions of a single independent variable and their derivatives. The video script emphasizes the importance of understanding and solving ODEs, particularly linear first-order equations, which are crucial in modeling various phenomena in physics and engineering.

💡Linear First-Order Differential Equations

These are a class of differential equations where the unknown function and its first derivative are both to the first power and appear in a linear combination. The video script explains that these equations cannot be solved by simple separation of variables but require a specific technique involving the concept of a missing piece.

💡Separable Equations

Separable equations are a type of first-order differential equation that can be solved by separating the variables on opposite sides of the equation and then integrating. The video script contrasts these with linear first-order equations, which often cannot be solved using this method.

💡Product Rule

The product rule is a fundamental calculus concept used to find the derivative of a product of two functions. In the video, the product rule is implicitly applied when discussing how to handle the 'missing piece' in linear first-order differential equations.

💡Implicit Differentiation

Implicit differentiation is a method used to find the derivative of an equation when the relationship between variables is not explicitly expressed. The video script uses the concept of implicit differentiation to explain how to treat the left-hand side of a linear first-order differential equation as if it were the result of a product rule with a missing component.

💡Exponential Functions

Exponential functions are mathematical functions of the form e^x, where e is the base of the natural logarithm. The video script emphasizes the importance of exponential functions in solving linear first-order differential equations, particularly when they are part of the 'missing piece' that needs to be identified.

💡Integration

Integration is the process of finding the function whose derivative is a given function, essentially the reverse of differentiation. In the video, integration is used to solve differential equations by eliminating the derivative term through the fundamental theorem of calculus.

💡Fundamental Theorem of Calculus

The fundamental theorem of calculus states that if a function is continuous on a closed interval and has a derivative that is integrable on that interval, then the function can be expressed as the integral of its derivative plus a constant. This theorem is crucial in solving differential equations, as it allows the transformation of derivatives into integrals.

💡Arbitrary Constant

An arbitrary constant is a constant that can take any value in the set of real numbers. In the context of solving differential equations, it is included to account for the fact that the antiderivative (or indefinite integral) of a function is not unique; it can be shifted vertically by any constant value.

Highlights

The video introduces techniques for solving linear first-order differential equations.

Linear first-order differential equations are defined and contrasted with separable equations.

The concept of a 'missing piece' in linear equations is introduced as a key to solving them.

The video explains how to identify and work with the 'missing piece' in linear equations.

An example is provided to demonstrate the process of solving a linear first-order differential equation.

The importance of understanding the underlying logic behind the technique is emphasized.

The video discusses the product rule's role in solving linear first-order differential equations.

The process of finding the exponential function (e to the power of X) as the 'missing piece' is detailed.

The video clarifies the conditions under which the 'missing piece' must repeat itself when taking derivatives.

The significance of the derivative of the exponential function in finding the 'missing piece' is explained.

The video demonstrates how to multiply the equation by the 'missing piece' to return it to a product rule form.

The process of integrating both sides of the equation to solve for the function Y is outlined.

The video shows how to handle the constant term when integrating and solving for Y.

The video concludes by reiterating the importance of understanding the concepts behind the technique for solving linear equations.

The video promises to provide more complex examples in future content to further illustrate the technique.

Transcripts

Browse More Related Video

Linear Differential Equations & the Method of Integrating Factors

Introduction to Initial Value Problems (Differential Equations 4)

Integrating Factor for Exact Differential Equations (Differential Equations 30)

Implicit Differentiation

Initial Value Problems!

First Order Linear Differential Equations

Related Tags

Differential Equations Mathematics Linear Equations Product Rule Exponential Functions First-Order Educational Content Math Tutorial Calculus Concepts Problem Solving