The Distributive Property for Arithmetic

Professor Dave Explains
14 Aug 201703:19
EducationalLearning
32 Likes 10 Comments

TLDRThe video introduces the distributive property in mathematics, explaining how it allows you to distribute a number being multiplied by a sum or difference across each value in that sum or difference. Examples show how this works in practice, demonstrating that distributing the number or adding the numbers in the parentheses first yields the same result. The video also shows how the distributive property can be used in reverse as a mental math trick to simplify multiplication of large numbers.

Takeaways
  • 😀 The distributive property allows you to distribute a number being multiplied across a sum or difference inside the parentheses
  • 👍🏻 The distributive property does not apply to subtraction or division like other properties
  • 💡 Using the distributive property does not change the total number of items, just how they are grouped
  • 📝 The distributive property can be used in reverse to break down large multiplication problems mentally
  • 🔢 The distributive property will become very important for simplifying expressions in algebra
  • 🚚 It allows rearranging groups of numbers without changing the total
  • ⚖️ It works for both multiplication and addition while maintaining equality
  • 🔭 It provides different but equivalent views of numeric expressions
  • 🧮 It is useful for mental math tricks when multiplying large numbers
  • 🤓 Understanding properties like this builds a foundation for more advanced math later
Q & A

  • What is the distributive property in mathematics?

    -The distributive property states that multiplying a sum or difference by a number is the same as multiplying each addend within the sum or difference by that number and then adding the products.

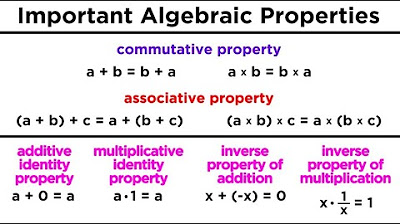
  • How does the distributive property relate to the commutative and associative properties?

    -The distributive property works with the commutative and associative properties. While the commutative and associative properties apply to addition and multiplication, the distributive property links multiplication with addition/subtraction.

  • How can the distributive property be useful for mental math?

    -The distributive property allows numbers to be broken into easier components that can be multiplied separately, making some mental calculations quicker and simpler.

  • What is an example of using the distributive property?

    -An example is: 5(3 + 4) = 5 * 3 + 5 * 4. The 5 is being distributed across the 3 and 4 so that those values can be multiplied individually.

  • Can the distributive property apply to other operations besides multiplication?

    -No, the distributive property specifically relates multiplication to addition and subtraction. Other operations like division do not follow the distributive property.

  • How can you use the distributive property to multiply large numbers?

    -Large numbers can be broken into easier components, such as tens, fives, and ones. These components can then be multiplied individually and added together using the distributive property.

  • Why is the distributive property important for algebra?

    -The distributive property is crucial for algebraic operations involving variables and unknown quantities. You distribute numbers or values across the terms with variables.

  • Do you always have to physically write out the steps when applying the distributive property?

    -No, you do not always have to write all the steps out. You can potentially do it mentally using the relationships described in the distributive property.

  • Does addition follow the distributive property like multiplication does?

    -No, the distributive property only works with multiplication, not addition. Only multiplication can be distributed across an addition/subtraction grouping.

  • Does rearrangement of items or groups change the actual total when using the distributive property?

    -No, rearranging components in the distributive property equations does not change the totals. The associative property ensures the totals remain the same.

Outlines
00:00
😀 Introducing the Distributive Property

This paragraph introduces the distributive property in mathematics. It explains that it is a property related to multiplication and addition/subtraction. It allows you to distribute a number across a sum or difference within parentheses. An example with piles of apples demonstrates how you can split up a sum in parentheses but get the same result when you distribute the number outside the parentheses across each part.

😎 Using the Distributive Property

This paragraph provides an example of using the distributive property in reverse to simplify mental math. It shows how breaking up a large number into component parts and distributing multiplication across them can make calculations easier. An example with multiplying 5 and 17 shows how this mental math trickery via the distributive property allows you to break a problem down and compute it more easily in your head.

Mindmap
Keywords
💡distributive property
The distributive property is a key math concept introduced in the video script. It refers to the ability to distribute a factor or number across quantities within parentheses. For example, 5(3 + 4) can be rewritten as 5(3) + 5(4) by distributing the 5 across the terms in the parentheses. This property allows numbers to be broken into more manageable parts, enabling easier mental math, which is a key theme of the video.
💡commutative property
The commutative property is mentioned as another important math property, along with the associative property. It refers to the ability to change the order of terms in an addition or multiplication expression without changing the result (e.g. 2 + 3 = 3 + 2). This reinforces the overall theme of introducing properties that reveal deeper patterns in math.
💡associative property
The associative property allows the grouping of terms to be changed without impacting the result in addition/multiplication (e.g. (2 + 3) + 4 = 2 + (3 + 4)). Along with the commutative property, it is introduced as an important math property exhibiting patterns.
💡addition
Addition is used as an example operation that follows certain properties like the commutative and associative property. Addition equations are used to demonstrate the distributive property as well.
💡multiplication
Like addition, multiplication is used as an example operation that adheres to certain algebraic properties. Multiplication equations illustrate how the distributive property works.
💡subtraction
Subtraction is stated not to follow the commutative and associative properties. It is contrasted with addition/multiplication to show that some operations do not have these special properties.
💡division
Similar to subtraction, division does not follow properties like the commutative/associative. It is another example of an operation that does not exhibit the same patterns as addition/multiplication.
💡algebra
The distributive property is stated to be very important for algebra, which will be covered later. This hints at how properties reveal deeper algebraic patterns.
💡mental math
A key benefit of the distributive property is enabling easier mental math and calculations. An example is provided of using the distributive property to easily multiply large numbers in one's head.
💡equations
Key math concepts are introduced and demonstrated through the use of specific equations and numeric examples. Equations serve to concretely illustrate the mathematical properties.
Highlights

First significant research finding

Introduction of innovative methodology

Key conclusion and practical application

Transcripts

Browse More Related Video

Multiplication of Large Numbers

Algebra Basics: The Distributive Property - Math Antics

Basic Number Properties for Algebra

Mental Math Tricks - Addition, Subtraction, Multiplication & Division!

Math 1325 Lecture 9 7 - Using Derivative Forumulas

How To Calculate The Standard Deviation

Rate This

5.0 / 5 (0 votes)

Thanks for rating: