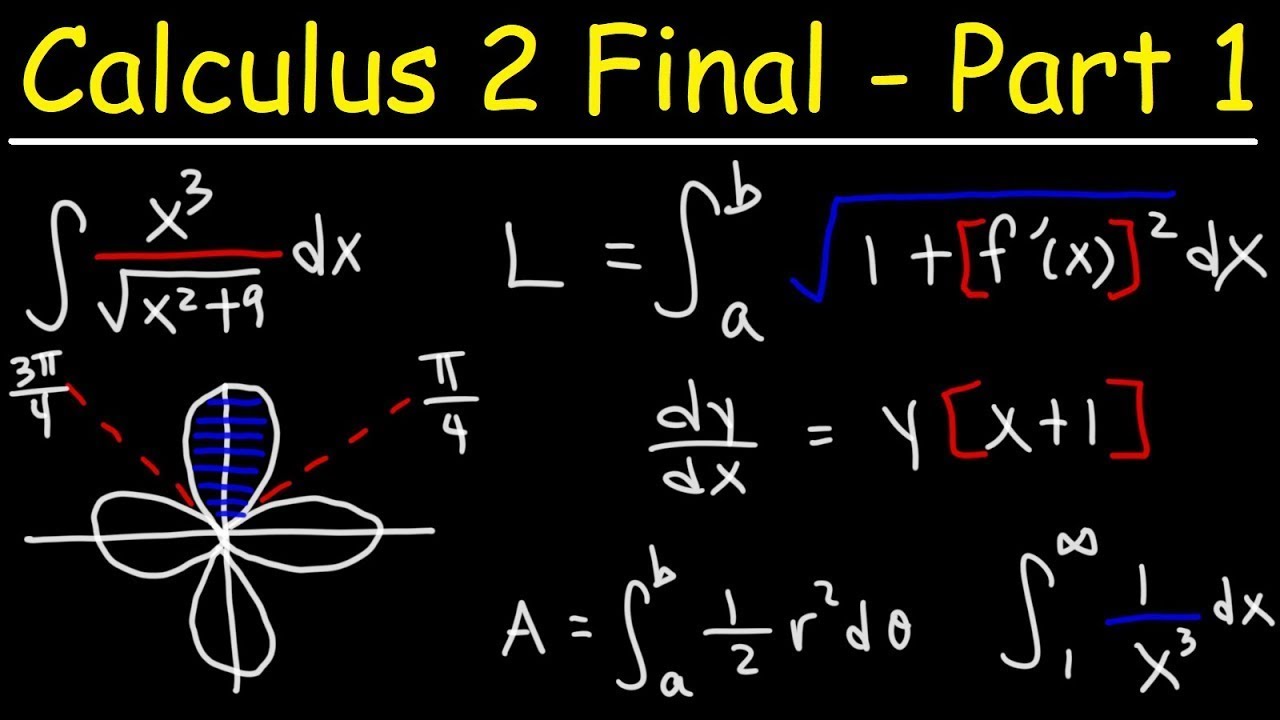
Integration by Completing the Square
TLDRThis video concludes the discussion on integration techniques by introducing integration by completing the square. It also revisits and contrasts various integrals, including partial fractions and improper integrals. The instructor emphasizes the importance of recognizing when to apply different techniques, such as algebraic manipulation, u-substitution, and integration by parts.
Takeaways
- 📚 The video concludes the discussion on techniques of integration in an AP Calculus course.
- 🔍 The new technique introduced is 'integration by completing the square'.
- 📈 The video compares and contrasts different integrals, including some that haven't been discussed in a while.
- 📝 The script reviews various integration techniques such as substitution (u-substitution), rearrangements, and completing the square.
- 📐 It mentions the use of geometry in integration, especially when dealing with equations that represent shapes.
- 📉 The video explains how to handle integrals with rational functions by decomposing them into partial fractions.
- 🚫 It clarifies that improper integrals, which have infinite limits or vertical asymptotes, are dealt with by using the limit of a definite integral.
- 🔄 The process of 'integration by completing the square' is demonstrated with examples that are one off from a perfect square in the denominator.
- 📌 The video emphasizes the importance of recognizing patterns in integrals that suggest the use of specific integration techniques.
- ✅ The script provides practice examples for students to apply the techniques discussed, including those involving completing the square.
- 📚 The summary at the end lists all the integration techniques covered in the course, preparing students for the AP exam.
Q & A
What is the main focus of the video?
-The main focus of the video is to discuss techniques of integration, with a particular emphasis on 'integration by completing the square' and comparing it with other integration techniques.
What is the AP Calculus course description mentioned in the video?
-The AP Calculus course description includes various techniques for finding antiderivatives such as substitution of variables, rearrangements into equivalent forms, completing the square, integration by parts, and dealing with rational functions and improper integrals.
What is the significance of the technique 'integration by completing the square'?
-Integration by completing the square is significant as it is a new technique introduced in the video, which allows for the integration of functions that have a quadratic expression in the denominator that is close to a perfect square.
How does the video compare different integration techniques?
-The video compares different integration techniques by providing examples of integrals that can be solved using various methods, including partial fraction decomposition, power rule application, and the new technique of completing the square.
What is the role of 'u-substitution' in integration?
-U-substitution is a technique for finding antiderivatives where a substitution of variables is made to simplify the integral into a form that is easier to integrate. It's also mentioned that if running a definite integral with u-substitution, the bounds need to be changed accordingly.
How is the video structured in terms of teaching the new technique?
-The video is structured by first introducing the new technique of 'integration by completing the square', then providing three examples to illustrate its application, and finally comparing and contrasting it with other integration techniques.
What is the difference between 'integration by parts' and 'integration by completing the square'?
-Integration by parts is a technique used for integrating products of functions (udv), while integration by completing the square is used for integrals with a quadratic expression in the denominator that is close to a perfect square, allowing for a simpler form of integration.
How does the video handle the topic of improper integrals?
-The video explains that improper integrals, which have limits at infinity or an integrand with a vertical asymptote, can be dealt with by using the limit of a definite integral.
What is the significance of the geometric approach to integration mentioned in the video?
-The geometric approach to integration is significant as it allows for the solution of integrals by recognizing geometric shapes or patterns, such as the upper half of a circle, and applying geometric formulas to find the area under the curve.
What are some examples of integrals that can be solved using the technique of 'integration by completing the square'?
-Examples of integrals that can be solved using 'integration by completing the square' include those where the denominator is a quadratic expression that is one more or less than a perfect square, such as 1/(x^2 - 10x + 25) or 1/(x^2 - 4x + 5).
Why might the video suggest that certain types of integrals are less likely to appear on the AP exam?
-The video suggests that certain types of integrals, such as those requiring heavy-duty algebra or multiple steps of u-substitution, are less likely to appear on the AP exam because they are more complex and time-consuming, and the exam tends to focus on more straightforward applications of integration techniques.
Outlines
📚 Introduction to Integration Techniques
The video begins with an overview of integration techniques, focusing on wrapping up the discussion and introducing a new method called 'integration by completing the square.' The instructor also plans to compare and contrast various integrals, including some that haven't been covered recently. The AP calculus course description is reviewed, highlighting techniques such as substitution of variables, rearrangements into equivalent forms, integration by parts, and dealing with rational functions and improper integrals. The video aims to refresh and integrate all these concepts, starting with three examples to demonstrate the new technique and comparing it with existing methods.
🔍 Completing the Square in Integration
This paragraph delves into the specifics of 'integration by completing the square,' a technique used when the integral's denominator is close to but not exactly a perfect square. The instructor provides examples where the denominator is off by one from a perfect square, illustrating how to recognize and apply this method. The video also touches on the use of geometry in solving integrals, particularly when the integral involves the square root of a squared term, and how to approach integrals that don't factor neatly, such as those requiring trigonometric substitution or more complex algebraic manipulation.
📐 Advanced Algebraic Manipulation in Integration
The instructor discusses a more complex scenario involving integrals that require significant algebraic manipulation, such as factoring out constants and using properties of radicals to transform the integral into a recognizable form. The paragraph includes an example where the integral is manipulated to resemble a known form, allowing the use of u-substitution to solve it. The video emphasizes the importance of recognizing patterns and applying the correct algebraic techniques to simplify the integration process, even though it might be more algebra-intensive than other methods.
📝 Summary of Integration Techniques for AP Calculus BC
The final paragraph provides a comprehensive summary of all the integration techniques covered in the video, suitable for the AP Calculus BC curriculum. It includes geometry-based integration, algebraic manipulation, u-substitution, integration by parts, partial fractions, and completing the square. The instructor reassures viewers that all necessary techniques have been covered and encourages them to practice applying these methods by using provided worksheets and materials. The video concludes with an offer of additional practice materials for those who need them, emphasizing the importance of knowing when to use each technique.
Mindmap
Keywords
💡Integration
💡U-substitution
💡Partial Fractions
💡Improper Integral
💡Completing the Square
💡Antiderivatives
💡Integration by Parts
💡Rational Functions
💡Trigonometric Integrals
💡Algebraic Manipulation
💡Geometry
Highlights
Introduction to integration by completing the square and comparison with other integration techniques.
Review of AP Calculus course description, focusing on integration and techniques of integration.
Explanation of substitution of variables (u-substitution) for finding antiderivatives.
Discussion of rearrangements into equivalent forms using algebraic tricks.
Application of long division and synthetic division for integration.
Introduction to completing the square as a new integration technique.
Comparison of integrals and the use of partial fractions for distinct factors.
Explanation of factoring and rewriting integrals for easier antiderivation.
Step-by-step process of completing the square for integration.
Recognition of integrals that require completing the square by comparing to near-perfect squares.
Use of geometry and circles to solve integrals involving square roots.
Detailed algebraic manipulation for integrating functions with complex denominators.
Review of common integration techniques: geometry, algebraic manipulation, u-substitution, integration by parts, partial fractions, long division, and completing the square.
Encouragement to practice different techniques and recognize which to use in various scenarios.
Availability of additional practice materials and worksheets for further study.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: