Keq derivation intuition | Chemical equilibrium | Chemistry | Khan Academy

Khan Academy
8 Sept 200915:49
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the derivation of the equilibrium constant formula through the Haber process, illustrating how reaction rates and probabilities relate to concentrations. It uses real numbers and a hypothetical small volume to explain how the forward and reverse reaction rates become equal at equilibrium, ultimately leading to the equilibrium constant formula.

Takeaways
  • 🔬 The script discusses the derivation of the equilibrium constant formula, focusing on the relationship between probabilities and concentrations.
  • 📚 The video aims to clarify the intuition behind the equilibrium constant formula, which was previously explained in a 'hand wavy' manner.
  • 🌟 The Haber process for producing ammonia is used as a real-world example to illustrate the concept of equilibrium in chemical reactions.
  • ⚖️ Equilibrium does not imply equal concentrations of reactants and products; it means that the rates of the forward and reverse reactions are the same.
  • 🧪 The concept of a 'small volume' (dV) is introduced to discuss the probabilities of reactions occurring within this space.
  • 🎲 The probability of a forward reaction is related to the concentration of reactants and a constant probability of reaction given their presence in the box.
  • 📏 The size of the 'box' (dV) is considered important, with an example given using the diameter of an ammonia molecule to estimate its volume.
  • 📉 The concentration of molecules in the box is calculated by scaling the overall concentration with the volume of the box.
  • 🔄 At equilibrium, the probability of the forward reaction is equal to the probability of the reverse reaction within the small volume.
  • 📝 The equilibrium constant is derived from setting the probabilities of the forward and reverse reactions equal to each other.
  • 🌡️ The equilibrium constant is temperature-dependent, as illustrated with the Haber process occurring at 300 degrees Celsius.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is the explanation of the derivation of the equilibrium constant formula, using the Haber process for ammonia production as an example.

  • What is the Haber process mentioned in the script?

    -The Haber process is an industrial method for the production of ammonia from nitrogen and hydrogen gases, which is crucial for the manufacture of fertilizers.

  • Why is the concept of equilibrium important in the context of the Haber process?

    -Equilibrium is important because it indicates the state where the rate of the forward reaction (producing ammonia) is equal to the rate of the reverse reaction (breaking down ammonia into nitrogen and hydrogen), allowing for a steady production of ammonia.

  • What does the script suggest about the relationship between probabilities and concentrations in chemical reactions?

    -The script suggests that the probability of a reaction occurring in a given volume is related to the concentrations of the reactants and that these probabilities can be used to derive the equilibrium constant formula.

  • How does the script describe the concept of 'dV' in the context of chemical reactions?

    -In the script, 'dV' is described as a small volume within a solution where the likelihood of a reaction occurring is considered, which is used to calculate the probability of reactants interacting.

  • What is the significance of the equilibrium constant in the script?

    -The equilibrium constant is significant as it is a measure of the extent to which a reaction proceeds before reaching equilibrium at a given temperature, and it is derived from the probabilities of forward and reverse reactions.

  • How does the script relate the probability of a reaction to the concentrations of reactants?

    -The script relates the probability of a reaction to the concentrations of reactants by suggesting that the probability of a reaction occurring in a small volume is proportional to the product of the concentrations of the reactants.

  • What is the role of temperature in the equilibrium constant as discussed in the script?

    -The role of temperature in the equilibrium constant is that it is a fixed parameter at which the equilibrium constant holds, and it affects the rate and probability of reactions.

  • What is the practical significance of understanding the equilibrium constant in industrial processes like the Haber process?

    -Understanding the equilibrium constant is crucial in industrial processes as it helps in optimizing the conditions for maximum yield of the desired product, such as ammonia in the Haber process.

  • How does the script use the concept of probabilities to derive the formula for the equilibrium constant?

    -The script uses the concept of probabilities by equating the probability of the forward reaction to the probability of the reverse reaction at equilibrium and then setting up an equation that relates these probabilities to the concentrations of the reactants and products, leading to the derivation of the equilibrium constant formula.

Outlines
00:00
🧪 Derivation of Equilibrium Constant from Probabilities

The script introduces the concept of deriving the equilibrium constant formula by relating it to the probabilities of molecular interactions within a small volume. The author revisits the topic after realizing that a previous attempt lacked clarity. The Haber process, a method for producing ammonia, is used as a real-world example to illustrate the concept of equilibrium concentrations and reaction rates. The author emphasizes that equilibrium does not imply equal concentrations but equal rates of forward and reverse reactions.

05:01
📏 Estimating Molecular Probabilities in a Defined Volume

The script delves into the probability of a reaction occurring within a small volume, denoted as dV. It uses the Haber process to explain how the probability of the forward reaction is calculated based on the concentrations of reactants and the volume of the reaction space. The author provides a detailed mathematical explanation of how to determine the probability of having a certain number of reactant molecules in the defined volume, using the concentration of hydrogen as an example and scaling it to the chosen volume size.

10:04
🔄 Calculating Forward and Reverse Reaction Probabilities

The script continues by discussing the calculation of the probability for both the forward and reverse reactions. It explains that these probabilities are determined by the product of the concentrations of the reactants and a constant that represents the likelihood of a reaction given the molecules are in the same volume. The author illustrates this with the example of hydrogen and nitrogen reacting to form ammonia, and then the reverse reaction of ammonia decomposing back into hydrogen and nitrogen.

15:06
🌡️ Equilibrium Constant as a Ratio of Reaction Probabilities

The final paragraph synthesizes the previous discussions by equating the probabilities of the forward and reverse reactions at equilibrium. It shows that the equilibrium constant is the ratio of these probabilities, which can be expressed in terms of the concentrations of the reactants raised to their respective stoichiometric coefficients. The author concludes by presenting a pseudo-derived formula for the equilibrium constant, emphasizing that it is based on the common-sense understanding of reaction probabilities within a small volume.

Mindmap
Keywords
💡Equilibrium
Equilibrium in the context of the video refers to a state in a chemical reaction where the rate of the forward reaction equals the rate of the reverse reaction, resulting in constant concentrations of reactants and products. This concept is central to the video as it explains how the equilibrium constant formula is derived. For example, the script mentions that 'the Haber process is in equilibrium, which doesn't mean that the concentrations are the same', illustrating the dynamic balance between the formation and decomposition of ammonia.
💡Haber Process
The Haber Process is a method used industrially to synthesize ammonia from nitrogen and hydrogen gases. It is highlighted in the video as an example to explain the concept of chemical equilibrium. The script states, 'This is how we get ammonia in the world and feed everyone', emphasizing its importance in producing fertilizers. The process is used to demonstrate how equilibrium concentrations are achieved and how the equilibrium constant is related to reaction rates.
💡Concentration
Concentration in chemistry is the measure of the amount of a substance contained in a given volume. It is a key factor in understanding chemical equilibrium as it influences the rate of reactions. The video script discusses how different concentrations of nitrogen, hydrogen, and ammonia are involved in the Haber Process, with the equilibrium concentrations being crucial for the derivation of the equilibrium constant formula.
💡Probability
Probability, as used in the video, refers to the likelihood of a particular event occurring, such as a chemical reaction. The script explores how the probability of reactants colliding and reacting in a small volume (dV) is related to their concentrations. It is used to illustrate the concept that 'the probability of going in that direction to 2NH3 in our little box, I claim... it's equal to some constant related to their concentration', showing how reaction rates are influenced by the chance of reactant molecules meeting.
💡Reaction Rate
Reaction rate is the speed at which a chemical reaction proceeds. It is a fundamental concept in the video as it is directly related to the equilibrium state. The script explains that at equilibrium, 'the rate of reaction of going in the rightward direction is the same as the rate of reaction of going in the leftward direction', highlighting how reaction rates determine whether a system is at equilibrium.
💡Stoichiometry
Stoichiometry is the quantitative relationship between reactants and products in a chemical reaction. It is essential in the video for understanding how the equilibrium constant formula is derived. The script mentions the stoichiometric coefficients of the reactants and products in the Haber Process, such as 'N2 plus 3H2', which are crucial for calculating the equilibrium constant.
💡Equilibrium Constant
The equilibrium constant is a measure of the extent to which a reaction proceeds at equilibrium. It is a central concept in the video, derived from the probabilities of forward and reverse reactions. The script explains that 'KF/KR is equal to the concentration of ammonia to the second power, divided by the concentration of hydrogen to the third power, times the concentration of nitrogen', demonstrating how the equilibrium constant relates to the concentrations of reactants and products.
💡Volume (dV)
In the video, volume (dV) refers to a small, arbitrary volume in which the likelihood of a chemical reaction occurring is considered. The script uses dV to illustrate how probabilities of reactions are calculated, stating 'if you pick some small volume in this solution... that in this volume, you're just as likely to have a reaction going into that direction as you are to have a reaction going in the backwards direction', emphasizing the spatial context in which reactions occur.
💡Scaling Factor
Scaling factor in the video is a term used to describe the adjustment made to concentration values to fit the volume of interest (dV). The script discusses how the concentration of reactants is scaled according to the chosen volume, explaining 'this was just equal to the concentration of hydrogen times some scaling factor', to determine the probability of reactants being present in a specific volume.
💡Kinetic Energy
Kinetic energy is the energy possessed by an object due to its motion. In the context of the video, it is mentioned in relation to the probability of reactant molecules colliding and reacting. The script suggests that 'there's some probability that they're going to react based on their configurations and their kinetic energy and how they're approaching each other', indicating that the energy of molecules influences their likelihood of reacting.
Highlights

Attempt to provide intuition behind the derivation of the equilibrium constant formula.

Exploration of the relationship between probabilities and concentrations in chemical reactions.

Introduction of the Haber process as a real-world example of a chemical equilibrium.

Explanation of how equilibrium concentrations do not imply equal concentrations.

Discussion on the concept of reaction rates and their equality at equilibrium.

Illustration of the probability of reactions occurring in a small volume (dV).

Use of the Haber process to demonstrate the calculation of reaction probabilities.

Introduction of a constant probability for reactions given certain conditions.

Calculation of the probability of having constituent particles in a given volume.

Estimation of the volume (dV) based on the diameter of an ammonia molecule.

Conversion of molar concentrations to the probability of finding molecules in a small volume.

Derivation of the forward reaction probability in terms of reactant concentrations.

Application of the same logic to calculate the reverse reaction probability.

Equating the probabilities of forward and reverse reactions at equilibrium.

Derivation of the equilibrium constant formula from reaction probabilities.

Explanation of how the equilibrium constant is dependent on temperature.

Final expression of the equilibrium constant in terms of reactant and product concentrations.

Transcripts

Browse More Related Video

Keq intuition | Chemical equilibrium | Chemistry | Khan Academy

What Is Equilibrium? AP Chemistry Unit 7, Topic 1 Daily Video

Le Chatelier's principle | Chemical equilibrium | Chemistry | Khan Academy

Chemical Equilibrium Constant K - Ice Tables - Kp and Kc

Chemical Equilibria and Reaction Quotients

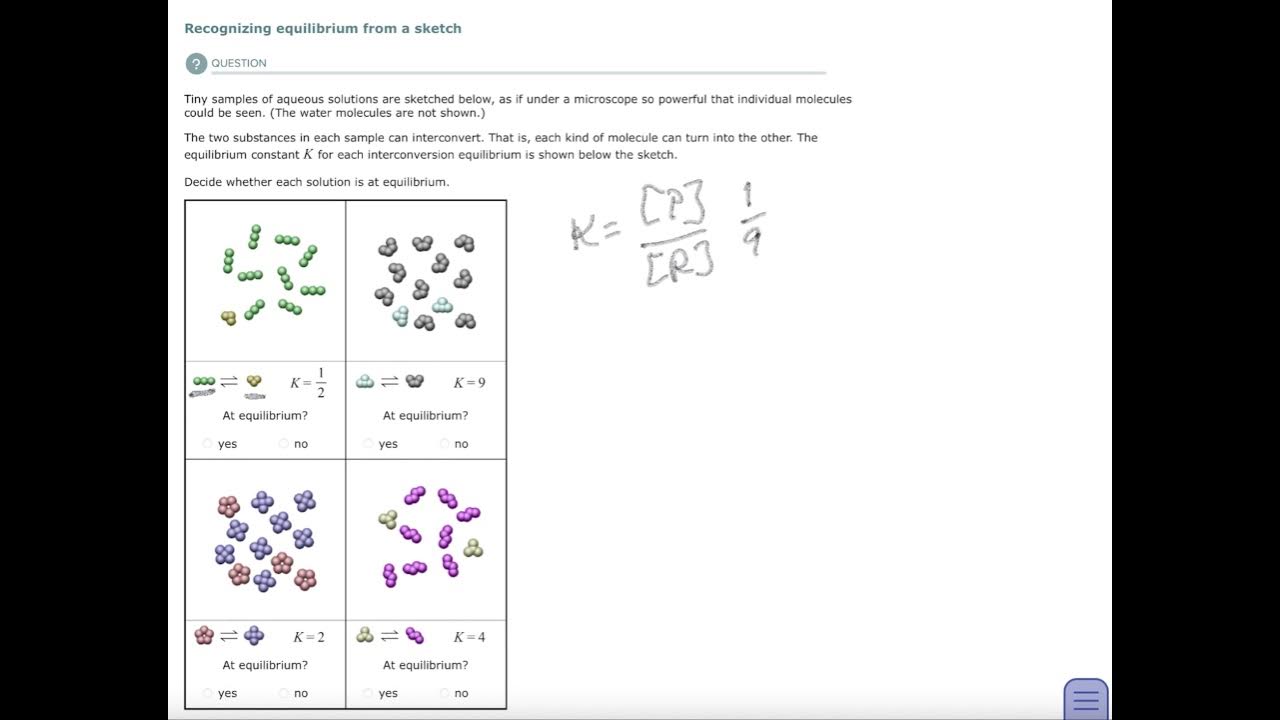
Aleks Recognizing equilibrium from a sketch

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Equilibrium ConstantHaber ProcessAmmonia ProductionChemical ReactionMolecular ProbabilitiesChemistry TutorialEducational ContentScience VideoReaction RatesConcentration Analysis