Calculating conditional probability | Probability and Statistics | Khan Academy

Khan Academy
9 Apr 201406:42
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of dependent probabilities using Rahul's food preferences as an example. It explains that the probability of Rahul eating a bagel for breakfast (A) is 0.6, and eating pizza for lunch (B) is 0.5. The script highlights that A and B are dependent events because the probability of A given B (0.7) differs from the probability of A alone. The video guides viewers through calculating the conditional probability of B given A, revealing it to be approximately 0.58, thus confirming the dependency between the events.

Takeaways
  • 🍕 Rahul's favorite foods are bagels and pizza.
  • 🥯 Event A represents Rahul eating a bagel for breakfast, with a probability of 0.6.
  • 🍕 Event B represents Rahul eating pizza for lunch, with a probability of 0.5.
  • 🤔 The conditional probability of A given B (eating a bagel given he eats pizza for lunch) is 0.7, indicating dependence between the events.
  • 🔄 Dependent events are identified when the probability of one event changes based on the occurrence of another.
  • 🧩 To find the probability of B given A, we need to understand the relationship between the events.
  • 📚 The probability of A and B happening together can be calculated using the formula P(A and B) = P(A | B) * P(B).
  • 📉 Knowing P(A | B) and P(B), we can deduce P(A and B) = 0.35.
  • 🔍 The probability of B given A can be found by rearranging the formula to P(B | A) = P(A and B) / P(A).
  • 📌 Solving for P(B | A) gives us approximately 0.58, showing that the probability of eating pizza increases if he eats a bagel for breakfast.
  • 🔄 The final result confirms the dependency between eating a bagel and pizza, as P(B | A) is higher than P(B) alone.
Q & A

  • What are Rahul's two favorite foods mentioned in the script?

    -Rahul's two favorite foods are bagels and pizza.

  • What does event A represent in the script?

    -Event A represents the event that Rahul eats a bagel for breakfast.

  • What does event B represent in the script?

    -Event B represents the event that Rahul eats pizza for lunch.

  • What is the probability of event A (Rahul eating a bagel for breakfast)?

    -The probability of event A is 0.6.

  • What is the probability of event B (Rahul eating pizza for lunch)?

    -The probability of event B is 0.5.

  • What is the conditional probability of event A given event B?

    -The conditional probability of event A given event B is 0.7.

  • Why does the conditional probability of A given B being different from the probability of A alone indicate that the events are dependent?

    -The fact that the probability of A given B is different from the probability of A alone shows that the occurrence of B influences the probability of A, indicating that the events are dependent.

  • What is the probability of both A and B happening?

    -The probability of both A and B happening is 0.35, which is calculated as the product of the probability of A given B (0.7) and the probability of B (0.5).

  • What is the probability of event B given event A, and how is it calculated?

    -The probability of event B given event A is approximately 0.58. It is calculated by dividing the probability of both A and B happening (0.35) by the probability of A (0.6).

  • What does the result of the probability of B given A being higher than the probability of B alone imply?

    -The result implies that the probability of Rahul eating pizza for lunch is higher when you know he has already eaten a bagel for breakfast, indicating that the events are dependent.

  • How does the script demonstrate the concept of dependent events?

    -The script demonstrates the concept of dependent events by showing that the probability of A (eating a bagel for breakfast) given B (eating pizza for lunch) is different from the probability of A alone, and that the probability of B given A is different from the probability of B alone.

Outlines
00:00
🍕 Understanding Dependent Probabilities

This paragraph introduces the concept of dependent probabilities using Rahul's eating habits as an example. Events A (Rahul eating a bagel for breakfast) and B (Rahul eating pizza for lunch) are defined, with their individual probabilities given as 0.6 and 0.5, respectively. The conditional probability of A given B is stated as 0.7, indicating that knowing Rahul has pizza for lunch changes the likelihood of him having a bagel for breakfast. This highlights the dependency between the events. The main task is to calculate the probability of B given A, which is approached by understanding the relationship between the joint probability of A and B and their individual probabilities.

05:00
🔢 Calculating Conditional Probability

The second paragraph delves into the calculation of the conditional probability of B given A. It explains that the probability of both A and B occurring can be represented as the product of the probability of A given B and the probability of B, or alternatively, as the product of the probability of B given A and the probability of A. Using the known values (probability of A given B as 0.7, probability of B as 0.5, and probability of A as 0.6), the joint probability of A and B is calculated to be 0.35. The paragraph then sets up an equation to solve for the probability of B given A, which is found by dividing the joint probability by the probability of A. The final result, rounded to the nearest hundredth, is 0.58, confirming the dependency between the events and showing that the likelihood of Rahul eating pizza increases if he has already eaten a bagel.

Mindmap
Keywords
💡Bagels
Bagels are a type of bread product, typically round, that is boiled and then baked. In the context of the video, bagels are one of Rahul's favorite foods, and the script discusses the probability of him eating a bagel for breakfast. This is used to introduce the concept of probability and dependent events in a relatable way.
💡Pizza
Pizza is a popular dish consisting of a yeasted flatbread topped with tomato sauce, cheese, and various other ingredients. In the video, pizza is mentioned as another favorite food of Rahul, and the probability of him eating pizza for lunch is discussed. This serves as another variable in the probability calculations that the video explores.
💡Probability
Probability is a measure of the likelihood that an event will occur. In the video, the probability of Rahul eating a bagel for breakfast and a pizza for lunch is calculated. The script uses these probabilities to illustrate concepts such as dependent and independent events, which are central to the video's theme of understanding probability.
💡Event A
In the script, Event A is defined as Rahul eating a bagel for breakfast. This is a specific event used to demonstrate how probabilities can be calculated and how they can change based on other events, such as eating pizza for lunch (Event B). Event A is a key component in the video's exploration of dependent probabilities.
💡Event B
Event B refers to Rahul eating pizza for lunch. Similar to Event A, Event B is used in the video to show how the probability of one event can affect another. The script discusses how the occurrence of Event B changes the probability of Event A, highlighting the concept of dependent events.
💡Conditional Probability
Conditional probability is the probability of an event given that another event has occurred. In the video, the conditional probability of Event A (eating a bagel for breakfast) given that Event B (eating pizza for lunch) has occurred is calculated. This is a central concept in the video, demonstrating how the occurrence of one event can change the likelihood of another.
💡Independence
Independence in probability theory refers to events that do not affect each other's likelihood of occurring. The video script discusses how the probability of Event A differs from the conditional probability of Event A given Event B, indicating that these events are not independent. This is a crucial point in understanding the relationship between the two events.
💡Dependent Events
Dependent events are events where the occurrence of one event affects the probability of another event. The video script clearly demonstrates this by showing that the probability of Rahul eating a bagel for breakfast changes when you know he has eaten pizza for lunch. This concept is fundamental to the video's discussion of probability.
💡Calculation
Calculation in this context refers to the mathematical process used to determine probabilities and their relationships. The video script walks through the steps of calculating the probability of B given A, using known probabilities of A and B, and the conditional probability of A given B. This process is essential for understanding how probabilities can be manipulated and interpreted.
💡Rounding
Rounding is the process of adjusting a number to a specified number of decimal places. In the video, the final calculated probability of B given A is rounded to the nearest hundredth. This is a common practice in statistics and probability to simplify results and make them more manageable.
💡Drum Roll
A drum roll is a rhythmic pattern played on drums, often used to build anticipation or suspense. In the video script, a 'drum roll' is metaphorically used to build excitement before revealing the final calculated probability. This adds a light-hearted touch to the otherwise technical content.
Highlights

Rahul's two favorite foods are bagels and pizza.

Event A represents Rahul eating a bagel for breakfast, and event B represents him eating pizza for lunch.

The probability of Rahul eating a bagel for breakfast (probability of A) is 0.6.

The probability of Rahul eating pizza for lunch (probability of B) is 0.5.

The conditional probability of Rahul eating a bagel for breakfast given he eats pizza for lunch is 0.7.

The difference in probabilities indicates that events A and B are dependent, not independent.

The probability of A given B is higher than the probability of A alone, showing a dependency between the events.

The task is to determine the probability of B given A.

The probability of both A and B happening can be calculated using the formula P(A and B) = P(A|B) * P(B).

Alternatively, P(A and B) can also be expressed as P(B|A) * P(A).

The probability of A and B happening is the product of P(A|B) and P(B), which is 0.35.

The probability of A is known to be 0.6, which is used to solve for P(B|A).

By setting up the equation 0.6 * P(B|A) = 0.35, the probability of B given A can be solved.

Dividing 0.35 by 0.6 gives the probability of B given A, which is approximately 0.58.

The probability of B given A is higher than the probability of B alone, confirming the dependency between the events.

The practical application of this understanding can help in making predictions about dependent events.

The method used in this transcript provides a clear approach to calculating conditional probabilities in dependent events.

Transcripts

Browse More Related Video

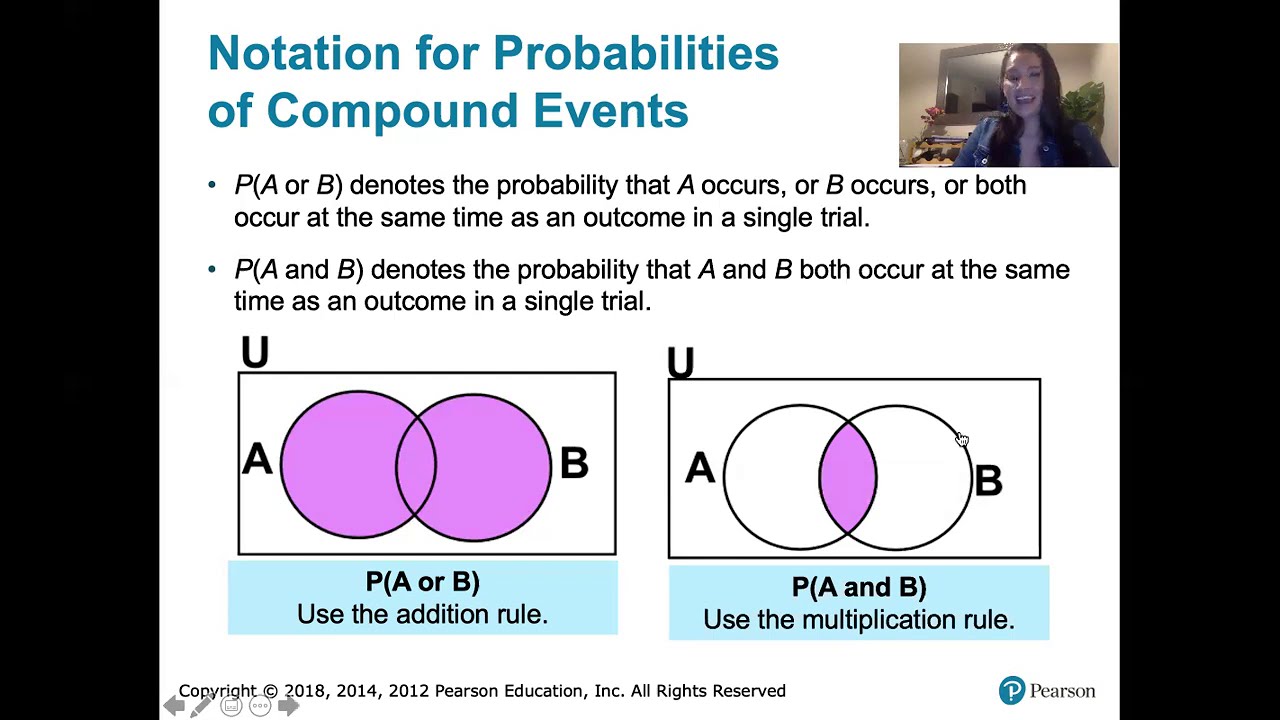
4.2.1 Addition and Multiplication Rules - Simple and Compound Events, and Their Probabilities

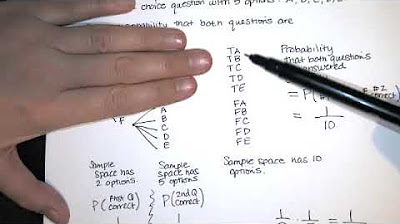
4.2.5.1 Addition and Multiplication Rules - The Rationale Behind the Multiplication Rule

Probability Tree Diagrams

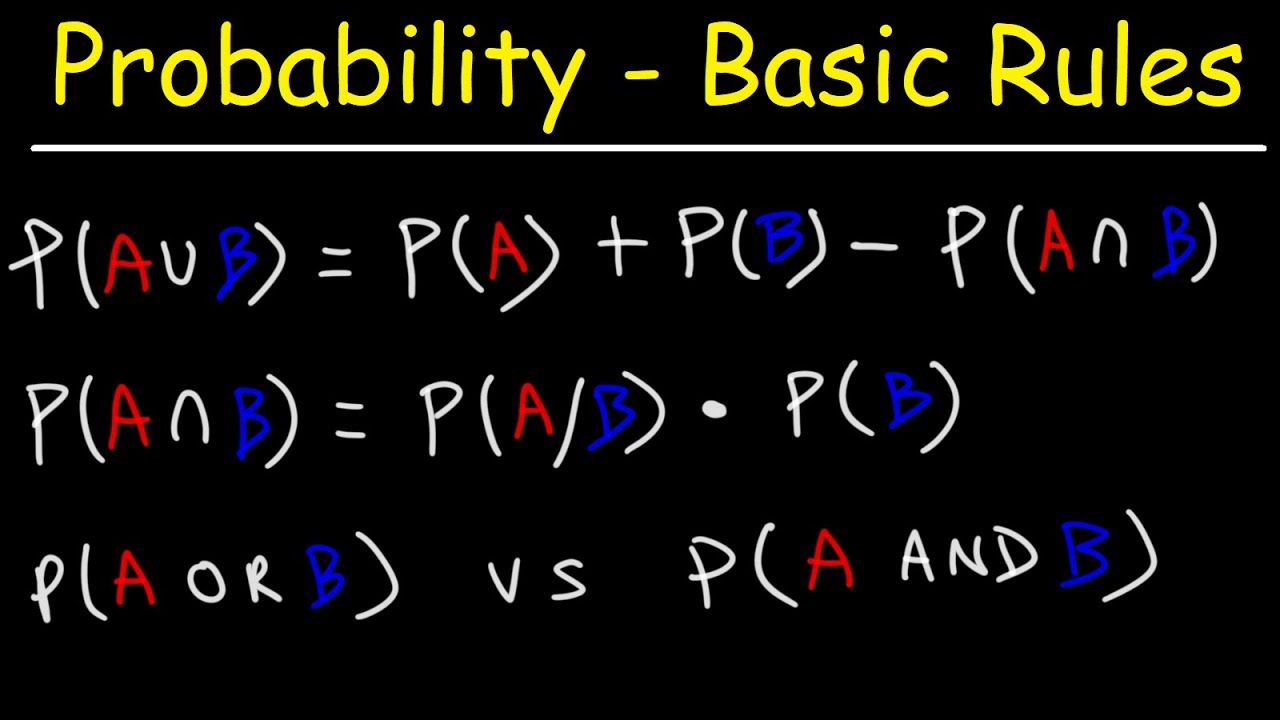
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events

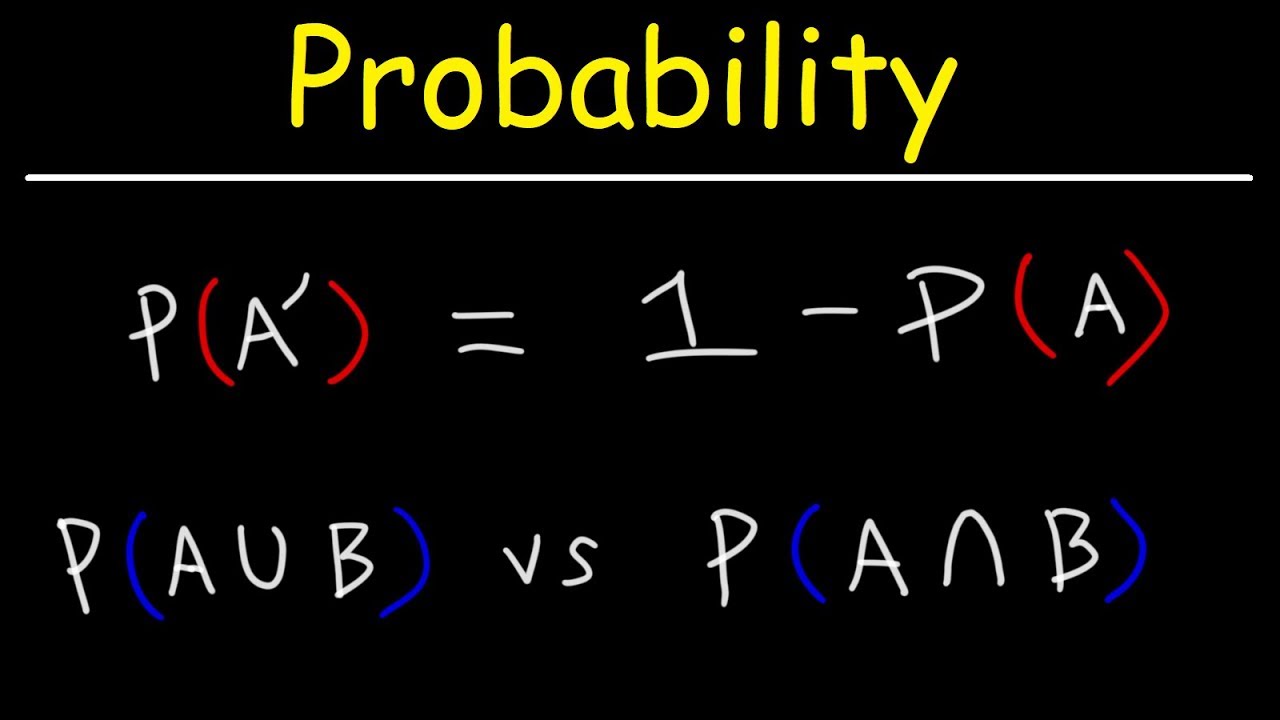
Probability of Complementary Events & Sample Space

"Mutually Exclusive" and "Independent" Events (...are VERY different things!)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityDependent EventsBagelsPizzaBreakfastLunchCalculusConditional ProbabilityMathematicsEducationalFood Choices