How To Find The Reference Angle In Radians and Degrees - Trigonometry

The Organic Chemistry Tutor
12 Oct 201712:28
EducationalLearning
32 Likes 10 Comments

TLDRThis video script provides a comprehensive guide on understanding and calculating reference angles. It explains that reference angles are always less than 90 degrees and are the angles between the terminal side of an angle and the x-axis. The script covers how to find reference angles for angles in different quadrants using specific formulas and demonstrates this with examples like 120 degrees and 210 degrees. It also addresses negative angles and their coterminal angles, as well as how to handle angles in radians, particularly common angles on the unit circle. The script concludes with converting reference angles back to radians, using examples to illustrate the process.

Takeaways
  • πŸ“ The reference angle is the angle between the terminal side of an angle and the x-axis, and it is always less than 90 degrees.
  • πŸ”’ For a 120-degree angle in quadrant two, the reference angle is found by subtracting the angle from 180 degrees, resulting in 60 degrees.
  • πŸ“‰ The reference angle for angles in different quadrants can be calculated using specific formulas: Quadrant 1 - equal to the angle, Quadrant 2 - 180 minus the angle, Quadrant 3 - the angle minus 180, and Quadrant 4 - 360 minus the angle.
  • πŸ“š To find the reference angle for 150 degrees in quadrant two, use the formula 180 - 150, which equals 30 degrees.
  • πŸ“ˆ For a 315-degree angle in quadrant four, the reference angle is calculated as 360 - 315, resulting in 45 degrees.
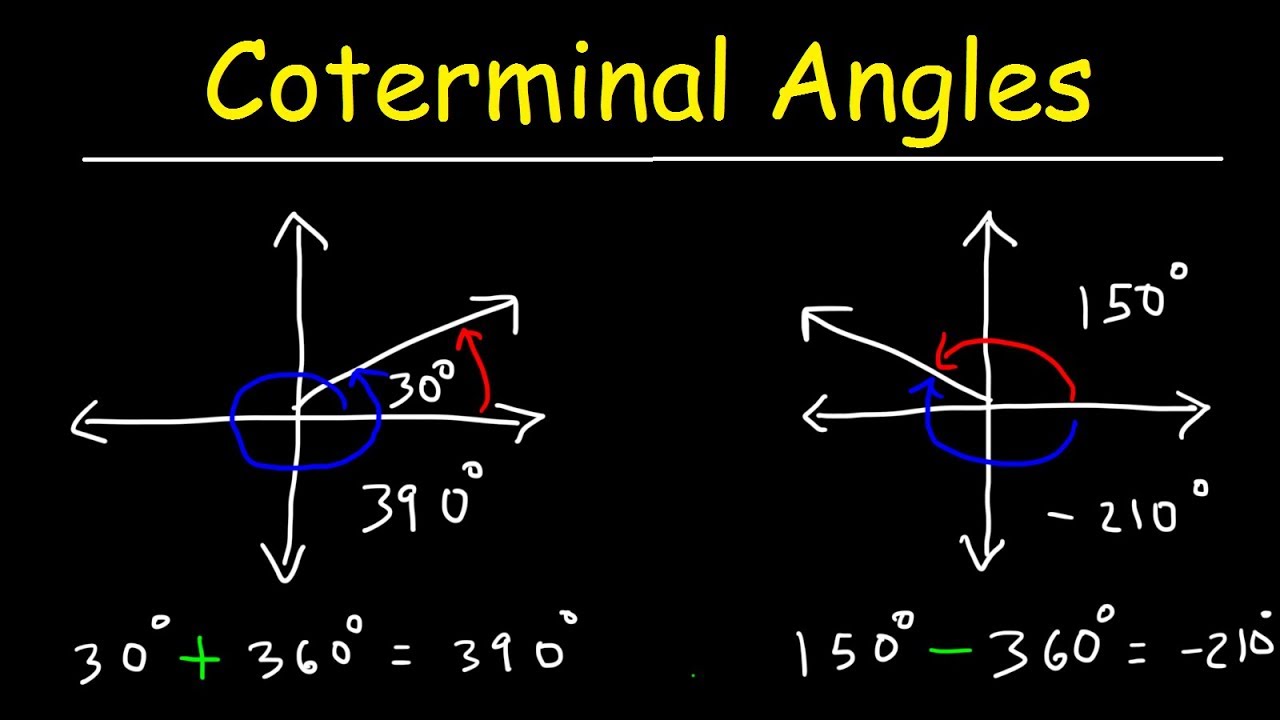
  • πŸ” For negative angles, find the coterminal angle by adding 360 degrees to the negative angle, then apply the appropriate formula based on the quadrant of the coterminal angle.
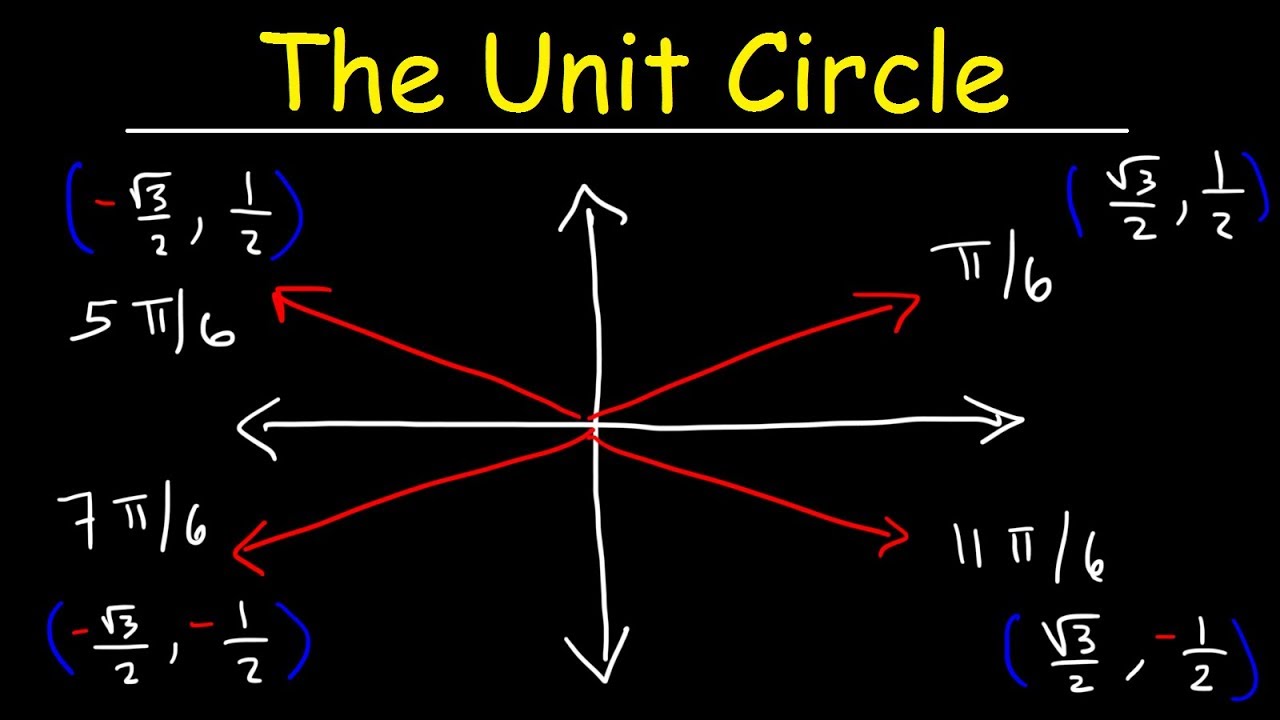
  • πŸ“ When dealing with angles in radians on the unit circle, common angles share the same reference angle, such as Ο€/3 for 2Ο€/3, 4Ο€/3, etc.
  • πŸ”’ For non-common angles in radians, convert to degrees first, determine the quadrant, and then find the reference angle using the appropriate formula.
  • πŸ“‰ To convert an angle from radians to degrees, multiply the radian measure by 180 and divide by Ο€.
  • πŸ”„ To convert degrees back to radians, multiply the degree measure by Ο€ and divide by 180.
  • πŸ“š The process of finding reference angles involves understanding the angle's location in relation to the unit circle and applying mathematical formulas based on the quadrant in which the angle resides.
Q & A

  • What is a reference angle?

    -A reference angle is the angle between the terminal side of an angle and the x-axis, and it is always less than 90 degrees, ranging between 0 and 90 degrees.

  • How do you find the reference angle for an angle of 120 degrees?

    -To find the reference angle for 120 degrees, which is in quadrant two, you subtract the angle from 180 degrees. So, the reference angle is 180 - 120 = 60 degrees.

  • What is the reference angle for an angle of 210 degrees relative to the positive x-axis?

    -For an angle of 210 degrees, which is in quadrant four, the reference angle is found by subtracting the angle from 360 degrees. So, the reference angle is 360 - 210 = 150 degrees.

  • What are the formulas for finding the reference angle in different quadrants?

    -In quadrant one, the reference angle is equal to the angle itself. In quadrant two, it is 180 - angle. In quadrant three, it is angle - 180. In quadrant four, it is 360 - angle.

  • How do you find the reference angle for an angle of 150 degrees?

    -Since 150 degrees is in quadrant two, the reference angle is found using the formula 180 - angle, which gives 180 - 150 = 30 degrees.

  • What is the reference angle for an angle of 315 degrees?

    -For 315 degrees, which is in quadrant four, the reference angle is found by subtracting the angle from 360 degrees, resulting in 360 - 315 = 45 degrees.

  • How can you find the reference angle for a negative angle, such as -150 degrees?

    -For negative angles, you first find the coterminal angle by adding 360 degrees to the negative angle. For -150 degrees, the coterminal angle is 210 degrees, which is in quadrant three. Then, find the reference angle by subtracting the angle from 180 degrees, so the reference angle is 180 - 210 = 30 degrees.

  • What is the reference angle for an angle in radians, such as 2Ο€/3?

    -For common angles on the unit circle like 2Ο€/3, the reference angle is the same as the angle itself, which is Ο€/3 radians.

  • How do you find the reference angle for a non-common angle in radians, such as 3Ο€/5?

    -First, convert the angle to degrees by multiplying the numerator by 180/Ο€. For 3Ο€/5, this gives 108 degrees, which is in quadrant two. Then, use the formula for quadrant two to find the reference angle: 180 - angle, which gives 180 - 108 = 72 degrees. Convert this back to radians by multiplying by Ο€/180, resulting in 2Ο€/5 radians.

  • How do you find the reference angle for a negative angle in radians, such as -8Ο€/9?

    -Convert the negative angle to degrees by multiplying the numerator by 180/Ο€. For -8Ο€/9, this gives -160 degrees. Find the coterminal positive angle by adding 360 degrees, resulting in 200 degrees, which is in quadrant three. Then, find the reference angle by subtracting the angle from 180 degrees, so the reference angle is 180 - 200 = 20 degrees. Convert this back to radians by multiplying by Ο€/180, resulting in 2Ο€/18 or Ο€/9 radians.

Outlines
00:00
πŸ“ Understanding Reference Angles and Their Calculation

This paragraph introduces the concept of reference angles, explaining how to find them for angles in different quadrants. The reference angle is defined as the angle between the terminal side of an angle and the x-axis, always being less than 90 degrees. The explanation includes examples of how to calculate reference angles for angles in quadrants two and four, using the formulas 180 minus the angle for quadrant two and 360 minus the angle for quadrant four. The paragraph also covers how to find reference angles for negative angles by finding their coterminal positive angles and then applying the same principles. Graphical illustrations are used to demonstrate the process visually.

05:00
πŸ“š Reference Angles in Radians and Non-Common Angles

This section delves into finding reference angles for angles given in radians, particularly focusing on common angles on the unit circle such as 2Ο€/3, 4Ο€/3, and 5Ο€/3. It explains that these common angles share the same reference angle, which can be easily identified. For non-common angles, such as 3Ο€/5, the paragraph suggests converting the angle to degrees first and then applying the quadrant-specific formulas to find the reference angle. The process includes converting the angle to degrees, identifying the quadrant, and using the appropriate formula to find the reference angle, which is then converted back to radians for consistency with the original angle format.

10:03
πŸ” Advanced Reference Angle Calculations with Radians

The final paragraph presents more complex examples of finding reference angles for angles in radians, including those with negative values. It demonstrates the process of converting radians to degrees, finding the coterminal positive angle, determining the quadrant, and applying the formula to find the reference angle. The examples include angles such as 9Ο€/8 and -8Ο€/9, where the reference angle is found by converting to degrees, adjusting for the correct quadrant, and then converting the resulting reference angle back to radians. This paragraph reinforces the methodical approach to finding reference angles for various types of radian measurements.

Mindmap
Keywords
πŸ’‘Reference Angle
A reference angle is the angle formed between the terminal side of an angle measured in standard position and the x-axis. It is always between 0 and 90 degrees. In the video's context, reference angles are used to simplify the analysis of trigonometric functions by focusing on the acute angle that an angle's terminal side makes with the x-axis. For instance, the reference angle for a 120-degree angle in quadrant two is found by subtracting 120 from 180, resulting in 60 degrees.
πŸ’‘Quadrant
In the context of the video, a quadrant refers to one of the four equal divisions of the coordinate plane by the x and y axes. Each quadrant has specific properties regarding the signs of the x and y coordinates of points within it. The video discusses reference angles in relation to different quadrants, such as quadrant one having the same reference angle as the angle itself, and quadrant two's reference angle being 180 minus the angle.
πŸ’‘Terminal Side
The terminal side of an angle is the half-line that extends from the origin in the direction that the angle points to. In the video, the reference angle is defined as the angle between the x-axis and the terminal side of the given angle. This concept is crucial for understanding how to calculate reference angles, as it helps visualize the geometric relationship between the angle and the coordinate axes.
πŸ’‘Coterminal Angle
A coterminal angle is an angle that shares the same terminal side as the original angle but is obtained by adding or subtracting full rotations (360 degrees) to the original angle. In the script, coterminal angles are used to find reference angles for negative angles by converting them to their positive counterparts within the standard 0-360 degree range, such as converting -150 degrees to 210 degrees.
πŸ’‘Radians
Radians are a unit of angular measurement used in trigonometry, where a full circle is 2Ο€ radians. The video discusses how to find reference angles for angles given in radians, especially for common angles on the unit circle like Ο€/3, 2Ο€/3, etc. The reference angle for an angle in radians is determined by its position relative to Ο€/2, Ο€, and 3Ο€/2.
πŸ’‘Unit Circle
The unit circle is a circle with a radius of one, centered at the origin of the coordinate plane. It is used in the video to discuss common angles in radians and their reference angles. For example, angles like Ο€/3, 2Ο€/3, and 5Ο€/3 have reference angles that are the same as the angle itself because they are positioned symmetrically on the unit circle.
πŸ’‘Negative Angle
A negative angle is an angle that is measured in the clockwise direction from the positive x-axis. The video explains how to find reference angles for negative angles by first finding their coterminal positive angles and then applying the appropriate formula based on the quadrant in which the coterminal angle lies, such as subtracting the angle from 180 for quadrant two or adding 180 for quadrant three.
πŸ’‘Degrees
Degrees are a unit of angular measurement where a full circle is 360 degrees. The video script frequently converts angles from radians to degrees to simplify the process of finding reference angles. For example, the angle 3Ο€/5 radians is converted to degrees by multiplying 3Ο€/5 by 180/Ο€, resulting in 108 degrees.
πŸ’‘Formula
In the context of the video, formulas are used to calculate reference angles based on the quadrant in which the angle lies. The video provides specific formulas for each quadrant: for quadrant one, the reference angle is equal to the angle itself; for quadrant two, it is 180 minus the angle; for quadrant three, it is the angle minus 180; and for quadrant four, it is 360 minus the angle.
πŸ’‘Trigonometric Functions
Although not explicitly mentioned in the script, trigonometric functions are the underlying theme for the discussion of reference angles. Reference angles are used to evaluate trigonometric functions more easily, as these functions are periodic and exhibit symmetry properties. The video's explanation of reference angles indirectly supports the understanding of trigonometric functions like sine, cosine, and tangent.
Highlights

Introduction to reference angles and their importance in trigonometry.

Explanation of how to find the reference angle for a 120-degree angle in quadrant two.

Drawing and visualizing the reference angle as the angle between the x-axis and the terminal side.

The rule that reference angles are always less than 90 degrees, between 0 and 90.

Calculating the reference angle for 210 degrees using the difference from 180 degrees.

General equations for finding reference angles in different quadrants.

Using the formula for quadrant two to find the reference angle for 150 degrees.

Visual representation of a full rotation and finding the reference angle for 315 degrees.

Understanding reference angles for negative angles by finding coterminal angles.

Graphical method to find the reference angle for negative 150 degrees.

Finding the reference angle for negative 240 degrees and its position in quadrant two.

Conversion of angles from radians to degrees to find reference angles for non-common angles.

Technique for finding reference angles for common angles in radians on the unit circle.

Conversion of 72 degrees back to radians to find the reference angle.

Finding the reference angle for nine pi divided by eight radians by converting to degrees.

Conversion of the reference angle for negative 8 pi divided by 9 back to radians.

Final example summarizing the process of finding reference angles for both positive and negative radians.

Transcripts

Browse More Related Video

REFERENCE ANGLES || PRE-CALCULUS

Coterminal Angles In Radians & Degrees - Basic Introduction, Trigonometry

Reference Angles Trigonometry, In Radians, Unit Circle - Evaluating Trig Functions

How To Find The Exact Values of Trig Functions

The Unit Circle, Basic Introduction, Trigonometry

Precalc 4.1-4.4 Review!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Reference AnglesMathematicsTrigonometryQuadrantsCalculusEducationalGeometryAngle CalculationRadiansDegrees