Precalc 4.1-4.4 Review!

SupernovaMath

23 Jan 202022:40

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video script offers a comprehensive guide on radian and degree measures, coterminal angles, and trigonometric functions. It explains how to find positive and negative coterminal angles in degrees and radians, and demonstrates the process of calculating trigonometric values for given angles using the unit circle and hand trick. The script also covers special right triangles, SOHCAHTOA, and identifying the quadrant of angles based on trigonometric conditions. It concludes with solving problems involving trigonometric functions and their reciprocals, aiming to prepare viewers for an upcoming test.

Takeaways

😀 To find coterminal angles in degrees, add or subtract 360 degrees.
😀 To find coterminal angles in radians, add or subtract 2π radians.
😀 For radians, convert to a common denominator when adding or subtracting fractions.
😀 On the unit circle, use the hand trick to find coordinates for reference angles.
😀 When graphing angles in radians, adjust to a common denominator to identify the correct quadrant.
😀 Reciprocal trig functions like secant and cosecant can be found by flipping sine and cosine values.
😀 Reference angles are always between 0 and 90 degrees.
😀 Use SOHCAHTOA to solve for sides in right triangles, remembering to identify opposite, adjacent, and hypotenuse correctly.
😀 For special right triangles, memorize the side ratios for 30-60-90 and 45-45-90 triangles.
😀 Determine the quadrant of an angle based on the signs of sine, cosine, and tangent using the ASTC rule.

Q & A

What is the purpose of the video?
-The purpose of the video is to explain and demonstrate how to find coterminal angles in both degrees and radians, as well as to calculate trigonometric functions for various angles on the unit circle.
How can you find a coterminal angle in degrees?
-To find a coterminal angle in degrees, you can add or subtract 360 degrees from the given angle.
What is the relationship between radians and degrees when finding coterminal angles?
-For radians, you add or subtract 2π (two pi radians) to find coterminal angles, which is equivalent to adding or subtracting 360 degrees.
How does the 'hand trick' help in finding coordinates on the unit circle?
-The 'hand trick' is a mnemonic device that helps to determine the coordinates of points on the unit circle for given reference angles by using the angles formed by the fingers of your hand.
What is the reference angle for 2π/3 radians?
-The reference angle for 2π/3 radians is π/3 radians, which is equivalent to 60 degrees.
How can you rationalize a trigonometric function value that involves a radical in the denominator?
-To rationalize a trigonometric function value with a radical in the denominator, multiply the numerator and denominator by the conjugate of the denominator.
What trigonometric function is used to find the y-coordinate on the unit circle?
-The sine function is used to find the y-coordinate on the unit circle.
What is the relationship between the trigonometric functions and their reciprocal functions?
-The reciprocal functions are the cosecant (reciprocal of sine), secant (reciprocal of cosine), and cotangent (reciprocal of tangent).
How can you determine the quadrant in which an angle lies based on the signs of its trigonometric functions?
-You can determine the quadrant by considering the signs of the sine, cosine, and tangent functions. For example, if cosine is positive and sine is negative, the angle lies in the fourth quadrant.
What is the significance of the Pythagorean triple in the context of special right triangles?
-A Pythagorean triple is a set of three positive integers that satisfy the Pythagorean theorem (a² + b² = c²). In the context of special right triangles, such as 30-60-90 and 45-45-90 triangles, the sides are in a specific ratio that forms a Pythagorean triple, which simplifies the calculation of trigonometric functions.
How does the video script help in understanding the concept of reference angles?
-The script explains the process of finding reference angles by subtracting or adding fractions of a full circle (360 degrees or 2π radians) to the given angle, which helps in understanding how to simplify the angle for trigonometric calculations.

Outlines

00:00

📚 Understanding Coterminal Angles and Trigonometric Functions

This paragraph introduces the concept of coterminal angles, both in degrees and radians, and demonstrates how to find positive and negative coterminal angles for given angles in standard position. The explanation covers the process of adding and subtracting 360 degrees or 2π radians to find coterminal angles. It also shows calculations for angles in degrees and their corresponding radians, including simplifying fractions of π. The paragraph sets the stage for further exploration of trigonometric functions on the unit circle.

05:03

📐 Trigonometric Functions on the Unit Circle

The speaker delves into the specifics of finding the coordinates of a point on the unit circle corresponding to a given angle θ, as well as the exact values of trigonometric functions at that angle. The explanation includes drawing the unit circle, identifying the quadrant in which the angle lies, and using the hand trick to find the reference angle and coordinates. The process involves determining the x-coordinate (cosine) and y-coordinate (sine) and then calculating other trigonometric functions such as tangent, secant, and cosecant, including rationalizing the results where necessary.

10:04

🔍 Identifying Reference Angles and Quadrants

This section focuses on identifying reference angles and determining the quadrant in which an angle lies. The paragraph explains the process of graphing angles in degrees and radians, finding the reference angle by subtracting from a multiple of π (or 180 degrees), and understanding which quadrant the angle is in based on its position relative to the multiples of π/2 (or 90 degrees). The explanation is crucial for determining the signs of trigonometric functions in different quadrants.

15:06

📉 Evaluating Trigonometric Functions for Specific Angles

The speaker provides a step-by-step method for finding the exact values of trigonometric functions for specific angles, including how to handle negative angles by finding their positive coterminal angles. The explanation includes using the reference angle to determine the ordered pair on the unit circle and then calculating the trigonometric functions based on this pair. The paragraph also touches on the repetitive nature of these calculations and the importance of understanding the unit circle and reference angles.

20:09

📐 Applying SOHCAHTOA and Understanding Special Right Triangles

This paragraph introduces the mnemonic SOHCAHTOA for solving right triangles and explains how to find the sides of a triangle when two sides and the included angle are known. It also covers special right triangles, specifically the 30-60-90 and 45-45-90 triangles, and emphasizes the need to memorize the side ratios for these triangles. The explanation includes setting up equations to solve for unknown sides using the known side ratios and the Pythagorean theorem.

🧭 Determining Quadrants Based on Trigonometric Function Signs

The speaker explains how to determine the quadrant in which an angle lies based on the signs of its trigonometric functions. The explanation includes a mnemonic for remembering the signs of functions in each quadrant and how to use given conditions, such as the sign of cosine or tangent, to identify the quadrant. The paragraph also provides examples of how to use these conditions to find the correct quadrant for different angles.

📝 Solving Trigonometric Problems Using Given Conditions

The final paragraph discusses solving trigonometric problems by using given conditions to determine the signs of trigonometric functions and thus the quadrant of the angle. The explanation includes drawing triangles in the correct quadrant based on the signs of cosine and sine and using the Pythagorean theorem to find unknown sides. The paragraph concludes with a summary of the types of problems covered in the video, aiming to help students prepare for their upcoming tests.

Mindmap

Keywords

💡Coterminal Angles

Coterminal angles are angles that share the same terminal side but have different measures. In the context of the video, the concept is used to find both positive and negative coterminal angles for given angles in standard position. The script explains how to find these by adding or subtracting 360 degrees for angles in degrees, and multiples of 2π for angles in radians, which is essential for understanding the periodic nature of trigonometric functions.

💡Radians

Radians are a unit of angular measure used in trigonometry, equivalent to the length of the arc of a circle that subtends the angle. In the video, the script discusses converting between degrees and radians, and how to work with radian measures when finding coterminal angles, which is crucial for understanding the relationship between angle measures and their trigonometric functions.

💡Degrees

Degrees are a common unit of angular measurement where a full circle is divided into 360 equal parts. The script uses degrees to explain the process of finding coterminal angles by adding or subtracting 360, demonstrating the fundamental concept of periodicity in trigonometry.

💡Unit Circle

The unit circle is a circle with a radius of 1, centered at the origin of a coordinate system. It is used in the video to determine the coordinates of points corresponding to specific angles, which is fundamental for understanding trigonometric functions and their values at various angles.

💡Trigonometric Functions

Trigonometric functions are mathematical functions that relate the angles of a triangle to the lengths of its sides. In the video, the script explains how to find the exact values of these functions at specific angles, such as sine, cosine, and tangent, which are key to understanding the relationship between an angle's measure and its coordinates on the unit circle.

💡Reference Angle

A reference angle is the acute angle formed by the terminal side of an angle and the x-axis. The video uses reference angles to determine the coordinates of points on the unit circle, which is essential for finding the values of trigonometric functions.

💡Hand Trick

The hand trick is a mnemonic device used to remember the coordinates of points on the unit circle for standard angles. The script mentions the hand trick as a method to find the ordered pair for reference angles, which is a practical tool for visual learners to understand trigonometric concepts.

💡Rationalizing

Rationalizing is the process of eliminating square roots from a fraction by multiplying the numerator and denominator by a form of 1 that contains the conjugate of the denominator. In the video, the script refers to rationalizing to simplify expressions involving trigonometric functions, which is important for obtaining exact values and simplifying expressions.

💡Pythagorean Triple

A Pythagorean triple consists of three positive integers that satisfy the Pythagorean theorem. The script mentions a Pythagorean triple (3, 4, 5) when discussing the sides of a right triangle, which is a specific example used to demonstrate the application of the Pythagorean theorem.

💡Special Right Triangles

Special right triangles are right triangles with angles that are multiples of 30 degrees or 45 degrees, which have side ratios that can be easily remembered. The video script discusses the properties of 30-60-90 and 45-45-90 triangles, which are fundamental for understanding the relationships between the angles and sides in these common geometric figures.

💡Quadrants

In the context of the unit circle, quadrants refer to the four equal divisions of the circle by the x and y axes. The script explains which trigonometric functions are positive or negative in each quadrant, which is crucial for determining the signs of trigonometric function values based on the angle's position.

Highlights

Introduction to the video on finding coterminal angles in both degree and radian measures.

Explanation of how to find coterminal angles by adding or subtracting 360 degrees.

Demonstration of converting between degrees and radians for coterminal angles using the relationship 360 degrees equals 2π radians.

Use of the 'hand trick' for finding coordinates on the unit circle corresponding to given angles.

Method for determining the coordinates of a point on the unit circle using reference angles.

Process of rationalizing the denominator when finding trigonometric function values.

Explanation of how to find the exact values of trigonometric functions at a given angle using the unit circle.

Mistake made during the explanation and the subsequent correction in the process.

Graphing angles in different quadrants to determine their reference angles.

Finding the quadrant containing an angle based on given trigonometric conditions.

Use of mnemonics like 'all students take calculus' to remember the signs of trigonometric functions in different quadrants.

Solving for unknown sides in right triangles using the Pythagorean theorem and trigonometric ratios.

Application of SOHCAHTOA mnemonic for solving right triangle problems.

Understanding the relationship between special right triangles and their angles and sides.

Using given trigonometric function values to determine the quadrant of an angle.

Drawing a triangle in the correct quadrant based on the signs of trigonometric functions.

Final summary of the problems covered in the video to prepare for a test on trigonometry.

Transcripts

Browse More Related Video

Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles

How To Find The Exact Values of Trig Functions

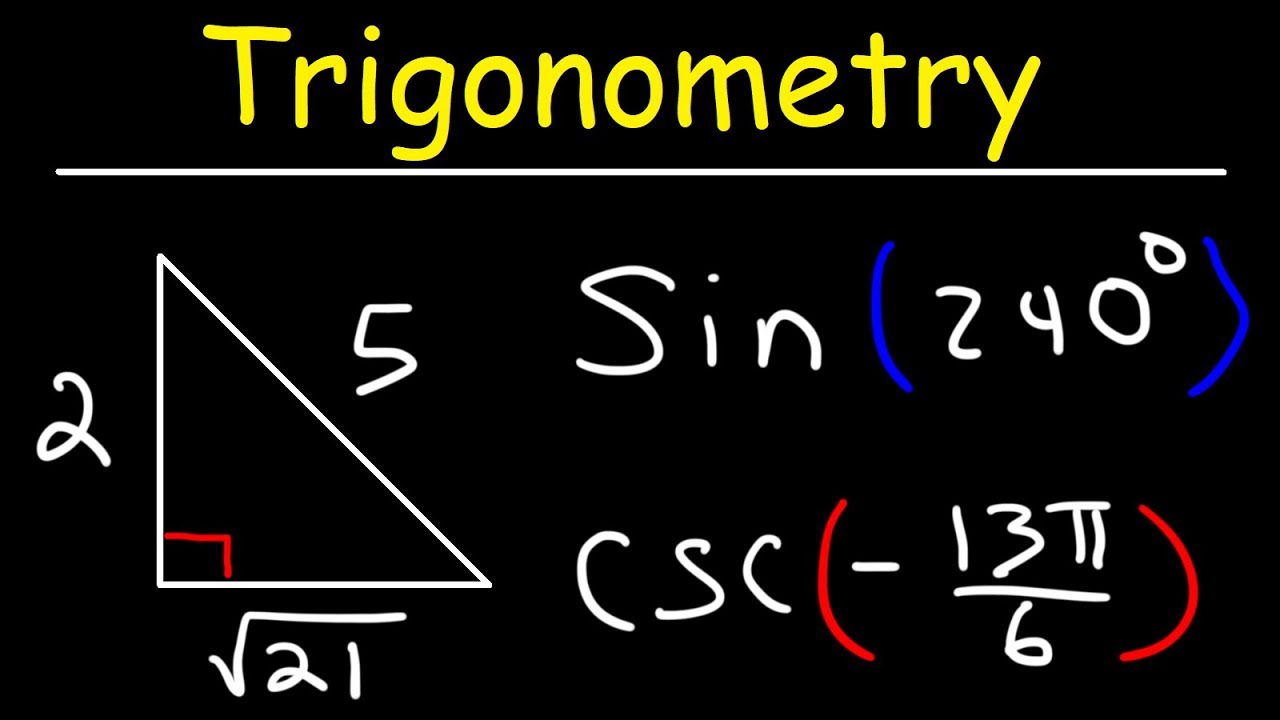
Trigonometry

How to Use the Unit Circle in Trigonometry (Precalculus - Trigonometry 7)

An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)

How To Find The Reference Angle In Radians and Degrees - Trigonometry

Related Tags

Trigonometry Guide Mathematics Tutorial Radian Measure Degree Measure Coterminal Angles Unit Circle Trig Functions Special Triangles SOHCAHTOA Pythagorean Theorem