Calculus 1 - Integration: Proof of the Fundamental Theorem of Calculus

Math With Allison
20 Nov 202309:05
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging video, Allison continues her integration series by delving into the proof of the Fundamental Theorem of Calculus. She begins by defining the area function, which represents the net area between the graph of a function and the x-axis over a given interval. Using the function f(T) = 2T + 3 as an example, she illustrates how to calculate the area under the curve without integration, employing the trapezoidal rule. The video then transitions to differentiating the area function, revealing that its derivative is the original function integrated. The proof of the theorem is presented, showing that the derivative of the area function is equal to the integrand, a key insight of the theorem. The video concludes with an application of the theorem, demonstrating how to find the derivative of an integral by substituting the variable inside the integral. This comprehensive explanation not only clarifies the theorem's significance but also its practical application in calculus.

Takeaways
  • 📐 The area function A(x) is defined as the integral from 'a' to 'x' of the function f(t) dt, where 'a' is a constant and 'x' is the variable.
  • 📈 The area function represents the net area between the graph of the function and the t-axis over the interval [a, x].
  • 🔢 An example is provided where f(t) = 2t + 3 and the area between t=2 and t=x is calculated using the trapezoidal rule.
  • 🟩 The area of the function is calculated without using integration, resulting in the formula A(x) = x^2 + 3x - 10.
  • 🧮 By setting up the integral for the area function, it is shown that A(x) = ∫(2t + 3) dt from 2 to x, which matches the area calculated previously.
  • 📉 The derivative of the area function A'(x) is found to be equal to the original function f(x), which is 2x + 3 in the example.
  • 🔑 The Fundamental Theorem of Calculus is introduced, which states that the integral of the derivative is the original function, and the derivative of the integral is the function itself.
  • 📚 A proof of the Fundamental Theorem of Calculus is given, showing that the derivative of the area function is the function being integrated.
  • 📌 The process of differentiating integrals is demonstrated by taking the function inside the integral and substituting the variable.
  • 📐 An example is shown where the integral of sin(t) * cos(2t) dt from 1 to 2 is solved by finding the antiderivative and applying the limits of integration.
  • 🔍 The final value obtained from the integral represents the area under the curve of the function between the given interval.
  • 🎓 The video concludes with a summary of the key points and an invitation to explore more mathematical topics in the playlist.
Q & A

  • What is the fundamental theorem of calculus?

    -The fundamental theorem of calculus establishes the relationship between differentiation and integration. It states that if a function is continuous over an interval [a, b] and F is an antiderivative of f on [a, b], then the definite integral of f from a to b is equal to F(b) - F(a).

  • What is the area function in the context of the fundamental theorem of calculus?

    -The area function, often denoted as A(x), represents the net area bounded by the graph of the function f(t), the t-axis, and the vertical lines t=a and t=x, where a is a constant and x is greater than or equal to a.

  • How is the area function defined mathematically?

    -The area function A(x) is defined as the integral from 'a' to 'x' of f(t) dt, where 'f' is a continuous function and 'x' is greater than or equal to 'a'.

  • What is the process of finding the area under a curve without using integration?

    -Without using integration, one can approximate the area under a curve by breaking it down into shapes like rectangles or trapezoids, calculating the area of each shape, and summing these areas to find the total area under the curve.

  • How does the area function relate to the derivative of the function?

    -The derivative of the area function A'(x) is equal to the original function f(x). This is because the rate of change of the area under the curve with respect to x is given by the function f(x) itself.

  • What is the antiderivative of the function f(t) = 2t + 3?

    -The antiderivative of the function f(t) = 2t + 3 is F(x) = x^2 + 3x + C, where C is the constant of integration.

  • How do you find the derivative of an integral using the fundamental theorem of calculus?

    -To find the derivative of an integral, you simply take the derivative of the function inside the integral and then replace the variable of integration with the variable of differentiation. The limits of integration remain unchanged.

  • What is the geometric interpretation of the derivative of the area function?

    -The geometric interpretation of the derivative of the area function is that it gives the height of the curve at a particular point, which is equivalent to the function value at that point.

  • How does the proof of the fundamental theorem of calculus involve limits?

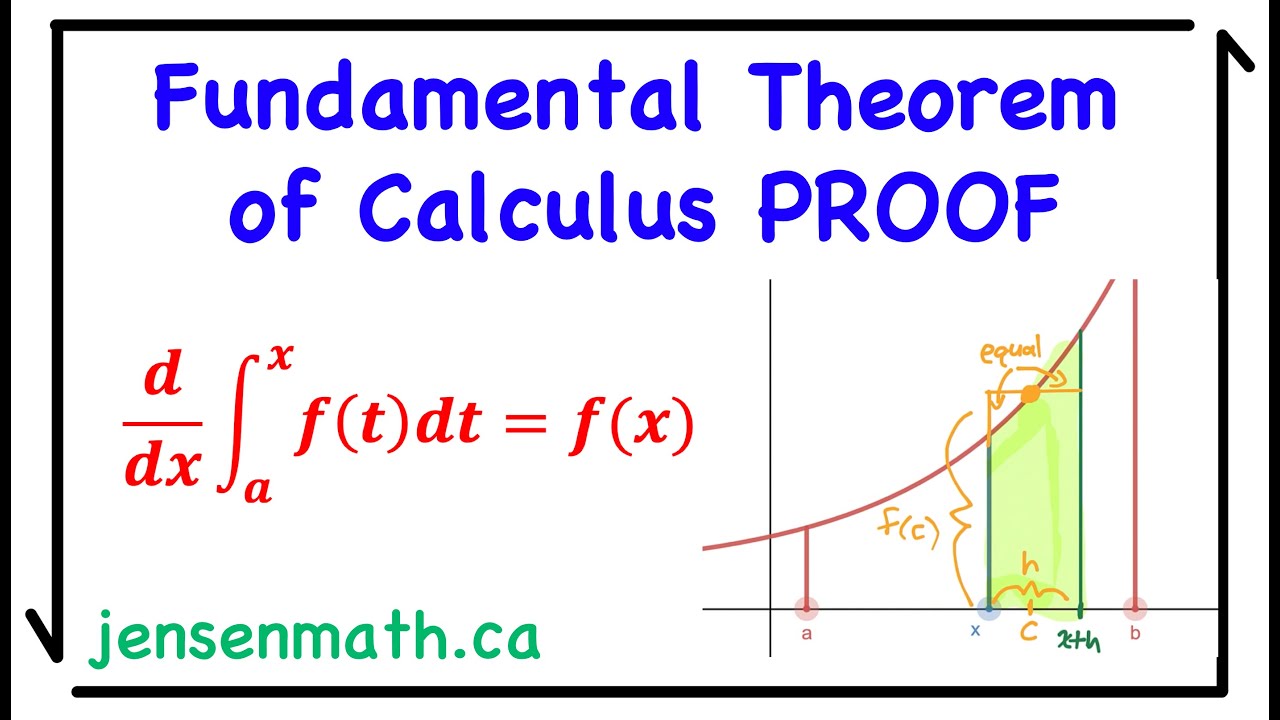
    -The proof involves taking the limit as the width of a rectangle (used to approximate the area under the curve) approaches zero, which leads to the definition of the derivative and shows that the derivative of the area function is the original function being integrated.

  • What is the practical application of the fundamental theorem of calculus?

    -The fundamental theorem of calculus is applied in various fields to calculate areas, volumes, central points (centroids), and in solving problems involving rates of change, such as in physics and engineering.

  • How does the fundamental theorem of calculus relate to antiderivatives?

    -The fundamental theorem of calculus states that the process of integration can be reversed by finding an antiderivative of the function. This means that the integral of a function f from a to b is equal to the difference of the antiderivative evaluated at b and a.

Outlines
00:00
📚 Introduction to the Fundamental Theorem of Calculus

The video begins with an introduction to the Fundamental Theorem of Calculus, focusing on the area function. The area function is defined as the integral from 'a' to 'x' of the function 'f', where 'F' is a continuous function and 'x' is greater than or equal to 'a'. The video uses a graphical representation to illustrate the concept of the area function, which represents the net area between the graph of the function and the 'x'-axis over a given interval. An example is provided using the function f(T) = 2T + 3, and the area under the curve from 2 to 'x' is calculated both graphically and algebraically. The area function is shown to be equal to x^2 + 3x - 10. The video then transitions to the proof of the Fundamental Theorem of Calculus, which relates differentiation and integration.

05:01
🔍 Deriving the Area Function and the Fundamental Theorem of Calculus

The second paragraph delves into the process of deriving the area function and the Fundamental Theorem of Calculus. The video demonstrates how to estimate the area under a curve using rectangles, which leads to the definition of the derivative of the area function. By taking the limit as the width of the rectangle approaches zero, the video shows that the derivative of the area function (a'(x)) is equal to the function 'f' itself. This is a key step in proving the Fundamental Theorem of Calculus, which states that the integral of 'f' from 'a' to 'x' is equal to the antiderivative of 'f' evaluated from 'a' to 'x'. The video concludes with an example of differentiating an integral, showing that the process involves substituting the variable of integration into the antiderivative. The example provided involves finding the integral of 2x with respect to x from 1 to 2, which is calculated as 4 - 1, equaling 3. The video ends with an invitation to explore more mathematical topics and a prompt for viewers to engage with the content.

Mindmap
Keywords
💡Integration
Integration is a fundamental concept in calculus that involves finding the accumulated value of a function over a specific interval. In the video, integration is used to calculate the area under a curve, which is a core theme of the video. For example, the script discusses integrating the function 2T + 3 from a to x to find the area function A(x).
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a theorem that links the concept of differentiation and integration, stating that these two operations are essentially the inverse of each other. In the video, this theorem is proven and used to demonstrate how the derivative of an integral results in the original function. It is central to the video's narrative as it explains the relationship between the area function and the original function.
💡Area Function
The area function, denoted as A(x) in the script, is a continuous function that represents the net area under the graph of another function from a point 'a' to 'x'. It is used in the video to illustrate the process of integration. The script provides an example where the area function is calculated for the function f(T) = 2T + 3 from 2 to x.
💡Continuous Function
A continuous function is a function that has no breaks or gaps in its graph. In the context of the video, the continuity of a function is important because it is a prerequisite for applying the Fundamental Theorem of Calculus. The script mentions that 'F' is a continuous function on the interval from 'a' to 'x'.
💡Anti-Derivative
An anti-derivative, also known as an integral, is a function whose derivative is equal to the original function. In the video, finding the anti-derivative of a function is shown as the process of integration. For instance, the anti-derivative of 2T is T^2, which is used to calculate the area function A(x).
💡Derivative
The derivative of a function measures the rate at which the function changes with respect to its variable. In the video, the derivative of the area function A(x) is shown to be equal to the original function being integrated, demonstrating the Fundamental Theorem of Calculus. The script illustrates this by taking the derivative of A(x) and finding it to be 2x + 3.
💡Trapezoid Rule
The trapezoid rule is a method for approximating the definite integral of a function by breaking the area under the curve into trapezoids. In the video, the trapezoid rule is used to estimate the area under the curve of the function f(T) = 2T + 3 from 2 to x before applying integration to find the exact area.
💡Limit
In calculus, a limit is a value that a function or sequence approaches as the input approaches some value. The concept of limits is used in the video to define the derivative and to make the approximation of the area under a curve more accurate as H approaches zero. The script shows the limit definition as part of the proof of the Fundamental Theorem of Calculus.
💡Net Area
Net area refers to the total area under a curve, taking into account any regions above and below the x-axis. In the video, the net area is calculated for the interval between the curve of the function f(T) and the T-axis from 'a' to 'x'. The script uses the net area to introduce the concept of integration.
💡Interval
An interval in mathematics is a set of numbers with a specific order, such as from 'a' to 'b'. In the context of the video, the interval is the range over which the function is integrated to find the area function. The script discusses integration over the interval from 'a' to 'x' and later from 'a' to 'b'.
💡Differentiable
A function is differentiable at a point if it has a derivative at that point. In the video, it is shown that the area function is differentiable on the interval AB, which is a consequence of the Fundamental Theorem of Calculus. The differentiability of the area function is demonstrated by finding its derivative, which is equal to the original function being integrated.
Highlights

Introduction to the proof of the fundamental theorem of calculus.

Definition of the area function A(x) for a continuous function f from a to x.

Explanation of the area function as the net area between the graph of the function and the x-axis on a given interval.

Visual representation of the area function with an example function f(t) = 2t + 3.

Calculation of the area under the curve using the trapezoid rule without integration.

Derivation of the area function A(x) = x^2 + 3x - 10 for the example function.

Use of integration to find the area function A(x) for the same example function.

Verification that the integral calculation matches the area of the trapezoid.

Derivation of the area function's derivative A'(x) = 2x + 3.

Proof that the derivative of the area function is the original function f(t).

Demonstration of the limit definition to refine the area estimation as H approaches zero.

Statement of the fundamental theorem of calculus linking integration and differentiation.

Application of the fundamental theorem to differentiate the integral of a function.

Example of differentiating the integral of sin(t) * cos(2t) with respect to x.

Explanation of how to find the integral of a function from a to b using its antiderivative.

Solution to an example problem calculating the integral of 2x from 1 to 2.

Illustration of the area between the function 2x and the x-axis from 1 to 2.

Conclusion and invitation to explore more mathematical topics in the playlist.

Transcripts

Browse More Related Video

1st Fundamental Theorem of Calculus PROOF | Calculus 1 | jensenmath.ca

Fundamental Theorem of Calculus Explained | Outlier.org

Derivatives of Integrals (w/ Chain Rule)

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Fundamental Theorem of Calculus 1 | Geometric Idea + Chain Rule Example

Calculus - Lesson 15 | Relation between Differentiation and Integration | Don't Memorise

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegrationDerivativeArea FunctionAntiderivativesTheorem ProofMathematicsEducationalSeries VideoTrapezoid RuleLimit Definition