Calculus (Version #2) - 9.1 The Second Fundamental Theorem of Calculus
TLDRThe video script introduces the Second Fundamental Theorem of Calculus, which is fundamental to understanding calculus but surprisingly straightforward. The theorem connects differentiation and integration, showing that the derivative of an integral of a function is the original function, minus a constant. The lesson begins with defining a function by an integral where one of the bounds is a variable. It uses an example function to illustrate the process of finding areas under the curve for different intervals and how these areas change with the variable. The script then explains how to find the minimum and maximum of such a function. The main focus is on applying the theorem to find derivatives, using examples that include integrating and then differentiating to return to the original function. It also covers more complex scenarios where the integral has variables for both the lower and upper bounds, demonstrating how to handle such cases by splitting the integral and applying the derivative. The lesson concludes with practice problems to reinforce the concept, aiming to prepare students for more advanced calculus topics and exams.
Takeaways
- 📚 The second fundamental theorem of calculus is introduced as an important concept that will be discussed.
- 🔍 The theorem is related to the concept of a function defined by an integral, where one of the bounds is a variable.
- 📈 An example is given to illustrate how the function F(X) is the antiderivative of f(t) evaluated from 0 to X.
- 🔢 The value of F(X) at specific points (e.g., F(0), F(1), F(2)) is calculated using geometric shapes to represent the integral.
- 📉 The minimum values of F(X) are identified as occurring at X = 0 and X = 4 in the given example.
- 📈 The maximum value of F(X) is found at X = 1.5, where the area under the curve is the largest before it starts decreasing.
- 🧮 The process of integrating and then differentiating a function is shown to return to the original function, which is the essence of the second fundamental theorem of calculus.
- 📝 The integral of a function f(t) from a to X is calculated, and then the derivative of the resulting antiderivative is taken with respect to X.
- 🎓 The derivative of the antiderivative (capital F) with respect to X is shown to be f(X), disregarding any constant from the lower boundary.
- ⛓ When dealing with a chain rule, the derivative of the outer function (G(X)) is multiplied by the inner function's derivative.
- 🧐 Practice problems are solved, illustrating the process of finding derivatives of functions defined by integrals with variable bounds.
Q & A
What is the second fundamental theorem of calculus?
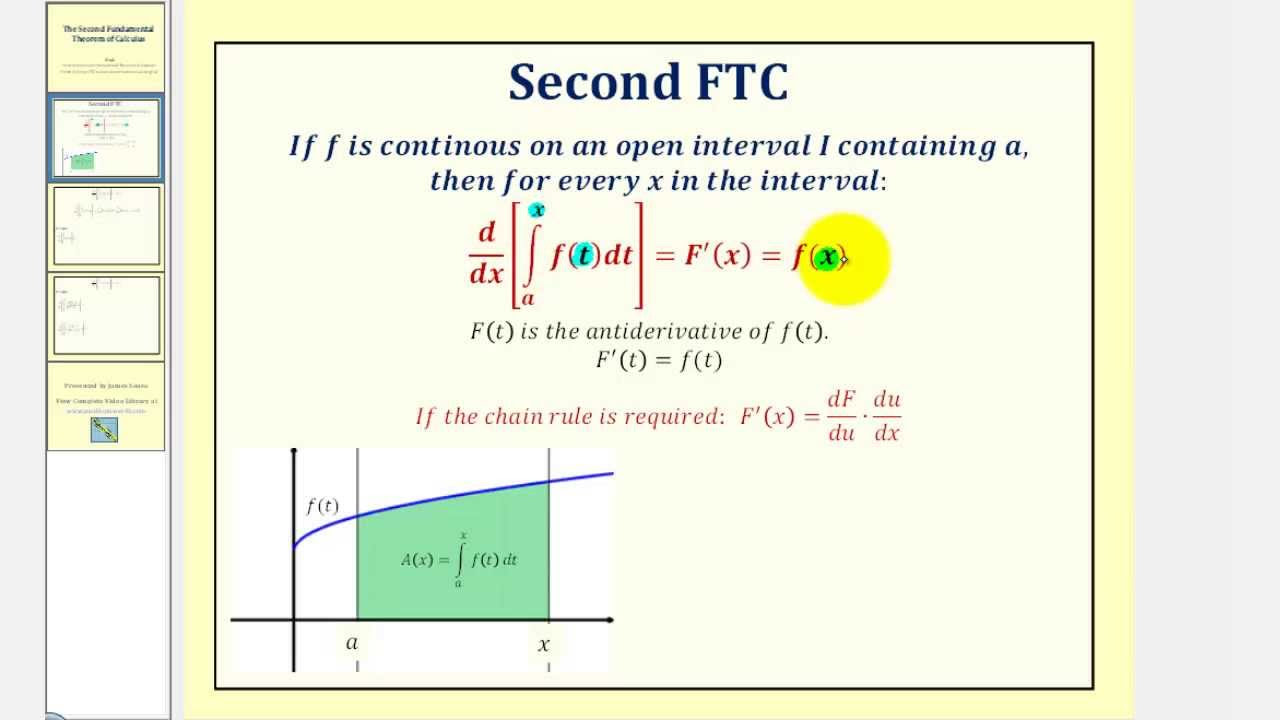
-The second fundamental theorem of calculus states that if you have a function defined by an integral from a constant lower boundary to a variable upper boundary, and you take the derivative of that function, you get the original function that was integrated, minus the value of the function at the constant lower boundary.
What does it mean for a function to be defined by an integral?
-A function is defined by an integral when one of the bounds of the integral (either the lower or upper bound) is a variable, and the other is a constant or another function.
What is the process to find the minimum value of a function defined by an integral?
-To find the minimum value, you evaluate the function at specific points to see where the function value is the smallest. In the provided script, the minimum values occur at x = 0 and x = 4.
How do you find the maximum value of a function defined by an integral?
-The maximum value occurs at the point where the area under the curve is the largest before it starts decreasing. In the script, the maximum is at x = 1.5.
What is the significance of the constant lower boundary in the integral when taking the derivative?
-The constant lower boundary does not affect the derivative of the integral function because when you take the derivative of a constant, it becomes zero.
How does the chain rule apply when taking the derivative of an integral with a variable upper boundary?
-The chain rule is applied by plugging in the upper boundary variable into the integrand and then multiplying by the derivative of the upper boundary variable.
What is the area of a semicircle in terms of its radius?
-The area of a semicircle is half the area of a full circle, which is calculated as (PI * R^2) / 2, where R is the radius of the semicircle.
How can you split an integral with variables in both the lower and upper boundaries?
-You can split the integral by choosing a constant number between the two variables as a new boundary and then summing the results of the two separate integrals on either side of this chosen number.
What happens to the integral when you change the order of the boundaries in an integral with variables?
-If you change the order of the boundaries, the integral becomes negative because you are integrating in the opposite direction.
Why does the specific number chosen as the new boundary when splitting an integral not affect the final result?
-The specific number chosen does not affect the final result because when taking the derivative of the integral (to find the antiderivative), the derivative of a constant is zero, thus the constant disappears.
How does the process of finding the derivative of an integral with a variable upper boundary simplify when using the second fundamental theorem of calculus?
-The process simplifies because instead of finding the antiderivative and then taking the derivative, you can directly substitute the variable upper boundary into the integrand and apply the chain rule if necessary, which is a quicker method.
Outlines
📚 Introduction to the Second Fundamental Theorem of Calculus
The video begins with an introduction to the second fundamental theorem of calculus, emphasizing its importance and reassuring that the content will be relatively easy to understand. The concept of a function defined by an integral is introduced, where one of the bounds is a variable. The function F(X) is exemplified as the antiderivative of another function f(t) over an interval from 0 to X. The process of evaluating F(X) for different values of X is demonstrated using geometric shapes to represent the integral's result.
🔍 Analyzing the Function's Behavior and Finding Extremes
The video continues with an analysis of the function F(X)'s behavior by plotting it on a graph and observing the area under the curve. The presenter calculates the minimum and maximum values of F(X), identifying the points at which these occur. The concept of integration from 0 to X of a function f(t) is explained, and the process of finding the derivative of the integral is demonstrated. This leads to the statement of the second fundamental theorem of calculus, which is the key takeaway of the lesson.
🧮 Applying the Second Fundamental Theorem to Find Derivatives
The presenter shows how to use the second fundamental theorem to find the derivatives of various functions. The process involves plugging in the upper boundary variable into the antiderivative and then applying the chain rule when necessary. Several examples are given to illustrate the technique, including handling functions with more complex upper bounds. The simplicity and efficiency of this method are highlighted as a shortcut to finding derivatives.
🔄 Dealing with Variables in Both Boundaries and Final Practice
The video concludes with a discussion of more complex scenarios where variables are present in both the lower and upper boundaries of the integral. The presenter demonstrates how to split the integral into parts and handle the change in direction when switching boundaries. The derivative of the resulting function is calculated, and the video ends with an encouragement to practice these problems, which are similar to those found on AP exams. The lesson is summarized, and the presenter bids farewell until the next lesson.
Mindmap
Keywords
💡Second Fundamental Theorem of Calculus
💡Antiderivative
💡Integral
💡Derivative
💡Function
💡Variable
💡Lower Bound
💡Upper Bound
💡Constant
💡Area Under the Curve
💡Chain Rule
Highlights
The lesson introduces the second fundamental theorem of calculus, emphasizing its importance and ease of understanding.
A function is defined by an integral where either the lower or upper bound is a variable.
The antiderivative of a function F, denoted as capital F, is illustrated through an example with an integral from 0 to X of f(t)dt.
Capital F of X is shown to equal zero when the lower and upper bounds are the same, demonstrating the concept of an integral with no area.
The process of calculating the area under the curve from 0 to 1 is demonstrated, resulting in a value of 1 for the function capital F at X=1.
The use of geometric shapes to calculate the area under the curve is explained, including the use of rectangles and triangles.
The concept of negative areas and how they affect the overall value of the integral is discussed.
The minimum values of capital F of X are identified as 0 and 4, indicating the points where the function reaches its smallest values.
The maximum value of capital F of X is found at X equals 1.5, where the area under the curve is at its largest before becoming negative.
The integral of a function is evaluated from 0 to X, and the process of finding the antiderivative is explained.
The second fundamental theorem of calculus is introduced, stating that the derivative of the integral (antiderivative) of a function is the function itself.
The application of the chain rule when the upper bound of the integral is a function of X is demonstrated.
Practice problems are provided to reinforce the concept of taking the derivative of an integral, showcasing a shortcut method.
The derivative of a function with an upper bound of X cubed is calculated, illustrating the use of the chain rule.
The method for dealing with variables in both the lower and upper bounds of an integral is discussed, using the properties of integrals to split the integral into parts.
The process of taking the derivative of an integral with variable bounds involves plugging in the bounds and applying the chain rule where necessary.
An example is given where the integral has a variable in both bounds, and the solution involves splitting the integral and applying the derivative.
The lesson concludes with a reminder of the importance of the second fundamental theorem of calculus and its practical applications in calculus problems.
Transcripts
Browse More Related Video

Calculus 1 - Integration: Proof of the Fundamental Theorem of Calculus

Derivatives of Integrals (w/ Chain Rule)

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Finding derivative with fundamental theorem of calculus: x is on both bounds | Khan Academy
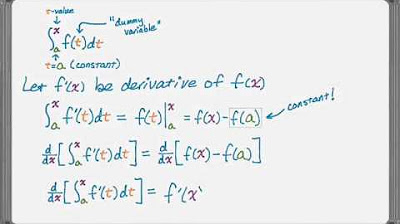
Second Fundamental Theorem of Calculus 2nd FTC
The Second Fundamental Theorem of Calculus
5.0 / 5 (0 votes)
Thanks for rating: