Compound Interest & Population Growth Word Problems - Logarithms
TLDRThe video script presents a series of mathematical word problems involving compound interest and population growth. It begins with a problem where $5000 is invested in an account with a 7% annual interest rate, compounded monthly, to calculate the final amount after 6 years. The script explains the formula for compound interest and applies it to find the final amount, which is approximately $7,653. It then explores continuous compounding, leading to a slightly higher amount. The video also addresses the time it takes for the initial investment to double, using logarithmic functions to solve for the time period. Subsequent problems involve calculating the expected dog population on an island with a 6% annual growth rate by 2025 and the year when the population will reach 1,000. Lastly, the script tackles bacterial growth, first with bacteria doubling every 20 minutes over 5 hours, resulting in 32 million bacteria, and then with bacteria tripling every 15 minutes over 6 hours, leading to 2.82 x 10^14 bacteria. The script is educational, providing clear explanations and mathematical steps for each problem.
Takeaways
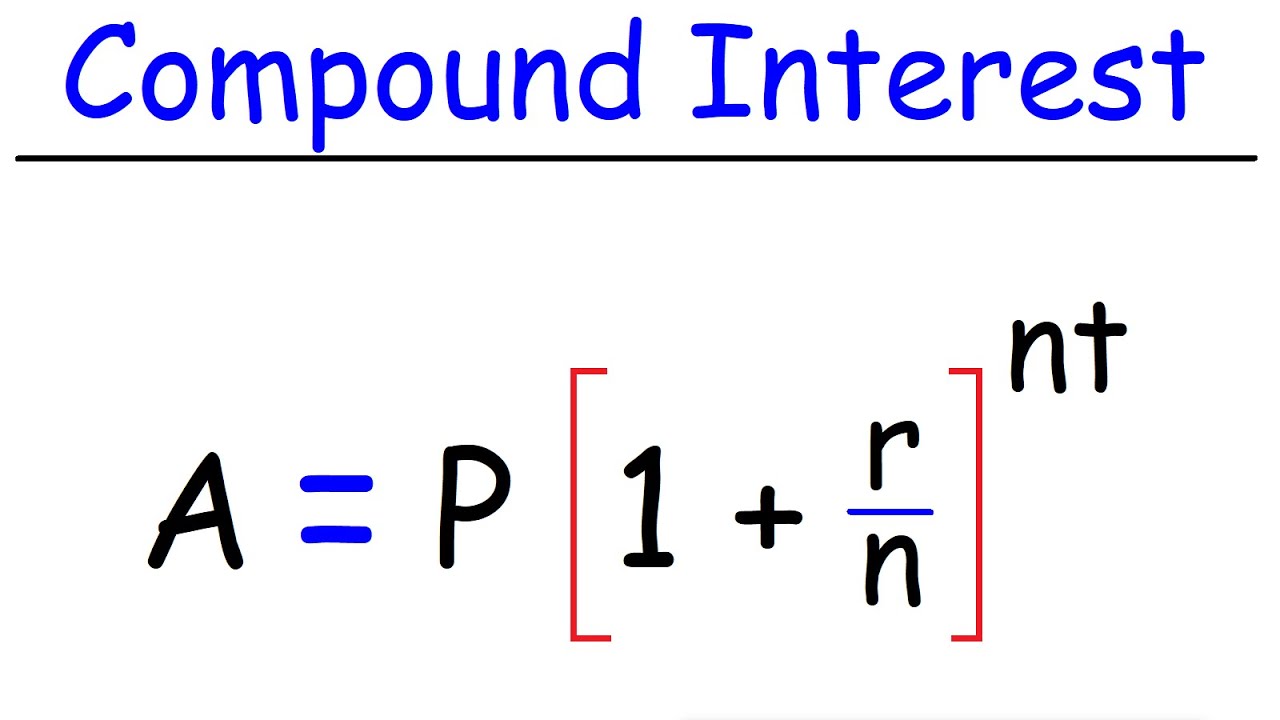
- 🧮 To calculate the final amount in an account with compound interest, use the formula: A = P(1 + r/n)^(nt), where A is the final amount, P is the principal, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time in years.
- 📈 The principal amount in the given example is $5,000, with an annual interest rate of 7% (0.07 as a decimal), compounded monthly (n = 12) over 6 years.
- 💵 After 6 years, the final amount in the account, with monthly compounding, would be approximately $7,653.03.
- 🔬 For continuous compounding, the formula is A = Pe^(rt), which results in a slightly higher amount compared to monthly compounding.
- ⏳ To find out how long it will take for the account to double, use the formula with natural logarithms: ln(2) = t * (ln(1 + r/n)/ln(1 + r)/n).
- 🐕 For population growth problems, the formula A = P(1 + r/n)^(nt) can also be used, where P is the initial population, r is the growth rate, n is the number of times the population increases per year, and t is the time in years.
- 🐾 If a population increases by a certain percentage each year, you can calculate future populations or the time it takes to reach a certain population size using the compound interest formula.
- 🌱 For exponential growth, like bacteria doubling, if the growth interval is known (e.g., every 20 minutes), you can determine future populations by raising 2 to the power of the number of intervals that occur over the time period.
- ⏱️ If bacteria triple every 15 minutes, and you want to find out the population after a certain number of hours, you first determine how many times the bacteria triple per hour and then apply the formula accordingly.
- 🔢 For complex exponential growth problems, scientific notation may be necessary to express the final population size.
- 📊 The concept of compounding can apply to various scenarios, including financial growth, population increases, and bacterial growth, each requiring slight modifications to the base formula to fit the context.
- 🧪 In all cases, understanding the base formula and the variables involved is crucial to accurately calculate and predict outcomes in exponential growth situations.
Q & A
What is the formula used to calculate the final amount in an account with compound interest?
-The formula used is A = P(1 + r/n)^(nt), where A is the final amount, P is the principal amount, r is the annual interest rate (as a decimal), n is the number of times interest is compounded per year, and t is the time in years.
If the interest rate is 7%, how do you express it as a decimal for the formula?
-To express the interest rate of 7% as a decimal, you divide 7 by 100, which gives you 0.07.
How often is the interest compounded if it is compounded monthly?
-If the interest is compounded monthly, the account is credited with interest 12 times a year, so n is 12.
What is the value of n if the interest is compounded daily?
-If the interest is compounded daily, the value of n is 365, as there are 365 days in a year.
What is the final amount in the account after 6 years with a principal of $5000, an annual interest rate of 7%, and monthly compounding?
-The final amount in the account after 6 years is approximately $7,653.00, calculated using the compound interest formula with the given values.
What equation is used for calculating the final amount when interest is compounded continuously?
-The equation used for continuous compounding is A = Pe^(rt), where A is the final amount, P is the principal, r is the annual interest rate as a decimal, and t is the time in years.
How much money will be in the account if it's compounded continuously for 6 years with a principal of $5000 and an annual interest rate of 7%?
-When compounded continuously for 6 years, the account will have approximately $7,609.81, using the continuous compounding formula.
How long will it take for an account to double in value if it starts with $5000, has an annual interest rate of 7%, and is compounded monthly?
-It will take approximately 9.95 years for the account to double in value, calculated using the formula for compound interest and solving for the time t.
If a population increases by 6% each year, how many dogs are expected to be on an island with an initial population of 200 dogs by the year 2025?
-By the year 2025, the expected number of dogs on the island will be about 479, using the formula for compound population growth.
In what year will the dog population on the island reach one thousand, starting from an initial population of 200 dogs with an annual increase of 6%?
-The dog population on the island is expected to reach one thousand by the year 2037, based on the compound population growth formula.
If a sample of bacteria counts 1000 and doubles every 20 minutes, how many counts of bacteria will there be in five hours?
-In five hours, the bacteria count will have doubled fifteen times, resulting in approximately 32,768,000 counts of bacteria.
If the bacteria triples every 15 minutes, how many counts of bacteria will there be in six hours, starting from an initial count of 1000?
-In six hours, if the bacteria triples every 15 minutes, the count will have tripled twenty-four times, resulting in approximately 2.82 x 10^14 counts of bacteria.
Outlines
💹 Compound Interest Calculations
This paragraph explains how to calculate the final amount in an account with a principal of $5000, an annual interest rate of 7%, and compounding interest applied monthly over 6 years. It introduces the formula A = P(1 + r/n)^(nt), where A is the final amount, P is the principal, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time in years. The paragraph also discusses the concept of continuous compounding using the formula A = Pe^(rt) and provides the final amounts for both scenarios. Lastly, it addresses the question of how long it would take for the account to double in value, using the formula A = P(1 + r/n)^(nt) and solving for t to find it would take approximately 9.95 years if compounded monthly.
🐕 Population Growth Prediction
The second paragraph deals with predicting the future population of dogs on an island, starting with 200 dogs and an annual growth rate of 6%. It uses the same compound interest formula to project the population at any given time, with P as the initial population, r as the growth rate, n as the number of times the population increases per year (annually, so n is 1), and t as the time in years. For the year 2025, it calculates the population to be approximately 479 dogs. It then solves for the year when the population will reach 1000 dogs, finding that it will occur around the year 2037. The paragraph also poses a related question about bacteria growth, which is left for the reader to solve.
🦠 Bacterial Growth Calculations
The third paragraph focuses on calculating the growth of bacteria, starting with a thousand counts of bacteria that double every 20 minutes. It provides two different equations based on whether the time frame is considered in minutes or hours. For a 5-hour period, the bacteria population is calculated to double 15 times, resulting in approximately 32,768,000 counts of bacteria. Additionally, the paragraph explores a scenario where bacteria triple every 15 minutes and calculates the population after 6 hours, resulting in approximately 2.82 x 10^14 counts of bacteria. It invites the reader to attempt a similar calculation for this scenario.
Mindmap
Keywords
💡Compound Interest
💡Principal
💡Interest Rate
💡Monthly Compounding
💡Continuous Compounding
💡Time (t)
💡Natural Logarithm
💡Population Growth
💡Exponential Growth
💡Bacteria Doubling
💡Scientific Notation
Highlights
The final amount in an account after 6 years with monthly compounded interest can be calculated using the equation A = P(1 + r/n)^(nt).
The principal amount (P) is the initial deposit of $5,000.
The annual interest rate (r) is 7%, which is 0.07 as a decimal.
The number of times interest is compounded per year (n) is 12 for monthly compounding.
For daily compounding, n would be 365, and for weekly, it's 52.
The final amount after 6 years with monthly compounding is approximately $7,653.
Continuous compounding uses the formula A = Pe^(RT), resulting in a slightly higher amount.
To find the time (t) it takes for an account to double in value, the equation A = P(1 + r/n)^(nt) is manipulated and solved for t.
Using natural logarithms helps solve for t when dealing with exponential equations.
It takes approximately 9.95 years for an account to double in value with monthly compounding.
The population growth of dogs on an island can be modeled using the same compound interest formula, with the population as P and the growth rate as r.
The expected number of dogs on the island by 2025 is about 479, given a 6% annual increase.
To find when the dog population will reach 1,000, the formula is solved for t, resulting in the year 2037.
Bacteria populations can be modeled similarly, with the initial count, doubling/tripling rate, and time period as variables.
In five hours, with bacteria doubling every 20 minutes, the count would be approximately 32,768,000.
If bacteria triple every 15 minutes, the count after six hours would be about 2.82 x 10^14.
The concept of compounding is applicable not only to financial growth but also to modeling population growth and bacterial proliferation.
Transcripts
Browse More Related Video
Exponential Growth and Decay Word Problems & Functions - Algebra & Precalculus

Exponential Growth

Exponential Growth and Decay Calculus Problem Solution | How To Find Relative Growth Rate

Compound interest and e (part 4) | Exponential and logarithmic functions | Algebra II | Khan Academy
Compound Interest

Review of Compound Interest (Precalculus - College Algebra 65)
5.0 / 5 (0 votes)
Thanks for rating: