Review of Compound Interest (Precalculus - College Algebra 65)

Professor Leonard

11 Nov 202046:13

EducationalLearning

32 Likes 10 Comments

TLDRThe video script presents a comprehensive exploration of the application of exponentials and logarithms in solving financial problems, particularly focusing on compound interest. It explains the concept of compound interest in various compounding scenarios, such as annually, semi-annually, quarterly, monthly, weekly, daily, and continuously. The script delves into the formulas associated with each compounding frequency, emphasizing the role of the principal (P), the future value (A), the interest rate (r), the number of compounds per year (n), and time (t) in determining the growth of an investment. The presenter also illustrates how to calculate the effective yield, which is the actual interest rate earned over one year when considering compounding. Furthermore, the script demonstrates how to solve for different variables in compound interest formulas, including time, present value, and rate, with a focus on using logarithms to address exponential equations. The video concludes with a discussion on the effective annual yield, contrasting the results between finite compounding periods and continuous compounding, highlighting the higher interest earned with continuous compounding.

Takeaways

📈 Compound interest is a powerful tool for modeling financial growth, and it's based on exponential functions.
🧮 The formula for compound interest involves the principal (P), the future value (A), the interest rate (r), the number of times interest is compounded per year (n), and the time (t) in years.
🔄 The difference between continuous and finite compounding is significant, with continuous compounding using the mathematical constant e, while finite compounding uses a different formula.
⏱️ Time is a crucial factor in compound interest calculations, and the number of times interest is compounded per year (n) can affect the growth of an investment.
💡 The concept of effective yield is introduced to show the actual interest earned over a year, which is typically higher than the nominal rate due to compounding.
🔢 Using a calculator is essential for accurate compound interest calculations, as the numbers involved can be very sensitive to rounding errors.
📊 To solve for unknown variables in compound interest problems, it's important to identify the missing variable and the appropriate formula to use.
🤔 Logarithms are used to solve exponential equations, particularly when finding the time it takes to reach a certain financial goal, such as doubling an investment.
💰 The formula for continuous compounding simplifies to using the natural exponential function e raised to the power of the product of the interest rate and time.
📉 The concept of effective annual yield is used to express the actual interest rate earned on an investment over one year, considering the effect of compounding.
🌐 Exponential growth, as modeled by compound interest, can be applied to various real-life scenarios, including financial investments and natural phenomena like population growth.

Q & A

What is the primary focus of the video?
-The video primarily focuses on the application of exponentials and logarithms in solving financial problems, specifically in the context of compound interest and its calculation.
What are the two formulas for compound interest discussed in the video?
-The two formulas for compound interest discussed are for interest compounded continuously and for interest compounded a finite number of times per year.
What does the variable 'n' represent in the compound interest formula?
-In the compound interest formula, 'n' represents the number of times the interest is compounded per year.
How does the concept of compound interest relate to natural growth or decay?
-Compound interest is an exponential model that can also describe natural growth or decay when uninhibited, such as the growth of a population like bacteria.
What is the significance of the mathematical constant 'e' in the context of continuously compounded interest?
-The mathematical constant 'e' is significant in continuously compounded interest because it represents the base of the natural logarithm, and as 'n' approaches infinity in the compounding formula, the formula's base approaches 'e'.
How does the frequency of compounding affect the growth of an investment?
-The more frequently interest is compounded, the greater the growth of an investment. This is because the principal balance on which interest is earned increases more frequently, leading to a larger amount of interest being added over time.
What is the effective yield of an investment?
-The effective yield of an investment is the actual amount of interest earned over a period, which is usually higher than the nominal interest rate due to the compounding effect.
How can one determine the amount that should be invested to reach a certain financial goal?
-To determine the amount that should be invested to reach a certain financial goal, one needs to identify the missing variable (present value), use the given interest rate, compounding frequency, and time period, and apply the appropriate compound interest formula to solve for the present value.
What is the process for solving exponential equations when the base is not common?
-When solving exponential equations with non-common bases, logarithms are used to isolate the exponential term. By applying a logarithm to both sides of the equation, the exponent can be moved to the front, allowing for the solution of the variable.
How long does it take to double an investment with a given interest rate and compounding frequency?
-To calculate the time it takes to double an investment, one can use the compound interest formula and solve for time 't' by setting the future value to twice the present value and applying logarithms to isolate and solve for 't'.
What is the effective annual yield and how is it calculated?
-The effective annual yield is the actual interest rate earned on an investment over one year, considering the compounding effect. It is calculated by taking the future value of an investment (considering a $1 investment), subtracting the principal, and then dividing by the principal, with the result expressed as a percentage.

Outlines

00:00

📈 Introduction to Exponentials and Logarithms in Finance

The video begins with an introduction to the application of exponentials and logarithms in solving financial problems, specifically focusing on interest. The speaker mentions that they won't go into depth as interest has been discussed in previous videos, but will provide a brief review for those unfamiliar. The main focus of the video is to understand how to solve for variables such as time and investment amount in different interest scenarios, including compound interest and continuous growth models.

05:00

💰 Compound Interest Formulas and Their Implications

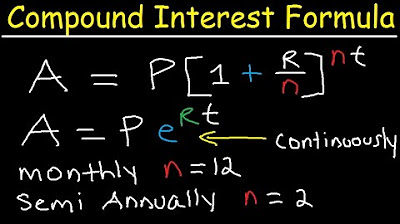
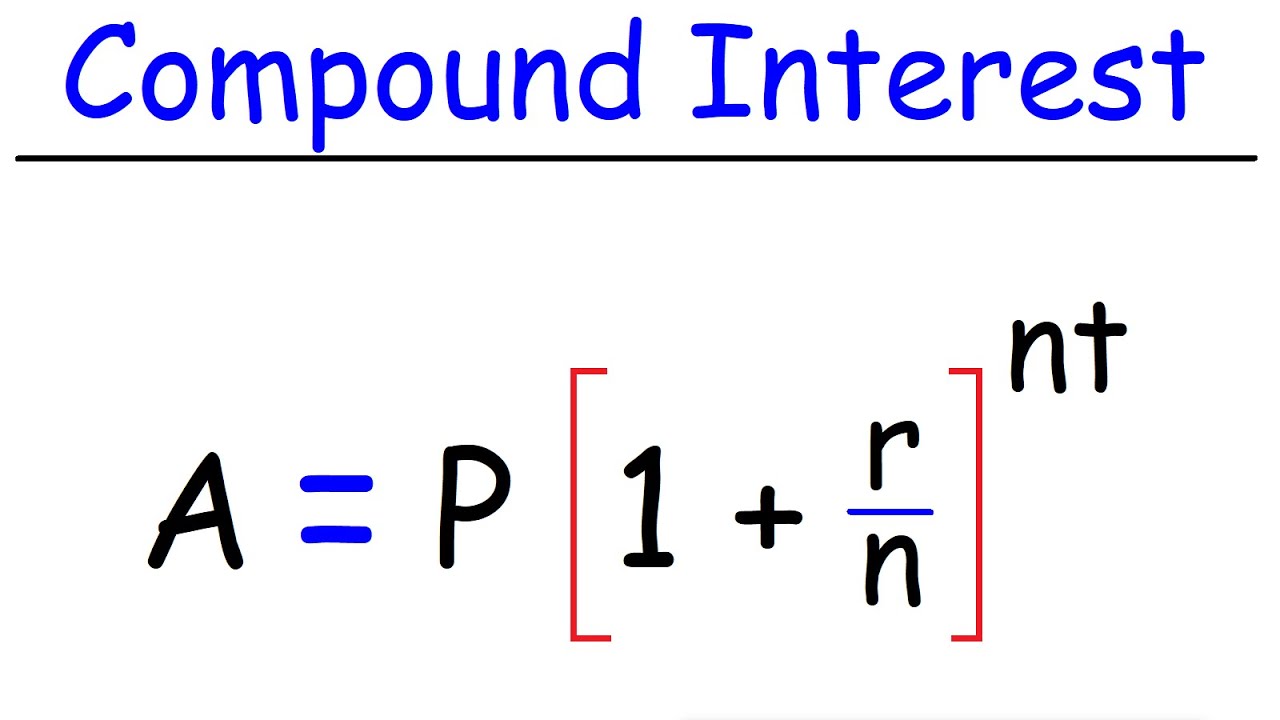
The speaker delves into the specifics of compound interest formulas, explaining the meaning of each variable within the formulas. They discuss the concept of principal (P), future value (A), interest rate (r), and time (t), emphasizing the importance of these variables in financial calculations. The speaker also introduces the idea of different compounding periods, such as annually, semi-annually, quarterly, and continuously, and how these affect the growth of an investment. The concept of effective yield is also touched upon, highlighting that compound interest results in more interest earned than the nominal rate suggests.

10:00

🔢 Solving Financial Problems with Compound Interest

The speaker provides a step-by-step guide on how to use the compound interest formulas to solve financial problems. They use a specific example of an investment of $3,000 at an annual interest rate of 9.25% compounded monthly for two and a half years. The speaker explains how to plug the values into the formula, the importance of using parentheses for accurate calculations, and the significance of avoiding rounding errors. The result is a future value of $3,777.17, demonstrating the power of compound interest over time.

15:01

🧮 Effective Rate and Continuous Compounding

The speaker discusses the concept of effective rate, which is the annual percentage rate that an investment actually earns when considering compounding. They differentiate between nominal rate and effective rate, explaining how the latter accounts for the compounding effect. The speaker then compares the effective rate of an investment compounded monthly to that of an investment compounded continuously, showing that continuously compounded interest yields slightly more growth over the same period. The speaker emphasizes the practicality of using logarithms to solve exponential equations in finance, especially when dealing with continuous compounding.

20:03

📊 Doubling Investments and Understanding Growth Rates

The speaker explores the concept of doubling an investment, explaining that the time required to double an investment is consistent regardless of the initial investment amount. They use the example of an investment at a 4.5% interest rate compounded quarterly to demonstrate how to calculate the time needed to double an investment. The speaker also discusses the use of logarithms in solving for the time it takes to achieve a doubling or any other multiplication factor in investments. They further illustrate the process with an example of continuous compounding at a 4.5% rate, reinforcing the effectiveness of logarithms in financial calculations.

25:03

🤔 Applying Exponential Concepts to Real-Life Finance

The speaker concludes the video by recapping the key concepts discussed, including the application of exponentials and logarithms in finance, the importance of understanding compound interest, and the calculation of effective rates. They also touch on the practical use of these concepts in real-life financial scenarios, such as investments, loans, and savings. The speaker aims to make complex financial topics more accessible and understandable, encouraging viewers to apply these mathematical tools to better manage their finances.

Mindmap

Keywords

💡Exponential growth

Exponential growth refers to a phenomenon where a quantity increases at a rate proportional to its current value. In the video, it is used to describe how compound interest can cause an investment to grow over time. The concept is central to the video's theme of financial modeling, as it illustrates how small initial investments can grow significantly through the power of compounding.

💡Logarithms

Logarithms are mathematical operations that allow for the simplification of exponential expressions. They are used in the video to solve for variables within exponential equations, such as determining the time it takes for an investment to double. The video demonstrates the use of logarithms in financial calculations, particularly when dealing with compound interest and to find the effective annual yield.

💡Compound interest

Compound interest is the interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. The video explains the concept in detail, showing how it can be modeled using exponential functions. It is a key concept in the video as it is the main application of exponentials and logarithms in solving financial problems.

💡Principal

The principal refers to the original amount of money invested or borrowed, before any interest is added. In the video, it is used in the context of compound interest formulas to illustrate how the principal amount grows over time. The concept is fundamental to understanding the growth of an investment or the cost of borrowing money.

💡Future value

Future value is the amount of money that a current sum of money is worth at a future date, taking into account the effects of compound interest. The video discusses how to calculate future value using different compounding periods, such as annually, monthly, or continuously. It is a critical concept for investors and borrowers to understand the potential growth or cost of their money over time.

💡Interest rate

The interest rate is a percentage of an amount of money, which banks or other financial institutions charge for lending money or for which they pay you for depositing money. The video emphasizes the importance of the interest rate in determining the growth of an investment or the cost of a loan, with higher rates leading to faster growth or higher costs.

💡Continuous compounding

Continuous compounding is a method of calculating compound interest where the interest is added an infinite number of times, at every instant. The video explains that this method can be modeled using the mathematical constant e (Euler's number). It is used to show how investments can grow more rapidly with continuous compounding compared to other compounding periods.

💡Effective yield

Effective yield, also known as the effective annual rate, is the actual rate of return on an investment when taking into account the effects of compounding. The video demonstrates how to calculate the effective yield to understand the true rate of interest earned on an investment over a year, which is often higher than the nominal rate due to compounding.

💡Time

In the context of the video, time refers to the duration for which an investment is held or a loan is taken out, typically expressed in years. It is a key variable in compound interest formulas, as the length of time can significantly affect the final amount of money, with longer periods leading to more substantial growth or costs.

💡Number of compounds

The number of compounds, denoted as 'n' in the video, refers to how many times interest is compounded within a year. It is used in the compound interest formula to determine the frequency of compounding, which can be annually, semi-annually, quarterly, monthly, weekly, daily, or more frequently. The video explains how the number of compounds affects the growth of an investment or the cost of a loan.

💡Calculator

A calculator is an electronic device used for performing calculations. In the video, the presenter emphasizes the importance of using a calculator for accurate financial calculations, especially when dealing with sensitive financial numbers and complex exponential and logarithmic operations. The calculator is used to perform the mathematical operations required to apply the concepts of compound interest and exponential growth.

Highlights

The video discusses the application of exponentials and logarithms in solving financial problems, specifically focusing on compound interest.

A brief review of compound interest is provided, including the formula for continuously compounded interest and its relation to natural growth or decay.

The presenter explains the meaning of the variables in the compound interest formula: principal (P), future value (A), interest rate (r), and time (t).

The importance of the number of compounds per year (n) is discussed, and how it affects the interest rate and time in the formula.

An example calculation is shown, illustrating how to calculate the future value of an investment with a given principal, rate, and time using monthly compounding.

The concept of effective yield is introduced, explaining why the actual interest earned over a year is more than the nominal rate suggests due to compounding.

The video demonstrates how to calculate the effective annual yield using both traditional and continuously compounded interest.

The presenter shows how to solve for missing variables in compound interest problems, such as the amount that should be invested to reach a certain goal.

The use of logarithms to solve exponential equations is explained, particularly when finding the time it takes to double an investment.

An example is provided to calculate how long it would take to double an investment with a 4.5% interest rate, compounded quarterly.

The difference between compounding continuously and with a finite number of compounds per year is highlighted, with an example showing the slightly shorter time for continuous compounding.

The presenter emphasizes the importance of accuracy when using a calculator for financial calculations, advising against intermediate rounding.

The video concludes with a promise to discuss exponential growth and decay, as well as an introduction to differential equations in the next video.

The role of the natural growth rate 'e' in continuously compounded interest is explained, showing its mathematical derivation from the limit of compounding as 'n' approaches infinity.

The impact of the number of compounds per year on the effective interest rate is detailed, showing how more frequent compounding results in a higher effective yield.

The video provides a step-by-step guide on using a calculator for compound interest calculations, emphasizing the need for parentheses to ensure correct order of operations.

The concept of the present value and future value in financial investments is clarified, with an explanation of how interest affects these values over time.

An in-depth look at the formula for compound interest with finite compounding periods is given, showing how to adjust the interest rate and time for different compounding frequencies.

Transcripts

Browse More Related Video

Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra

Compound Interest

Compound interest and e (part 2) | Exponential and logarithmic functions | Algebra II | Khan Academy

Introduction to compound interest and e | Algebra II | Khan Academy

Continuous Money Flow: Future Value with increasing rate of growth

Compound interest and e (part 3) | Exponential and logarithmic functions | Algebra II | Khan Academy

Related Tags

Exponential Growth Logarithms Compound Interest Financial Modeling Investment Strategies Effective Yield Continuous Compounding Doubling Time Interest Rate Financial Education