Exponential Growth and Decay Calculus Problem Solution | How To Find Relative Growth Rate

Jake's Math Lessons
20 Jun 202107:53
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging video, Jake introduces viewers to the concept of exponential growth and decay, specifically through the lens of bacterial growth exemplified by E. coli. Starting with an initial population of 60 cells, he explains how these bacteria divide every 20 minutes, leading to a rapid increase in population. The video is structured as a two-part problem: first, determining the relative growth rate, and second, deriving an expression for the number of cells after 't' hours. Jake simplifies the process by suggesting to solve part B (the expression for cell count over time) before part A, which involves calculating the growth rate. Using the formula for exponential growth, y(t) = y0 * e^(kt), where y0 is the initial cell count, 'e' is the base of the natural logarithm, 'k' is the growth rate, and 't' is time in hours, he demonstrates how to find 'k' by calculating the cell count after one hour. The final expression for the number of cells, y(t) = 60 * e^(natural log of 8) * t, provides a clear solution to part B. For part A, Jake reveals the relative growth rate as 207.9% per hour, offering a percentage for a more intuitive understanding. The video concludes with an encouragement to subscribe for more math lessons and support the community.

Takeaways
  • 🌱 The video discusses exponential growth and decay, specifically using the example of E. coli bacteria in a nutrient broth medium.
  • 📈 The initial population of the E. coli culture is 60 cells, which doubles every 20 minutes.
  • ⏱ Part A of the problem asks for the relative growth rate, while Part B asks for an expression representing the number of cells after 't' hours.
  • 🔑 The presenter suggests solving Part B first, as it will make finding the relative growth rate (Part A) easier.
  • 📊 The general formula for exponential growth or decay is given as y = y₀ * e^(k*t), where y₀ is the initial population, k is the relative growth rate, and t is time.
  • 🔍 To find the value of k (the relative growth rate), the presenter uses the known point after one hour when the population is 480 cells.
  • 🧮 By solving the equation 480 = 60 * e^(k*1), the value of k is found to be the natural logarithm of 8.
  • 📐 The expression for the number of cells after 't' hours is derived as y(t) = 60 * e^(natural log of 8 * t).
  • 📌 The relative growth rate (Part A) is approximately 207.9% per hour, obtained by calculating the natural log of 8 and converting it to a percentage.
  • 👍 The presenter encourages viewers to like, subscribe, and join the community for more math lessons.
  • 🎓 The video aims to help viewers with their calculus homework and tests, making them easier to manage.
Q & A

  • What is the subject of the video?

    -The video is about exponential growth and decay, specifically focusing on a bacteria growth problem involving E. coli.

  • What is the bacterium used as an example in the video?

    -E. coli, a common inhabitant of the human intestines, is used as an example in the video.

  • How often does the E. coli bacterium divide in the example?

    -In the given example, the E. coli bacterium divides into two cells every 20 minutes.

  • What is the initial population of the E. coli culture in the problem?

    -The initial population of the E. coli culture is 60 cells.

  • What are the two parts of the question being addressed in the video?

    -Part A asks for the relative growth rate, and Part B asks for an expression for the number of cells after 't' hours.

  • Why does Jake suggest solving Part B before Part A?

    -Jake suggests solving Part B first because finding the expression for the number of cells after 't' hours will make finding the relative growth rate (Part A) easier.

  • What is the general formula for exponential growth or decay?

    -The general formula for exponential growth or decay is y = y_0 * e^(k*t), where y_0 is the initial amount, e is the base of the natural logarithm, k is the relative growth or decay rate, and t is time.

  • How does the video calculate the number of E. coli cells after one hour?

    -The video calculates the number of cells after one hour by doubling the initial population every 20 minutes, resulting in 480 cells after one hour.

  • What is the value of 'k' in the exponential growth formula for this problem?

    -The value of 'k' is the natural log of 8, which is approximately 2.079.

  • What is the relative growth rate of the E. coli culture per hour?

    -The relative growth rate of the E. coli culture per hour is 207.9 percent.

  • How does the video encourage viewers to engage with the content?

    -The video encourages viewers to like, subscribe, and hit the bell icon to join the Jake's Math Lessons community for more content and support.

  • What does the final expression for the number of cells after 't' hours look like?

    -The final expression is y = 60 * e^(natural log of 8 * t).

Outlines
00:00
📚 Introduction to Exponential Growth and Decay with E. Coli Bacteria

In this segment, Jake introduces the topic of exponential growth and decay, specifically focusing on bacteria problems involving E. Coli, a common bacterium in the human intestines. He explains that the bacterium divides every 20 minutes and starts with an initial population of 60 cells. The problem is divided into two parts: finding the relative growth rate (Part A) and deriving an expression for the number of cells after 't' hours (Part B). Jake suggests solving Part B first to make Part A easier. The general formula for exponential growth or decay is introduced, which is y(t) = y0 * e^(k*t), where y0 is the initial population, e is the base of the natural logarithm, k is the relative growth rate, and t is time in hours.

05:00
🧮 Solving for the Relative Growth Rate and Cell Population Expression

Jake continues by demonstrating how to use the exponential growth formula to find the relative growth rate and the expression for the cell population after 't' hours. He uses the information that the cell population doubles every 20 minutes to calculate the number of cells after one hour, which turns out to be 480 cells. By substituting the known values into the formula, he solves for the relative growth rate 'k', which is found to be the natural logarithm of 8. The expression for the number of cells after 't' hours is then given by y(t) = 60 * e^(natural log of 8 * t). Finally, Jake calculates the relative growth rate as approximately 207.9 percent per hour. He encourages viewers to like, subscribe, and join his community for more math lessons.

Mindmap
Keywords
💡Exponential growth
Exponential growth refers to the increase in a quantity where the rate of growth is proportional to the current quantity, resulting in growth at an increasingly rapid rate. In the context of the video, this concept is applied to a population of E. coli bacteria, which doubles every 20 minutes in a nutrient broth. The presenter uses this concept to demonstrate how the number of bacteria increases exponentially over time, leading to a rapid multiplication from the initial count.
💡E. coli
E. coli, or Escherichia coli, is a type of bacteria commonly found in the intestines of humans and other warm-blooded animals. It can serve as a model organism in microbiology studies. In the video, E. coli is used to illustrate the concept of exponential growth in microbiology, where the initial population in the experiment starts with 60 cells and grows as the bacteria divide every 20 minutes.
💡Relative growth rate
The relative growth rate in the context of the video refers to the constant rate at which the population of E. coli is increasing in the experiment. The presenter discusses finding this rate as part of solving the problem, indicating that it's a measure of how quickly the population grows relative to its size. The growth rate is crucial for calculating the population at any given time using the exponential growth formula.
💡Decay problems
Decay problems, often paired with growth problems in mathematical contexts, involve quantities that decrease over time, typically at rates proportional to their current value. While the video primarily discusses growth, decay is its conceptual opposite and is important in understanding the full spectrum of exponential change. This can apply to populations, radioactive decay, or depreciation of assets.
💡Calculus
Calculus is a branch of mathematics focused on change and motion, dealing with derivatives and integrals of functions. The video references calculus, particularly when discussing formulas and rates of change found in exponential growth and decay scenarios. The presenter also mentions resources like a calculus study guide to help viewers understand and apply these concepts.
💡Doubling time
Doubling time is the period it takes for a quantity to double in size or value at a constant growth rate. In the video, the doubling time for E. coli is specified as 20 minutes. This concept helps in understanding how quickly the population of bacteria reaches a certain size, which is crucial for predicting growth over several hours.
💡Natural logarithm
The natural logarithm (ln) is the logarithm to the base 'e', a mathematical constant approximately equal to 2.71828. It's used in calculating the time constants in growth and decay formulas. In the video, the natural logarithm of 8 is used to determine the relative growth rate 'k', demonstrating the mathematical process needed to solve exponential growth problems.
💡Population model
A population model is a mathematical representation of how populations change over time. In the video, the exponential growth formula Y(t) = Y0 * e^(kt) is used as a model to predict the number of E. coli cells over time, where Y0 is the initial population, k is the growth rate, and t is time. This model helps in understanding how biological and ecological systems evolve.
💡Function of time
A function of time in mathematics represents how a quantity changes over time. In the video, the population of E. coli is expressed as a function of time, Y(t), which allows for the calculation of the population at any given time based on the initial conditions and the growth rate. This concept is central to solving the problem presented in the video.
💡Cheat sheet
A cheat sheet in educational contexts refers to a concise set of notes used for quick reference. The presenter mentions a 'calculus cheat sheet' or 'integral calculus study guide' that viewers can use to understand and apply complex formulas related to exponential growth and decay, highlighting its utility as a learning aid in the video's theme of solving mathematical problems in calculus.
Highlights

Jake introduces an exponential growth and decay problem involving E. coli bacteria.

The initial population of E. coli in the culture is 60 cells.

Cells divide every 20 minutes, leading to exponential growth.

Part A of the problem asks for the relative growth rate, and Part B asks for the expression for the number of cells after 't' hours.

Jake suggests solving Part B first to make finding the relative growth rate easier.

The general form of the exponential growth formula is introduced: y = y0 * e^(k*t).

y0 is the initial population, e is the constant (approximately 2.7), k is the relative growth rate, and t is time.

To find k, Jake proposes using a known point on the growth curve, such as the cell count after one hour.

After one hour, the population is calculated to be 480 cells, starting from 60 cells.

The formula 480 = 60 * e^(k*1) is set up to solve for k.

By taking the natural log of both sides, k is found to be the natural log of 8.

The expression y(t) = 60 * e^(natural log of 8 * t) is derived to represent the number of cells after 't' hours.

The relative growth rate, Part A, is determined by calculating the value of k, which is approximately 2.079 or 207.9% per hour.

Jake encourages viewers to subscribe for more math lessons and support the channel.

The video concludes with a summary of the problem-solving process and an invitation to join the community for further learning.

The importance of finding the exponential expression to describe scenarios is emphasized.

The video provides a step-by-step guide to solving exponential growth problems using the formula y = y0 * e^(k*t).

The practical application of the formula is demonstrated through a real-world example of bacterial growth.

Jake's method of solving the problem showcases the relationship between the growth rate 'k' and the time variable 't'.

Transcripts

Browse More Related Video

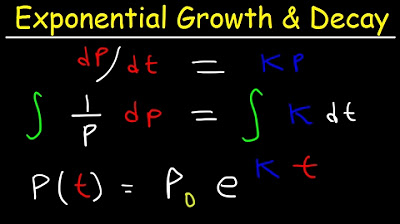
Exponential Growth and Decay Calculus, Relative Growth Rate, Differential Equations, Word Problems

Exponential Growth

2019 AP Calculus AB & BC Free Response Question #1

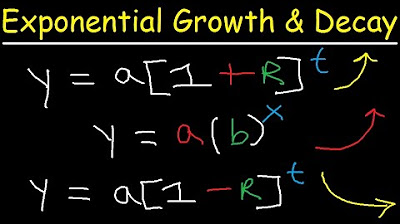
Exponential Growth and Decay Word Problems & Functions - Algebra & Precalculus

AP Calculus AB - 7.8 Exponential Models With Differential Equations

Modeling population with simple differential equation | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Exponential GrowthMath ProblemBacteriaE. ColiDoubling TimeRelative Growth RateCalculusJake's LessonsPopulation DynamicsScientific CalculationEducational ContentYouTube Tutorial