Part 1, Solving Using Matrices and Cramer's Rule

Mr H Tutoring
21 Jun 202304:10
EducationalLearning
32 Likes 10 Comments

TLDRThe video introduces a novel method for solving systems of linear equations using matrices, specifically Cramer's Rule. It demonstrates the process with a step-by-step example involving two variables, highlighting the efficiency of this technique for more complex systems with multiple variables and equations. The video emphasizes the reduced likelihood of errors and time-saving benefits of Cramer's Rule over traditional methods, and teases an upcoming example with three variables to further illustrate its value.

Takeaways
  • 📚 Introduction to Cramer's Rule: The script introduces Cramer's Rule as a method for solving systems of linear equations using matrices, which is particularly useful for systems with three or more variables.
  • 🔢 Determinant Calculation: It explains the process of calculating the determinant of the coefficient matrix, which is fundamental to Cramer's Rule.
  • 📌 Matrix Setup: The script outlines the initial setup of the matrix by extracting coefficients from the given equations to form the determinant.
  • 🤔 Substitution Process: It details the process of substitution, where specific elements are replaced to find the determinants for the individual variables (DX and DY).
  • 📊 Determinant for X: The script demonstrates how to find the value for DX by substituting the column related to X and calculating the determinant.
  • 📊 Determinant for Y: Similarly, it shows how to find DY by focusing on the column related to Y and performing the determinant calculation.
  • 🔍 Solving for X: The method for solving for X is explained by dividing DX by D, the original determinant, to find the value of X.
  • 🔍 Solving for Y: The script describes how to solve for Y by dividing DY by D, yielding the value of Y.
  • 👍 Advantages of Cramer's Rule: The script highlights the advantages of Cramer's Rule, especially in saving time and reducing the chance of errors when dealing with multiple variables.
  • 📈 Complexity with More Variables: It acknowledges that while Cramer's Rule is efficient for higher variable counts, it may be less efficient for simple two-variable systems.
  • 🎥 Upcoming Content: The script teases future content, promising an example with three variables to further illustrate the value of Cramer's Rule.
Q & A

  • What is Cramer's Rule?

    -Cramer's Rule is a method for solving a system of linear equations with as many equations as there are variables, using determinants of matrices.

  • How does Cramer's Rule differ from other methods like substitution or elimination?

    -Cramer's Rule uses the concept of determinants of matrices to find the solution, which can be advantageous when dealing with systems of equations involving three or more variables, where other methods may become more complex and time-consuming.

  • What is the first step in applying Cramer's Rule?

    -The first step is to form the matrix of coefficients by taking the coefficients of the variables from the system of equations.

  • How do you calculate the determinant of a matrix in Cramer's Rule?

    -For a 2x2 matrix, the determinant is calculated as (a*d) - (b*c), where a, b, c, and d are the elements of the first two rows and columns of the matrix.

  • What is DX in the context of Cramer's Rule?

    -DX represents the determinant of a modified matrix where the column corresponding to the variable X (the first column) has been replaced with the constants from the right side of the equations.

  • What is DY in the context of Cramer's Rule?

    -DY represents the determinant of another modified matrix, similar to DX, but with the second column (corresponding to the variable Y) being replaced with the constants from the right side of the equations.

  • How do you find the value of X using Cramer's Rule?

    -To find the value of X, you divide DX by the determinant D. The result gives you the value of X.

  • How do you find the value of Y using Cramer's Rule?

    -To find the value of Y, you divide DY by the determinant D. The result gives you the value of Y.

  • Why might Cramer's Rule be preferred over other methods for systems with more variables?

    -Cramer's Rule can be more efficient for systems with three or more variables because it simplifies the process of solving, reducing the chance of error and saving time compared to methods like elimination or substitution.

  • What is the main takeaway from the video script for someone learning Cramer's Rule?

    -The main takeaway is that Cramer's Rule provides an alternative method for solving systems of linear equations, especially useful for systems with multiple variables, and can be more efficient and less prone to mistakes in those scenarios.

  • How does the video script illustrate the application of Cramer's Rule?

    -The video script walks through a step-by-step process of applying Cramer's Rule to a system of two linear equations with two variables, showing how to calculate the determinants and use them to find the values of X and Y.

Outlines
00:00
📚 Introduction to Cramer's Rule

The paragraph introduces a novel method for solving systems of linear equations, Cramer's Rule, which utilizes matrices. It contrasts this approach with more traditional methods like elimination and substitution. The explanation begins with setting up the determinant of the coefficient matrix, using coefficients from a given system of two equations with two variables. The process of calculating the determinant to find the value of D is described in detail.

🔍 Calculation of DX and DY

This section delves into the specific steps for calculating DX and DY, which are essential for applying Cramer's Rule. It explains how to modify the coefficient matrix by replacing elements to form new matrices for which the determinants are then computed. The paragraph outlines the process of finding DX by replacing the first column with the constants from the right side of the equations and similarly, finding DY by considering only the first column and modifying the rest of the matrix accordingly. The arithmetic involved in these calculations is also explained.

Mindmap
Keywords
💡Cramer's Rule
Cramer's Rule is a mathematical method used to solve a system of linear equations with as many equations as there are variables. It relies on the concept of determinants, which are calculated from the coefficients of the variables in the equations. In the video, the presenter uses Cramer's Rule to solve a two-variable system, demonstrating how it can be applied to find the values of x and y. The method is particularly useful for systems with three or more variables, where traditional elimination or substitution methods can be more cumbersome.
💡Matrices
A matrix is a rectangular array of numbers, symbols, or expressions, arranged in rows and columns. In the context of the video, matrices are used to represent the system of linear equations, with the coefficients of the variables forming the rows of the matrix. The determinant of a matrix, a scalar value derived from the matrix's elements, plays a crucial role in Cramer's Rule. The video demonstrates how to manipulate matrices to solve for the variables x and y.
💡Determinant
The determinant is a scalar value that can be computed from the elements of a square matrix. It is a fundamental concept in linear algebra and has various applications, including finding the invertibility of a matrix and solving systems of linear equations through Cramer's Rule. In the video, the determinant is used to find the values of x and y by comparing the determinants of the original matrix and the modified matrices for each variable.
💡Linear Equations
Linear equations are mathematical equations in which the highest power of the variable is one. They represent straight lines in a two-dimensional space and are the focus of the video. The script discusses solving a system of two linear equations with two variables using Cramer's Rule, which is an alternative to methods like substitution or elimination.
💡Substitution
Substitution is a method used to solve systems of linear equations by expressing one variable in terms of another from one equation and then substituting it into the other equation. While the video focuses on Cramer's Rule, substitution is mentioned as a common method for solving two-variable systems, which is typically quicker but may not scale as efficiently for larger systems.
💡Elimination
Elimination is a technique for solving systems of linear equations by adding or subtracting equations to eliminate one variable, making it easier to solve for the remaining variable. Like substitution, this method is efficient for two-variable systems but can become complex with more variables. The video introduces Cramer's Rule as a more powerful tool for systems with multiple variables.
💡Variables
In mathematics, a variable is a symbol that represents a number that can change. In the context of the video, x and y are variables in the system of linear equations being solved. The goal is to find the values of these variables that satisfy both equations simultaneously. The video demonstrates how Cramer's Rule can be used to find the values of x and y.
💡Coefficients
Coefficients are the numerical factors multiplying the variables in a linear equation. They determine the slope of the line represented by the equation. In the video, the coefficients of the variables x and y are used to construct the matrix and calculate the determinants needed for Cramer's Rule.
💡Video Script
A video script is a written plan for the content of a video, including dialogue, narration, and descriptions of visual elements. The provided transcript is a video script that outlines the steps and explanations for using Cramer's Rule to solve a system of linear equations with two variables, and it introduces the method in a way that is accessible to viewers who may not be familiar with the concept.
💡Educational Content
Educational content refers to material designed to teach or instruct viewers on a particular subject. The video script provided is an example of educational content, as it aims to teach the viewer about Cramer's Rule and its application in solving systems of linear equations.
💡Solving Equations
Solving equations refers to the process of finding the values of the variables that satisfy the equations. In the context of the video, this involves using Cramer's Rule to solve a system of linear equations with two variables, demonstrating how to calculate the determinants and use them to find the values of x and y.
Highlights

Introduction to a new method for solving systems of equations using matrices.

Explaining Cramer's Rule as an alternative to elimination and substitution methods.

Step-by-step process of finding the determinant of the coefficient matrix.

Calculation of the determinant resulting in the value of 10.

Procedure for finding DX by replacing elements in the first column and recalculating the determinant.

Result of DX calculation, obtaining a value of negative 20.

Explanation of how to find DY by altering the first column and calculating the determinant again.

DY calculation resulting in a value of 5.

Method to find the value of X by dividing DX by D.

Determination of X's value as negative 2.

Process for finding the value of Y by dividing DY by D.

Conclusion that Y equals two and a half or 2.5.

Discussion on the practicality and efficiency of Cramer's Rule for higher variables and equations.

Advantages of Cramer's Rule in reducing the time and effort required to solve complex systems of equations.

Preview of an upcoming video demonstrating Cramer's Rule with three variables.

Encouragement for viewers to subscribe and engage with the content.

Transcripts

Browse More Related Video

PreCalculus - Matrices & Matrix Applications (32 of 33) Using Cramer's Rule to Find x=? y=?

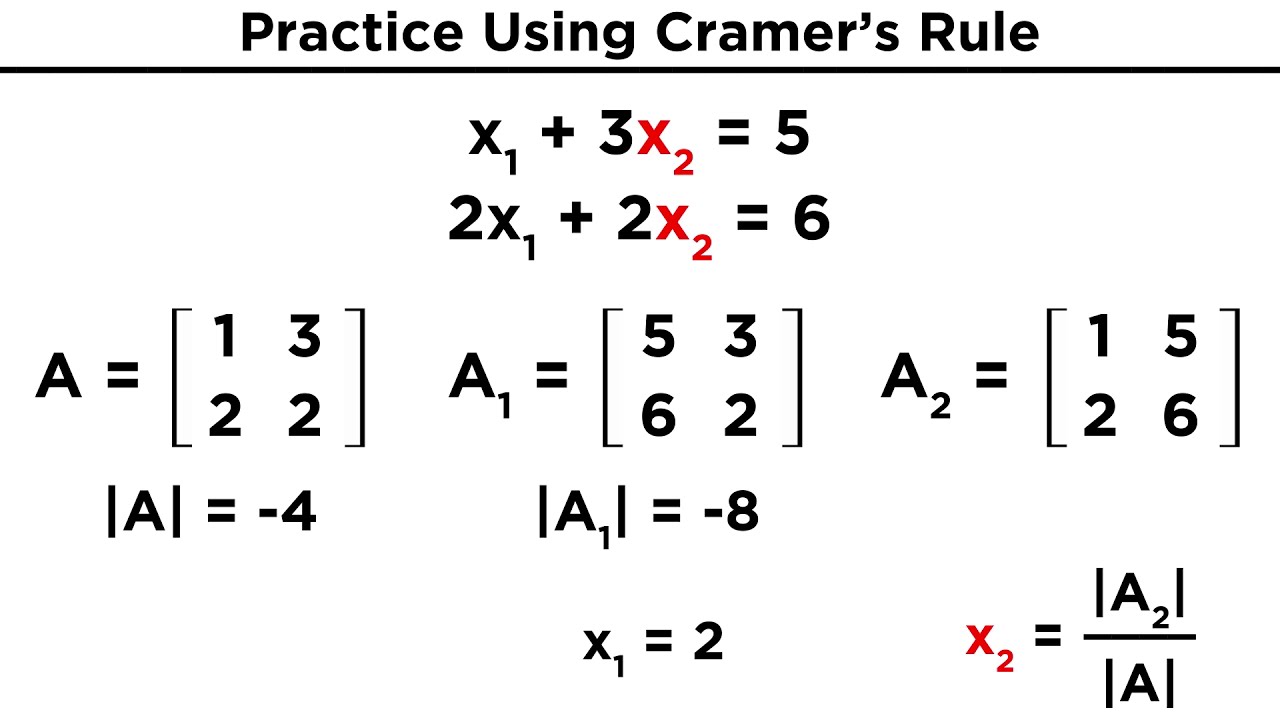
Solving Systems Using Cramer's Rule

PreCalculus - Matrices & Matrix Applications (3 of 33) Row Echelon Form

Substitution Method For Solving Systems of Linear Equations, 2 and 3 Variables, Algebra 2

PreCalculus - Matrices & Matrix Applications (6 of 33) Method of Gaussian Elimination: 2x2 Matrix

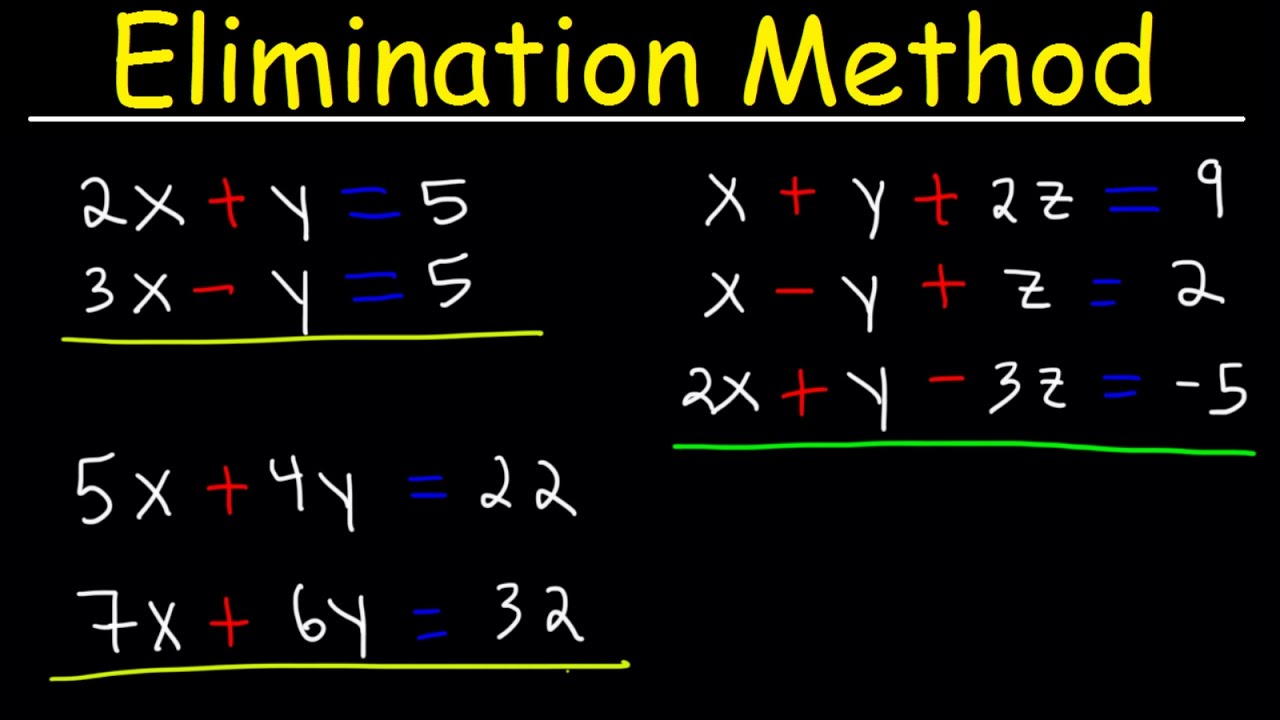
Elimination Method For Solving Systems of Linear Equations Using Addition and Multiplication, Algebr

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Cramer's RuleMatrix MathEquation SolvingMathematics TutorialLinear AlgebraEducational ContentMath TipsEfficient CalculationHigher VariablesMathematics Techniques