All Physics GCSE Equations EXPLAINED

Science Shorts
9 Apr 201820:00
EducationalLearning
32 Likes 10 Comments

TLDRThe video script is an informative guide for students preparing for their GCSE physics exams, focusing on the importance of understanding and memorizing key equations. It covers essential topics like Ohm's law, voltage, current, power, mechanics, and energy conservation, providing both the formulas and practical tips on how to apply them, especially in problem-solving and graph analysis. The script emphasizes the need to comprehend the meaning behind the equations to successfully tackle various physics problems and exams.

Takeaways
  • 📝 Ohm's Law (V=IR) is fundamental in electricity and is likely to be required for exams.
  • 🔌 Understanding voltage (V) as energy lost per Coulomb of charge is crucial for grasping electrical concepts.
  • 💡 The concept of current (I) represents the rate of flow of charge, measured in coulombs per second.
  • 🔋 Power in electricity is the rate of energy transfer or usage, measured in watts (joules per second).
  • 🚗 Newton's second law (F=ma) illustrates the relationship between force, mass, and acceleration.
  • 📈 In graphs, the gradient of a line representing distance vs. time can indicate speed.
  • 🚀 Acceleration is the change in velocity over time and is measured in meters per second squared.
  • 🌊 Wave speed (V) can be determined by frequency (F) times wavelength (λ), or V=fλ.
  • 📊 Density (ρ) is calculated by mass divided by volume, with units in kilograms per meter cubed.
  • 🔄 In any collision, the total momentum is conserved, with momentum (p) being the product of mass and velocity.
  • 🔓 Kinetic and gravitational potential energy can often be equated in physics problems involving motion and height.
Q & A

  • What is the most important equation to remember for electricity in the context of GCSE physics exams?

    -Ohm's Law is the most important equation, which is represented as V = IR, where V stands for voltage or potential difference, I is the current, and R is the resistance.

  • How can you rearrange Ohm's Law to find the resistance (R) when given values for voltage (V) and current (I)?

    -You can rearrange Ohm's Law to find the resistance (R) by using the formula R = V/I, where V is the voltage and I is the current.

  • What is the definition of voltage or potential difference (PD)?

    -Voltage or potential difference (PD) is the energy lost per Coulomb of charge. It can be represented as the energy (E) divided by the charge (Q), or mathematically as V = E/Q.

  • What does the term 'current' represent in the context of electricity?

    -Current represents the rate of flow of charge, typically measured in amperes (A), which is the number of coulombs (Q) of charge per second (t), represented as I = Q/t.

  • How is power calculated in the context of electricity?

    -Power is calculated as the rate of energy transferred or used, measured in watts (W). The equation for power is P = E/t, where E is energy in joules (J) and t is time in seconds (s). Alternatively, power can also be calculated using P = V * I, where V is voltage and I is current.

  • What is the relationship between power, voltage, and current when using Ohm's Law?

    -Using Ohm's Law, the relationship between power (P), voltage (V), and current (I) can be expressed as P = I^2 * R, where R is the resistance.

  • How is speed defined and what is its unit?

    -Speed is defined as the rate at which something moves, and it is represented as the distance (d) divided by the time (t) taken to cover that distance, mathematically expressed as v = d/t. The unit of speed is meters per second (m/s).

  • What is the equation for acceleration and what does it represent?

    -The equation for acceleration is a = ΔV/Δt, where ΔV is the change in velocity and Δt is the change in time. This equation represents the rate of change of velocity, indicating how quickly an object speeds up or slows down, and its unit is meters per second squared (m/s²).

  • What is Newton's second law of motion and how is it represented?

    -Newton's second law of motion states that force equals mass times acceleration, represented as F = ma, where F is the force, m is the mass of the object, and a is its acceleration.

  • How is work done by a force defined and what is its unit?

    -Work done by a force is defined as the energy transferred by the force, and it is measured in joules (J). The equation to calculate work done is W = F * d, where F is the force applied and d is the distance over which the force is applied.

  • What is the formula for kinetic energy and how can it be rearranged to find the speed (v)?

    -The formula for kinetic energy is KE = 1/2 * m * v², where m is the mass and v is the speed. To find the speed, the formula can be rearranged to v = √(2 * KE / m).

  • How can you calculate the frequency of a wave if you know the time period (T) of the wave?

    -The frequency of a wave can be calculated using the formula f = 1/T, where f is the frequency in Hertz (Hz) and T is the time period of the wave in seconds (s).

Outlines
00:00
📚 Understanding Core Physics Equations

This paragraph emphasizes the importance of understanding and remembering key physics equations, particularly for electricity and mechanics. It begins with a discussion on the necessity of knowing Ohm's law (V=IR) for electric exams and how understanding the principles behind the equations aids in memorization. The explanation then transitions to the concept of voltage (energy loss per Coulomb of charge) and current (rate of charge flow per second). The paragraph further explains power calculation (rate of energy transfer), offering alternative equations for calculating power in the context of electricity. The importance of unit recognition in understanding and deriving equations is highlighted throughout the discussion.

05:03
🏎️ Kinematics and Newton's Laws in Motion

This section delves into the realm of kinematics, focusing on the concepts of speed, acceleration, and Newton's second law of motion. It introduces the formula for average speed (distance/time) and explains how graphs can be used to determine speed from distance-time graphs. The crucial role of acceleration (change in velocity over time) is underscored, along with its unit (meters per second squared). The paragraph then presents Newton's second law (F=ma), illustrating the relationship between force, mass, and acceleration. Additionally, the concept of weight as a force (mg) is clarified, emphasizing its significance in physics problems.

10:04
🌐 Energy Conservation and Hooke's Law

The focus of this paragraph is on the conservation of energy and the application of Hooke's law. It introduces the concept of kinetic energy (1/2mv^2) and gravitational potential energy (mgh), and how they relate to each other in scenarios involving falling or thrown objects. The principle of energy conservation is highlighted, where kinetic energy equals potential energy at different points in a system. Hooke's law (F=kx) is also discussed, explaining the force exerted by a spring and how it relates to the spring constant and the extension of the spring. The paragraph rounds off by discussing momentum (mass x velocity), emphasizing its role in collisions and the conservation of total momentum.

15:06
🌊 Waves, Density, and Percentages in Physics

This paragraph covers a variety of topics, starting with wave properties, specifically the wave equation (V=fλ), where V represents the speed of the wave, f is the frequency, and λ is the wavelength. The concept of wavelength and time period is explained, along with how to derive frequency from the time it takes for a wave to form. The paragraph then moves on to discuss density, defined as mass per unit volume, and its implications. Lastly, the topic of percentages is addressed, with a focus on efficiency and percentage change. The importance of converting percentages to decimals for calculations is stressed, and the method for calculating percentage changes is outlined.

Mindmap
Keywords
💡Ohm's Law
Ohm's Law is a fundamental principle in physics and electrical engineering that states the relationship between voltage (V), current (I), and resistance (R) in a conductor. In the video, it is emphasized as a crucial equation to remember, represented as V = IR. It is used to explain how these three variables interact, and it is particularly important when analyzing electrical circuits and graphs in exams.
💡Voltage (V)
Voltage, or potential difference, is the energy lost per Coulomb of charge. In the context of the video, it is described as the energy per unit charge, represented as V = e/Q. Voltage is a key concept in understanding electrical circuits and is integral to Ohm's Law. It is also discussed in relation to graphs where voltage might be plotted against current.
💡Current (I)
Current is the rate of flow of electric charge, typically measured in amperes (A). In the video, it is explained as the number of coulombs passing through a conductor per second. Current is a fundamental concept in the study of electricity and is directly related to voltage and resistance through Ohm's Law.
💡Resistance (R)
Resistance is the opposition to the flow of electric current in a material. In the video, it is described using Ohm's Law, where resistance is calculated as the voltage divided by the current (R = V/I). Understanding resistance is essential for analyzing the behavior of electrical components and circuits.
💡Power
Power is the rate at which energy is transferred or converted. In the video, it is defined as energy per second and is measured in watts (W). The equation for power is given as P = E/t, where E is energy and t is time. Power is a crucial concept in understanding the efficiency and performance of electrical systems, and alternative equations for calculating power in electrical circuits are also discussed.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. In the video, it is defined by the equation ΔV/Δt, where ΔV is the change in velocity and Δt is the change in time. Acceleration is a fundamental concept in mechanics, describing how quickly an object speeds up, slows down, or changes direction.
💡Newton's Second Law
Newton's Second Law of Motion states that the force acting on an object is equal to the mass of that object multiplied by its acceleration (F = ma). In the video, this law is emphasized as a critical equation for understanding the relationship between force, mass, and acceleration. It is used to predict the motion of objects when forces are applied.
💡Momentum
Momentum is a measure of the quantity of motion of an object, defined as the product of mass and velocity (p = mv). In the video, it is highlighted as an important concept in physics, indicating how difficult it is to stop an object based on its mass and speed. The law of conservation of momentum is also mentioned, stating that total momentum is conserved in any collision.
💡Kinetic Energy
Kinetic energy is the energy that an object possesses due to its motion. In the video, the formula for kinetic energy is given as KE = 1/2 mv^2, where m is the mass and v is the velocity of the object. It is used to calculate the energy an object has when it is moving and is a key concept in understanding energy transformations in mechanical systems.
💡Gravitational Potential Energy
Gravitational potential energy is the energy an object possesses due to its position in a gravitational field. In the video, it is represented by the formula GPE = mgh, where m is the mass, g is the acceleration due to gravity (9.8 m/s^2 on Earth), and h is the height above a reference point. This concept is used to calculate the energy an object has because of its elevated position.
💡Wave Equation
The wave equation relates the speed of a wave (v) to its frequency (f) and wavelength (λ), expressed as v = fλ. In the video, this equation is introduced to explain how waves propagate through a medium. Understanding this relationship is essential for studying the properties and behavior of waves.
💡Density
Density is a measure of mass per unit volume of a substance. In the video, it is defined as mass divided by volume (ρ = m/V), where ρ (rho) represents density, m is mass, and V is volume. Density is an important property in physics and chemistry, used to characterize materials and calculate how much mass is contained in a given volume.
Highlights

The importance of understanding and remembering equations for success in physics exams, particularly at the GCSE and A-level.

Ohm's law (V=IR) as a fundamental equation in electricity that students must remember and its application in various physics problems.

The concept of voltage or potential difference (V) as the energy lost per Coulomb of charge and its representation in the form of energy divided by charge (V=e/Q).

The definition of current as the rate of flow of charge, measured per second, and its representation in the equation Q/t (current equals charge divided by time).

The calculation of power in electricity, which is the rate of energy transferred or used, measured in watts, and its relation to energy and time (P=E/t).

Alternative ways to calculate power, including P=V×I and P=I²R, which are derived from Ohm's law and are applicable in various electrical scenarios.

The concept of speed as a rate, defined as the distance traveled per unit of time, and its relation to the gradient of a distance-time graph.

Acceleration as the change in speed over time, and its significance in understanding how fast an object can accelerate, represented by the equation ΔV/Δt.

Newton's second law of motion (F=ma) that relates force, mass, and acceleration, highlighting that force results in acceleration but not necessarily a change in speed.

The concept of weight as a force, calculated as mass times the gravitational field strength (W=mg), with g being 9.8 m/s² on Earth.

Work done, which is the energy transferred by a force, measured in joules, and its calculation using Force Times Distance (W=F×d).

Hooke's law in relation to springs, where the force needed to pull a spring is equal to the spring constant (K) times the extension (X) of the spring.

Momentum, which represents the quantity of motion, calculated as mass times velocity (p=mv), and its conservation in collisions.

Kinetic energy, the energy of motion, calculated as half the mass times the speed squared (KE=1/2mv²), and its relationship with speed and mass.

Gravitational potential energy (GPE), calculated as mass times gravitational field strength times height (GPE=mgh), and its equivalence to kinetic energy in free-fall scenarios.

Wave equation relating the speed of a wave (V) to its frequency (F) and wavelength (λ), expressed as V=Fλ.

Density as a measure of mass per unit volume, calculated as mass divided by volume (ρ=m/V), and its units in kilograms per cubic meter.

Efficiency as a percentage, calculated by dividing useful energy by total energy and multiplying by 100, which is crucial in assessing energy usage.

Percentage change calculation, where the change is divided by the original amount and then multiplied by 100 to express it as a percentage.

Transcripts

Browse More Related Video

All GCSE physics equations

All IGCSE Physics Equations you need to know for the new syllabus

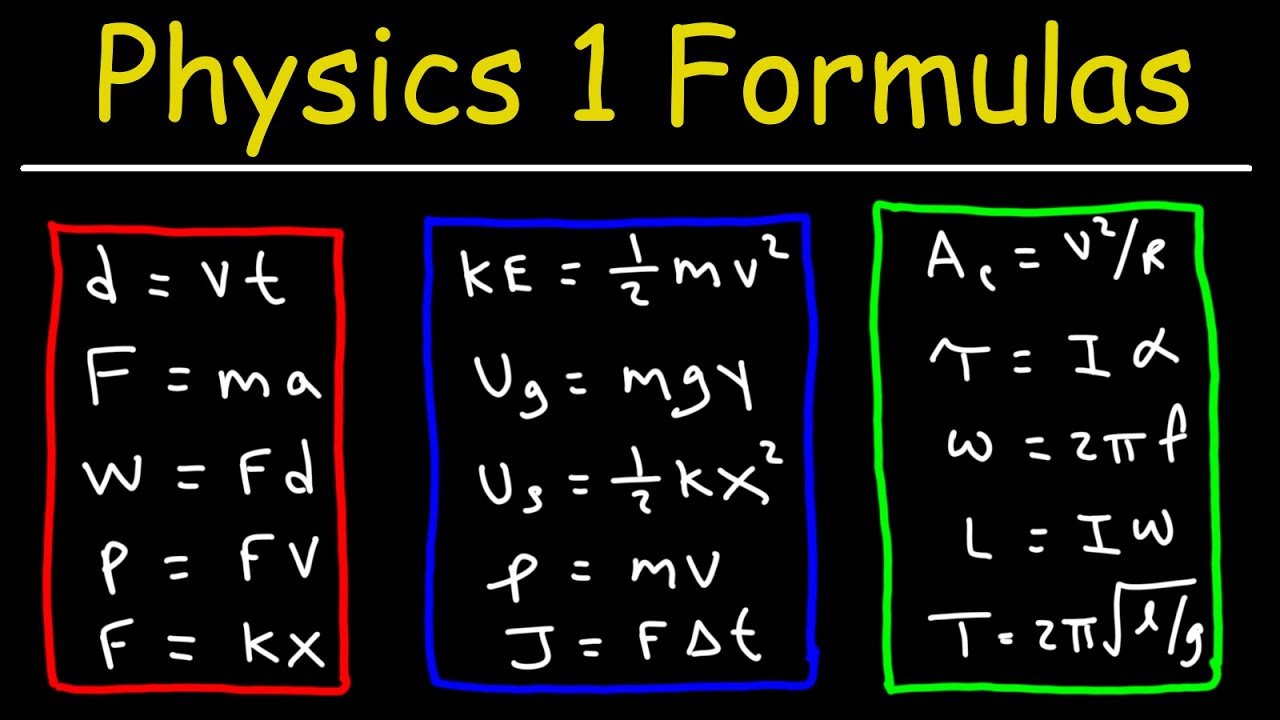
Physics 1 Formulas and Equations - Kinematics, Projectile Motion, Force, Work, Energy, Power, Moment

Physics 1 Final Exam Review

Dosage Calculations (Comprehensive Review)

Are You an Electrician? These are 5 Formulas You Should Know!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics EquationsStudy GuideExam PrepOhm's LawElectricity BasicsPower CalculationsSpeed & AccelerationNewton's LawsEnergy FormsWave PropertiesEfficiency & Percentages