Integration of Rational Functions into Logarithms By Substitution & Long Division

The Organic Chemistry Tutor
21 Feb 201719:19
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on integrating functions involving natural logs and rational functions. It begins with the fundamental anti-derivative of 1/x, which is the natural log of x, and progresses to more complex examples, demonstrating the use of absolute values, constant factorization, and substitution methods. The script also covers the application of long division for integration when dealing with higher-degree numerators. Each step is explained with clarity, making it an informative resource for those seeking to understand the intricacies of integration techniques.

Takeaways
  • 📚 The anti-derivative of 1/x is the natural log of |x| plus a constant (Ln|x| + C).
  • 🔄 When a constant is multiplied by a function, the constant can be moved to the front of the integral (7/x = 7*Ln|x| + C).
  • 📈 For integrals of the form 1/(x + k), use substitution with u = x + k, and du = dx to find the anti-derivative (Ln|x| + C).
  • 🔢 When the derivative inside the integral is a simple polynomial, like 1/(x + 5), use substitution to replace and simplify (Ln|x + 5| + C).
  • 🧩 For more complex fractions, like x^2 - 4 / x, separate the numerator and denominator into simpler parts and integrate each (1/2 * x^2 - 4*Ln|x| + C).
  • 🔍 Use long division to integrate complicated rational functions when substitution or other methods are not applicable (x^2 - 3x + 2 / x + 1 = x - 4 + 6*Ln|x + 1| + C).
  • 🌐 For integrals with a denominator of the form x^n, use substitution to simplify the expression and then integrate (x^2 + 7x + 12 / x + 3 = x^2/3 + 5*Ln|x - 3| + C).
  • 📊 When integrating a function with a high degree in the numerator compared to the denominator, consider long division (x^2 - 3x + 2 / x + 1 = x - 1 - 1/(x + 1) + C).
  • 🛠️ Use U-substitution to simplify integrals where the derivative of the integrand is directly related to the integrand itself (2x/(x - 1)^2 = 2*Ln|x - 1| - 2/(x - 1) + C).
  • 📋 When the numerator's degree is higher than the denominator and cannot be factored or simplified, long division is often the best approach (x^2 - 3x + 2 / x + 1 = x - 1 - 1/(x + 1) + C).
Q & A

  • What is the anti-derivative of 1/x?

    -The anti-derivative of 1/x is the natural log of x plus C, denoted as ∫(1/x) dx = ln|x| + C.

  • Why is an absolute value used when integrating 1/x?

    -An absolute value is used because the natural log function only accepts positive arguments, and x can be negative, so we take the absolute value to ensure the result is defined.

  • What is the anti-derivative of a constant divided by x?

    -The anti-derivative of a constant divided by x, such as 7/x, is the constant multiplied by the natural log of x plus C, which is ∫(7/x) dx = 7ln|x| + C.

  • How do you find the anti-derivative of 1/(x + 5)?

    -You can use substitution. Let u = x + 5, so du = dx. Then ∫(1/(x + 5)) dx = ∫(1/u) du = ln|u| + C = ln|x + 5| + C.

  • What is the anti-derivative of 3/(x - 4)?

    -The anti-derivative of 3/(x - 4) is 3 times the natural log of the absolute value of (x - 4) plus C, which is ∫(3/(x - 4)) dx = 3ln|x - 4| + C.

  • How do you integrate x/(x^2 - 3) using U-substitution?

    -Let U = x^2 - 3, then du = 2x dx. Now, x dx = du/2, and the integral becomes ∫(1/U) du = (1/2) ln|U| + C = (1/2) ln|x^2 - 3| + C.

  • What is the anti-derivative of (x^2 - 4)/x?

    -The anti-derivative is separated into two parts: ∫(x^2/x) dx + ∫(-4x/x) dx = x^2/2 - 4ln|x| + C.

  • How do you integrate ln(x^2)/x?

    -Let U = ln(x), then du = (1/x) dx. The integral ∫(ln(x^2)/x) dx = (1/3) ∫(u^2/x) du = (1/3) (xln(x) - u^2) + C = (1/3) (xln(x) - ln(x)^2) + C.

  • What is the anti-derivative of (x^2 + 7x + 12)/(x + 3)?

    -The expression (x^2 + 7x + 12) can be factored into (x + 4)(x + 3). After factoring, we can cancel out the (x + 3) term and integrate the remaining expression to get (1/2)x^2 + 4x + C.

  • How do you use long division to integrate a polynomial over a linear term?

    -Long division is used when the degree of the numerator is higher than the denominator. You divide the numerator by the denominator to find the quotient and remainder, then integrate each term separately.

  • What is the anti-derivative of (x^2 - 3x + 2)/(x + 1)?

    -Using long division, the fraction x^2 - 3x + 2 / x + 1 is equivalent to x - 4 + 2/(x + 1). The anti-derivative is then x^2/2 - 4x + 2ln|x + 1| + C.

  • How do you integrate 2x/(x - 1)^(2)?

    -Let U = x - 1, then du = dx. The expression 2x/U^2 becomes 2(U + 1)/U^2. The integral is ∫(2(U + 1)/U^2) du = 2(U + 1)ln|U| - 2/U + C = 2(x - 1 + 1)ln|x - 1| - 2/(x - 1) + C = 2ln|x| - 2/(x - 1) + C.

  • What is the anti-derivative of (x^2 - 2)/(x + 1)?

    -Using long division, the fraction x^2 - 2 / x + 1 simplifies to x - 1 - 2/(x + 1). The anti-derivative is (1/2)x^2 - x - 2ln|x + 1| + C.

Outlines
00:00
📚 Integration of Functions and Natural Logarithms

This paragraph introduces the concept of integrating functions that involve natural logarithms. It begins with an example of finding the anti-derivative of 1/x, which is shown to be the natural log of x plus a constant C. The video emphasizes the importance of using absolute values when dealing with natural log functions to avoid negative numbers. The paragraph proceeds to solve several similar problems, each involving the integration of a function and applying the concept of substitution to verify the solutions.

05:05
🔢 Using U-Substitution for Complex Integrals

This section delves into the method of U-substitution for solving more complex integrals. It demonstrates the process through several examples, starting with the integral of x^2 - 4 / x. The video explains how to break down the fraction and apply U-substitution, leading to the solution. It also covers the integral of x^2 + 7x + 12 / x + 3, where factoring is used to simplify the integral before applying long division. The paragraph emphasizes the use of various techniques, such as substitution and factoring, to find anti-derivatives of complex functions.

10:07
📈 Long Division and Factoring for Difficult Integrals

This paragraph focuses on the application of long division and factoring to solve difficult integrals. It begins with the integral of x^2 - 3x + 2 / x + 1, where the video shows how to perform long division and integrate the resulting simpler fractions. The paragraph then tackles the integral of x^3 - 3x^2 + 5 / x - 3, again using long division to simplify the expression before integrating. The video highlights the importance of recognizing when to use long division and when to factor expressions to find the anti-derivatives of given functions.

15:07
🌟 Advanced Integration Techniques and Problem Solving

The final paragraph of the video script explores advanced integration techniques, including the use of U-substitution for more challenging integrals. The video demonstrates how to integrate 2x / (x - 1)^2 by solving for x in terms of U and separating the fraction into simpler parts. The paragraph concludes with the integral of x^2 - 2 / x + 1, where long division is again used to simplify the expression. The video emphasizes the need to adapt and apply different integration strategies based on the characteristics of the function being integrated.

Mindmap
Keywords
💡anti-derivative
The anti-derivative, also known as the integral, is a fundamental concept in calculus that represents the reverse process of differentiation. In the context of the video, finding the anti-derivative of a given function, such as 1/x, involves determining another function whose derivative would be the original function. For instance, the anti-derivative of 1/x is the natural log of x, denoted as ln(x).
💡natural log
The natural log, often denoted as ln or log, is a logarithmic function with the property that the logarithm of a number is the exponent to which another fixed number, the base (usually e, where e is an irrational number approximately equal to 2.71828), must be raised to obtain that number. In the video, natural log functions are integral to finding the anti-derivatives of various functions, such as 1/x, where the anti-derivative is ln(x).
💡absolute value
The absolute value of a number is the non-negative value of that number without regard to its sign. It is used to ensure that only non-negative numbers are involved in calculations where negative values are not permissible, such as within the natural log function. In the video, absolute values are used to adjust expressions so that they can be input into natural log functions, which only accept positive arguments.
💡constant
In mathematics, a constant is a value that does not change. In the context of integration, constants can be factored out of the integral and are added to the result as a separate term. The video demonstrates this by showing that the anti-derivative of a constant divided by a variable, such as 7/x, results in the constant multiplied by the natural log of the variable, with the constant also included in the final answer as part of the constant C.
💡substitution
Substitution is a technique used in calculus to simplify complex integration problems by replacing a part of the integrand with a new variable. This makes the integration process more manageable, especially when dealing with complicated expressions. In the video, substitution is used to transform difficult integrals into more straightforward forms, such as replacing x + 5 with the variable U, which simplifies the process of finding the anti-derivative.
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function at any given point. It is used to find the slope of a curve at a specific point and is closely related to the concept of the anti-derivative. In the video, the derivative is used to understand the relationship between the integral and the original function, as well as to perform certain substitution methods.
💡integration by parts
Integration by parts is a technique used to integrate a product of two functions. It is based on the product rule for derivatives and involves breaking down the integral into simpler parts that can be more easily integrated. The video does not explicitly mention integration by parts but uses similar concepts to break down complex integrals into manageable pieces.
💡long division
Long division in the context of calculus refers to a method for dividing a polynomial by another polynomial, which can be used to simplify complex integrands. This technique helps in breaking down the numerator into terms that can be easily integrated, often resulting in a simpler expression to work with.
💡factoring
Factoring is the process of breaking down a polynomial into a product of other polynomials or factors. In the context of integration, factoring can simplify the integrand and make it easier to find the anti-derivative. The video shows how factoring can be used to simplify complex expressions before integrating.
💡U substitution
U substitution is a variant of the substitution method used in integration, where a part of the integrand is assigned to a new variable, called U. This allows for the simplification of the integral by changing the original variable to U and its derivative to a corresponding expression in terms of U. The video demonstrates U substitution by replacing x + 4 with U and then solving for the integral in terms of U before substituting back to the original variable.
💡power rule
The power rule is a basic rule in calculus that allows for the differentiation of a function raised to a power. It states that the derivative of x^n, where n is a constant, is nx^(n-1). In the context of integration, the power rule can also be applied in reverse to find anti-derivatives of power functions. The video does not explicitly mention the power rule but uses similar principles when integrating power functions.
Highlights

The anti-derivative of 1/x is the natural log of x plus C, which is a fundamental result in integration involving natural log functions.

When integrating functions with absolute values, such as the absolute value around x in the natural log function, it ensures that the result does not include negative numbers.

The method of constant factorization is demonstrated by moving the constant 7 in front of the natural log function when integrating 7/x.

The use of u-substitution is introduced as a technique to simplify integration, exemplified by its application in the integral of 1/(x + 5).

The concept of natural log is extended to include expressions like 5 - 2x by substituting and integrating accordingly, resulting in 4 times the natural log of the expression.

Integration by substitution is further illustrated with the example of 1/(5 - 2x), where the derivative and the integral are manipulated to solve for the anti-derivative.

The use of U-substitution for more complex fractions, such as in the case of x/(x^2 - 3), is demonstrated to simplify the integration process.

The technique of breaking down a fraction into smaller parts is shown, as in the case of x^2 - 4/x, which is separated into two simpler integrals.

The concept of exponentiation in natural logs is explored, as seen in the integral of ln(x^2)/x, resulting in a unique anti-derivative expression.

The method of factoring and canceling is introduced, as shown in the integral of (x^2 + 7x + 12)/(x + 3), where the expression is factored to cancel out x + 3.

Long division is used as an alternative integration method when factoring and substitution are not applicable, as demonstrated with the integral of (x^2 - 3x + 2)/(x + 1).

The process of long division is detailed, showing how to reduce complex fractions to simpler integrable forms, as seen in the example of 2x/(x - 1)^2.

Integration of rational functions with higher degree numerators than denominators is discussed, using long division to find the anti-derivative of the given function.

The video provides a comprehensive overview of various techniques in integration involving natural log functions, showcasing the versatility and application of these methods.

Transcripts

Browse More Related Video

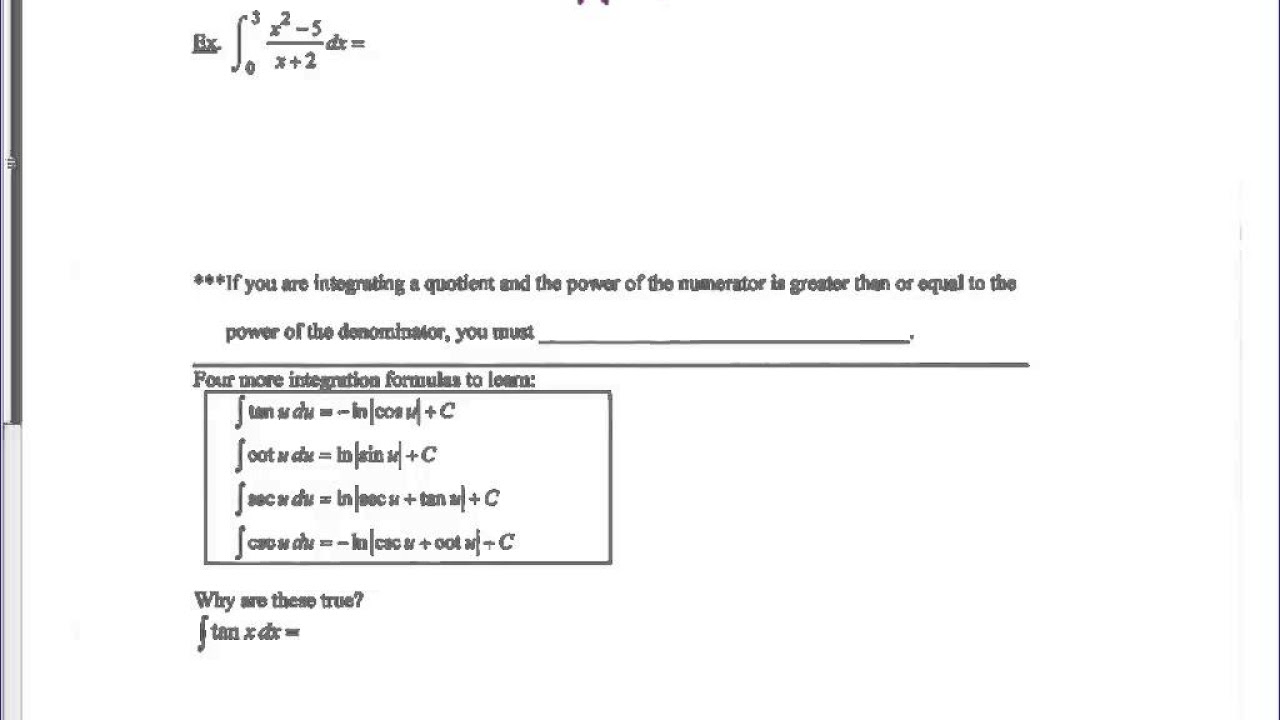
Natural Log Function Integration

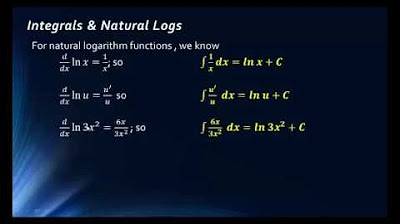
MATH 1325 Lecture 12 3 Integrals of Exponents and Logarithms

Dividing expressions to evaluate integral | AP Calculus BC | Khan Academy

Derivatives - Power Rule

Special Integration in a Linear Differential Equation Problem (Differential Equations 18)

Indefinite Integral of 7^lnx

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TutorialIntegration TechniquesLogarithmic FunctionsAnti-DerivativesMathematics EducationAdvanced MathSubstitution MethodComplex FunctionsOnline Learning