The Chain Rule for Derivatives in Calculus - [1-5]
TLDRThe Chain Rule is a fundamental concept in calculus that enables the differentiation of complex functions. It is particularly useful for handling nested functions, where the output of one function becomes the input for another. The rule states that the derivative of a composite function is the derivative of the outer function multiplied by the derivative of the inner function. Through examples, the video script demonstrates how to apply the Chain Rule to various scenarios, emphasizing its importance in solving a wide range of calculus problems. Understanding the Chain Rule is crucial for mastering calculus, as it is applicable in numerous mathematical contexts.
Takeaways
- 📚 The Chain Rule is fundamental in calculus, especially for taking derivatives of composite functions.
- 🔢 Don't confuse the Chain Rule with basic derivative rules; they apply to different scenarios.
- 🌟 The Chain Rule allows you to find derivatives of complex functions by breaking them down into simpler parts.
- 🧠 Remember that the Chain Rule involves taking the derivative of the outer function and multiplying it by the derivative of the inner function.
- 📈 When applying the Chain Rule, always start by identifying the 'big picture' function and the inner function.
- 👉 For example, in sin(x^2), the 'big picture' is sin(u) and the inner function is u = x^2.
- 🔁 The process of applying the Chain Rule often involves using it repeatedly for nested functions.
- 📊 Practice is key to mastering the Chain Rule; work through various examples to understand its application.
- 🌐 The Chain Rule is not limited to simple functions; it can be applied to a wide range of mathematical expressions.
- 💡 The Chain Rule is a powerful tool that opens up the ability to compute derivatives of many types of functions.
- 📚 Always practice caution when differentiating functions, ensuring not to fall into common traps or make incorrect assumptions.
Q & A
What is the primary focus of the Chain Rule section in calculus?
-The Chain Rule section focuses on teaching how to take derivatives of nested functions, which are functions that have one function inside another. It is considered the bread and butter of calculus, especially for taking derivatives.
Why is it a mistake to assume that the derivative of sine squared is just cosine squared times x?
-It's a mistake because the derivative rules apply only to functions that are in their exact form. For example, the derivative of sine is cosine, but this applies to 'sine of x' only. If the function is modified, like 'sine of x squared', you can't directly apply the rule without considering the modification, which in this case requires the use of the Chain Rule.
What is the Chain Rule's role in calculus?
-The Chain Rule is essential for taking derivatives of composite or nested functions, where one function is 'nested' inside another. It allows you to break down complex functions into simpler parts, take the derivative of each part separately, and then combine the results to find the derivative of the whole function.
How does the Chain Rule apply to the function f(x) = sin(x^2)?
-The Chain Rule applies by first taking the derivative of the outer function, which is the sine function, and then multiplying by the derivative of the inner function, which is x^2. The derivative of sin(x^2) is cos(x^2) * 2x, as per the Chain Rule.
What is the general formula for the Chain Rule?
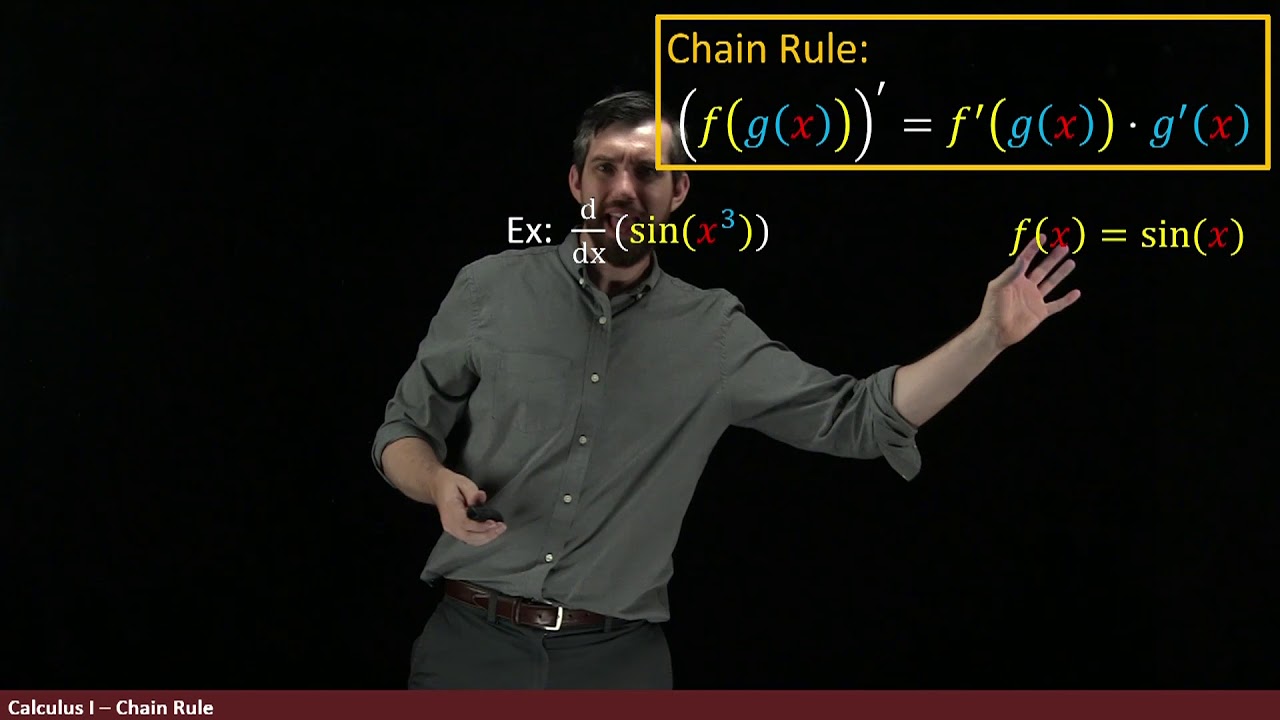
-The general formula for the Chain Rule is (d/dx)[f(g(x))] = f'(g(x)) * g'(x), where f'(x) is the derivative of the outer function and g'(x) is the derivative of the inner function.
Can you provide an example of a nested function other than the ones discussed?
-Yes, an example of a nested function is f(x) = cos(3x + 2). Here, the outer function is the cosine function, and the inner function is 3x + 2.
What is the derivative of the function G(x) = √(x^2 - 7x)?
-The derivative of G(x) = √(x^2 - 7x) is (x^2 - 7x)^(-1/2) * (2x - 7), which simplifies to (2x - 7) / (2√(x^2 - 7x)). This is derived by applying the Chain Rule and considering the exponent as a power of one-half.
How does the Chain Rule relate to the tangent function?
-The Chain Rule is used with the tangent function when the argument of the tangent is a function itself. For example, if you have tan(3x), you first take the derivative of the outer function (tangent), which is secant squared, and then multiply by the derivative of the inner function (3x), which is 3.
What is the process for finding the derivative of a function that is both cubed and squared, like (tan(x^3))^2?
-First, you take the derivative of the outermost function, which is squaring, resulting in 2*(tan(x^3)). Then, you take the derivative of the inner function, which is cubing, resulting in 3*(x^2). Finally, you multiply these two results together, and by the Chain Rule, you get 6x^2 * tan(x^3) * secant^2(x^3).
How does the Chain Rule apply to the function f(x) = (x - 6) / (x + 7)^3?
-To find the derivative of f(x) = (x - 6) / (x + 7)^3, you use the quotient rule, which is a specific case of the Chain Rule. You first find the derivatives of the numerator and the denominator separately, then apply the formula: (bottom derivative * top - top derivative * bottom) / (bottom squared). In this case, the derivative of the bottom (x + 7)^3 is 3(x + 7)^2 * (x + 7) derivative, and the derivative of the top (x - 6) is 1. The result is ((x + 7)^2 * (x - 6) - (x - 6) * 3(x + 7)^2) / (x + 7)^4.
What is the significance of understanding the Chain Rule in calculus?
-Understanding the Chain Rule is crucial because it allows you to handle more complex functions that can't be simplified with basic rules. It is a fundamental tool for solving real-world problems that involve rates of change and it opens up the ability to tackle a wide range of calculus problems.
Outlines
📚 Introduction to the Chain Rule
The paragraph introduces the Chain Rule as a fundamental concept in calculus, emphasizing its importance in deriving functions. It explains that while basic derivatives have been covered, the Chain Rule deals with more complex functions where one function is nested inside another. The讲师 uses the example of the function sine of x squared to illustrate the mistake of naively applying basic derivative rules and highlights the need to understand the Chain Rule to correctly derive such functions.
🔍 Explanation of the Chain Rule
This paragraph delves deeper into the essence of the Chain Rule, explaining it as a process of taking derivatives of nested functions. The讲师 clarifies that the Chain Rule involves taking the derivative of the outer function and then multiplying it by the derivative of the inner function. The explanation is supported by the formal definition of the Chain Rule and examples of nested functions like sine of 2x and tangent of x to the fourth power, reinforcing the concept with practical applications.
📈 Application of the Chain Rule in Derivatives
The paragraph demonstrates the practical application of the Chain Rule in deriving complex functions. It shows how to break down a function into its components, take the derivative of the outer function, and then multiply by the derivative of the inner function. The讲师 uses the example of a function with a square root and a power function to illustrate the process, emphasizing the importance of understanding the Chain Rule to tackle more complicated calculus problems.
🎓 Understanding the Chain Rule in Various Functions
This section further explores the application of the Chain Rule in different types of functions, such as tangent of 3x and functions raised to a power. The讲师 explains that the Chain Rule is not only applicable to the examples given but is a general method for finding derivatives of composite functions. The paragraph also highlights that the Chain Rule has been implicitly used in previous examples, and mastering it is crucial for solving a wide range of calculus problems.
🌟 Advanced Application of the Chain Rule
The paragraph presents a more complex application of the Chain Rule, involving functions like sine cubed of x and cosine cubed of x. The讲师 shows how to apply the Chain Rule to each term separately and then combine the results. The explanation includes simplifying the expression by factoring out common terms, demonstrating a deeper level of understanding and manipulation of the Chain Rule.
📚 Final Example: Chain Rule with Division and Cubic Functions
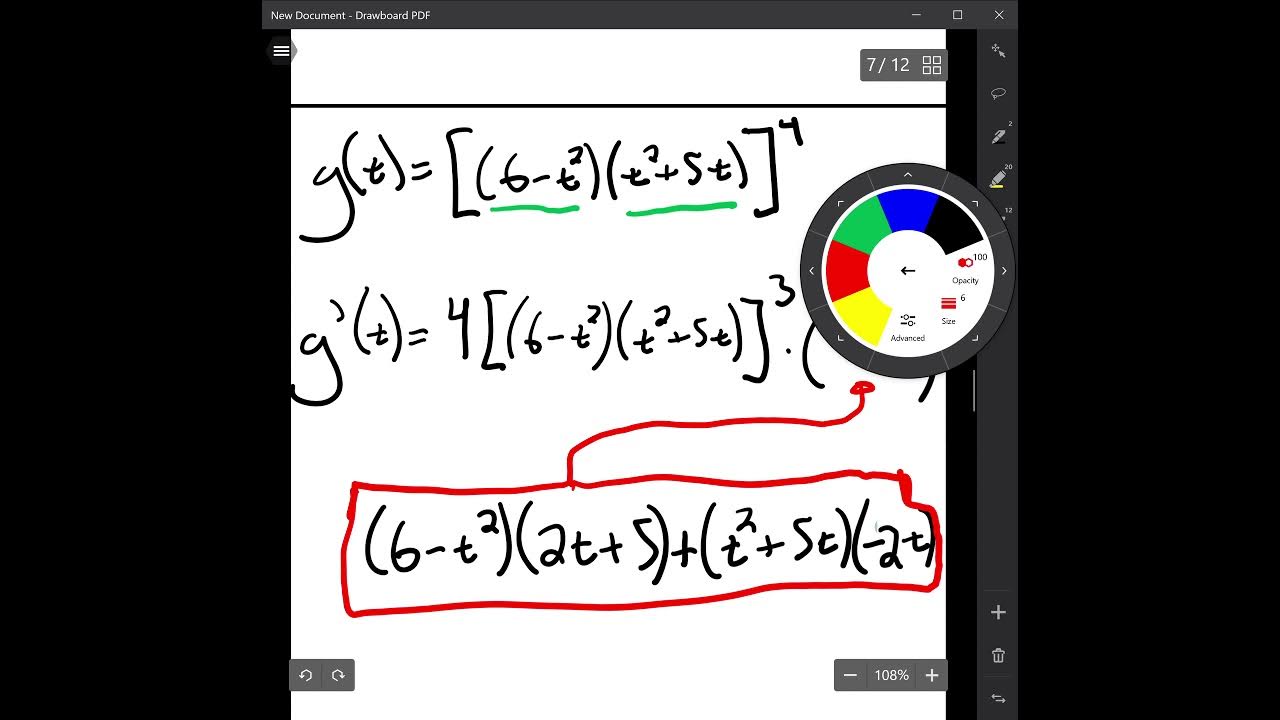
The final paragraph provides a comprehensive example involving a function that includes both division and cubing of variables. The讲师 applies the Chain Rule to the function, breaking down the process step by step. The explanation covers the derivative of the cubic part, the division, and the combination of these components to arrive at the final derivative. This example serves to solidify the understanding of the Chain Rule and its application in various scenarios.
Mindmap
Keywords
💡Chain Rule
💡Derivatives
💡Trigonometric Derivatives
💡Composite Functions
💡Polynomials
💡Sine Function
💡Cosine Function
💡Tangent Function
💡Exponents
💡Secant Function
Highlights
The Chain Rule is introduced as a fundamental concept in calculus, particularly for taking derivatives.
The importance of not underestimating the Chain Rule is emphasized, as it is crucial for more advanced calculus topics.
The Chain Rule is described as a method for dealing with nested functions, where a function of X is nested inside another function of X.
An example is provided to illustrate the mistake of incorrectly applying the derivative of sine as cosine to the function sine of x squared.
The correct derivative of sine of x squared is demonstrated as 2x times the cosine of x squared, highlighting the process of the Chain Rule.
The Chain Rule is formalized with a composite function, where the derivative of the composite function is the derivative of the outer function times the derivative of the inner function.
Nested functions such as sine of 2x and tangent of x to the fourth power are mentioned as scenarios where the Chain Rule is necessary.
The process of the Chain Rule is likened to linking together components, emphasizing its iterative nature in dealing with nested functions.
A new example is introduced, involving the square root of x squared minus 7X, to demonstrate the application of the Chain Rule in more complex scenarios.
The Chain Rule is applied to the example of tangent of 3x, showing how it has been used in previous simpler problems without explicit mention.
The concept of the Chain Rule is further reinforced by walking through the problem of 1 plus cosine squared of X raised to the sixth power.
A detailed breakdown of the Chain Rule application is provided for the problem of sine cubed of x plus cosine cubed of x, emphasizing the importance of understanding nested functions.
The Chain Rule is applied to a more complex function involving a cubed term squared, tangent of x to the third, demonstrating its versatility.
A final example involving a cubic term and a division of two functions, x minus 6 over x plus seven cubed, is used to drive home the application of the Chain Rule.
The problem of x minus 6 over x plus seven cubed is solved using the Chain Rule, showcasing its importance in tackling a variety of calculus problems.
The Chain Rule is highlighted as a tool that must be mastered for solving a wide range of calculus problems, particularly those involving nested or composite functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: