Solid of Revolution (part 8)

Khan Academy

27 Apr 200804:15

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses the process of solving a complex calculus problem involving the evaluation of a definite integral, specifically an antiderivative. The speaker methodically works through the problem, simplifying expressions and dealing with fractions, ultimately arriving at the solution of 65 pi over 6. The video highlights the challenge of solving volume of revolution problems and encourages viewers to seek further assistance if needed.

Takeaways

📚 The script discusses the process of evaluating a complex definite integral, specifically an antiderivative.
🧠 The calculation requires patience and methodical working through the problem, as it involves multiple steps and simplifications.
🔢 The initial step involves evaluating the antiderivative at a specific point (x=2) and subtracting the evaluation at another point (x=1).
👀 The script highlights the importance of careful arithmetic, as mistakes can lead to incorrect results in the calculation process.
📉 The problem presented is a real challenge, even for someone experienced with such calculations, due to the complexity of the fractions involved.
🌀 The script describes the process of simplifying terms by finding common denominators, which is crucial for handling fractions in mathematical problems.
📌 The final result of the calculation is given as 65 pi over 6, demonstrating that even complex problems can be simplified to a more manageable form.
🔄 The context of the problem involves finding the volume of a shape created by a line rotating around a given line, which is a standard calculus problem.
🛠️ The script serves as a review and demonstration of handling difficult fractions and integrals, which are fundamental concepts in calculus.
🎥 The speaker acknowledges the difficulty of the problem and the potential for confusion, encouraging viewers to seek further clarification if needed.
🚀 The script concludes by suggesting that this is a challenging but not insurmountable problem, and offers further assistance for those interested.

Q & A

What was the main task discussed in the transcript?
-The main task discussed in the transcript was evaluating a complex definite integral, specifically an antiderivative.
What was the initial step in solving the problem?
-The initial step in solving the problem was to evaluate the antiderivative at the given point, which was 2 in this case.
What was the first term that needed to be calculated after the initial evaluation?
-The first term that needed to be calculated after the initial evaluation was subtracting the antiderivative evaluated at 1.
How did the speaker handle the arithmetic errors during the problem-solving process?
-The speaker acknowledged the arithmetic errors and corrected them as they went along, demonstrating a methodical approach to problem-solving.
What was the significance of the fraction 40 pi in the simplification process?
-The fraction 40 pi represented the simplified result of the initial terms 16 pi minus 8 pi after combining like terms.
What was the role of common denominators in solving this problem?
-Common denominators played a crucial role in combining the fractions and simplifying the expression, especially when subtracting the terms involving pi.
What was the final simplified result of the problem?
-The final simplified result of the problem was 65 pi over 6.
How did the speaker describe the shape of the object whose volume was being calculated?
-The speaker described the shape as a kind of wide ring with hard edges on the upper and inner parts, and curved on the outside.
What was the unusual aspect of this volume of revolution problem?
-The unusual aspect of this problem was that the shape was rotated around the line y equals negative 2, which is not a typical axis of rotation.
What was the speaker's overall impression of the problem?
-The speaker found the problem to be challenging and 'hairy', indicating that it was complex and potentially confusing, but also noted that the final answer was not as complicated as it initially seemed.

Outlines

00:00

📚 Calculating the Volume of a Revolution - A Challenging Derivative Problem

This paragraph delves into the complex process of evaluating a definite integral, specifically an antiderivative, to calculate the volume of a shape created by a line rotation. The speaker meticulously works through the problem, starting with the evaluation at x=2 and subtracting the value at x=1. The process involves handling various terms involving pi, such as -16pi, -4pi/3, and +4pi, and simplifying the expressions. The challenge lies in dealing with fractions and finding common denominators to combine terms. The speaker humorously acknowledges the difficulty of the problem, referring to the 'hairy' nature of the math involved. Despite the complexity, the problem is resolved into a simpler form, 65pi/6, which represents the volume of the shape obtained from rotating the given line around the line y=-2. The paragraph concludes with a brief review of the problem and an offer for further assistance.

Mindmap

Keywords

💡Definite Integral

A definite integral is a fundamental concept in calculus that represents the signed area under a curve within a specified interval. In the context of the video, the definite integral is used to calculate the volume of a shape obtained by revolving a curve around the y-axis, which is a classic application of integral calculus. The process involves setting up the integral, evaluating it at the given limits, and subtracting the results to find the net area, which then translates to the volume of the solid of revolution.

💡Antiderivative

An antiderivative, also known as an indefinite integral, is a function whose derivative is the given function we are trying to integrate. In the video, finding the antiderivative is a crucial step in evaluating the definite integral. It represents the function that, when differentiated, will yield the original function, allowing the calculation of the area under the curve and, by extension, the volume of the solid formed by rotation.

💡Volume of Revolution

The volume of revolution is the amount of space occupied by a solid that is created by rotating a plane figure around an axis. In the video, the problem at hand involves calculating the volume of a shape that is obtained by rotating a certain curve around the line y = -2. This is done by using the method of disks or washers, depending on whether the solid is formed by including or excluding the material between the curve and the axis of rotation.

💡Simplifying Fractions

Simplifying fractions involves reducing them to their simplest form by dividing both the numerator and the denominator by their greatest common divisor (GCD). In the video, the process of simplifying fractions is essential for cleaning up the expressions obtained from the integral calculations, making the final answer more understandable and manageable.

💡Common Denominator

A common denominator is a denominator that is shared by two or more fractions. By finding a common denominator, fractions can be combined or compared more easily because they are expressed with the same denominator. In the context of the video, the speaker seeks a common denominator to simplify the mathematical expressions and to combine terms that have been separated by different denominators.

💡Rotation

In mathematics, rotation refers to the transformation of a shape by turning it around a fixed point or axis. In the video, rotation is the process by which the curve is revolved around the y-axis to form the shape whose volume is being calculated. This is a key concept in the method of disks, which is used to find the volume of solids of revolution.

💡Curve

A curve is a continuous, smooth shape that can be represented by a mathematical function. In the context of the video, the curve is the function that is being revolved around the y-axis to create the shape whose volume is being calculated. The properties of the curve, such as its derivative and antiderivative, are essential for setting up and solving the integral that gives the volume of the solid of revolution.

💡Limits of Integration

The limits of integration are the values between which the definite integral is evaluated. They define the interval over which the area under the curve is calculated. In the video, the limits of integration are the values at which the antiderivative is evaluated to find the volume of the solid formed by the rotation of the curve.

💡Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus is a central result that connects the process of differentiation and integration. It states that if a function is continuous on a closed interval and has an antiderivative on that interval, then the definite integral of the function over that interval can be calculated by evaluating the antiderivative at the endpoints and taking the difference of these values.

💡Pi (π)

Pi, commonly denoted by the Greek letter π, is a mathematical constant that represents the ratio of a circle's circumference to its diameter. In the video, π appears in the calculations as the volume of the shape involves circular components, and the constant π is used to account for the curvature and the area of the circles in the solid of revolution.

💡Fractions

Fractions are mathematical expressions that represent the division of two integers, where the numerator is the quantity being divided and the denominator is the number by which it is divided. In the video, fractions are used extensively in the calculation of the volume of revolution, and the process of simplifying them is a key part of reaching the final answer.

Highlights

The process of evaluating a complex antiderivative involving definite integrals.

The challenge of coming up with the next term in a hairy derivative calculation.

The evaluation of the antiderivative at specific points, such as x=2 and x=1.

The subtraction of evaluated results to find the final value of the antiderivative.

The simplification of complex mathematical expressions involving pi and fractions.

The translation of a mathematical problem into a real-world application, such as the volume of a strange-shaped ring.

The method of finding the volume of an object by rotating it around a given line.

The importance of dealing with fractions and finding common denominators for complex calculations.

The process of simplifying fractions to make the final answer more understandable.

The final answer of the problem, which is 65 pi over 6.

The acknowledgment of the difficulty in solving such problems and the potential for making mistakes.

The description of the unique shape of the object, with hard edges on the upper and inner parts and a curved outside.

The review of the problem-solving process and the offer to provide more complex problems if desired.

The conclusion of the video with a promise to continue the topic in the next video.

Transcripts

Browse More Related Video

INTEGRAL CALCULUS BETA GAMMA FUNCTION LECTURE 12

Washer method rotating around vertical line (not y-axis), part 2 | AP Calculus AB | Khan Academy

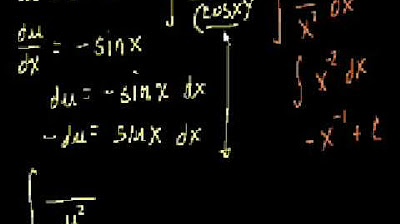
Another u-subsitution example

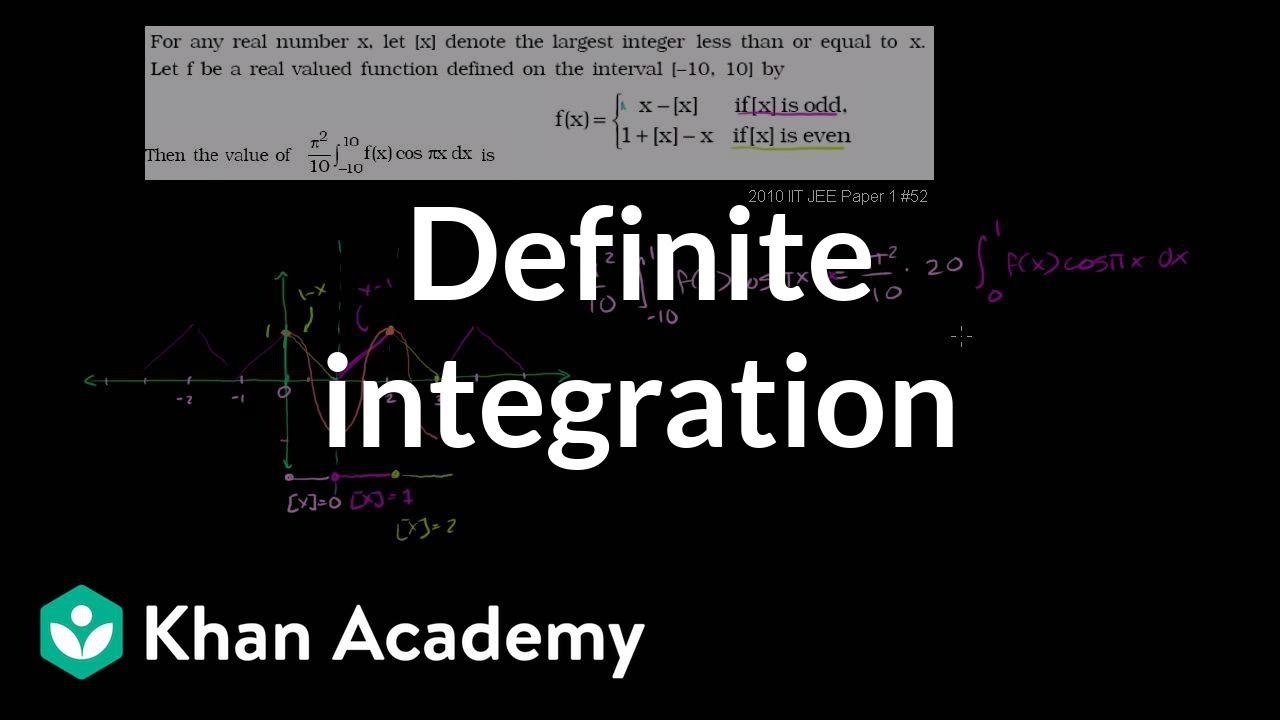
Periodic Definite Integral

Calculus AB Homework 7.5 Shell Method

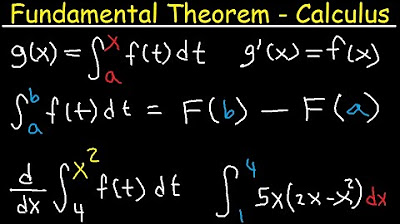
Fundamental Thereom of Calculus Explained - Part 1 & 2 Examples - Definite Integral

Solid of Revolution (part 8)

Takeaways

Q & A

What was the main task discussed in the transcript?

What was the initial step in solving the problem?

What was the first term that needed to be calculated after the initial evaluation?

How did the speaker handle the arithmetic errors during the problem-solving process?

What was the significance of the fraction 40 pi in the simplification process?

What was the role of common denominators in solving this problem?

What was the final simplified result of the problem?

How did the speaker describe the shape of the object whose volume was being calculated?

What was the unusual aspect of this volume of revolution problem?

What was the speaker's overall impression of the problem?