Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (48 of 92) How Oscillator Increases energy

Michel van Biezen
7 Apr 201804:54
EducationalLearning
32 Likes 10 Comments

TLDRThe script explains the difference between classical and quantum mechanical oscillators, emphasizing that while classical oscillators can have continuous amplitude values, quantum oscillators require energy quantization. It details how the energy of a quantum oscillator increases in fixed steps, with amplitude changes occurring in quantum leaps. The frequency remains constant, but the amplitude increases proportionally to the square root of the energy levels, resulting in distinct oscillatory states at different energy levels.

Takeaways
  • πŸ“ˆ The energy of a quantum mechanical oscillator increases in quantized steps, unlike classical oscillators where energy can increase continuously.
  • πŸ” The frequency of a quantum oscillator is constant and depends only on the spring constant (K) and the mass (m) of the object.
  • 🌐 In a classical mechanical oscillator, the amplitude of oscillation can vary infinitely, whereas in a quantum oscillator, amplitude is quantized.
  • πŸ”„ The amplitude of a quantum oscillator is proportional to the square root of its energy, leading to discrete energy levels.
  • πŸ“Š At the lowest energy level (N=0), the probability density function of a quantum oscillator shows a different distribution compared to a classical oscillator.
  • πŸš€ The energy of a quantum oscillator at state N is given by the formula E = (N+1/2)Δ§Ο‰, where Ο‰ is the angular frequency and Δ§ is the reduced Planck constant.
  • 🌟 The amplitude of oscillation for a quantum oscillator increases as you move to higher energy states, with each state being a multiple of the square root of the energy.
  • πŸ“ˆ When transitioning from the N=0 state to N=1 state, the energy increases by a factor of 3, and the amplitude increases by the square root of 3.
  • πŸ”’ At the N=10 state, the energy is 21 times that of the N=0 state, and the amplitude is approximately 4.58 times greater.
  • 🌌 The probability density function for higher quantum states shows a different distribution, with a higher likelihood of finding the particle at the center of oscillation.
  • πŸŒ€ The key difference between classical and quantum oscillators is that quantum oscillators have discrete energy levels and amplitudes, while classical oscillators have continuous energy variations.
Q & A

  • How does a quantum mechanical oscillator increase its energy?

    -A quantum mechanical oscillator increases its energy by increasing its amplitude in quantum steps. Since the frequency is constant and depends only on the spring constant (k) and the mass (m), the only way to increase the energy is by quantizing the amplitude.

  • What determines the frequency of a quantum mechanical oscillator?

    -The frequency of a quantum mechanical oscillator is determined by the spring constant (k) and the mass (m) of the object. It is proportional to the square root of k divided by m.

  • How is the energy of a quantum mechanical oscillator related to its amplitude?

    -The energy of a quantum mechanical oscillator is proportional to the square of its amplitude. The amplitude, in turn, is proportional to the square root of the energy.

  • What is the probability density function of a quantum mechanical oscillator at the N equals zero state?

    -At the N equals zero state, the probability density function of a quantum mechanical oscillator shows that the object is more likely to be found in the middle of the oscillation and less likely towards the edges.

  • How does the amplitude of a quantum mechanical oscillator change when transitioning from the N equals zero state to the N equals one state?

    -When transitioning from the N equals zero state to the N equals one state, the amplitude of the quantum mechanical oscillator increases by the square root of the energy increase. For the N equals one state, the energy is three times that of the N equals zero state, so the amplitude increases to the square root of 3 times the original amplitude.

  • What is the relationship between the energy levels and amplitude in a quantum mechanical oscillator?

    -The amplitude of a quantum mechanical oscillator increases with each energy level. The amplitude jumps to the next quantum state by the square root of the energy difference between the levels relative to the N equals zero level.

  • What is the energy difference between the N equals zero state and the N equals two state in a quantum mechanical oscillator?

    -The energy at the N equals two state is five times the energy at the N equals zero state.

  • How does the oscillatory state of a quantum mechanical oscillator at the N equals ten state look like?

    -At the N equals ten state, the oscillatory state of a quantum mechanical oscillator has an amplitude that is the square root of 21 times the amplitude of the N equals zero state, which is approximately 4.58 times the original amplitude.

  • What is the key difference between a classical mechanical oscillator and a quantum mechanical oscillator in terms of amplitude?

    -In a classical mechanical oscillator, the amplitude can increase gradually and have an infinite number of values. In contrast, a quantum mechanical oscillator has quantized amplitudes that increase in quantum steps.

  • Why does the probability of finding a particle differ at the edges and the middle of the oscillation for a classical oscillator?

    -For a classical oscillator, the probability of finding the particle is higher at the edges because that's where the speed is slower, and lower in the middle where the speed is faster.

  • How does the concept of quantization affect the energy levels of a quantum mechanical oscillator?

    -Quantization affects the energy levels of a quantum mechanical oscillator by requiring that the energy levels are discrete and not continuous. Each energy level corresponds to a specific amplitude, and the oscillator can only transition between these levels in quantum jumps.

Outlines
00:00
πŸ“ˆ Quantum Oscillator Energy Increase

This paragraph discusses the energy increase in a quantum mechanical oscillator. It explains that unlike classical mechanical oscillators, where energy can increase continuously, quantum oscillators require energy to increase in discrete steps or 'quantum jumps'. The frequency of the quantum oscillator remains constant and depends only on the spring constant (K) and the mass of the object. The energy of the oscillator is proportional to the square of its amplitude, and in the quantum world, the amplitude also quantizes, leading to quantized energy levels. The paragraph further illustrates this with an example of an oscillator transitioning from the ground state (N=0) to higher energy states (N=1, N=2, ... N=10), showing how the amplitude and energy levels change according to the square root of the energy difference from the ground state.

Mindmap
Keywords
πŸ’‘Quantum Mechanic Oscillator
A quantum mechanic oscillator is a system that exhibits periodic motion at a frequency determined by its physical properties, such as the mass of the object and the spring constant. Unlike a classical mechanical oscillator, its energy levels are quantized, meaning it can only exist in discrete energy states. In the video, this concept is used to explain how energy increases in quantum jumps, with the amplitude of oscillation changing in quantized steps.
πŸ’‘Amplitude
Amplitude in the context of an oscillator refers to the maximum displacement from the equilibrium position during its oscillation. In quantum mechanics, amplitude is quantized, and it is related to the energy of the system. The amplitude increases in quantum steps as the energy of the quantum oscillator increases, which is a departure from classical mechanics where amplitude can change continuously.
πŸ’‘Energy Quantization
Energy quantization is a fundamental principle in quantum mechanics that states that the energy of a system can only exist in discrete amounts, or quanta. This is in contrast to classical systems where energy can vary continuously. In the video, it is explained that the energy of a quantum oscillator increases in quantized steps, with each higher energy level corresponding to a larger amplitude of oscillation.
πŸ’‘Frequency
Frequency refers to the number of oscillations or cycles a system completes in a unit of time. In the context of the video, the frequency of a quantum mechanical oscillator is constant and determined by the properties of the system (the spring constant and the mass). It does not change with the energy level, unlike the amplitude, which does change as the energy increases.
πŸ’‘Probability Density Function
The probability density function (PDF) describes the likelihood of finding a particle in a particular state or at a particular position within a quantum system. In the video, the PDF for a quantum oscillator is contrasted with the classical oscillator, showing that particles are more likely to be found at the center of oscillation in quantum states, unlike in classical systems where they are more likely to be at the edges.
πŸ’‘Classical Mechanical Oscillator
A classical mechanical oscillator is a system that can be described using classical physics, where the energy and amplitude of oscillation can vary continuously. In the video, this is contrasted with a quantum mechanical oscillator, which has discrete energy levels and quantized amplitude changes. The classical oscillator is used to introduce the concept of oscillatory motion before delving into the quantum mechanical aspects.
πŸ’‘Square Root of Energy
The square root of energy is used in quantum mechanics to relate the energy levels of a system to its amplitude of oscillation. In the context of the video, the amplitude of a quantum oscillator is proportional to the square root of its energy. This means that as the energy of the quantum oscillator increases in quantized steps, the amplitude also increases, but only by the square root of the energy change.
πŸ’‘Quantum Jumps
Quantum jumps refer to the sudden transitions between discrete energy levels in a quantum system. In the video, this concept is used to explain how a quantum oscillator increases its energy by making jumps to higher energy states, each with a corresponding larger amplitude of oscillation. These jumps are not gradual but occur in quantized steps.
πŸ’‘Angular Frequency (Omega)
Angular frequency, denoted by Omega, is a measure of how quickly an oscillatory system vibrates, and it is constant for a given quantum mechanical oscillator. In the video, it is emphasized that Omega cannot change because it is determined by the fixed properties of the system (mass and spring constant), and thus the only way to increase the energy is by changing the amplitude of oscillation in quantized steps.
πŸ’‘N Equals Zero State
The 'N equals zero state' refers to the lowest energy state or the ground state of a quantum mechanical oscillator. In the video, this state is used as a reference point to discuss how the amplitude of oscillation and the energy of the system increase as the system transitions to higher energy states (N equals one, two, etc.).
πŸ’‘Energy Level
An energy level is a specific value of energy that a quantum system can have. In the context of the video, the energy levels of a quantum oscillator are quantized and correspond to different amplitudes of oscillation. The video explains how the energy of the oscillator increases as it transitions from one energy level to another, with each level being a multiple of the energy at the ground state.
Highlights

A quantum mechanical oscillator increases its energy by increasing its amplitude in quantum steps.

The frequency of a quantum mechanical oscillator is constant and depends only on the spring constant (k) and the mass (m) of the object.

In a classical mechanical oscillator, the amplitude of oscillation can increase gradually, whereas in a quantum mechanical oscillator, the amplitude is quantized.

The total energy of a classical oscillator is proportional to the square of the amplitude (E ∝ A^2).

In a quantum mechanical oscillator, the amplitude is proportional to the square root of the energy (A ∝ √E).

The angular frequency (Ο‰) of a quantum mechanical oscillator is fixed and determined by the square root of k over m (Ο‰ ∝ √k/m).

The probability density function for a quantum mechanical oscillator at the lowest energy level (N=0) shows a different distribution compared to a classical oscillator.

At the N=0 state, particles are more likely to be found at the edges of the oscillation due to slower speeds, unlike in the classical case.

When moving to the N=1 state, the energy is three times that of the N=0 state, and the amplitude increases to the square root of three times the original amplitude.

For the N=2 state, the energy is five times the original, and the amplitude increases to the square root of five times the original amplitude.

The amplitude of a quantum oscillator jumps to the next quantum state, which is determined by the square root of the energy level relative to the N=0 energy level.

The N=10 state of a quantum oscillator has an energy level 21 times that of the N=0 state, with a corresponding amplitude increase.

The frequency of oscillation remains unchanged in a quantum mechanical oscillator; only the amplitude changes as it jumps between energy levels.

Quantum jumps in amplitude are a fundamental aspect of how a quantum oscillator increases its energy in the quantum world.

The theoretical framework for quantum mechanical oscillators provides insights into the quantization of energy and amplitude in quantum systems.

The comparison between classical and quantum mechanical oscillators highlights the discrete nature of energy levels in quantum mechanics.

Understanding quantum mechanical oscillators is crucial for applications in quantum physics and technology, such as quantum computing and cryptography.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (51 of 92) Oscillator Amplitude - Diatomic

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (46 of 92) Quantum Nature of Oscillator 2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (44 of 92) What is the Selective Rule?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (50 of 92) What is Oscillator Amplitude?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (38 of 92) Simple Harmonic Oscillator

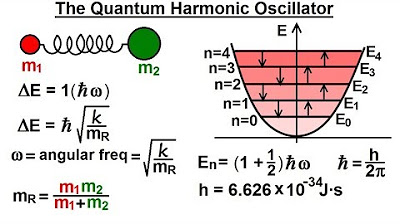
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsOscillatorEnergyAmplitudeQuantizationFrequencyConstancyEnergyLevelsSpringConstantMassOscillationProbabilityDensityQuantumJumpsPhysicsEducation