Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (46 of 92) Quantum Nature of Oscillator 2

Michel van Biezen
5 Apr 201804:23
EducationalLearning
32 Likes 10 Comments

TLDRThe lecture explores the quantum nature of oscillators, highlighting that like small particles, they exhibit wave-like properties when their energy levels are discrete. It explains how the energy difference between quantum states can be calculated and relates this to the emission or absorption of photons. The example of a diatomic molecule demonstrates how quantum steps become less noticeable as the mass of the oscillator increases, transitioning from quantum to classical behavior, with the emitted radiation moving from the infrared to the radio spectrum.

Takeaways
  • 🌊 Quantum oscillators exhibit wave-like properties when they have small mass and high oscillatory frequencies.
  • πŸ“ˆ The energy levels of quantum oscillators are discrete, with stepped-like functions rather than continuous ones.
  • πŸ“Š The energy difference between quantum states can be observed and is significant for small oscillators.
  • πŸ’‘ The quantum nature of an oscillator can be studied using the example of a diatomic molecule with a specific spring constant and mass.
  • πŸŒ€ The frequency of a typical quantum oscillator is extremely high, leading to rapid oscillations.
  • 🚦 The photon energy associated with an oscillator is equal to the energy difference between adjacent quantum states (h-bar Omega).
  • πŸ”½ As the mass of an oscillator increases, its angular frequency (Omega) decreases, leading to smaller energy differences between states.
  • πŸ“Ά The wavelength of a photon emitted or absorbed by an oscillator can be calculated and is related to the oscillator's angular frequency.
  • 🌐 When the mass of an oscillator is significantly increased, the quantum steps become indistinguishable, and the system behaves as a normal oscillator, not a quantum one.
  • πŸ” To maintain quantum mechanical effects, oscillators need to consist of very small atoms moving at high frequencies with a strong spring constant.
  • 🌈 The quantum mechanical nature of an oscillator is evident when the emitted or absorbed photons fall within the infrared range, as opposed to radio waves for larger oscillators.
Q & A

  • What is the quantum nature of oscillators?

    -The quantum nature of oscillators refers to their wave-like properties when they have small enough mass and move at sufficiently low speeds. As particles or oscillators become larger or more massive, they no longer exhibit wave-like behavior.

  • How do quantum oscillators differ from classical oscillators in terms of energy levels?

    -Quantum oscillators have discrete or stepped energy levels, unlike classical oscillators, which have continuous energy levels. The energy differences between quantum states are noticeable and quantized.

  • What is the relationship between the mass of an oscillator and its quantum behavior?

    -The smaller the mass of the oscillator, the more pronounced its quantum behavior. As the mass increases, the oscillator's quantum steps become less noticeable, and it behaves more like a classical oscillator with continuous energy levels.

  • How can the frequency of a diatomic molecule be calculated?

    -The frequency of a diatomic molecule can be calculated using the spring constant and the masses of the atoms involved. The angular frequency (Omega) is determined by the formula Omega = sqrt(k/m), where k is the spring constant and m is the reduced mass of the oscillator.

  • What is the significance of the energy difference between adjacent quantum states?

    -The energy difference between adjacent quantum states is significant because it determines the energy of a photon that can be absorbed or emitted by the oscillator. This energy difference is given by the product of Planck's constant (h-bar) and the angular frequency (Omega).

  • How can the wavelength of a photon associated with a quantum oscillator be calculated?

    -The wavelength of a photon associated with a quantum oscillator can be calculated using the energy difference between two energy states and the speed of light. The formula is lambda = 2Ο€c / (h-bar * Omega), where lambda is the wavelength, c is the speed of light, and h-bar and Omega are as previously defined.

  • What is the wavelength range of a photon emitted by a typical diatomic molecule oscillator?

    -The wavelength range of a photon emitted by a typical diatomic molecule oscillator, with a spring constant of 2000 N/m and atomic mass units of about 30, is in the infrared range, specifically around 9000 nanometers or nine micrometers.

  • How does the mass of an oscillator affect the wavelength of the photon it emits?

    -As the mass of the oscillator increases, the angular frequency (Omega) decreases, leading to smaller energy differences between states. This results in a longer wavelength for the photon emitted, shifting from the infrared range to longer radio waves as the mass becomes very large.

  • What conditions are necessary for an oscillator to exhibit quantum mechanical properties?

    -For an oscillator to exhibit quantum mechanical properties, it needs to have a very small mass moving at high speeds or frequencies, and a strong spring constant. Atoms within an object or diatomic molecules typically meet these conditions.

  • What happens when an oscillator becomes so large that it no longer exhibits quantum effects?

    -When an oscillator becomes so large that it no longer exhibits quantum effects, the differences between adjacent energy levels become too small to recognize, and the energy states become a continuous function. At this point, the oscillator behaves like a normal classical oscillator, emitting a continuous electromagnetic wave rather than photons.

  • How can the quantum steps between quantum states be observed?

    -The quantum steps between quantum states can be observed as discrete energy changes when an oscillator absorbs or emits a photon. These steps are noticeable as the energy levels are quantized and not continuous, allowing for the identification of individual energy state transitions.

Outlines
00:00
πŸ“ Quantum Nature of Oscillators

This paragraph introduces the concept of quantum oscillators, drawing an analogy with small particles that exhibit wave-like properties when they move at certain speeds. It explains that quantum oscillators, like diatomic molecules, have quantized energy levels, which result in stepped energy differences between states. The paragraph uses the example of a diatomic molecule with a specific spring constant and mass to illustrate how the oscillatory frequency and the associated photon wavelength can be calculated. It highlights the transition from quantum to classical behavior as the mass of the oscillator increases, resulting in smaller energy differences and longer wavelengths that are no longer considered photons.

Mindmap
Keywords
πŸ’‘Quantum Nature
The quantum nature refers to the intrinsic properties of particles at a microscopic level, where they exhibit both wave-like and particle-like characteristics. In the context of the video, it explains how small particles or oscillators with low mass and high frequency can have quantized energy levels, which are distinct and discrete, unlike classical oscillators. The video uses the example of a diatomic molecule to illustrate how its quantum nature allows for the emission or absorption of photons at specific wavelengths.
πŸ’‘Wave-Particle Duality
Wave-particle duality is a fundamental concept in quantum mechanics that states particles can exhibit both wave-like and particle-like properties. In the video, this concept is applied to oscillators, where small particles demonstrate wave-like behavior when they move at certain speeds and scales. This duality is what allows for the quantization of energy levels in quantum oscillators.
πŸ’‘Quantum Oscillators
Quantum oscillators are systems that follow the principles of quantum mechanics, where the energy levels are quantized, meaning they can only take on certain discrete values. In the video, the term is used to describe oscillators with small masses that exhibit quantum behavior, such as a diatomic molecule, where the energy differences between states are noticeable and stepped, rather than continuous.
πŸ’‘Energy Level Quantization
Energy level quantization is the phenomenon where the energy levels of a quantum system are discrete and not continuous. In the video, this concept is crucial for understanding the quantum nature of oscillators, as it explains how the energy differences between quantum states are stepped functions, leading to the emission or absorption of photons at specific wavelengths.
πŸ’‘Angular Frequency
Angular frequency, denoted by Omega (Ο‰), is a measure of how quickly an oscillator vibrates or a wave oscillates, taking into account both the frequency and the period of the oscillation. In the context of the video, angular frequency is used to calculate the energy differences between quantum states and subsequently the wavelength of the photon that could be absorbed or emitted by a quantum oscillator.
πŸ’‘Photon
A photon is a quantum of electromagnetic radiation, carrying energy and having both wave-like and particle-like properties. In the video, photons are either absorbed or emitted by quantum oscillators when they transition between energy levels. The energy of the photon is equal to the energy difference between two adjacent quantum states.
πŸ’‘Wavelength
Wavelength is the distance between two consecutive points in a wave that are in phase, such as crest to crest or trough to trough. In the context of the video, the wavelength of a photon emitted or absorbed by a quantum oscillator is calculated based on the energy difference between quantum states and the angular frequency of the oscillator. This calculation helps determine the type of electromagnetic radiation, such as infrared or radio waves.
πŸ’‘Spring Constant
The spring constant, often denoted by k, is a measure of the stiffness of a spring. It describes the amount of force needed to extend or compress the spring by a certain distance. In the video, the spring constant is used to determine the angular frequency of an oscillator, which is crucial for understanding the quantum mechanical properties of the system.
πŸ’‘Atomic Mass Units
Atomic mass units (amu) are a unit of mass used to express the mass of atoms and molecules. One amu is defined as one twelfth of the mass of a carbon-12 atom. In the video, the mass of each atom in a diatomic molecule is given in atomic mass units, which is used to calculate the properties of the quantum oscillator.
πŸ’‘Infrared Range
The infrared range is a portion of the electromagnetic spectrum that lies just beyond the visible light spectrum. It is characterized by wavelengths longer than those of visible light but shorter than those of microwaves, typically ranging from about 700 nanometers to 1 millimeter. In the video, the calculated wavelength of a photon emitted by a quantum oscillator falls within the infrared range, indicating its quantum mechanical nature.
πŸ’‘Radio Waves
Radio waves are a type of electromagnetic wave with wavelengths longer than infrared light, extending from about 1 millimeter to 100 kilometers. They are often used for long-distance communication. In the video, when the mass of the oscillator is hypothetically increased to mimic a larger object like a grain of sand, the wavelength of the radiation associated with the oscillator falls into the radio wave range, indicating a transition from quantum to classical behavior.
Highlights

Quantum oscillators exhibit wave-like properties similar to small particles.

As particles increase in size, they lose their wave-like behavior.

Quantum oscillators have noticeable energy level differences between quantum states.

The energy differences in quantum oscillators are stepped functions, not continuous.

A typical oscillator, like a diatomic molecule, has a spring constant equivalent of 2,000 Newtons per meter.

The mass of each atom in the diatomic molecule example is about 30 atomic mass units.

The angular frequency of the diatomic molecule oscillator is about 2 times 10 to the 14 per second.

The oscillatory frequency of the diatomic molecule is approximately 32 oscillations per second.

Quantum steps between quantum states are observable in the oscillator's behavior.

The photon energy absorbed or emitted by an oscillator equals the energy difference between two adjacent states.

The wavelength of a photon associated with the oscillator can be calculated using the energy difference and the speed of light.

For the diatomic molecule oscillator, the photon wavelength is about 9,000 nanometers, in the infrared range.

As the mass in the oscillator increases, the angular frequency and energy differences decrease.

A larger mass oscillator, like a grain of sand, would have a much lower frequency and emit very long radio waves.

At a certain mass, the quantum mechanical effect is no longer observable, and the oscillator behaves as a normal oscillator.

Quantum mechanical oscillators require very small atoms moving at high frequencies with a strong spring constant.

Atoms within an object or a diatomic molecule typically exhibit the quantum mechanical nature of an oscillator.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (48 of 92) How Oscillator Increases energy

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (42 of 92) k=? of a Diatomic Molecule

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (44 of 92) What is the Selective Rule?

Why don't quantum effects occur in large objects? double slit experiment with tennis balls

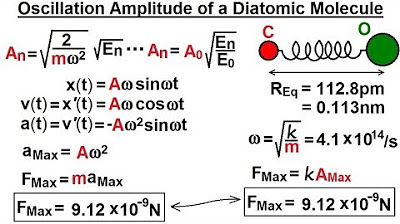
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (51 of 92) Oscillator Amplitude - Diatomic

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsOscillatorBehaviorWavePropertiesEnergyLevelsPhotonEmissionMassInfluenceInfraredWavelengthRadioWaveRangeMolecularPhysicsQuantumStates