Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?

Michel van Biezen
29 Mar 201806:13
EducationalLearning
32 Likes 10 Comments

TLDRThe lecture introduces the concept of the quantum harmonic oscillator, emphasizing its distinction from the classical harmonic oscillator through quantized energy levels. It explains that energy levels are specific and not continuous, with the smallest possible energy being non-zero. The energy levels are described by the formula a_n = (n + 1/2) * H_bar * Omega, where H_bar is Planck's constant divided by 2 pi, and Omega is the angular frequency determined by the reduced mass and spring constant. The lecture also touches on the physical representation of the quantum harmonic oscillator, such as diatomic molecules, and how their vibrational frequencies are unique due to their structure and intermolecular forces. The concept of quantum jumps between energy levels and the emission or absorption of energy is also discussed, providing a bridge between quantum theory and observable molecular vibrations.

Takeaways
  • 🎡 The quantum harmonic oscillator is a quantum version of the classical harmonic oscillator with quantized energy levels.
  • πŸš€ Energy in a quantum harmonic oscillator can only exist in specific, discrete values, unlike the continuous energy levels in the classical model.
  • 🌟 The smallest energy level, e_sub_0, is not equal to zero, which is a significant departure from classical physics.
  • πŸ“ˆ The energy levels are given by the formula e_sub_n = (n + 1/2) * H_bar * Omega, where n is an integer.
  • πŸ”„ Quantum jumps occur between energy levels, with each jump having an equal value defined by the energy difference formula.
  • πŸ”§ The angular frequency (Omega) of the oscillator is determined by the spring constant and the reduced mass of the system.
  • 🌐 The reduced mass is calculated as the product of the two masses divided by the sum of the two masses, relevant for systems like diatomic molecules.
  • πŸ”„ The oscillator's frequency is constant for a given molecule due to its specific structure and intermolecular forces.
  • πŸ’₯ The quantum harmonic oscillator can gain or lose energy through quantum jumps, radiating out energy when losing energy.
  • πŸ€” The lecture will further explore the significance of the non-zero lowest energy level and the implications of these quantum levels in the real world.
  • 🌟 The quantum harmonic oscillator provides a bridge between theoretical concepts and observable phenomena in the vibrational behavior of atoms and molecules.
Q & A

  • What is the main difference between a classical harmonic oscillator and a quantum harmonic oscillator?

    -The main difference is that in the quantum harmonic oscillator, the energy levels are quantized, meaning that only specific, discrete energy values are allowed, whereas in the classical case, energy can take on any value.

  • What is the smallest energy a quantum harmonic oscillator can have?

    -The smallest energy a quantum harmonic oscillator can have is denoted as e_sub_0, which is not equal to zero.

  • How are the energy levels of a quantum harmonic oscillator represented?

    -The energy levels are represented by the formula e_sub_n = (n + 1/2) * H_bar * Omega, where n is an integer starting from 0, H_bar is Planck's constant divided by 2 pi, and Omega is the angular frequency of the oscillator.

  • What is Planck's constant and its role in the energy levels of a quantum harmonic oscillator?

    -Planck's constant is a fundamental constant in quantum mechanics, represented by the symbol H. It is very small but extremely important in the quantum world. In the context of a quantum harmonic oscillator, it appears in the energy level formula as H_bar (H divided by 2 pi) and determines the quantization of energy levels.

  • What is the significance of the angular frequency (Omega) in the quantum harmonic oscillator?

    -The angular frequency, Omega, is determined by the spring constant and the reduced mass of the oscillator system. It defines the frequency at which the system oscillates and is crucial in calculating the energy levels of the quantum harmonic oscillator.

  • How is the reduced mass of a diatomic molecule calculated?

    -The reduced mass (M_sub_R) of a diatomic molecule is calculated as the product of the two masses (m1 and m2) divided by the sum of the two masses (m1 + m2).

  • What is the relationship between the spring constant and the angular frequency in a quantum harmonic oscillator?

    -The angular frequency, Omega, is defined as the spring constant divided by the reduced mass. This relationship is essential for determining the oscillation frequency and the energy levels of the quantum harmonic oscillator.

  • How do quantum leaps occur in a quantum harmonic oscillator?

    -Quantum leaps occur when the oscillator gains or loses energy in discrete amounts equal to H_bar * Omega. The energy level of the oscillator can increase or decrease by these quantized amounts.

  • Why is the lowest energy level of a quantum harmonic oscillator not equal to zero?

    -The lowest energy level, e_sub_0, is not equal to zero because of the zero-point energy, which is a fundamental concept in quantum mechanics indicating that there is a minimum amount of energy that a quantum system can have.

  • What is the significance of the quantized energy levels in the quantum harmonic oscillator?

    -The quantized energy levels are significant because they represent the discrete energy changes that can occur in quantum systems, which is a key difference from classical systems where energy can vary continuously.

  • How do the properties of a diatomic molecule, such as mass and intermolecular forces, influence its quantum harmonic oscillator model?

    -The mass of the atoms and the intermolecular forces between them determine the spring constant and the reduced mass, which in turn define the angular frequency and the quantized energy levels of the quantum harmonic oscillator model for that molecule.

Outlines
00:00
πŸ“ Introduction to Quantum Harmonic Oscillators

This paragraph introduces the concept of a quantum harmonic oscillator, emphasizing the quantization of energy levels in the quantum world. It explains that unlike classical harmonic oscillators, quantum harmonic oscillators can only have specific energy values, denoted as e(sub 1), e(sub 2), e(sub 3), etc. The smallest energy level, e(sub 0), is highlighted as being non-zero and is a topic for further discussion. The energy levels are mathematically defined using Planck's constant (H bar), the angular frequency (Omega), and an integer (n), with the formula a sub n = (n + 1/2) times H bar times Omega. The angular frequency is related to the oscillator's properties, such as the spring constant and the reduced mass of the system, which are influenced by intermolecular forces. The paragraph sets the stage for a deeper exploration of quantum harmonic oscillators and their significance in the quantum realm.

05:00
πŸ” Vibrational Frequencies and Quantum Energy Levels

The second paragraph delves into the specifics of how the vibrational frequency of a quantum harmonic oscillator is determined by the masses of the particles involved and the intermolecular forces between them. It explains that the oscillator's angular frequency is calculated based on these factors, and this frequency, when combined with Planck's constant and the formula involving n plus 1/2, gives the energy of each quantum level. The paragraph also discusses the quantum jumps in energy levels, where the oscillator gains or loses energy in discrete amounts. It sets up a discussion for later in the lecture about the lowest possible energy and the significance of the non-zero ground state energy level, as well as the practical implications and real-world connections to the theoretical concepts being presented.

Mindmap
Keywords
πŸ’‘Quantum Harmonic Oscillator
The quantum harmonic oscillator is a fundamental system in quantum mechanics that generalizes the concept of a classical harmonic oscillator to the quantum realm. Unlike its classical counterpart, the quantum harmonic oscillator has discrete energy levels, meaning it can only occupy specific energy states. This concept is central to the video's theme, as it introduces the idea of quantization of energy in the quantum world, with examples such as diatomic molecules like oxygen and nitrogen illustrating how their vibrational frequencies are determined by their structure and intermolecular forces.
πŸ’‘Quantized Energy Levels
Quantized energy levels refer to the property of certain quantum systems, such as the quantum harmonic oscillator, where the energy can only take on discrete values rather than a continuous range. This quantization is a fundamental aspect of quantum mechanics and contrasts with classical physics, where energy can vary continuously. In the context of the video, the energy levels are denoted by 'e_sup_n', and the script explains that these levels are specific and not just any arbitrary value.
πŸ’‘Planck's Constant
Planck's constant is a fundamental constant in quantum mechanics that relates the energy of a photon to its frequency. It is denoted by 'h' and is used in the formula for the energy of a quantum state in a harmonic oscillator. In the video, Planck's constant is divided by 2Ο€ (denoted as 'H_bar') to calculate the energy levels of the quantum harmonic oscillator, indicating its critical role in the quantization of energy.
πŸ’‘Angular Frequency
Angular frequency, denoted by 'Omega', is a measure of how quickly an object rotates or oscillates, and is defined as the rate of change of the angle per unit time. In the context of the quantum harmonic oscillator, 'Omega' is determined by the properties of the oscillator, such as the spring constant and the reduced mass of the system. It is crucial in calculating the energy levels of the oscillator, as it directly influences the quantized energy values.
πŸ’‘Reduced Mass
The reduced mass is a concept used in physics when considering the motion of two objects connected by a spring or other elastic force. It is the product of the two masses divided by the sum of the two masses, and it simplifies the analysis of their motion. In the video, the reduced mass is used to determine the angular frequency of a diatomic molecule, which in turn is used to calculate its quantized energy levels.
πŸ’‘Intermolecular Forces
Intermolecular forces are the forces that occur between molecules and influence their physical properties, such as boiling and melting points, solubility, and the formation of chemical bonds. In the video, these forces are what bind the two atoms in a diatomic molecule and create the spring-like behavior that allows for the quantization of energy levels in the quantum harmonic oscillator model.
πŸ’‘Quantum Jumps
Quantum jumps refer to the discrete changes in energy levels that a quantum system can undergo. In the context of the quantum harmonic oscillator, these jumps represent the transition from one energy level to another, with each jump being of equal value. The video explains that the energy difference between levels is defined by 'H_bar times Omega', illustrating the quantized nature of energy transitions in quantum systems.
πŸ’‘Diatomic Molecule
A diatomic molecule consists of two atoms bonded together. In the video, diatomic molecules like oxygen (O2) and nitrogen (N2) are used as examples to illustrate how their vibrational frequencies and energy levels are determined by their structure and the intermolecular forces between the atoms. These molecules have specific frequencies due to their structure, which is a direct result of the quantum harmonic oscillator model.
πŸ’‘Spring Constant
The spring constant, often denoted by 'k', is a measure of a spring's stiffness and quantifies the force required to extend or compress the spring by a given distance. In the quantum harmonic oscillator, the spring constant is related to the angular frequency 'Omega' and is determined by the intermolecular forces between the atoms in a diatomic molecule. The video script explains that the angular frequency is defined by the spring constant divided by the reduced mass, which is crucial for calculating the energy levels of the oscillator.
πŸ’‘Energy Quantization
Energy quantization is the concept that certain physical properties, such as the energy of an atomic system, can only take on discrete values rather than a continuous range. This is a key principle in quantum mechanics and is central to the video's discussion of the quantum harmonic oscillator. The script explains that the energy levels 'e_sup_n' are quantized, meaning they are specific and not equal to zero, and that the energy of the system can only change in discrete 'quantum jumps'.
πŸ’‘Zero-Point Energy
Zero-point energy is the lowest possible energy that a quantum mechanical system can have, and it is not equal to zero. In the context of the quantum harmonic oscillator, the script mentions that the smallest energy level 'e_sub_0' is not zero, which is a direct consequence of the uncertainty principle and the wave-like nature of particles in quantum mechanics. The video promises to discuss this concept further, highlighting its significance in understanding the behavior of quantum systems.
Highlights

The quantum harmonic oscillator is introduced as a quantum version of the classical harmonic oscillator with quantized energy levels.

Energy levels in the quantum harmonic oscillator are specific and not continuous, unlike in the classical harmonic oscillator.

The smallest energy a quantum harmonic oscillator can have is denoted as e_sub_0, and it is not equal to zero.

Quantum jumps occur between energy levels, with each jump having an equal value of energy.

The energy levels are defined by the formula a_sub_n = (n + 1/2) * H_bar * Omega, where H_bar is Planck's constant divided by 2 pi.

H_bar is a fundamental constant in quantum mechanics, representing Planck's constant divided by 2 pi.

The angular frequency Omega is defined by the oscillator's spring constant divided by the reduced mass.

A quantum oscillator can be represented by a diatomic molecule with two different atoms connected by intermolecular forces.

The spring constant in a quantum oscillator is determined by the intermolecular forces between the atoms.

The reduced mass of a diatomic molecule is calculated as the product of the two masses divided by the sum of the two masses.

The frequency of oscillation for a diatomic molecule is constant and specific due to its structure and the forces between the atoms.

The energy of a quantum harmonic oscillator is directly related to its frequency of oscillation and Planck's constant.

Quantum harmonic oscillators can gain and lose energy in discrete jumps, radiating out energy when they lose energy.

The quantum leaps between energy levels are characterized by a constant value, defined by H_bar times Omega.

The significance of the non-zero lowest energy level and the nature of these energy levels will be further discussed in the lecture.

The quantum harmonic oscillator serves as a bridge between theoretical concepts and real-world applications, such as understanding vibrational rates of atoms and molecules.

The lecture will explore the meaning behind the quantum harmonic oscillator, its lowest possible energy, and the implications of its energy levels.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (38 of 92) Simple Harmonic Oscillator

Advanced Quantum Mechanics Lecture 3

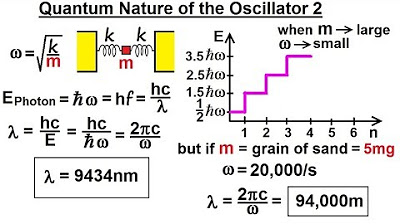
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (46 of 92) Quantum Nature of Oscillator 2

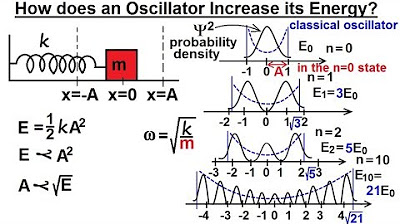
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (48 of 92) How Oscillator Increases energy

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (50 of 92) What is Oscillator Amplitude?

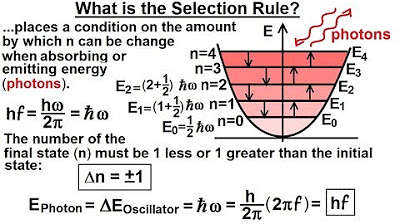
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (44 of 92) What is the Selective Rule?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumPhysicsHarmonicOscillatorEnergyLevelsQuantumJumpsPlancksConstantVibrationalFrequenciesAtomicStructureMolecularBondsReducedMassAngularFrequency