Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (44 of 92) What is the Selective Rule?

Michel van Biezen
3 Apr 201803:10
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the concept of selection rules in quantum mechanics, focusing on the behavior of quantum mechanical oscillators. It explains that these oscillators can gain or lose energy by emitting or absorbing photons, and that the energy difference between states is quantified by the Planck's constant times the angular frequency of oscillation. The selection rule dictates that the change in quantum number (Ξ”n) when absorbing or emitting energy can only be by one, meaning the oscillator can only transition to an adjacent energy state. This principle is key to understanding the quantization of energy levels and the energy exchange involving photons, as established by the photoelectric effect.

Takeaways
  • πŸ“Œ Quantum mechanical oscillators can gain or lose energy by emitting or absorbing photons.
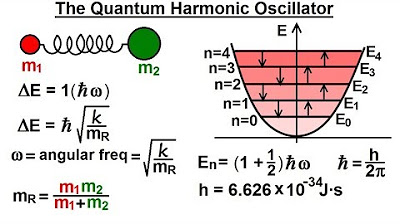
  • πŸ”§ The lowest energy state of an oscillator is not zero, but 1/2 Hbar times Omega, where Omega is the angular frequency of oscillation.
  • ⚑ The energy difference between consecutive quantum states is exactly one Hbar times Omega.
  • 🚫 The selection rule imposes a condition on how much the quantum number (n) can change when energy is absorbed or emitted, which must be by one unit.
  • 🌟 The final state's quantum number must be one less or one greater than the initial state after a photon is absorbed or emitted.
  • πŸ”„ The oscillator can only transition between adjacent energy levels, not jumping over multiple levels at once.
  • πŸ’° The energy of the photon involved in the transition is equal to the energy difference between the two adjacent states.
  • 🎒 The harmonic oscillator can only increase or decrease by one level at a time, reflecting the discrete nature of energy changes.
  • 🌐 The energy of the photon (E) is related to the frequency (F) by the equation E = h * F, derived from the photoelectric effect.
  • πŸ“ˆ The selection rule is fundamental for understanding quantum transitions and the energy exchange in quantum systems.
Q & A

  • What is the selection rule in quantum mechanics?

    -The selection rule in quantum mechanics is a condition that dictates the amount by which the quantum number (n) can change when a quantum mechanical oscillator absorbs or emits energy, typically in the form of photons.

  • How does the selection rule relate to the energy levels of a quantum mechanical oscillator?

    -The selection rule states that the energy difference between two adjacent energy levels of a quantum mechanical oscillator is equal to the energy of a single photon absorbed or emitted, which means the quantum number can only change by one (Ξ”n = Β±1).

  • What is the significance of the ground state energy level in a quantum mechanical oscillator?

    -The ground state energy level of a quantum mechanical oscillator is significant because it is not equal to zero; it is 1/2 Δ§Ο‰, where Ο‰ is the angular frequency of the oscillation. This indicates that there is a minimum energy state that the oscillator cannot fall below.

  • What is the relationship between the energy levels and the frequency of oscillation in a quantum mechanical oscillator?

    -The energy difference between consecutive energy levels in a quantum mechanical oscillator is directly related to the frequency of oscillation (Ο‰). The energy difference is given by Δ§Ο‰, where Δ§ is the reduced Planck's constant.

  • How does the selection rule limit the possible transitions for a quantum mechanical oscillator?

    -The selection rule limits the possible transitions of a quantum mechanical oscillator by stating that the oscillator can only transition to an energy level that is one less or one greater than its current state, meaning it can only absorb or emit photons with energy equal to the energy difference between adjacent levels.

  • What is the basic unit of energy for a photon?

    -The basic unit of energy for a photon is given by the equation E = hΞ½, where E is the energy, h is Planck's constant, and Ξ½ is the frequency of the photon. This relationship was established by the photoelectric effect.

  • How does the selection rule ensure that the energy of the emitted or absorbed photon matches the energy difference between two states?

    -The selection rule ensures this by stating that the quantum number change (Ξ”n) must be Β±1, which corresponds to the transition between adjacent energy levels. Since the energy difference between these levels is Δ§Ο‰, the energy of the emitted or absorbed photon must be Δ§Ο‰, matching the energy difference.

  • What is the consequence of the selection rule for the harmonic oscillator's energy transitions?

    -The consequence of the selection rule is that the harmonic oscillator can only transition one level at a time, either increasing or decreasing its energy by the amount equivalent to the energy difference between two adjacent states.

  • How does the selection rule relate to the photoelectric effect?

    -The selection rule is related to the photoelectric effect because it confirms that the energy of a photon is equal to the energy difference between two states (Δ§Ο‰), which is the basic unit of photon energy as discovered by the photoelectric effect.

  • What happens when a quantum mechanical oscillator absorbs or emits a photon?

    -When a quantum mechanical oscillator absorbs a photon, it gains energy and transitions to a higher energy state, while emitting a photon results in the loss of energy and a transition to a lower energy state, both following the selection rule with Ξ”n = Β±1.

  • Can a quantum mechanical oscillator jump two or more states at once according to the selection rule?

    -No, according to the selection rule, a quantum mechanical oscillator can only change by one state at a time, as the energy difference between non-adjacent states is not equal to the energy of a single photon.

Outlines
00:00
πŸ“š Introduction to Quantum Mechanics Oscillator and Selection Rule

This paragraph introduces the concept of the selection rule in quantum mechanics, specifically in relation to quantum mechanical oscillators. It explains that these oscillators can gain or lose energy by emitting or absorbing photons, and that the energy levels are quantized, with the lowest energy state not being zero but rather 1/2 H-bar times Omega. The selection rule is then defined, stating that the change in quantum number (n) when absorbing or emitting energy can only be by one unit, meaning the final state's quantum number must be one less or one greater than the initial state. This is directly related to the energy difference between adjacent quantum states, which is quantified as H-bar times Omega. The explanation concludes by discussing the implications of this rule, emphasizing that the oscillator can only change energy by one level at a time, and the energy of the photon involved in the process is equal to the energy difference between two adjacent states.

Mindmap
Keywords
πŸ’‘Quantum Mechanics
Quantum Mechanics is the fundamental theory in physics that describes the behavior of matter and energy at the smallest scales, such as atoms and subatomic particles. In the context of this video, it is the framework within which the quantum mechanical oscillator operates, and the selection rules are a key concept explaining how these oscillators interact with energy in the form of photons.
πŸ’‘Oscillator
An oscillator is a system that vibrates or oscillates with a certain frequency. In quantum mechanics, an oscillator can be a particle that moves back and forth in a potential well. The video focuses on the energy transitions of such an oscillator, particularly how it gains or loses energy through the emission or absorption of photons.
πŸ’‘Selection Rule
The selection rule is a principle in quantum mechanics that governs the possible electronic transitions between energy levels in an atom or a molecule. It specifies the conditions under which an electron can absorb or emit a photon, essentially determining which energy level changes are allowed. In the video, the selection rule is used to explain how a quantum mechanical oscillator can change its energy state by one quantum of energy.
πŸ’‘Energy Level
In quantum mechanics, energy levels are the specific, quantized amounts of energy that a system can have. These levels are discrete and not continuous. For a quantum mechanical oscillator, the energy levels are determined by the frequency of oscillation and the quantum number (n). The video discusses how these energy levels are not continuous and how they relate to the selection rule.
πŸ’‘Photon
A photon is a particle of light that carries energy and has both wave-like and particle-like properties. In the context of the video, photons are the means by which a quantum mechanical oscillator can change its energy state. The energy of the photon corresponds to the difference in energy between two adjacent energy levels of the oscillator.
πŸ’‘h-bar
h-bar, or reduced Planck's constant, is a fundamental constant in quantum mechanics that is equal to Planck's constant divided by 2Ο€ (h/2Ο€). It is used in the equations describing quantum systems to represent the quantization of energy levels. In the video, h-bar is used in the formula for the energy difference between adjacent levels of a quantum mechanical oscillator.
πŸ’‘Omega
Omega (Ο‰) represents the angular frequency of an oscillation in quantum mechanics. It is related to the frequency (f) of the oscillation by the equation Ο‰ = 2Ο€f. In the context of the video, Omega is a key parameter that determines the energy levels of the quantum mechanical oscillator and the energy of the photons associated with transitions between these levels.
πŸ’‘Quantum Number (n)
The quantum number (n) is an integer that labels the energy levels in a quantum system. In the context of the video, it is used to describe the energy states of the quantum mechanical oscillator. The selection rule states that when a quantum mechanical oscillator absorbs or emits a photon, the quantum number can only change by one, indicating a transition to an adjacent energy level.
πŸ’‘Energy Transition
An energy transition is the process by which a quantum system moves from one energy state to another. In quantum mechanics, these transitions are quantized, meaning they occur in discrete steps. The video focuses on the energy transitions of a quantum mechanical oscillator, which can only occur between adjacent energy levels due to the selection rule.
πŸ’‘Harmonic Oscillator
A harmonic oscillator is a type of oscillator that experiences a restoring force proportional to the displacement from its equilibrium position. In the context of quantum mechanics, the harmonic oscillator model is used to describe systems that can oscillate with a constant frequency. The video discusses the properties of a quantum mechanical oscillator, which behaves like a harmonic oscillator but with the added principles of quantum mechanics.
πŸ’‘Photoelectric Effect
The photoelectric effect is a phenomenon in which electrons are emitted from a material when it is exposed to light of a certain frequency. This effect was one of the key pieces of evidence that led to the development of quantum mechanics and the concept of photons. In the video, the photoelectric effect is mentioned as the basis for understanding how the energy of a photon is related to its frequency.
Highlights

Exploration of the selection rule, a key property in quantum mechanics related to oscillators.

Quantum mechanical oscillators gain or lose energy through the emission or absorption of photons.

The lowest energy level of an oscillator is 1/2 H-bar times Omega, which is not zero.

The energy difference between consecutive quantum states is exactly one H-bar times Omega.

The selection rule places a condition on how much the quantum number (n) can change during energy absorption or emission.

The change in the quantum number (Delta n) can only be one, meaning the oscillator can only absorb or emit energy in single photon increments.

The final state's quantum number must be one less or one greater than the initial state after photon absorption or emission.

The energy of the photon involved in the process is equal to the energy difference between two adjacent states of the oscillator.

The oscillator can only change by one level at a time, not by two or more simultaneously.

The energy absorbed or emitted is equal to the photon's energy difference between two adjacent levels.

The selection rule is fundamental for understanding the behavior of quantum oscillators and their interaction with photons.

The concept of H-bar (H over 2 pi) and Omega (angular frequency) are introduced to explain the energy quantization.

The energy of a photon is equal to h times the frequency, as discovered by the photoelectric effect.

The selection rule is a specific limit that dictates the quantized nature of energy changes in quantum oscillators.

The lecture provides a detailed explanation of the quantum mechanical oscillator's energy levels and the implications of the selection rule.

Transcripts

Browse More Related Video

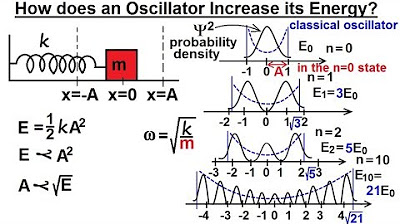
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (48 of 92) How Oscillator Increases energy

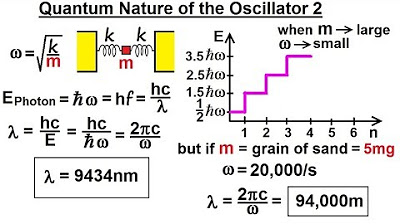
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (46 of 92) Quantum Nature of Oscillator 2

Advanced Quantum Mechanics Lecture 4

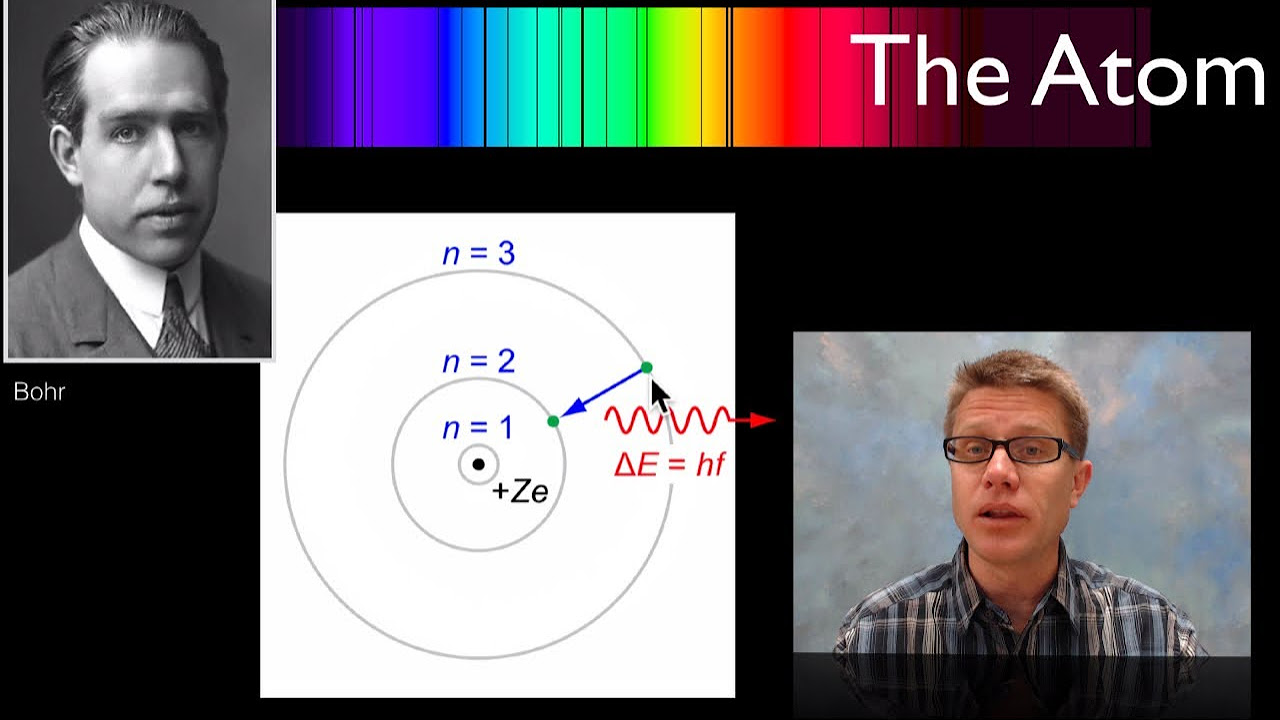
The Bohr Atom

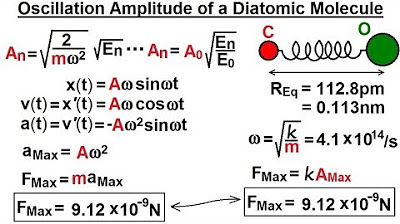
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (51 of 92) Oscillator Amplitude - Diatomic

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsSelectionRulesEnergyLevelsPhotonEmissionHarmonicOscillatorsQuantumStatesEnergyTransitionsPhysicsEducationOnlineLectureScientificExploration