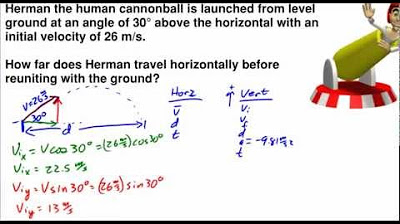
2021 AP Physics 1 Free Response #1 (First Administration)
TLDRIn this educational video, the host tackles the 2021 AP Physics 1 free response questions, focusing on a kinematics problem involving a stunt cyclist jumping over cars. The host derives an expression for the horizontal distance traveled based on the ramp's height, angle, and the combined mass of the cyclist and bicycle, using conservation of energy and kinematic equations. The video also explores the implications of doubling the ramp's height and sketches a graph of the vertical velocity component as a function of time.
Takeaways
- 📚 The speaker is analyzing the 2021 AP Physics 1 free response questions, focusing on the first administration's questions.
- 🔍 The speaker plans to read the questions carefully and attempt to solve them correctly on the first try, with corrections to be provided in the description if needed.
- 🤹 The scenario involves a stunt cyclist using a ramp to jump over cars, starting from rest at the top of the ramp.
- 📐 The task is to derive an expression for the horizontal distance x_0 in terms of h_0, θ_0, m_0, and physical constants, which is a kinematics problem.
- 🚀 The initial velocity is determined using conservation of energy, assuming negligible energy in the wheels' rotation.
- ⚖️ Potential energy at the top of the ramp is converted into kinetic energy at the launch point, leading to the equation v_0^2 = 2g h_0.
- 🧭 The horizontal displacement x_0 is calculated using the kinematic equation involving the time of flight, which is determined by the vertical motion.
- 🔄 The vertical motion is analyzed using the kinematic equation for displacement with initial upward velocity and acceleration due to gravity.
- 📉 The final expression for x_0 is derived using trigonometric identities and the previously found expression for v_0^2.
- 🔢 If the vertical distance h_0 is doubled, the number of cars the cyclist can jump over also doubles, leading to a conclusion of 12 cars.
- 📈 The speaker is asked to sketch a graph of the vertical component of the cyclist's velocity as a function of time, indicating initial and final velocity components.
Q & A
What is the primary problem being solved in the transcript?
-The primary problem is deriving an expression for the horizontal distance (x₀) traveled by a stunt cyclist after launching from a ramp, in terms of the height (h₀), launch angle (θ₀), mass (m₀), and gravitational acceleration (g).
What method is used to find the initial velocity of the stunt cyclist?
-The initial velocity is found using the conservation of energy principle, where the potential energy at the top of the ramp is converted into kinetic energy at the launch point.
Why is the rotational kinetic energy of the wheels neglected in this problem?
-The rotational kinetic energy of the wheels is neglected because there is no information provided about the energy of the wheels, so it is assumed to be negligible.
What is the expression derived for the initial velocity (v₀) of the cyclist?
-The initial velocity (v₀) is derived as v₀ = sqrt(2gh₀), where g is the gravitational acceleration and h₀ is the height of the ramp.
How is the time of flight calculated in this problem?
-The time of flight is calculated by considering the vertical motion of the cyclist, where the displacement is zero (since the cyclist lands back at the same vertical level) and solving the kinematic equation for time.
What kinematic equation is used to relate the horizontal distance (x₀) to time?
-The kinematic equation x₀ = v₀ * cos(θ₀) * t is used, where v₀ * cos(θ₀) is the horizontal component of the velocity and t is the time of flight.
What trig identity is used to simplify the final expression for x₀?
-The trigonometric identity used is 2 * sin(θ₀) * cos(θ₀) = sin(2θ₀), which simplifies the expression for x₀.
How does doubling the height (h₀) of the ramp affect the number of cars the cyclist can jump over?
-Doubling the height (h₀) results in doubling the horizontal distance (x₀), which means the cyclist can jump over twice as many cars, increasing the number from 6 to 12.
What does the graph of the vertical component of the cyclist's velocity (v_y) look like?
-The graph of the vertical component of the cyclist's velocity (v_y) is linear, starting at v₀ * sin(θ₀) and decreasing linearly to -sqrt(2gh₀) due to gravitational acceleration.
Why is the vertical velocity component symmetric around the time axis?
-The vertical velocity component is symmetric because of the conservation of energy and the assumption of no air resistance, meaning the kinetic energy remains the same at launch and landing, but with the direction of the vertical component reversed.
Outlines
🚴♂️ Physics of a Stunt Cyclist's Ramp Jump
The speaker begins by introducing the 2021 AP Physics 1 free response questions, focusing on the first question involving a stunt cyclist using a ramp to jump over cars. The task is to derive an expression for the horizontal distance (x0) the cyclist travels in terms of physical constants and given parameters like the vertical distance (h0), ramp angle (θ0), and combined mass (m0). The approach involves using conservation of energy to find the initial velocity (v0), assuming negligible energy loss in the wheels. The potential energy at the top of the ramp is converted into kinetic energy at the launch point, leading to the equation v0^2 = 2gh0. The kinematics of the jump are then discussed, breaking down the initial velocity into horizontal and vertical components, and using the time taken to travel horizontally to find x0. The final expression for x0 is derived using trigonometric identities and the energy equation, resulting in x0 = 2h0 sinθ0 cosθ0. The speaker also addresses a hypothetical scenario where the vertical distance is doubled, concluding that the number of cars jumped would also double.
📊 Analyzing the Stunt Cyclist's Vertical Velocity Over Time
In the second paragraph, the focus shifts to analyzing the vertical component of the stunt cyclist's velocity as a function of time, from the moment the cyclist leaves the ramp until just before landing. The speaker sketches a graph to represent this, starting with the initial vertical velocity component (v0 sine θ0) and ending with the final component just before landing, which is symmetrically opposite due to the conservation of energy and the absence of air resistance. The graph illustrates a linear decrease in vertical velocity due to gravity, with the initial and final velocities indicated on the vertical axis. The speaker uses the derived expression for v0 and the kinematic equations to explain the symmetry and the linear nature of the graph, emphasizing the importance of understanding both energy conservation and kinematic principles in analyzing the motion of the stunt cyclist.
Mindmap
Keywords
💡AP Physics 1
💡Free Response Questions
💡Stunt Cyclist
💡Conservation of Energy
💡Kinematics
💡Initial Velocity
💡Trigonometric Functions
💡Potential Energy
💡Kinetic Energy
💡Graph
💡Vertical Component
Highlights
Introduction to the 2021 AP Physics 1 free response questions.
Explanation of the first question involving a stunt cyclist and a ramp.
Assumption of negligible energy in the wheels for simplicity.
Derivation of the expression for initial velocity using conservation of energy.
Expression for initial velocity \( v_0^2 = 2g h_0 \) derived.
Breakdown of initial velocity into horizontal and vertical components.
Use of kinematic equations to find the horizontal displacement \( x_0 \).
Time calculation based on vertical motion and acceleration due to gravity.
Expression for \( x_0 \) in terms of \( v_0, \theta_0, \) and \( g \).
Trigonometric identity application in deriving the final expression for \( x_0 \).
Final expression for \( x_0 \) as \( 2 h_0 \sin(\theta_0) \cos(\theta_0) \).
Implication of doubling the vertical distance on the number of cars jumped.
Theoretical maximum number of cars that could be jumped over if \( h_0 \) is doubled.
Instruction to sketch a graph of the vertical component of the cyclist's velocity.
Graph representation of the initial and final vertical velocity components.
Explanation of symmetry in the graph due to conservation of energy and kinematics.
Final graph showing the linear decrease and increase in vertical velocity.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: