Decimals: Notation and Operations
TLDRIn this insightful video, Professor Dave delves into the world of decimals, building on our understanding of place values from units to hundreds and beyond. He introduces the concept of decimals as a means to represent fractions, such as slices of pizza, in a more straightforward way than improper fractions or mixed numbers. By extending place values to the right of the decimal point, he explains how decimals can accurately represent values between whole numbers, using examples from tenths to thousandths. The video further explores how to perform operations with decimals, emphasizing the importance of aligning place values for accurate calculation. This engaging lesson lays the groundwork for future discussions on converting between fractions and decimals, enhancing our grasp of numerical magnitudes and their operations.
Takeaways
- 😀 Decimals allow us to represent fractions of numbers using place values to the right of the decimal point
- 😊 The tenths place is 0.1, hundredths place is 0.01, thousandths place is 0.001, and so on, getting 10x smaller each time
- 🤓 We can represent mixed numbers like 1 1/10 as decimals like 1.1 by putting the fraction in the appropriate place value
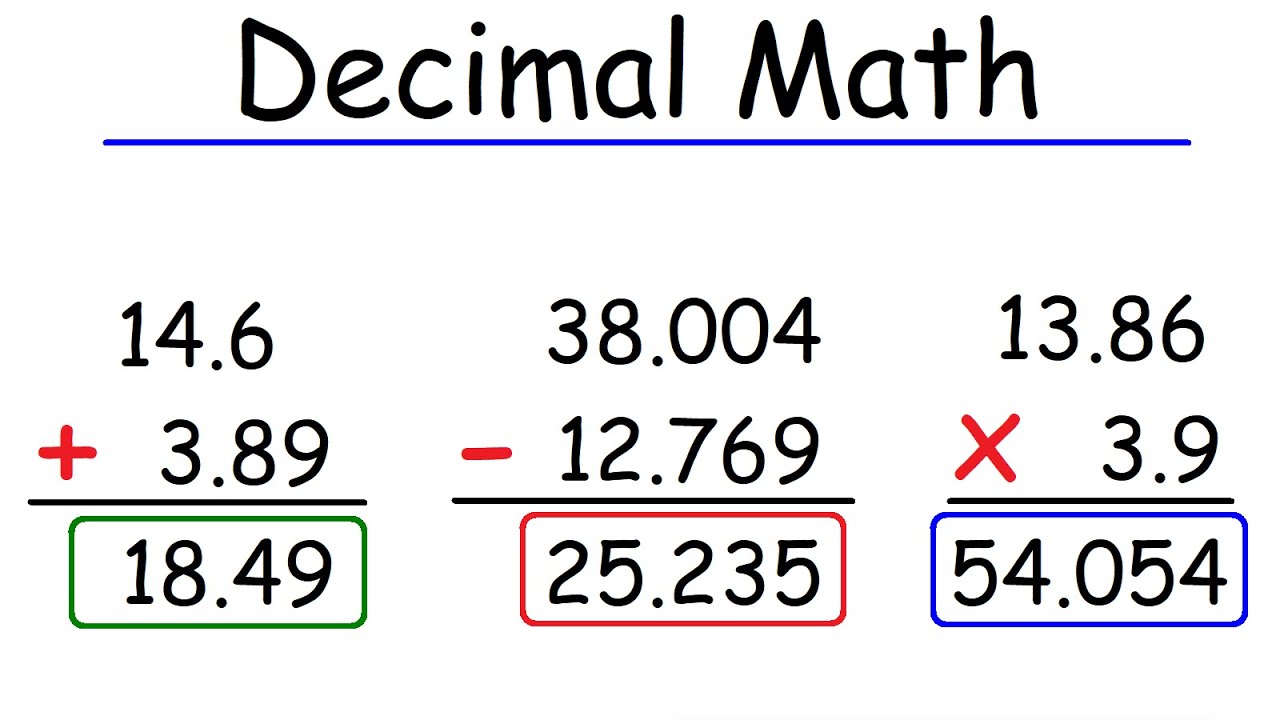
- 😃 Operations with decimals work just like regular numbers, we just have to line up the place values properly
- 😉 Place values allow a digit like 1 to represent vastly different magnitudes depending on its place value position
- 🧐 Converting between fractions and decimals will be covered later
- 👍🏻 The decimal point separates the whole number places on the left from the fractional places on the right
- 🙂 Each place value is 10x greater than the place to its right and 10x smaller than the place to its left
- 😯 Decimals allow us to precisely represent numbers between whole numbers using additional place value positions
- 🤔 Check comprehension on using place values with decimals to represent fractional quantities
Q & A
What are decimals used for?
-Decimals allow us to represent fractional values using place value, like representing 1 and 1/10 pizzas as 1.1. This lets us precisely represent values between whole numbers.
How does the decimal point work?
-The decimal point separates the whole number place values from the fractional place values. The first place value to the right of the decimal point is the tenths place.
How do the place values work to the right of the decimal point?
-To the right of the decimal point, each place value represents a factor of 10 smaller than the previous place value. For example, the tenths place is 10 times smaller than the ones place.
How can extremely small fractions be represented with decimals?
-By adding more place values to the right of the decimal point, such as the hundredths place and thousandths place, we can represent precise fractional values no matter how small.
How are decimals added and subtracted?
-To add or subtract decimals, you line up the decimal points and add or subtract each place value normally, just as with whole numbers.
How can fractions be converted to decimals?
-Fractions can be converted to decimals by dividing the numerator by the denominator. The result will be a decimal representation of the fraction.
Can decimals represent infinitely large numbers?
-Yes, by adding more place values to the left of the decimal point, decimals can represent extremely large numbers.
What operations can be performed on decimals?
-Decimals can be added, subtracted, multiplied, and divided just like regular numbers. The decimal points just need to be aligned first.
Are decimals more precise than fractions?
-Yes, decimals can represent fractional values to a greater precision than most fractions, since more place values can be added.
How are decimals useful in real life?
-Decimals are extremely useful for calculations in science, engineering, accounting, and anywhere that requires precise numerical values.
Outlines
📝 Understanding Decimals
This paragraph introduces the concept of decimals as a way to represent fractional values between whole numbers using place values. It explains how the decimal point is used to extend place values to the right to show tenths, hundredths, thousandths, etc. Examples are given using pizza slices to illustrate decimal values like 0.1 for one tenth.
Mindmap
Keywords
💡Place values
💡Decimal point
💡Fractions
💡Operations
💡Magnitude
💡Conversion
💡Unit fractions
💡Division
💡Visualization
💡Patterns
Highlights
Proposes a new deep learning model called ClipCap that can generate captions for images without paired image-text data
ClipCap leverages CLIP, a contrastive vision-language model, to align image regions with caption fragments
Achieves state-of-the-art performance on COCO and Flickr30k datasets compared to previous unpaired captioning methods
Points out a limitation of current image captioning models - reliance on large paired image-text datasets
Proposes a training method involving cross-modal alignment between image regions and text fragments
Emphasizes the value of not needing paired training data which is expensive to obtain at scale
Ablation studies validate the importance of the cross-modal alignment objective
Qualitative examples show ClipCap can generate descriptive, grounded captions compared to retrieval baselines
Limitation - sometimes repeats redundant phrases or hallucinates incorrect details in captions
Main conclusion is ClipCap significantly advances unpaired image captioning while removing reliance on paired data
Future work could explore refinements to reduce repetitive or incorrect phrases in generated captions
Overall an impactful paper that makes unpaired image captioning much more practical
Code and models are publicly available to facilitate follow-up research
Presents a new technical approach to a long-standing vision & language problem using self-supervision
Impressive results on standard benchmarks demonstrate effectiveness of the ClipCap framework
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: