AP Calculus AB: Lesson 3.1 Elementary Derivative Rules

Michelle Krummel

15 Oct 202046:27

EducationalLearning

32 Likes 10 Comments

TLDRThis lesson introduces various derivative rules for calculating derivatives of mathematical functions without using the limit definition. It covers power functions, exponential functions, and trigonometric functions, explaining the power rule, constant rule, and exponential rule. The instructor demonstrates how to apply these rules with examples and emphasizes the importance of memorizing common derivatives for efficiency. The lesson also discusses the sum/difference rule, constant multiple rule, and introduces the derivative of sine and cosine functions. The session concludes with practice problems to apply these rules and find the equations of tangent lines to given functions at specific points.

Takeaways

📚 The lesson covers rules for calculating derivatives of power, exponential, and trigonometric functions without using the limit definition of the derivative.
🔢 Power functions are expressed in the form y = k * x^p, where k and x are real numbers, and p is a real number power.
📈 The derivative of a power function follows the pattern where the original exponent becomes the new coefficient, and the new exponent is one less than the original.
👉 For example, the derivative of x^5 is 5x^4, showcasing the power rule in action.
📌 The derivative of x raised to any real number can be quickly found using the power rule, simplifying the process significantly.
📉 The constant rule states that the derivative of any constant value is zero, as constants do not change with respect to the variable.
🔼 The exponential rule is applied to functions of the form y = a^x, where the derivative is a^x times the natural logarithm of the base 'a'.
🤝 The constant multiple rule allows us to factor out constants from within the derivative, simplifying the calculation.
📊 Trigonometric functions follow specific derivative rules: sine(x) derivatives to cosine(x), and cosine(x) derivatives to -sine(x).
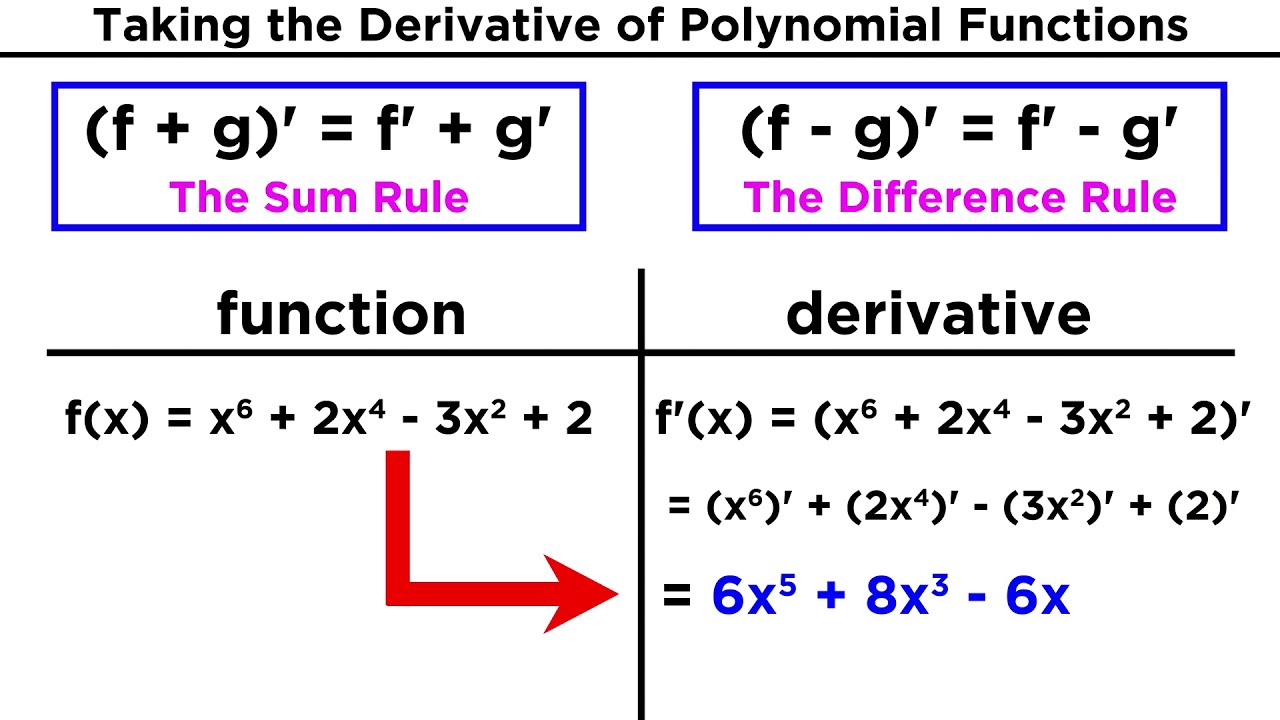
➕ The sum/difference rule allows for the derivative of a sum or difference of functions to be the sum or difference of their individual derivatives.
📘 Memorizing these rules is crucial for efficiently finding derivatives in calculus, avoiding the more complex limit definition method.

Q & A

What is the general form of a power function?
-A power function is generally in the form of y = k * x^p, where k and x are real numbers and p is a real number exponent.
How does the power rule simplify the process of finding derivatives for power functions?
-The power rule simplifies the process by allowing us to find the derivative without using the limit definition. It states that if f(x) = x^n, then f'(x) = n * x^(n-1).
What is the derivative of x^5 using the power rule?
-Using the power rule, the derivative of x^5 is 5 * x^(5-1), which simplifies to 5x^4.
What is the pattern observed in the derivatives of power functions regarding the exponents?
-The pattern is that the original exponent becomes the coefficient in the derivative, and the new exponent is one less than the original exponent.
How is the derivative of a constant function defined according to the constant rule?
-According to the constant rule, the derivative of a constant function, where f(x) = c (a real number), is f'(x) = 0, since the slope of a constant function is always zero.
What is the exponential rule for finding the derivative of an exponential function?
-The exponential rule states that for a positive real number a, if f(x) = a^x, then f'(x) = a^x * ln(a), where ln(a) is the natural logarithm of a.
What is the derivative of e^x?
-The derivative of e^x is e^x itself, because the natural logarithm of e is 1, making the derivative e^x * 1.
What is the constant multiple rule in calculus?
-The constant multiple rule states that the derivative of c * f(x), where c is a constant and f(x) is a differentiable function, is c * f'(x), allowing us to factor out the constant when taking the derivative.
What are the sine and cosine rules for derivatives?
-The sine rule states that the derivative of sin(x) is cos(x), and the cosine rule states that the derivative of cos(x) is -sin(x).
How can the sum/difference rule be used to find the derivative of a function that is a sum or difference of two functions?
-The sum/difference rule allows us to find the derivative of f(x) ± g(x) by taking the derivative of f(x) and adding or subtracting the derivative of g(x), which means (f ± g)'(x) = f'(x) ± g'(x).
What is the process for finding the equation of a tangent line to a curve at a given point?
-To find the equation of a tangent line at a point, you need to find the function's value at that point (to get the y-coordinate) and its derivative at that point (to get the slope). The equation of the tangent line is then y - y1 = m(x - x1), where (x1, y1) is the point of tangency and m is the slope.

Outlines

00:00

📚 Introduction to Derivative Rules for Functions

This paragraph introduces the topic of derivatives, specifically focusing on power, exponential, and trigonometric functions. It explains that the lesson will cover the calculation of derivatives without using the limit definition. Power functions are defined as functions of the form y = kx^p, with examples provided. The process of finding derivatives using the limit definition is discussed, and the pattern for the derivatives of power functions is identified, where the original exponent becomes the coefficient and the new exponent is one less than the original.

05:00

🔍 Power Rule and Constant Rule for Derivatives

The power rule for derivatives is explained, which states that for a function f(x) = x^n, the derivative f'(x) = n * x^(n-1). Examples are given to illustrate the rule, and the concept of rewriting functions in a simplified form without negative exponents is discussed. The constant rule is also introduced, which states that the derivative of a constant value is zero. This is demonstrated with various examples, including the derivative of the square root of x and the importance of memorizing certain derivatives for efficiency.

10:03

📈 Derivative Rules for Exponential and Trigonometric Functions

The exponential rule is introduced, which allows for the differentiation of functions of the form y = a^x, with the derivative being y' = a^x * ln(a). Examples using bases 2, 5, and e are provided, highlighting that e^x is its own derivative. The constant multiple rule is also discussed, explaining that a constant factor can be taken outside the derivative. The sine and cosine rules for derivatives are introduced, with the derivative of sin(x) being cos(x) and the derivative of cos(x) being -sin(x), emphasizing the need to memorize these relationships.

15:06

🔢 Derivative Rules for Sum, Difference, and Constant Multiple

The sum/difference rule is explained, which allows for the differentiation of sums and differences of functions by taking the derivative of each term separately. The constant multiple rule is further elaborated upon with examples of linear functions, where the derivative is simply the coefficient of x. The paragraph also covers the application of these rules to various functions, including those involving square roots and fractions, and the simplification required before differentiation.

20:07

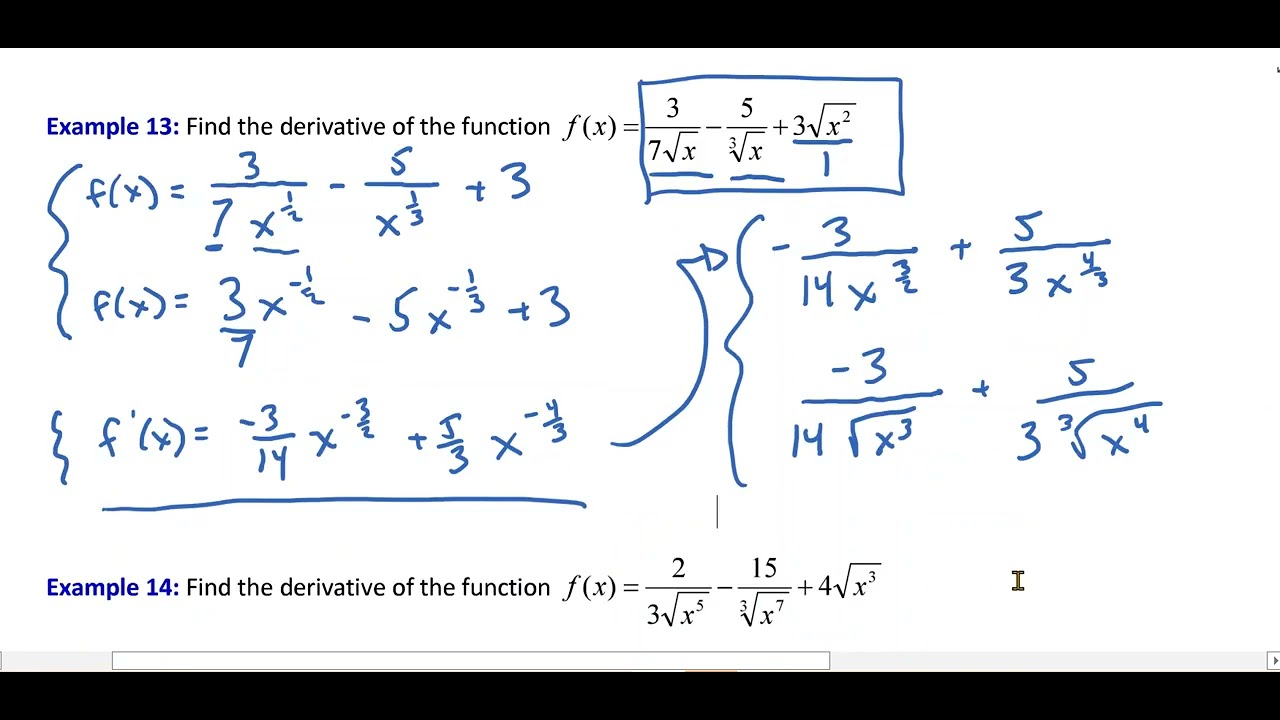
🤔 Application of Derivative Rules to Complex Functions

This paragraph delves into applying the derivative rules to more complex functions, such as those involving both exponential and power components. It demonstrates the process of rewriting functions to fit the derivative rules and combining the results to find the overall derivative. Examples include functions with terms like x^(5/2), x^4, and 2^x, where the power and exponential rules are combined with the constant multiple rule to find the derivative.

25:10

📘 Simplification and Derivatives of Products and Quotients

The paragraph discusses the need to simplify expressions before applying derivative rules, especially when dealing with products and quotients. It clarifies that derivative rules do not apply to the multiplication or division of derivatives directly. Examples of simplifying complex expressions into forms that can be differentiated using known rules are provided, including the process of rewriting terms with negative exponents and separating fractions.

30:15

📌 Finding Tangent Lines and Rates of Change

The application of derivatives to find the equations of tangent lines and rates of change is explored. The process involves finding the function value and its derivative at a specific point to determine the slope of the tangent line. Examples include finding the tangent line to functions at given points, emphasizing the importance of memorizing derivative rules for common functions to simplify the process.

35:17

🎓 Summary of Derivative Rules and Encouragement for Practice

The final paragraph summarizes the various derivative rules covered in the lesson, including the power, exponential, sum/difference, constant multiple, and rules for functions involving products and quotients. It emphasizes the utility of these rules in simplifying the process of finding derivatives without the need for the limit definition. The instructor encourages practice to master these rules and improve the speed and accuracy of derivative calculations.

Mindmap

Keywords

💡Derivative

A derivative in calculus represents the rate at which a function changes with respect to its variable. It is a fundamental concept in the study of functions and their behavior. In the video, derivatives are calculated for various types of functions, such as power, exponential, and trigonometric, to understand their rates of change without using the limit definition.

💡Power Function

A power function is a mathematical function of the form y = k * x^p, where k and x are real numbers, and p is a real number exponent. The video discusses the derivatives of power functions and introduces the power rule for finding derivatives, which states that the derivative of x^p is p * x^(p-1).

💡Limit Definition

The limit definition of the derivative is a formal approach to defining the derivative of a function at a certain point, using the concept of limits in calculus. Although the video aims to teach derivative calculations without this method, it mentions the limit definition as the traditional way to find derivatives, such as f'(x) = lim(h->0) [(f(x+h) - f(x))/h].

💡Exponential Function

An exponential function is a function where the variable is the exponent, typically represented as y = a^x, where 'a' is the base, and 'x' is the exponent. The video explains the exponential rule for derivatives, which states that the derivative of a^x is a^x * ln(a), where ln(a) is the natural logarithm of the base 'a'.

💡Trigonometric Functions

Trigonometric functions are functions of an angle, commonly used in mathematics and engineering. The video covers the derivatives of sine and cosine functions, stating that the derivative of sin(x) is cos(x) and the derivative of cos(x) is -sin(x), which are essential for solving problems involving periodic phenomena.

💡Constant Rule

The constant rule in calculus states that the derivative of a constant value is zero. This is because a constant does not change with respect to the variable, so its rate of change is zero. The video uses this rule when discussing the derivative of a constant function, such as y = 2, where the derivative is simply 0.

💡Constant Multiple Rule

The constant multiple rule allows for the factorization of a constant out of a derivative. If 'c' is a constant and 'f(x)' is a differentiable function, then the derivative of c * f(x) is c * f'(x). The video demonstrates this rule by showing that the derivative of a function multiplied by a constant can be calculated by multiplying the constant by the derivative of the function.

💡Sum/Difference Rule

The sum/difference rule states that the derivative of a sum or difference of functions is the sum or difference of their derivatives. If f(x) and g(x) are differentiable functions, then the derivative of (f(x) ± g(x)) is f'(x) ± g'(x). The video uses this rule to find the derivatives of functions that are sums or differences of other functions.

💡Slope of a Tangent Line

The slope of a tangent line to a curve at a given point is equal to the derivative of the function at that point. The video explains how to find the equation of a tangent line by using the function's value and its derivative at a specific point, illustrating this with examples such as the tangent line to y = x^2 at x = 4.

💡Leibniz Notation

Leibniz notation is a form of notation used to express the derivative, written as dy/dx or df/dx, where 'y' or 'f' represents the function and 'x' represents the variable. The video mentions this notation in the context of taking the derivative of one function with respect to another, such as da/db, which represents the derivative of 'a' with respect to 'b'.

Highlights

Introduction to rules for calculating derivatives of power, exponential, and trigonometric functions without using the limit definition.

Explanation of power functions and their general form, y = k * x^p, where k and x are real numbers and p is a real number power.

Derivatives of power functions are discussed, showing the pattern where the original exponent becomes the new coefficient and the new exponent is one less.

The power rule for derivatives is introduced, stating that for a power function f(x) = x^n, the derivative f'(x) = n * x^(n-1).

Differentiation between power functions and exponential functions, emphasizing the variable's position in each function type.

The exponential rule for derivatives is presented, showing how to find the derivative of a function in the form of a^x where a is a positive real number.

Constant rule for derivatives is explained, stating that the derivative of a constant value is zero.

Introduction of the constant multiple rule, which allows for the separation of constants from functions when taking derivatives.

Derivative rules for sine and cosine functions are presented, with sine(x)' = cos(x) and cosine(x)' = -sin(x).

Sum and difference rule for derivatives is explained, allowing for the derivative of a sum or difference of functions to be the sum or difference of their derivatives.

Practical application of derivative rules to find the slope of the tangent line to a function at a given point.

Demonstration of finding the derivative of a function involving both a power function and an exponential function.

Explanation of how to handle negative exponents when applying the power rule for derivatives.

The importance of memorizing derivative rules for efficiency in solving calculus problems.

Differentiation between Leibniz notation and function notation when dealing with derivatives, and how to apply rules correctly with varying notation.

Final practice problems that integrate various derivative rules to find the equations of tangent lines to given functions at specific points.

Emphasis on the efficiency of using derivative rules compared to the limit definition for finding derivatives.

Transcripts

Browse More Related Video

Derivatives of Trig Functions - Calculus | MCV4U

Calculus AB Homework 3.2 The Product Rule

AP Calculus AB: Lesson 3.2 The Product Rule

Basic Derivative Rules - Part 2: Combining the Rules

Derivatives of Polynomial Functions: Power Rule, Product Rule, and Quotient Rule

1.5 - The Power and Sum Difference Rules

Related Tags

Derivative Rules Power Functions Exponential Functions Trigonometric Functions Calculus Lesson Limit Definition Educational Content Mathematics Tutorial Slope of Tangent Leibniz Notation Constant Multiple Rule