Video 87 - Ricci & Einstein Tensors

Tensor Calculus

5 Jun 202221:36

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the intricacies of tensor calculus, focusing on the derivation and significance of the Ricci tensor and Ricci scalar from the Riemann tensor through contraction. It explains the symmetrical properties of the Ricci tensor and introduces the Ricci scalar as an invariant scalar value. The script further explores the contracted Bianchi identity, leading to the definition of the Einstein tensor, which is pivotal in Einstein's theory of general relativity due to its zero divergence property. The video concludes with a transition to the study of curves in upcoming lessons.

Takeaways

📚 The script introduces the Ricci tensor and the Einstein tensor, both derived from the Riemann tensor through contraction.
🔍 The Ricci tensor is obtained by contracting the Riemann tensor between the upper index and the middle index on the bottom, resulting in a second-rank symmetric tensor.
📉 Contracting the Riemann tensor in any other way than the upper index with the middle bottom index results in either zero or redundant information.
🔄 The Ricci tensor is symmetric, meaning its value does not change when the two indices are swapped.
📈 The Ricci scalar is defined as the contraction of the Ricci tensor, or equivalently, the double contraction of the Riemann tensor, yielding an invariant scalar value.
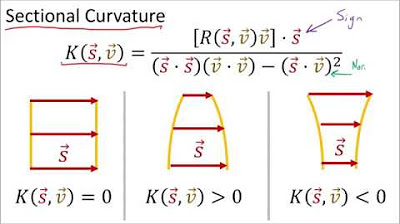
🌐 The Gaussian curvature (kappa) is shown to be equal to one-half of the Ricci scalar, providing a method to find curvature from the Ricci scalar.
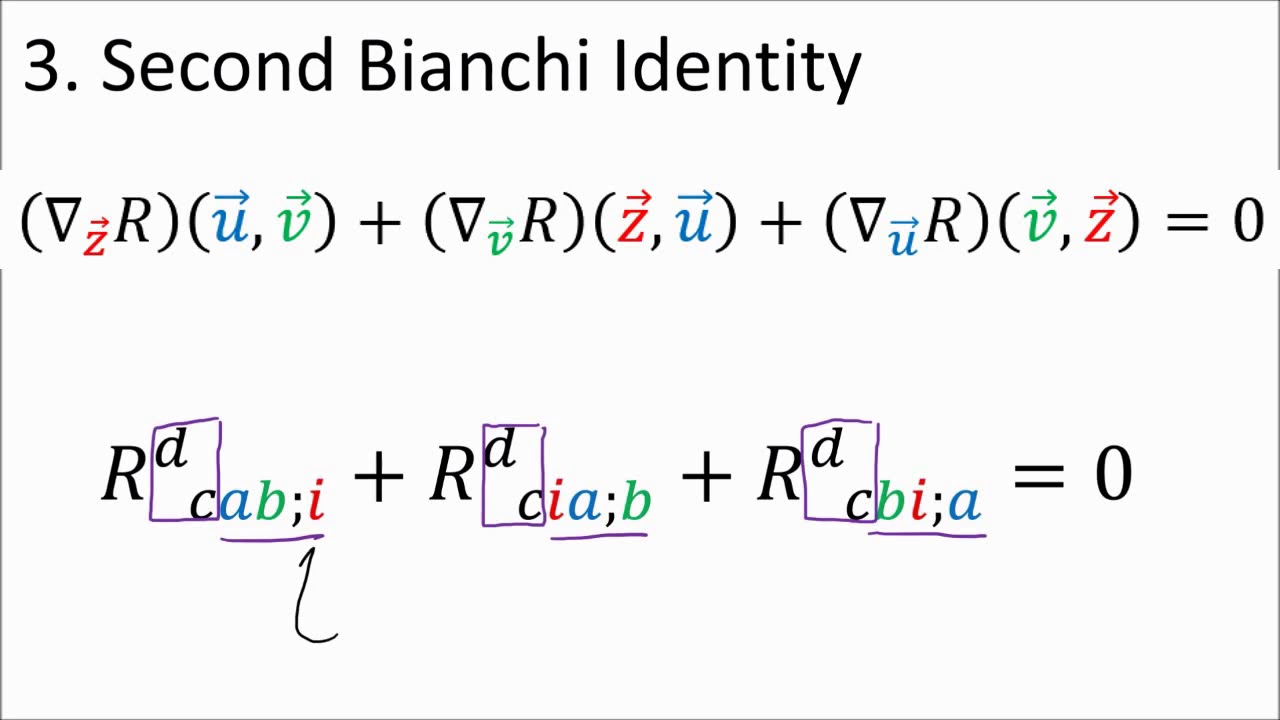
🔗 The contracted Bianchi identity, derived from the second Bianchi identity, reveals that the divergence of the Ricci tensor plus an additional term equals zero.
🌌 The Einstein tensor is defined to have a divergence of zero, making it a normalized version of the Ricci tensor, and is crucial in Einstein's general theory of relativity.
🔑 The Einstein tensor carries the same curvature information as the Ricci tensor but with the added property of having zero divergence.
🔄 The script demonstrates the derivation of key tensor operations and identities, emphasizing the importance of index placement in tensor contractions.
🚀 The video concludes with a transition to the next topic, which will focus on curves, indicating a shift from studying curvature in surfaces to curves.

Q & A

What is the Ricci tensor?
-The Ricci tensor is a second-rank tensor derived from the Riemann tensor through a specific contraction between the upper index and the middle index on the bottom, resulting in a tensor with two free indices.
Why is the Ricci tensor the only meaningful contraction from the Riemann tensor?
-Contracting the first index with the middle one on the bottom results in zero, and contracting the upper index with the third one on the bottom gives the same components as the original but with negative signs, thus the Ricci tensor is the only contraction that yields new and meaningful information.
How is the Ricci tensor related to the Riemann tensor?
-The Ricci tensor is derived from the Riemann tensor by performing a contraction, which reduces the rank of the tensor from four to two.
What is the Ricci scalar?
-The Ricci scalar is an invariant scalar value obtained by contracting the Ricci tensor, effectively contracting its two indices to get a single scalar quantity.
How does the Ricci scalar relate to the Gaussian curvature?
-The Gaussian curvature, denoted by kappa, is equal to one half of the Ricci scalar, providing a way to find the curvature value from the scalar.
What is the contracted Bianchi identity?
-The contracted Bianchi identity is an important relationship that shows the divergence of the Ricci tensor with an additional term, resulting in a tensor whose divergence is zero.
Why is the divergence of the Ricci tensor not zero, but the divergence of the Einstein tensor is?
-The divergence of the Ricci tensor is not zero by itself, but when an additional term is included, as in the definition of the Einstein tensor, the divergence becomes zero, which is a key property for the Einstein tensor.
What is the significance of the Einstein tensor in the context of general relativity?
-The Einstein tensor is a pivotal component in Einstein's theory of general relativity, representing the curvature of space-time in a way that its divergence is zero, which is a requirement for the field equations of general relativity.
How is the Einstein tensor defined in relation to the Ricci tensor?
-The Einstein tensor is defined as the Ricci tensor with an additional term that normalizes it, ensuring that its divergence is zero.
What property of the Einstein tensor makes it suitable for representing the curvature of space-time in general relativity?
-The Einstein tensor's property of having a zero divergence makes it suitable for representing the curvature of space-time in general relativity, as it meets the requirement for the field equations.
What is the next topic that the script mentions will be covered in the series?
-The next topic mentioned in the script is curves, which will be the focus of the subsequent video in the series.

Outlines

00:00

📚 Introduction to the Ricci Tensor and Einstein Tensor

This paragraph introduces the Ricci tensor, derived from the Riemann tensor through contraction, specifically between the upper index and the middle index on the bottom. It explains that this contraction results in a second-rank tensor, which is the only meaningful way to contract the Riemann tensor. The paragraph also explores other potential contractions, demonstrating that they either result in zero or are redundant. The Ricci tensor is shown to be symmetric with respect to its two free indices.

05:02

🔍 Exploring the Ricci Tensor's Properties and the Ricci Scalar

The second paragraph delves deeper into the properties of the Ricci tensor, explaining how it can be used to form the Ricci scalar through a contraction, which is an invariant scalar value. It also discusses the Gaussian curvature and its relationship with the Ricci scalar, showing that the Gaussian curvature is equal to half of the Ricci scalar. Additionally, the paragraph revisits the second Bianchi identity and demonstrates how the Ricci tensor can be manipulated to derive important identities.

10:03

🌐 Derivation of the Contracted Bianchi Identity and Divergence

In this paragraph, the contracted Bianchi identity is derived, which involves manipulating the second Bianchi identity and introducing contractions to reduce the rank of the tensor. The resulting identity reveals the divergence of the Ricci tensor and shows that adding a specific term normalizes the tensor so that its divergence equals zero. This leads to the definition of the Einstein tensor, which is a key concept in the study of general relativity.

15:04

🌌 The Einstein Tensor and Its Role in General Relativity

The Einstein tensor is introduced as a tensor that carries the same curvature information as the Ricci tensor but with a divergence equal to zero. This property is crucial for its application in Einstein's theory of general relativity, where it represents the curvature of space-time. The paragraph explains the definition of the Einstein tensor and its symmetry, which is a result of the symmetry of the Ricci tensor and the metric tensor. The Einstein tensor is shown to be a pivotal component in the field equations of general relativity.

20:06

🛤️ Transition to the Study of Curves in Tensor Calculus

The final paragraph wraps up the discussion on the Ricci and Einstein tensors and their significance in the study of curvature, particularly in the context of surfaces. It then announces a shift in focus to the study of curves, indicating that future videos will explore this topic in the context of tensor calculus.

Mindmap

Keywords

💡Riemann Tensor

The Riemann Tensor is a fundamental concept in differential geometry and general relativity, describing the curvature of spacetime. In the video, it is the starting point for deriving other tensors, such as the Ricci Tensor and the Einstein Tensor. The script discusses how contraction of the Riemann Tensor leads to the formation of the Ricci Tensor, emphasizing its importance in understanding the intrinsic curvature properties of a manifold.

💡Ricci Tensor

The Ricci Tensor is a type of contraction of the Riemann Tensor, obtained by contracting the last two indices. It is a second-rank symmetric tensor that provides a measure of the curvature of a space at a point. In the script, the Ricci Tensor is derived from the Riemann Tensor and is shown to be symmetric, which is crucial for its role in the Einstein field equations of general relativity.

💡Einstein Tensor

The Einstein Tensor is a modification of the Ricci Tensor, with an additional term that ensures its divergence is zero. This property is significant as it aligns with the requirement for a tensor representing the curvature of spacetime in Einstein's field equations. The script explains how the Einstein Tensor is derived from the contracted Bianchi identity, which is a key step in normalizing the tensor.

💡Contraction

In the context of the video, contraction refers to the operation of reducing the rank of a tensor by summing over shared indices. The script demonstrates how contraction is used to derive the Ricci Tensor from the Riemann Tensor and further to the Ricci Scalar. Contraction is a key operation in tensor calculus, allowing for the simplification and combination of tensorial information.

💡Ricci Scalar

The Ricci Scalar is obtained by contracting the Ricci Tensor, resulting in an invariant scalar quantity that can be used to describe the curvature of a manifold. In the script, the Ricci Scalar is shown to be related to the Gaussian curvature and is an important quantity in the study of curvature and its physical implications.

💡Gaussian Curvature

Gaussian Curvature is a measure of the intrinsic curvature of a surface at a point, defined as the product of the principal curvatures. In the video, the Gaussian Curvature is related to the Ricci Scalar, with the script showing that it can be found by multiplying the Ricci Scalar by one-half, providing a direct connection to the tensorial description of curvature.

💡Bianchi Identity

The Bianchi Identity is a fundamental identity in the theory of Riemannian geometry, relating the covariant derivative of the Riemann Tensor to itself. In the script, the contracted form of the Bianchi Identity is derived, which is significant because it leads to the definition of the Einstein Tensor and provides insight into the properties of curvature.

💡Covariant Derivative

The covariant derivative is a way to differentiate tensors in a way that is consistent with the curvature of the space they are defined on. In the script, the covariant derivative is used in the derivation of the contracted Bianchi identity and is crucial for understanding how tensors change under parallel transport along curves in a curved space.

💡Metric Tensor

The Metric Tensor is a fundamental object in differential geometry that defines the notion of distance and angle in a curved space. In the script, the Metric Tensor is used to raise and lower indices of tensors, which is essential for the manipulation and contraction of tensors like the Riemann and Ricci Tensors.

💡Divergence

In the context of the video, divergence refers to a mathematical operation that measures the magnitude of a vector field's source or sink at a given point. The script discusses how the divergence of the Einstein Tensor is zero, which is a key property for its role in the field equations of general relativity, indicating that the tensor is 'conservative' in a certain sense.

Highlights

Introduction of the Ricci tensor, derived from the Riemann tensor through contraction.

Explanation of the contraction process and its effect on the rank of the resulting tensor.

Demonstration of the Ricci tensor's symmetry with respect to its two indices.

Derivation of the Ricci scalar from the Ricci tensor, an invariant scalar value.

Relation of the Gaussian curvature to the Ricci scalar, showing kappa equals one half of the Ricci scalar.

Application of the second Bianchi identity to derive an important identity involving the Ricci tensor.

Transformation of the contracted Bianchi identity into a form that reveals the divergence of the Ricci tensor.

Introduction of the Einstein tensor and its definition in relation to the Ricci tensor.

Explanation of the Einstein tensor's divergence being equal to zero and its significance.

Discussion on the symmetry of the Einstein tensor due to the symmetry of the metric tensor.

Connection of the Einstein tensor to Einstein's work in general relativity and its role in representing space-time curvature.

The unique method of forming the Ricci tensor by contracting the Riemann tensor in a specific manner.

The zero value obtained when contracting certain indices of the Riemann tensor, indicating the uniqueness of the Ricci tensor's definition.

The practical application of the Ricci tensor in determining the Gaussian curvature of a surface.

The contracted Bianchi identity's role in normalizing the Ricci tensor to create the Einstein tensor.

Shift in focus from curvature of surfaces to curves, indicating the progression of the series.

Transcripts

Browse More Related Video

Tensor Calculus 26 - Ricci Tensor/Scalar Properties

Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)

Tensor Calculus 25 - Geometric Meaning Ricci Tensor/Scalar (Volume Form)

Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries

The Einstein Field Equations | Tensor Calc Finale

Einstein Field Equations - for beginners!

Related Tags

Tensor Calculus Ricci Tensor Einstein Tensor Space-Time Curvature Theory General Relativity Riemann Tensor Bianchi Identity Curvature Scalar Metric Tensor