Calculus Made EASY! Understanding the derivative
TLDRIn this informative video, Doctor Ji explains the concept of derivatives in calculus, highlighting the difference between average and instantaneous slope. He clarifies that while the slope of a linear function is constant and equals the secant line, the slope of a quadratic function varies and can be represented graphically by the derivative function. The video also introduces the process of differentiation and different notations used to represent derivatives, including the second and third derivatives.
Takeaways
- 📚 The derivative in calculus represents the instantaneous rate of change or slope of a function.
- 🔄 Derivatives can be misunderstood as just 'rise over run'; however, in calculus, they encompass both average and instantaneous slopes.
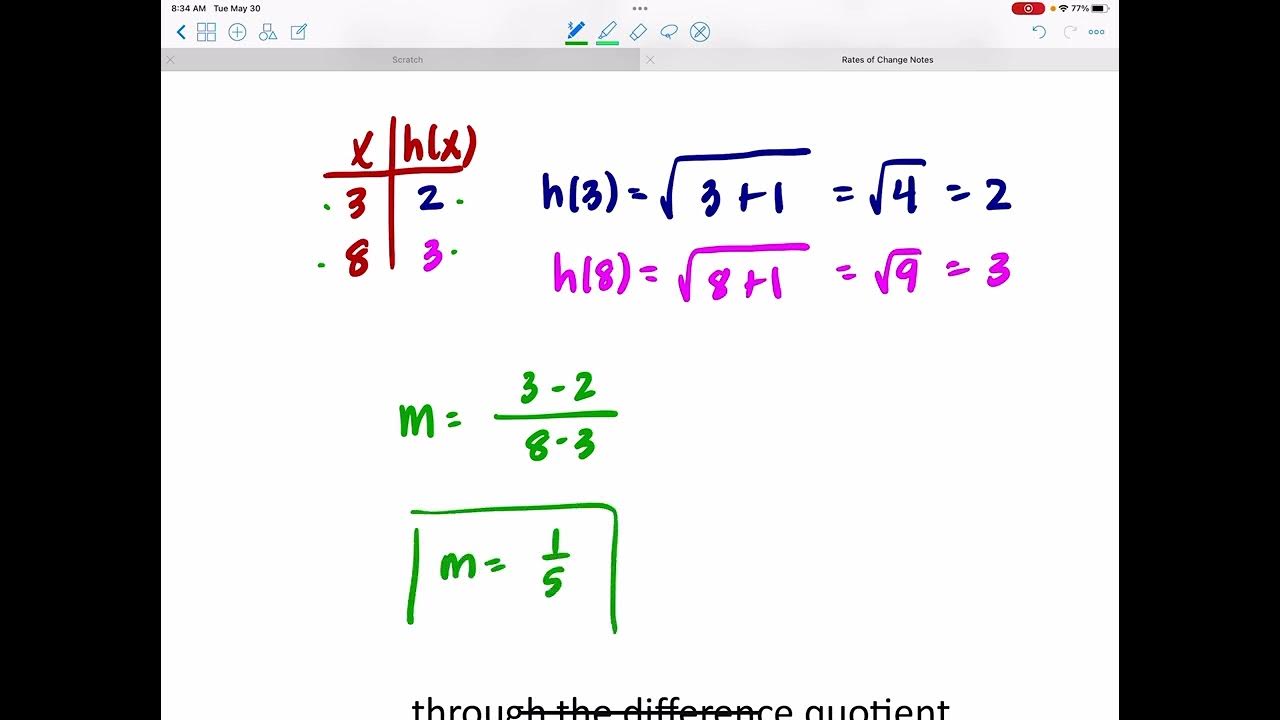
- 📈 The average slope is calculated using the rise over run between two points on a graph, representing the secant line.
- 📊 The instantaneous slope is the slope of the tangent line at a specific point on a graph, touching but not intersecting the graph.
- 🤖 For linear functions, the slope is constant and equals both the average and instantaneous slopes, making calculus unnecessary for finding instantaneous change.
- 📈 In contrast, quadratic functions have varying slopes at different points, requiring calculus to determine the instantaneous slope.
- 🔢 Differentiation is the process used in calculus to find the derivative of a function.
- 📊 The derivative function can be graphed, showing the pattern of how the slope changes across the original function.
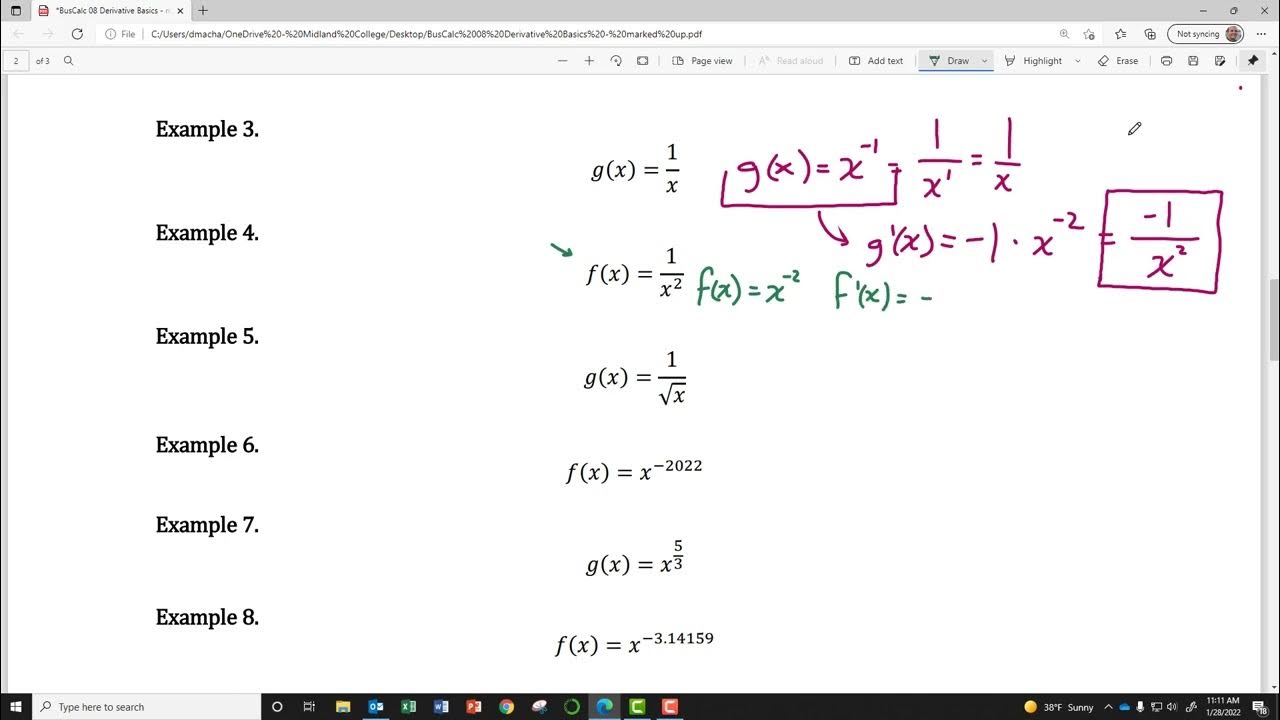
- 📉 Derivatives decrease in degree by one compared to the original function: linear to constant, quadratic to linear, and so on.
- 📝 There are multiple notations for representing derivatives, with Leibniz and Lagrange notations being the most common.
- 🔄 Further differentiation can yield higher-order derivatives, such as the second and third derivatives, each with its notation.
Q & A
What is the fundamental concept of the derivative in calculus?
-The derivative in calculus represents the instantaneous rate of change or the instantaneous slope of a function at a specific point. It describes the rate at which a quantity changes with respect to another quantity at that particular point.
What is the difference between average slope and instantaneous slope?
-Average slope is the slope of a line (secant line) that connects two points on a graph, calculated as the rise over run (change in y over change in x) between those two points. Instantaneous slope, on the other hand, is the slope of the tangent line at a specific point on the graph, which touches the graph only at that point and provides the slope at that exact location.
How do you find the slope of a linear function?
-The slope of a linear function can be found by using two points on the function and calculating the rise over run (change in y over change in x) between those points. Alternatively, the slope can be determined directly from the function's equation if it's in the form y = mx + b, where m represents the slope.
Why is calculus necessary for finding the slope of a quadratic function?
-Calculus is necessary for finding the slope of a quadratic function because the slope changes at every point on the curve. Unlike linear functions, where the slope is constant, quadratic functions require differentiation to determine the varying slope at any given point, which is represented by the instantaneous slope.
What is the relationship between the degree of the original function and its derivative?
-The derivative of a function is always one degree lower than the original function. For example, the derivative of a quadratic function (degree 2) is linear (degree 1), the derivative of a cubic function (degree 3) is quadratic (degree 2), and so on.
How does the derivative of a constant function behave?
-The derivative of a constant function is always zero because the slope of a flat horizontal line, which represents no change, is zero regardless of the constant's value.
What are the two methods for finding derivatives?
-There are two primary methods for finding derivatives: using the definition of the derivative, which involves limits and the concept of delta change, and using derivative rules, which are a set of formulas that simplify the process of differentiation once the basic concept is understood.
What is the notation used to represent the derivative of a function?
-The derivative of a function can be represented using various notations, such as dy/dx, f'(x), or d/dx(f(x)). These notations indicate that the derivative is being taken with respect to the variable x.
What is the significance of the second and third derivatives?
-The second derivative represents the rate of change of the first derivative, giving insight into the concavity and curvature of the function. The third derivative provides information about the rate of change of the second derivative, offering further detail on the function's behavior, such as its inflection points.
How does the slope of the derivative function relate to the original function?
-The slope of the derivative function at any given x-value corresponds to the instantaneous slope of the original function at that point. This means that the graph of the derivative function can be used to visualize the slopes of the original function at all of its points.
What is the role of calculus in understanding changes in functions?
-Calculus, particularly differential calculus, is essential for understanding and quantifying instantaneous changes in functions. It allows us to find the exact rate of change or slope at any point on a curve, which is crucial for modeling and analyzing a wide range of real-world phenomena that involve change over time or space.
Outlines
📚 Introduction to Derivatives in Calculus
This paragraph introduces the concept of derivatives in calculus, explaining that derivatives represent the instantaneous rate of change or slope of a function. It differentiates between average slope, which is the rise over run, and instantaneous slope, which is the slope of the tangent line at a specific point on a graph. The explanation includes a comparison between linear and quadratic functions, highlighting that while the slope of a linear function is constant and can be found without calculus, the slope of a quadratic function varies and requires calculus to determine the instantaneous slope at specific points.
🔍 Understanding the Derivative Function
This paragraph delves into the mathematical and graphical aspects of derivatives. It explains how the derivative function, denoted as f'(x), provides the slope of the tangent line at any given point on the original function, f(x). The difference between finding the y-coordinate of a point on the original function and the slope at that point on the derivative function is clarified. The paragraph also touches on the concept of higher-order derivatives and the rule that the derivative of a function is always one degree lower than the original function. The importance of differentiation in finding derivatives is mentioned, with a promise to cover different methods of differentiation in future videos.
📝 Derivative Notation and Further Differentiation
The final paragraph discusses various notations used to represent derivatives, including the common Leibniz and Lagrange notations. It explains that derivatives can be denoted in multiple ways, and these notations can be adapted for different function formats, such as y = f(x). The concept of finding higher-order derivatives, such as the second and third derivatives, is introduced, with notations for these also provided. The video concludes with a call to action for viewers to subscribe and follow the YouTube channel and social media platforms for more content.
Mindmap
Keywords
💡derivative
💡instantaneous rate of change
💡slope
💡secant line
💡tangent line
💡differentiation
💡linear function
💡quadratic function
💡rate of change
💡rise over run
💡derivative notation
Highlights
The fundamental concept of the derivative in calculus is introduced as the instantaneous rate of change or slope.
Derivative can be misleading for students as it differs from the traditional understanding of slope as rise over run.
The derivative can be represented as Delta Y over Delta X, indicating the change in the dependent variable over the change in the independent variable.
There is a distinction between average slope, represented by the secant line, and instantaneous slope, represented by the tangent line.
For linear functions, the slope can be found using two points and is consistent as both the average and instantaneous slope.
The slope of a linear function is constant and equals the slope of the secant line as well as any imaginable tangent lines.
The equation y = mx + b represents the linear function with 'm' as the slope, which is the average and instantaneous slope.
Calculus is not necessary to find the instantaneous change in a linear function since its slope is instantaneous at any point.
Quadratic functions require calculus to find the instantaneous slope as the slope changes and is not immediately apparent from secant lines.
Differentiation is the process used in calculus to find the derivative of a function.
The derivative function graphically represents the pattern of changing slopes of the original function.
Derivatives decrease in degree by one compared to the original function, with the derivative of a linear function being constant.
A constant original function results in a derivative that is always zero, as the slope of a flat line is zero.
Two methods for differentiation are mentioned: using the definition of the derivative and using derivative rules.
Different notations are used to represent derivatives, with Leibniz and Lagrange notations being the most common.
Higher order derivatives, such as the second and third derivatives, can be found, each represented with specific notations.
The video provides a comprehensive overview of the concept of derivatives, their calculation, and their graphical representation.
The practical application of derivatives in understanding the behavior of functions, particularly in determining slopes, is discussed.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: