Tensor Calculus Lecture 7d: The Voss-Weyl Formula

MathTheBeautiful

16 Apr 201420:06

EducationalLearning

32 Likes 10 Comments

TLDRIn this lecture, the speaker discusses the F vile formula, an alternative expression for the divergence in tensor notation, highlighting its significance and elegance. The formula is named after Hermann Weyl, a renowned 20th-century geometer, and Conrad Foss, a living mathematician the speaker had the pleasure of meeting. The speaker emphasizes the simplicity and beauty of the formula, which allows for the evaluation of divergences and Laplacians without the need for computing Christoffel symbols. The lecture also covers the derivation of the formula and its application to various coordinate systems, promising future lectures on its practical use.

Takeaways

📚 The F vile formula is named after Hermann Weyl and Conrad Foss, with Weyl being a renowned geometer and Foss still living in Switzerland.
🌐 Hermann Weyl's book 'Space, Time, Matter' is highly regarded and is recommended for study, especially after understanding the lectures on the F vile formula.
🤔 The F vile formula provides an alternative expression for the divergence, which is an invariant combination in tensor notation.
📉 The formula allows for the evaluation of divergences without the need for computing Christoffel symbols, offering a more direct approach.
📝 The derivation of the F vile formula involves the application of the product rule and understanding the partial derivatives of the metric tensor.
🔍 The significance of the F vile formula lies in its ability to simplify expressions and provide a more structured and aesthetically appealing result.
📚 The formula is often used in the context of the Laplacian operator, which is closely related to the divergence.
🧩 The derivation process involves recognizing the symmetry in the indices and the cancellation of terms, leading to a neat and compact result.
📈 The formula is particularly useful in various coordinate systems, where it can simplify the expressions for divergences and Laplacians.
🔬 The script suggests that the F vile formula is an elegant tribute to someone's accomplishments when something simple is named after them.
🎓 The speaker plans to dedicate future lectures to the applications of the F vile formula, emphasizing its importance in the field of mathematics and physics.

Q & A

Who was Hermann Weyl, and what is his significance in geometry?
-Hermann Weyl was a preeminent geometer of the 20th century, originally from Germany. He made significant contributions to mathematics and physics, particularly in geometry and relativity. He wrote a notable book called 'Space-Time-Matter,' which is highly regarded for its thorough and thoughtful exposition of these topics.
What is the F Weyl formula, and why is it important?
-The F Weyl formula is an elegant mathematical expression used as an alternative to computing the Divergence in geometry. It allows for the evaluation of Divergence without needing to compute Christoffel symbols, making the process simpler and the resulting expressions more structured and aesthetically pleasing.
Who is Conrad Föss, and what role does he play in the F Weyl formula?
-Conrad Föss is a Swiss mathematician whose name is associated with the F Weyl formula, although he was unaware of this until later in life. He is a respected figure in mathematics, still active and contributing to the field. The formula's name is mainly recognized in Russian literature.
Why is the F Weyl formula significant in the context of tensor notation and geometric analysis?
-The F Weyl formula is significant because it provides a more direct and conceptually clear way to evaluate Divergence using tensor notation. It avoids the need for more complex computations involving Christoffel symbols, thereby offering a more intuitive understanding of the underlying geometry.
What is the relationship between the F Weyl formula and the Laplacian?
-The F Weyl formula is closely related to the Laplacian, as the Divergence is only a step away from the Laplacian. In particular, the formula can be used to express the Laplacian in different coordinate systems, revealing structural insights into how terms are grouped in the resulting expressions.
How does the F Weyl formula compare to traditional methods of computing Divergence?
-The F Weyl formula offers a simpler and more elegant approach to computing Divergence by avoiding the computation of Christoffel symbols. This makes the process more efficient and the results more aesthetically appealing, with better-structured expressions.
Why did the speaker choose to discuss the F Weyl formula in these lectures?
-The speaker chose to discuss the F Weyl formula because of its elegance and significance in geometric analysis. It provides a valuable alternative method for computing Divergence and is a tribute to the accomplishments of the mathematicians associated with it.
What is the significance of the metric tensor in the derivation of the F Weyl formula?
-The metric tensor plays a crucial role in the derivation of the F Weyl formula, as it is used to compute the covariant derivative and other key operations in tensor calculus. The formula ultimately provides a more streamlined way to handle these computations.
Why does the speaker emphasize the aesthetic appeal of the F Weyl formula?
-The speaker emphasizes the aesthetic appeal of the F Weyl formula because it leads to more structured and visually pleasing expressions, reflecting the underlying geometric harmony. This aesthetic quality is often valued in mathematical expressions for its clarity and simplicity.
How does the speaker's personal connection to the F Weyl formula influence the discussion?
-The speaker's personal connection to the F Weyl formula, particularly through meeting Conrad Föss and being inspired by Hermann Weyl's work, adds depth to the discussion. It highlights the formula's historical and mathematical significance, as well as the speaker's appreciation for its elegance.

Outlines

00:00

📚 Introduction to the F-Vile Formula

In this introductory paragraph, the speaker expresses excitement about discussing the F-Vile formula, a significant mathematical concept in geometry and relativity. The formula is named after two mathematicians, Hermann Weyl and Conrad Foss. Weyl, a renowned German geometer, contributed greatly to the field with his book 'Space, Time, Matter', which the speaker highly recommends. Foss, who is still living in Switzerland, was met by the speaker and found to be a wonderful mathematician and person. The speaker emphasizes the elegance of the F-Vile formula, which provides an alternative expression for the divergence of a tensor, an important invariant in differential geometry. The formula's advantage is that it allows for the evaluation of divergences using more primary concepts, potentially bypassing the need for Christoffel symbols, which are more complex to compute.

05:01

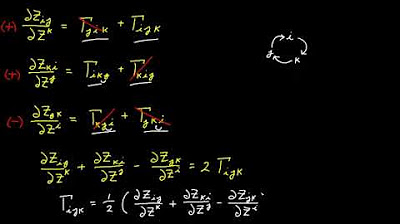
🔍 Derivation and Significance of the F-Vile Formula

This paragraph delves into the derivation of the F-Vile formula, starting with the necessary mathematical ingredients. The speaker outlines the process of applying the product rule to the determinant of the metric tensor, which involves partial derivatives and the use of Christoffel symbols. The approach involves treating the determinant as a composite function of its entries, each dependent on spatial coordinates. The speaker then derives the formula for the derivative of the metric tensor with respect to spatial coordinates, highlighting the use of tensor notation and the Einstein summation convention. The paragraph concludes with the expression for the derivative of the square root of the metric tensor, which is a key step in deriving the F-Vile formula itself.

10:01

📘 The F-Vile Formula and Divergence

The speaker continues the discussion by focusing on the F-Vile formula's application to the divergence of a tensor. The paragraph begins with an analysis of the derivative of the square root of the metric tensor, leading to the formulation of the F-Vile formula. The speaker emphasizes the formula's simplicity and elegance, noting that it allows for the calculation of divergences without the need for Christoffel symbols. The paragraph also touches on the historical aspect of the formula, mentioning that it is primarily recognized in Russian literature, and the speaker's personal encounter with Foss, who was unaware of the formula named after him. The speaker concludes by expressing the importance of naming simple yet profound mathematical concepts after their discoverers as a tribute to their contributions.

15:02

📘 Further Exploration of the F-Vile Formula

In the final paragraph, the speaker hints at further exploration of the F-Vile formula, particularly its application in evaluating divergences and Laplacians of general functions in various coordinate systems. The speaker also mentions plans for future lectures, which will focus on applications of the formula, suggesting that the current lecture is more theoretical and focused on derivations. The speaker expresses a desire to delve deeper into the practical uses of the F-Vile formula and its significance in the broader context of mathematical physics. The paragraph concludes with the speaker's intention to cover additional topics that were not fully addressed in previous lectures, possibly including linear algebra in tensor terms, as a preparation for upcoming subjects.

Mindmap

Keywords

💡Fvile Formula

The Fvile Formula is a mathematical expression that provides an alternative way to calculate the divergence of a tensor field in the context of differential geometry. It is significant in the video as it is the main subject being discussed. The formula is named after two mathematicians, Hermann Weyl and Conrad Foss, and is used to derive the divergence and Laplacian of a scalar field in various coordinate systems, as illustrated by the speaker's intention to show its derivation and applications.

💡Divergence

Divergence is a concept in vector calculus that measures the magnitude of a vector field's source or sink at a given point. In the video, the divergence is an invariant combination derived from the covariant derivative, which is a key step in developing the Fvile Formula. The speaker mentions that the Fvile Formula allows for the evaluation of divergence without the need for computing Christoffel symbols, streamlining the process.

💡Christoffel Symbols

Christoffel symbols are used in differential geometry and general relativity to describe the curvature of a manifold. In the context of the video, they are part of the standard method for calculating the divergence of a tensor field. The speaker notes that while there is nothing particularly difficult about calculating these symbols, especially with modern computer algebra systems, the Fvile Formula offers a more direct approach.

💡Covariant Derivative

The covariant derivative is a generalization of the directional derivative to curved spaces. It is used in the video to construct tensors that remain invariant under coordinate transformations. The speaker explains that applying the covariant derivative to a tensor and then contracting the indices results in an invariant, which is a key concept in the development of the Fvile Formula.

💡Metric Tensor

The metric tensor is a fundamental concept in differential geometry, defining the geometry of a space through the inner product of vectors. In the video, the metric tensor is used in the calculation of the covariant derivative and the determinant, which are essential steps in deriving the Fvile Formula. The speaker discusses the derivative of the metric tensor with respect to spatial coordinates, which is crucial for the formula.

💡Determinant

The determinant is a scalar value that can be computed from the elements of a square matrix, providing important information about the matrix, such as its invertibility. In the video, the determinant of the metric tensor is discussed in the context of deriving the Fvile Formula, with the speaker mentioning the need to calculate its partial derivative with respect to the spatial coordinates.

💡Laplacian

The Laplacian is a differential operator that is widely used in physics and mathematics to describe phenomena such as the diffusion of heat or the flow of electric potential. In the video, the speaker mentions the Laplacian in relation to the Fvile Formula, indicating that the formula can be used to evaluate the Laplacian of a scalar field in various coordinate systems.

💡Spherical Coordinates

Spherical coordinates are a three-dimensional coordinate system that specifies point positions by the distance from a fixed origin, the angle from a reference direction, and the angle from a reference plane. The speaker refers to spherical coordinates as an example of a coordinate system where the Fvile Formula can be applied to evaluate the divergence and Laplacian.

💡Product Rule

The product rule is a fundamental principle in calculus that describes the derivative of a product of two functions. In the video, the speaker applies the product rule to derive the Fvile Formula, emphasizing its utility in simplifying the process of calculating the divergence of a tensor field.

💡Index Notation

Index notation is a method used in tensor analysis to denote the components of tensors and their operations. The speaker uses index notation throughout the video to express the Fvile Formula and related mathematical expressions, highlighting its utility in compactly representing complex relationships in differential geometry.

💡Einstein Summation Convention

The Einstein summation convention is a notational convention used in tensor analysis where an implied summation is assumed over repeated indices. The speaker mentions this convention in the context of deriving the Fvile Formula, noting its efficiency in expressing the relationships between tensors and their derivatives.

Highlights

Introduction of the F vile formula, a significant mathematical expression in geometry and its relation to Hermann V and Conrad Foss.

Hermann V, a renowned geometer of the 20th century, known for his contributions to geometry and the book 'Space, Time, Matter'.

Conrad Foss, a living mathematician based in Switzerland, met with the speaker and discussed the history of the subject.

The F vile formula is named after Foss in Russian literature, highlighting its importance in the field.

The formula provides an alternative expression for the Divergence, an invariant combination in tensor notation.

Advantages of the F vile formula include the ability to evaluate the Divergence without computing Christoffel symbols.

The formula leads to more structured and aesthetically appealing expressions compared to traditional methods.

Connection between the Divergence and the Laplacian, with the F vile formula offering insights into both.

The derivation of the F vile formula involves applying the product rule and understanding the covariant derivative.

Explanation of the metric tensor and its role in the derivation, including its partial derivative and determinant.

Use of the chain rule to find the derivative of the determinant of the metric tensor with respect to spatial coordinates.

The importance of the Einstein summation convention in simplifying tensor calculations.

Derivation of the derivative of the square root of the metric tensor, a crucial step in the F vile formula.

The final derivation of the F vile formula, showing its simplicity and elegance.

Application of the F vile formula in evaluating the Divergence and Laplacian of general functions in various coordinate systems.

Upcoming lectures will focus on the practical applications of the F vile formula and its significance in the field.

The speaker's personal experience and enthusiasm for the subject, highlighting the human aspect of mathematical discovery.

The significance of the F vile formula in the broader context of geometry and its potential impact on future research.

Transcripts

Browse More Related Video

Video 49 - Voss Weyl Formula

Video 30 - Christoffel Symbol Evaluation

Tensor Calculus 14: Gradient explanation + examples

Video 31 - Christoffel Symbol Examples

Calculus 1 Lecture 0.1: Lines, Angle of Inclination, and the Distance Formula

How do you derive the period of oscillation for a pendulum?