Calculus Chapter 1 Lecture 4 Computing Taylor Series
TLDRIn this calculus lecture, Professor Greist explores the computation of Taylor series, introducing efficient methods to derive them, such as substitution and combination. The lecture demonstrates the process using examples like the function 1/x * sin(x)^2 and cos^2(x), leading to the discovery of the hyperbolic sine and cosine functions. These functions, defined in terms of exponentials, are highlighted for their importance and utility, with their Taylor series derived by leveraging known series for exponential functions. The lecture also touches on the relationship between derivatives and Taylor series, illustrating how the former can be understood through the latter, and introduces the concept of higher order terms (HOT) for simplifying series computations.
Takeaways
- 📚 The lesson focuses on computing Taylor series, providing methods for clean and quick computations.
- 🔍 The definition of a Taylor series is revisited, emphasizing the need for effective computation methods.
- 🔄 Substitution and combination methods are highlighted as alternatives to taking multiple derivatives.
- 📈 An example using the function 1/x * sin(x^2) demonstrates the substitution method.
- 🧮 The Taylor series for sin(x^2) is derived by substituting x^2 into the Taylor series for sin(x).
- 💡 The series is then simplified, resulting in terms like x - x^5/3! + x^9/5! etc.
- ✨ Combination methods are illustrated with the example of cos^2(x), showing polynomial multiplication techniques.
- 🔢 Hyperbolic trigonometric functions (cosh and sinh) are introduced, along with their definitions.
- 📊 The Taylor series for hyperbolic functions are computed, showing relationships to their trigonometric counterparts.
- 🔄 Derivatives of hyperbolic functions are explored, demonstrating that the derivative of sinh is cosh and vice versa.
Q & A
What is the main topic of lecture 4 by Professor Greist?
-The main topic of lecture 4 is computing Taylor series, covering methods for clean and quick computations.
What is the definition of a Taylor series?
-A Taylor series is an infinite sum of terms that represents a function as a power series of its derivatives at a single point.
What is an alternative method to computing Taylor series by taking derivatives?
-An alternative method is substitution, where you can plug in a known series into a function to simplify the computation.
How does the substitution method work with the function 1/x * sin^2(x)?
-You use the known Taylor series for sin(x), plug in x^2, and then multiply by 1/x to obtain the series for 1/x * sin^2(x).
What is a method to obtain the full Taylor series for a function?
-One method is to sum the derivatives of the function evaluated at 0, which can be done by properly adding up the coefficients of the x term.
What is the relationship between the Taylor series for cosine squared(x) and sine squared(x)?
-The Taylor series for cosine squared(x) minus sine squared(x) simplifies to 1, which is analogous to the identity for cosine and sine on a unit circle.
What are the definitions of hyperbolic cosine (cosh(x)) and hyperbolic sine (sinh(x))?
-Cosh(x) is defined as (e^x + e^-x) / 2, and sinh(x) is defined as (e^x - e^-x) / 2.
How do the hyperbolic trigonometric functions differ from the standard trigonometric functions?
-Hyperbolic trigonometric functions involve exponentials in their definitions and grow rapidly, unlike the standard trigonometric functions which are periodic.
What is the relationship between cosh squared(x) and sinh squared(x)?
-Cosh squared(x) minus sinh squared(x) equals 1, which is a hyperbolic version of the Pythagorean identity for sine and cosine.
How can you compute the Taylor series for cosh(x)?
-You can compute the Taylor series for cosh(x) by substituting the known series for e^x and e^-x, and then combining terms according to degree, leaving only even powers.
What is the derivative of sinh(x) with respect to x?
-The derivative of sinh(x) with respect to x is cosh(x), which can be seen by differentiating the terms of the Taylor series for sinh(x).
What is the meaning of 'higher order terms' (HOT) in the context of Taylor series?
-Higher order terms (HOT) is an informal way to denote the tail of an infinite series, indicating that the series continues with terms of progressively higher degree.
How can you simplify the computation of a function involving a Taylor series?
-You can simplify the computation by using the HOT notation to ignore terms that are not significant for the desired accuracy, focusing on the relevant terms.
Outlines
📚 Introduction to Computing Taylor Series
Professor Greist begins Lecture 4 by introducing the topic of computing Taylor series. He explains that while the definition of a Taylor series is known, the challenge lies in finding efficient computation methods. The lecture will explore various methods, including substitution and combination, using the example of computing the Taylor series for 1/x * sin(x)^2. The professor demonstrates how using the known Taylor series for sin(x) and simplifying can lead to a cleaner computation. Additionally, he introduces the concept of summation for obtaining the full Taylor series and touches on polynomial multiplication techniques for combining terms.
🔍 Exploring Hyperbolic Trigonometric Functions
The second paragraph delves into the world of hyperbolic trigonometric functions, starting with the definitions of hyperbolic cosine (cosh) and sine (sinh) of x. These functions are characterized by their rapid growth due to the exponential components in their definitions. The professor explains how these functions differ from their circular counterparts and introduces the hyperbolic tangent (tanh). He also discusses the unique identities within hyperbolic trigonometry, such as cosh^2(x) - sinh^2(x) = 1, and hints at the existence of other hyperbolic functions like secant, cosecant, and cotangent. The focus then shifts to deriving the Taylor series for these hyperbolic functions, using the known series for e^x and simplifying the terms to obtain the series for cosh and sinh.
📘 Derivatives and Integrals of Hyperbolic Functions
In this section, Professor Greist discusses the properties of hyperbolic functions, particularly focusing on their derivatives. He shows that the derivative of sinh(x) is cosh(x) and vice versa, which mirrors the relationship between the sine and cosine functions. The professor also introduces the concept of higher-order terms (HOT) as a shorthand for expressing the tail of a Taylor series, which is particularly useful when performing computations. An example is given to illustrate how to simplify the computation of a function involving e^(sin(x)^2) by using the Taylor series for sin(x)^2 and e^x, and then combining terms to obtain a simplified series.
🔑 Conclusion and Preview of Convergence Issues
The final paragraph wraps up the lecture by summarizing the key points covered, including the computation of Taylor series and the introduction of hyperbolic sine and cosine functions. The professor also hints at the next topic for the upcoming lecture, which will address the challenges of convergence in Taylor series. This sets the stage for a deeper exploration of the conditions under which Taylor series converge and the implications for their applications in mathematics.
Mindmap
Keywords
💡Taylor Series
💡Differentiation
💡Substitution
💡Combination
💡Hyperbolic Functions
💡Exponential Function
💡Polynomial Multiplication
💡Trigonometric Functions
💡Convergence
💡Higher Order Terms (HOT)
Highlights
Introduction to lecture 4 on computing Taylor series with Professor Greist.
Definition of Taylor series and the question of the best way to compute them.
Exploration of methods for clean and quick computations of Taylor series.
Use of substitution method with the function 1/x * sin(x^2).
Simplification of Taylor series computation by using known series for sine and x^2.
Derivation of the full Taylor series for the given function using summation.
Introduction of hyperbolic trigonometric functions: cosh(x) and sinh(x).
Explanation of the rapid growth of hyperbolic functions due to their exponential definitions.
Introduction to hyperbolic tangent function and its relationship with cosh and sinh.
Comparison of hyperbolic and trigonometric identities, particularly cosh^2(x) - sinh^2(x) = 1.
Investigation of the Taylor series for hyperbolic cosine using the series for e^x.
Cancellation of odd degree terms in the Taylor series for cosh(x).
Derivation of the Taylor series for sinh(x) by subtracting the series for e^(-x) from e^x.
Identification of the derivative of sinh(x) as cosh(x) from the Taylor series.
Derivation of the derivative of cosh(x) as sinh(x) using Taylor series differentiation.
Introduction of higher order terms (HOT) as a shorthand for expressing tail ends of series.
Application of HOT in simplifying the computation of Taylor series for complex functions.
Conclusion of the lecture with a preview of the next lesson on convergence issues.
Transcripts
Browse More Related Video
Calculus Chapter 1 Lecture 3 Taylor Series
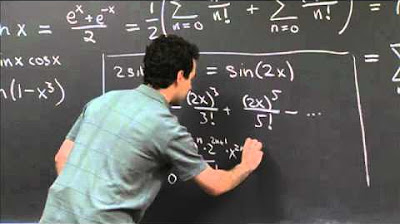
Finding Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010

Calculus Chapter 1 Lecture 5 Convergence
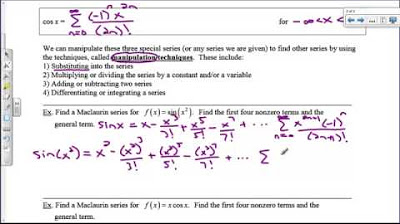
Taylor Series Day1

The Subtle Reason Taylor Series Work | Smooth vs. Analytic Functions

Taylor's Series for sec(x) | MIT 18.01SC Single Variable Calculus, Fall 2010
5.0 / 5 (0 votes)
Thanks for rating: