Parametric Basics BC Calc
TLDRThis video explores parametric equations, polar coordinates, and vectors, focusing on graphing non-linear functions using a third variable (time). It demonstrates how to sketch curves, find slopes, eliminate parameters to derive standard equations, and calculate arc lengths. Examples include graphing a parabola and an ellipse, and calculating derivatives to determine concavity.
Takeaways
- 📚 The lesson covers parametric equations, polar coordinates, and vectors, starting with the basics of parametric equations.
- 📈 Parametric equations are used to graph non-functions, which are curves that do not pass the vertical line test, using two variables X and Y in terms of a third variable, typically time.
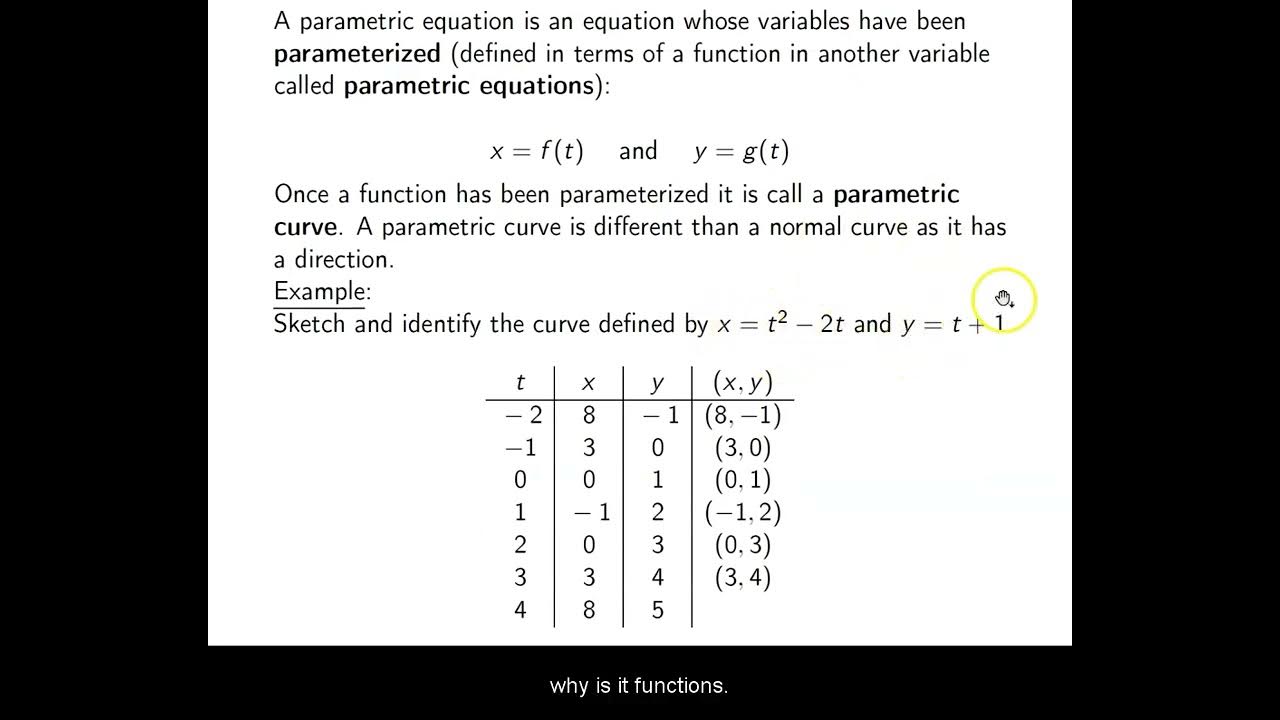
- 📝 An example is provided to illustrate how to sketch a parametric curve using the equations x = t^2 - 4 and y = 12t over the interval -2 to 3 for t.
- 📊 A table is set up with values of t, x, and y to visualize the curve and its direction over time, emphasizing the introduction of time as a third variable in a two-dimensional space.
- 🧩 The concept of eliminating the parameter is introduced to convert parametric equations into a more familiar form, such as finding the equation of a sideways parabola from given parametric forms.
- 🔍 Another example demonstrates how to eliminate the parameter to derive the equation of an ellipse from parametric equations involving sine and cosine functions.
- 📐 The process of finding the slope of a curve at a given point using parametric equations is explained, which involves calculating dy/dt divided by dx/dt.
- 📉 An example calculation shows how to find the slope of the tangent line at a specific point on a curve defined by parametric equations.
- 📚 The second derivative, represented as d²y/dx², is discussed as a way to find the concavity of a curve at a given point using parametric differentiation.
- 📏 The arc length formula for parametric equations is introduced, which involves integrating the square root of the sum of the squares of dy/dt and dx/dt from one parameter value to another.
- 🛣️ An example calculation of arc length is provided to determine the distance traveled along a curve over a specific interval of the parameter.
Q & A
What are parametric equations and why are they used?
-Parametric equations are a way to describe the coordinates of points in a plane using a third variable, typically representing time. They are used to graph curves that do not represent functions in the traditional sense, meaning they fail the vertical line test.
How many variables are typically involved in parametric equations and what do they represent?
-Parametric equations involve three variables. Two of them, usually X and Y, represent the coordinates in the plane, and the third variable, often denoted as 't', represents a parameter, such as time.
Can you provide an example of a parametric equation and how to graph it?
-An example given in the script is x = t^2 - 4 and y = 12t, with 't' ranging from -2 to 3. To graph it, you would create a table of values for 't', calculate corresponding 'x' and 'y' values, and then plot these points to sketch the curve.
What does it mean to 'eliminate the parameter' in parametric equations?
-Eliminating the parameter means finding an equation that does not explicitly contain the parameter 't'. This is done by expressing 't' from one equation and substituting it into the other, resulting in a direct relationship between 'x' and 'y'.
How can you find the slope of a curve represented by parametric equations?
-The slope of a curve at a particular point in parametric form is found by calculating dy/dt divided by dx/dt, where dy/dt is the derivative of the y equation with respect to 't', and dx/dt is the derivative of the x equation with respect to 't'.
What is the significance of the second derivative in parametric equations?
-The second derivative, denoted as d²y/dx², provides information about the concavity of the curve at a particular point. It is calculated by differentiating dy/dx with respect to 't' and then dividing by dx/dt.
How can you find the arc length of a curve represented by parametric equations?
-The arc length is found by integrating from 'a' to 'b' (the 't' values) the square root of (dy/dt)² + (dx/dt)², which represents the integral of the speed of the particle moving along the curve.
What is the relationship between the trigonometric identities and parametric equations?
-Trigonometric identities can be used to eliminate the parameter in parametric equations involving trigonometric functions. For example, using the identity cos²θ + sin²θ = 1, you can derive the equation of an ellipse from parametric equations involving sine and cosine.
Can you provide an example of finding the slope using parametric equations?
-An example given is for the parametric equations x = cos(t) and y = sin(t). The slope dy/dx at a point is found by taking the derivative of y with respect to 't' (cosine) and dividing by the derivative of x with respect to 't' (-sine), resulting in -cotangent(t).
How do you find the second derivative of a curve represented by parametric equations?
-To find the second derivative, you differentiate the expression for the first derivative (dy/dx) with respect to 't' and then divide by dx/dt. This gives you d²y/dx², which can be evaluated at specific 't' values to find the concavity at those points.
What is the purpose of sketching a curve with arrows in parametric equations?
-Sketching a curve with arrows indicates the direction of motion along the curve as the parameter 't' increases. It helps visualize the path and orientation of the curve in the coordinate plane.
Outlines
📚 Introduction to Parametric Equations and Graphing
This paragraph introduces the concept of parametric equations, which are used to graph non-linear functions that do not pass the vertical line test. The instructor explains that parametric equations involve two variables, X and Y, both expressed as functions of a third variable, typically time (T). An example is given where the equations x = t^2 - 4 and y = 12t are used to sketch a curve over a specific interval for T. The process involves setting up a table of values for T, calculating corresponding X and Y values, and sketching the curve with directional arrows to indicate the path taken over time. The paragraph also touches on the concept of eliminating the parameter to convert parametric equations into a more familiar form, using the example equations x = 3cos(T) and y = 4sin(T) to derive the equation of an ellipse.
📈 Calculating Slope and Second Derivatives with Parametric Equations
The second paragraph delves into the process of finding the slope of a curve represented by parametric equations. The slope is found by calculating dy/dt divided by dx/dt, which simplifies due to the cancellation of the differentials with respect to T. An example is provided using the equations x = cos(T) and y = sin(T), resulting in a slope of -cot(T). Another example involves finding the slope at a specific point (2,3) using the equations x = sqrt(T) and y = 1/4 * T^2 - 4, which requires solving for T to ensure the same T value is used for both X and Y. The second derivative, represented as d^2y/dx^2, is also discussed, with an example showing how to find it by differentiating dy/dx with respect to T and then dividing by dx/dt, resulting in a simplified expression involving T.
📏 Understanding Arc Length in Parametric Equations
The final paragraph focuses on the concept of arc length in the context of parametric equations. The arc length formula is presented, which integrates from T=a to T=b the square root of (dy/dt)^2 + (dx/dt)^2. Using the first example from the script, the X equation x = T^2 - 4 is analyzed to find dx/dt and its square, and dy/dt is identified as 12. The formula is then applied to calculate the total distance traveled along the curve, providing a method to quantify the length of a path described by parametric equations. The instructor concludes by summarizing the key points covered in the lesson, including slope, second derivative, and arc length, and indicates that further discussion will continue in the next session.
Mindmap
Keywords
💡Parametric Equations
💡Slope
💡Arc Length
💡Second Derivative
💡Ellipse
💡Tangent Line
💡Direction
💡Non-Function
💡Eliminate the Parameter
💡Trigonometric Functions
Highlights
Introduction to parametric equations and their use in graphing non-functions.
Parametric equations involve two variables, X and Y, typically expressed in terms of a third variable representing time.
Example provided to illustrate sketching a curve using parametric equations x = t^2 - 4 and y = 12t.
Setting up a table of values for t, x, and y to graph the curve from t = -2 to t = 3.
Method of sketching a smooth curve with direction indicated by arrows.
Explanation of eliminating the parameter to convert parametric equations into a more familiar form.
Demonstration of deriving a sideways parabola from parametric equations.
Introduction to another example of eliminating the parameter using trigonometric functions.
Derivation of an ellipse equation from parametric equations involving sine and cosine.
Explanation of how to find the slope of a curve using parametric equations by calculating dy/dt divided by dx/dt.
Example of calculating the slope of the tangent line at a given point using parametric equations.
Method for finding the second derivative using parametric equations by differentiating dy/dx with respect to t.
Calculation of the second derivative at a specific point to determine concavity.
Introduction to the concept of arc length in parametric equations.
Explanation of the arc length formula and its application in calculating the distance traveled along a curve.
Example calculation of arc length using the integral from a to b of the square root of dy/dt squared plus dx/dt squared.
Final summary of the key concepts covered in the lesson on parametric equations, slope, second derivative, and arc length.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: