Parametric Equations

RH Mathematics

8 Feb 202221:17

EducationalLearning

32 Likes 10 Comments

TLDRThis video offers a comprehensive introduction to parametric equations, explaining how they trace out curves in the x-y plane using a time variable. It covers the basics of graphing parametric equations, including the unit circle, and delves into calculus applications, such as finding slopes, acceleration, and identifying vertical/horizontal tangents. The instructor also discusses how to compute the length of a parametric curve and contrasts it with traditional arc length calculations, providing formulas and examples to solidify understanding.

Takeaways

📚 Parametric equations are sets of equations for x and y in terms of an independent variable, often time (t), that trace out a curve in the x-y plane.
🔄 Traditional functions map x to y (y = f(x)), whereas parametric equations map a parameter t to both x and y coordinates (x = f(t), y = g(t)).
🌐 A familiar example of parametric equations is x = cos(t) and y = sin(t), which traces out the unit circle with a counterclockwise orientation.
📈 Parametric equations can be graphed using graphing calculators in parametric mode, but this method is not typically used for problem-solving in AP Calculus.
📉 To find the slope of the tangent line to a parametric curve, calculate dy/dx by dividing dy/dt by dx/dt, which are the derivatives of y and x with respect to t, respectively.
🚀 An example of a vector function representing position is given by x(t) and y(t), and its derivative with respect to t gives the velocity vector.
🔢 The second derivative of y with respect to x for parametric equations is found by differentiating dy/dx with respect to t and then dividing by dx/dt.
📊 The length of a parametric curve can be computed using the integral of the square root of (dx/dt)^2 + (dy/dt)^2 with respect to t, from t1 to t2.
🔍 To find vertical or horizontal tangents on a parametric curve, solve for when dx/dt = 0 (vertical) or dy/dt = 0 (horizontal), ensuring the other derivative is non-zero.
📚 The script also covers how to find the acceleration vector and how to determine the concavity of a parametric curve, which involves understanding second derivatives.

Q & A

What are parametric equations?
-Parametric equations are a set of equations for x and y in terms of an independent variable, often denoted as time 't', which trace out an oriented curve in the x-y plane.
How is the orientation of a parametric curve determined?
-The orientation of a parametric curve is determined by the direction in which the curve is traced as the independent variable 't' increases. For example, the unit circle traced by x = cos(t) and y = sin(t) has a counterclockwise orientation.
How can you graph parametric equations using a calculator?
-You can graph parametric equations using a calculator by switching it to parametric mode and inputting the equations for x and y in terms of 't'. Then, set the appropriate 't' window to view the curve.
What is the relationship between the slope of the tangent line to a parametric curve and the derivatives of x and y with respect to 't'?
-The slope of the tangent line to a parametric curve is given by the derivative of y with respect to 't' (dy/dt) divided by the derivative of x with respect to 't' (dx/dt), which is written as dy/dt over dx/dt.
How can you represent the position of a particle in motion as a vector function?
-The position of a particle in motion can be represented as a vector function where the components of the vector are the x and y coordinates as functions of time 't'.
What is the formula for finding the second derivative of y with respect to x in parametric equations?
-The second derivative of y with respect to x in parametric equations is found by taking the derivative of dy/dx (which is dy/dt over dx/dt) and then dividing by dx/dt again.
How can you determine if a parametric curve has a vertical tangent?
-A parametric curve has a vertical tangent where the derivative of x with respect to 't' (dx/dt) is equal to zero, and the derivative of y with respect to 't' (dy/dt) is non-zero.
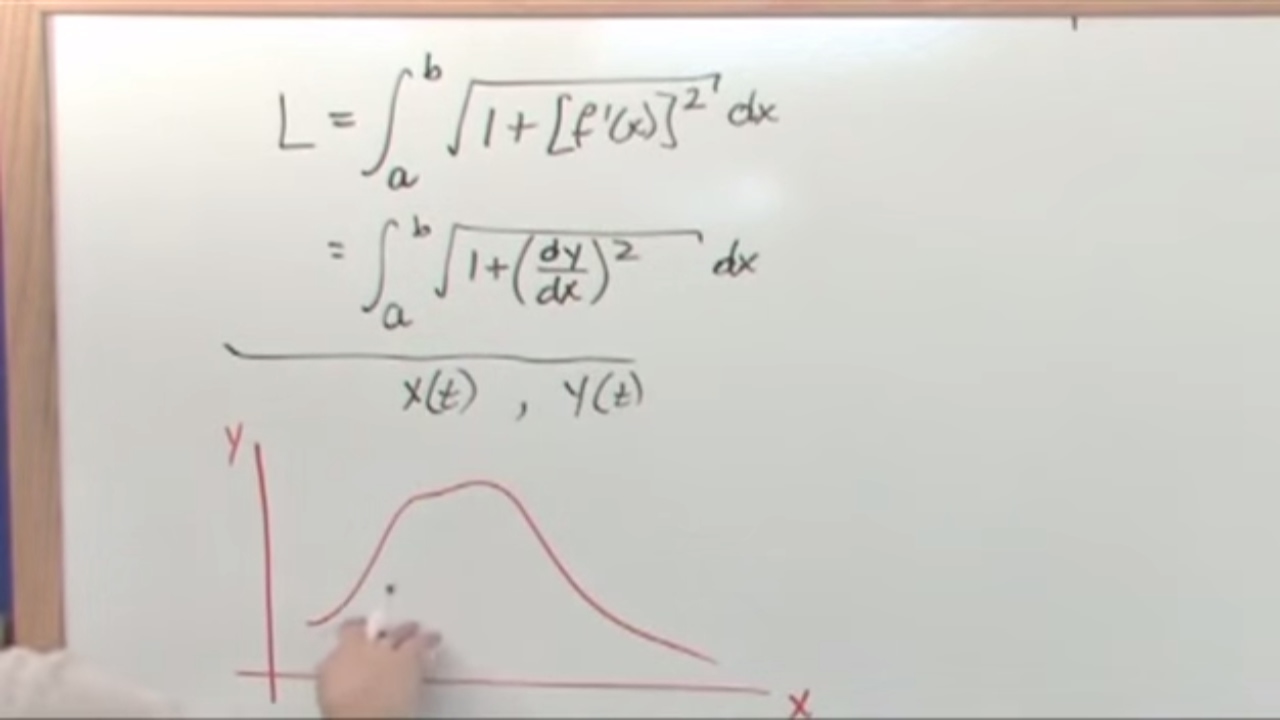
What is the formula for calculating the arc length of a parametric curve?
-The arc length of a parametric curve is calculated by integrating the square root of (dx/dt)^2 + (dy/dt)^2 with respect to 't' from 't1' to 't2'.
How can you find the acceleration of an object given its position as a function of time?
-The acceleration of an object can be found by taking the second derivative of its position vector with respect to time 't', which gives the acceleration vector.
What is the difference between finding the second derivative of y with respect to x in parametric equations and finding the second derivative of a vector function?
-The second derivative of y with respect to x in parametric equations involves taking the derivative of dy/dx and then dividing by dx/dt, whereas the second derivative of a vector function involves taking the derivative of each component of the vector separately and then finding the derivative of the resulting vector.

Outlines

00:00

📚 Introduction to Parametric Equations

This paragraph introduces parametric equations as a method of expressing x and y in terms of a third independent variable, typically time (t). It explains that these equations trace out a curve in the x-y plane and contrasts them with traditional functions where y is a function of x. The unit circle is given as an example of a parametric curve, with x = cos(t) and y = sin(t), and the orientation of the curve is discussed. The paragraph also touches on the use of graphing calculators to visualize parametric equations, but notes that this is not a method for solving problems in AP Calculus.

05:00

📈 Graphing Parametric Equations by Hand and with Technology

The speaker demonstrates how to graph parametric equations both manually and using a calculator set to parametric mode. They emphasize that while graphing is a visual tool, it is not a problem-solving method in AP Calculus. The process of setting up a three-column chart for t, x(t), and y(t) is shown, and an example graph is plotted to illustrate the process. The paragraph concludes with a brief introduction to calculus involving parametric equations, explaining how to find the slope of the tangent line to a curve by dividing dy/dt by dx/dt.

10:00

🚀 Calculus with Parametric Equations: Particle Motion and Vector Functions

This section delves into applying calculus to parametric equations, starting with an example of particle motion. The position of the particle is given as a function of time, and the speaker shows how to express this position as a vector function. The velocity vector is derived by differentiating the position vector with respect to time, and an example calculation of acceleration at a specific time is provided. The concept of the slope of the path, or dy/dx, is also discussed, and the conditions for vertical and horizontal tangents to a curve are explained.

15:03

🔍 Identifying Vertical and Horizontal Tangents in Parametric Curves

The speaker explains how to find points on a parametric curve where the tangent is vertical or horizontal. They describe the mathematical conditions for these scenarios, emphasizing that a vertical tangent occurs where dx/dt is zero (and dy/dt is non-zero), and a horizontal tangent occurs where dy/dt is zero. The paragraph includes an example problem where the function's points of vertical tangency are calculated by setting the derivative of the x-component equal to zero and solving for t.

20:06

📝 Second Derivatives and Arc Length in Parametric Equations

This paragraph discusses the calculation of the second derivative of y with respect to x in parametric equations, which is necessary for analyzing concavity. The process involves differentiating dy/dx with respect to t and then dividing by dx/dt. The speaker contrasts this with finding the second derivative of a function with respect to t. The paragraph concludes with an explanation of how to compute the arc length of a parametric curve, which involves integrating the square root of the sum of the squares of dx/dt and dy/dt from one time value to another.

📏 Computing Arc Length of Parametric Curves

The final paragraph provides a step-by-step guide on how to calculate the arc length of a parametric curve given by a function of t, from time t1 to t2. The process involves finding the derivatives dx/dt and dy/dt, squaring them, adding them together, and then taking the square root. This expression is then integrated over the interval [t1, t2]. An example calculation is provided, showing how to use a calculator to find the arc length, and the result is presented.

Mindmap

Keywords

💡Parametric Equations

Parametric equations are a set of equations that define the coordinates of a point in terms of a third variable, often referred to as the parameter. In the script, this concept is used to describe how the x and y coordinates of a point on a curve can be expressed as functions of a parameter, such as time 't'. The video script explains that these equations allow for the tracing of an oriented curve in the xy-plane, which is central to the theme of the video.

💡Independent Variable

An independent variable is a variable that can be changed or varied freely without being dependent on other variables. In the context of the video, 'time t' serves as the independent variable in parametric equations, with 'x' and 'y' being dependent on it. The script uses the term to illustrate the concept of how the position of a point changes over time.

💡Unit Circle

The unit circle is a circle with a radius of one centered at the origin of a coordinate system. The script mentions the unit circle as an example of a curve that can be traced out using parametric equations, specifically x = cos(t) and y = sin(t), which are fundamental in understanding the orientation and shape of the curve.

💡Graphing

Graphing in the script refers to the process of visually representing the parametric equations on a coordinate plane. The video describes how to use a calculator to graph parametric equations in 'parametric mode', providing a visual understanding of the curves they represent, such as the unit circle.

💡Derivative

A derivative in calculus represents the rate at which a quantity changes with respect to another quantity. The script discusses how to find the derivative of parametric equations, such as dy/dt and dx/dt, which are crucial for understanding the slope of the tangent line to the curve at any given point.

💡Tangent Line

A tangent line is a straight line that touches a curve at a single point without crossing it. The video script explains how to calculate the slope of the tangent line to a curve defined by parametric equations by using the derivatives dy/dx, which is found by dividing dy/dt by dx/dt.

💡Particle Motion

Particle motion refers to the movement of a point or object through space over time. The script uses the concept of particle motion to illustrate how parametric equations can describe the position of an object as a function of time, and how derivatives can be used to find the object's velocity and acceleration.

💡Vector Functions

Vector functions are functions that map a scalar to a vector, representing quantities with both magnitude and direction. In the script, the position of a particle is described as a vector function of time, which is a way to express the object's location in terms of its x and y coordinates as functions of time.

💡Acceleration

Acceleration is the rate of change of velocity with respect to time. The script explains how to find the acceleration of an object moving along a path defined by parametric equations, which is the derivative of the velocity vector, providing insight into the object's changing speed and direction.

💡Arc Length

Arc length is the measure of the distance between two points along a curve. The video script discusses the formula for calculating the arc length of a parametric curve, which involves integrating the square root of the sum of the squares of the derivatives of the parametric equations with respect to the parameter.

💡Concavity

Concavity refers to the curvature of a function, indicating whether a curve bends inwards or outwards. The script explains how to find the second derivative of y with respect to x to determine the concavity of a parametric curve, which is important for understanding the shape and behavior of the curve.

Highlights

Introduction to parametric equations as a set of equations for x and y in terms of an independent variable, often time (t).

Explanation of how parametric equations trace out an oriented curve in the x-y plane.

Comparison between traditional functions and parametric equations in terms of input and output variables.

Demonstration of how to graph parametric equations using a calculator in parametric mode.

The concept of orientation in parametric equations and its relation to the direction of the curve.

Example of the unit circle using parametric equations x = cos(t) and y = sin(t).

Illustration of the process to graph parametric equations by hand using a three-column chart.

Calculus application in parametric equations, specifically finding the slope of the tangent line.

Derivation of the formula for the slope of a tangent line in parametric form, dy/dx = (dy/dt) / (dx/dt).

Particle motion example using parametric equations and the concept of vector functions.

Derivation of velocity and acceleration vectors from parametric equations of position.

Method to find vertical and horizontal tangents on a parametric curve by analyzing dy/dx.

Procedure to determine where a parametric curve has a vertical tangent by setting dx/dt to zero.

Explanation of how to compute the second derivative of y with respect to x in parametric equations.

Clarification on the difference between the second derivative of y with respect to x and the second derivative of a vector function.

Introduction to the formula for computing the length of a parametric curve.

Example calculation of the arc length of a parametric curve using integrals.

Emphasis on the importance of knowing when to use the arc length formula for parametric equations versus traditional functions.

Transcripts

Browse More Related Video

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 4)

Lesson 11 - Arc Length In Parametric Equations (Calculus 2 Tutor)

Calculus Chapter 4 Lecture 35 Arclength 1

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 1)

AP Calculus BC Lesson 9.3

Lec 32 | MIT 18.01 Single Variable Calculus, Fall 2007

Related Tags

Parametric Equations Calculus Graphing Unit Circle Tangent Slope Particle Motion Vector Functions Derivatives Arc Length Educational Video