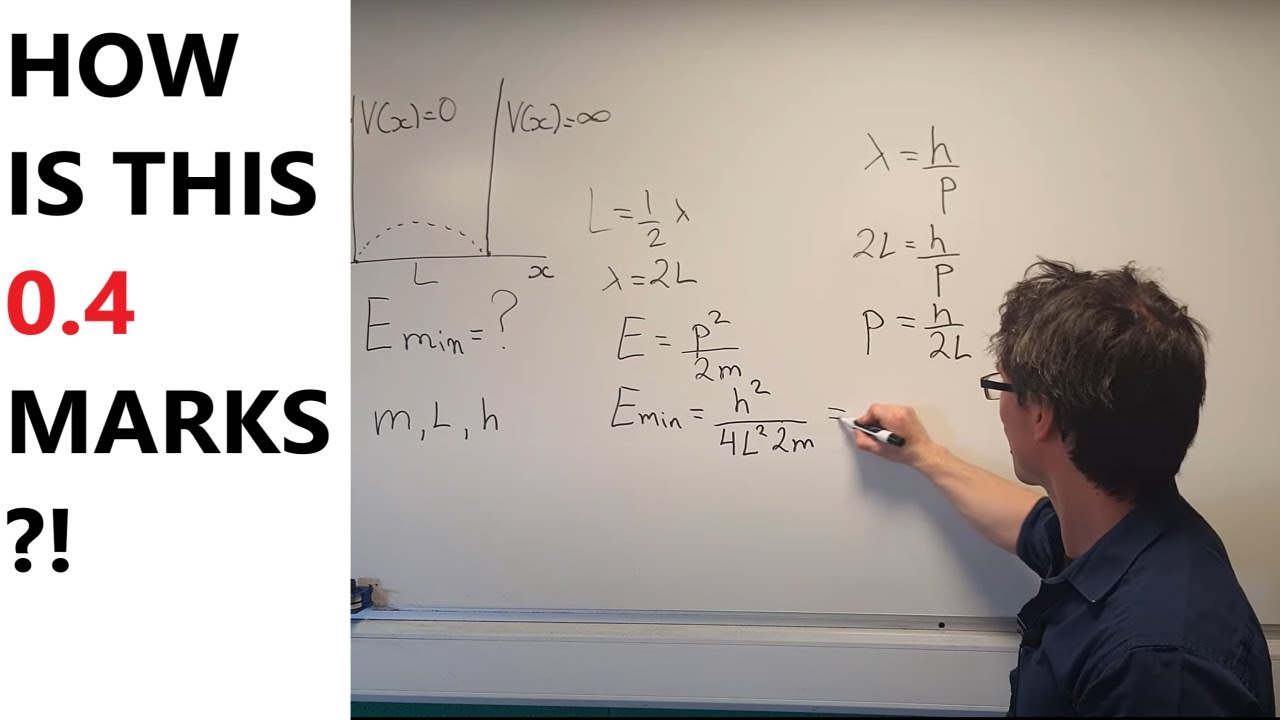Max Planck used DIMENSIONAL ANALYSIS

ZPhysics
2 Mar 202208:39
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains the concept of Planck length, the smallest meaningful length in physics. It derives the Planck length using dimensional analysis of fundamental constants: the speed of light, reduced Planck's constant, and the gravitational constant. By combining these constants, the video arrives at the Planck length of approximately 1.61 ร— 10โปยณโต meters, highlighting its significance as the scale where classical physics breaks down, and quantum gravity takes over. The video also emphasizes the power of dimensional analysis in solving complex physics problems.

Takeaways
  • ๐Ÿ”ฌ The Planck length is the smallest meaningful length in physics.
  • ๐Ÿš€ Constants like the speed of light (c), reduced Planck's constant (โ„), and gravitational constant (G) are essential in deriving the Planck length.
  • ๐Ÿ“ The speed of light (c) has dimensions of length (L) over time (T), denoted as L/T.
  • โš›๏ธ The dimensions of Planck's constant (h) can be derived from the energy equation E = hf, resulting in dimensions of mass (M) times length squared (Lยฒ) divided by time (T).
  • ๐ŸŒ The gravitational constant (G) is derived using Newton's law of gravitation, with dimensions of length cubed (Lยณ) over mass (M) and time squared (Tยฒ).
  • ๐Ÿ”— Max Planck proposed combining these constants to derive a fundamental length, leading to the Planck length.
  • ๐Ÿ“‰ The formula for the Planck length is the square root of โ„ times G divided by cยณ.
  • ๐Ÿงฎ Dimensional analysis confirms that this formula results in the dimension of length.
  • ๐Ÿ“ The calculated Planck length is approximately 1.61 ร— 10โปยณโต meters.
  • ๐ŸŒ€ Lengths smaller than the Planck length are physically meaningless due to 100% uncertainty in particle positions, necessitating a quantum theory of gravity.
Q & A

  • What is the Planck length, and why is it considered significant in physics?

    -The Planck length is approximately 1.61 x 10^-35 meters. It is considered significant because, according to current theories, lengths below this scale do not make physical sense, leading to complete uncertainty in the position of particles. A quantum theory of gravity is required to understand phenomena at this scale.

  • Which constants are used to derive the Planck length?

    -The Planck length is derived using three constants: the speed of light (c), the reduced Planck's constant (ฤง), and the gravitational constant (G).

  • How is the dimensional analysis of the speed of light (c) represented?

    -The dimensional analysis of the speed of light (c) is represented as the dimensions of length (L) divided by the dimensions of time (T), or simply L T^-1.

  • What are the dimensions of Planck's constant (h), and how are they derived?

    -The dimensions of Planck's constant (h) are derived using the energy equation E = hf, where f is the frequency. The dimensions are mass (M), length squared (L^2), and time to the power of minus one (T^-1), written as M L^2 T^-1.

  • How are the dimensions of the gravitational constant (G) determined?

    -The dimensions of the gravitational constant (G) are determined using Newton's law of gravitation. G has the dimensions of length cubed (L^3), time to the power of minus two (T^-2), and mass to the power of minus one (M^-1).

  • What is the significance of canceling out dimensions when deriving the Planck length?

    -Canceling out dimensions helps simplify the expression, leaving only the dimensions of length (L). This confirms that the derived quantity has the correct units, which in this case, correspond to a length.

  • Why is dimensional analysis considered a powerful tool in physics?

    -Dimensional analysis is powerful because it allows physicists to derive relationships between different physical quantities, check the consistency of equations, and even derive new quantities, like the Planck length, from fundamental constants.

  • What does it mean when the script says that below the Planck length, we have '100% uncertainty in the position of particles'?

    -This means that at scales smaller than the Planck length, the uncertainty in the position of particles becomes so large that classical concepts of space and position lose meaning, indicating the need for a quantum theory of gravity.

  • How do the units of mass (M), length (L), and time (T) combine to give the Planck length?

    -The units of mass (M), length (L), and time (T) from the constants ฤง, G, and c combine such that the resulting expression after simplification only has the units of length (L), leading to the Planck length.

  • What would be the next step in understanding physics at scales smaller than the Planck length?

    -The next step would involve developing or utilizing a quantum theory of gravity, as our current theories break down at scales smaller than the Planck length, leading to 'fuzziness' or uncertainty.

Outlines
00:00
๐Ÿ” Deriving the Planck Length from Fundamental Constants

This paragraph introduces the concept of the smallest meaningful length in physics, known as the Planck length. It details the derivation of the Planck length by using three fundamental constants: the speed of light (c), the reduced Planck's constant (โ„), and the gravitational constant (G). The text explains the dimensional analysis of these constants, showing how they can be rearranged to produce a quantity with the dimensions of length. This forms the basis for understanding the Planck length as a fundamental unit of measurement in physics.

05:03
๐Ÿงฎ Dimensional Analysis and Calculation of the Planck Length

This paragraph continues the discussion by calculating the Planck length using the previously derived formula. Through dimensional analysis, the units of the constants are simplified, and the Planck length is computed as approximately 1.61 x 10^-35 meters. The text emphasizes that lengths smaller than this do not make physical sense according to current theories, due to the uncertainty in the position of particles. It concludes by highlighting the importance of dimensional analysis in solving complex physics problems and suggests further learning resources for those interested in physics competitions or exams.

Mindmap
Keywords
๐Ÿ’กPlanck length
The Planck length is the smallest meaningful length in physics, around 1.61 ร— 10^(-35) meters. It represents a scale at which classical concepts of space and time cease to be valid, and quantum gravitational effects dominate. The video explains how this length is derived using fundamental constants like the speed of light, Planck's constant, and the gravitational constant.
๐Ÿ’กDimensional analysis
Dimensional analysis is a mathematical technique used to understand physical quantities by analyzing their dimensions (such as length, time, and mass). In the video, dimensional analysis is used to derive the Planck length by combining the dimensions of fundamental constants. It shows how dimensions can help in identifying meaningful combinations of physical constants.
๐Ÿ’กSpeed of light (c)
The speed of light, denoted by 'c', is a fundamental constant in physics, approximately 3.0 ร— 10^8 meters per second. It represents the maximum speed at which information or matter can travel in the universe. In the video, 'c' is used as part of the calculation to derive the Planck length, emphasizing its role in the interplay between space and time.
๐Ÿ’กPlanck's constant (h)
Planck's constant is a fundamental constant that relates the energy of a photon to its frequency. In the video, the reduced Planck's constant (h-bar), which is Planck's constant divided by 2ฯ€, is used in deriving the Planck length. This constant is central to quantum mechanics, as it sets the scale for quantum effects.
๐Ÿ’กGravitational constant (G)
The gravitational constant, denoted by 'G', is a fundamental constant that appears in Newton's law of universal gravitation. It quantifies the strength of gravity between two masses. In the video, 'G' is combined with other constants to derive the Planck length, highlighting its role in the fundamental description of gravitational interactions.
๐Ÿ’กNewton's law of gravitation
Newton's law of gravitation states that the gravitational force between two masses is proportional to the product of their masses and inversely proportional to the square of the distance between them. The video uses this law to find the dimensions of the gravitational constant 'G', which is crucial in the derivation of the Planck length.
๐Ÿ’กEnergy (E)
Energy, represented by 'E', is a central concept in physics, describing the capacity to do work. In the video, energy is related to Planck's constant and frequency through the equation E = hf, where 'f' is the frequency. This relationship is used to derive the dimensions of Planck's constant, which is then used to calculate the Planck length.
๐Ÿ’กFrequency
Frequency refers to the number of occurrences of a repeating event per unit time, often measured in hertz (Hz). In the video, frequency is linked to energy through Planck's constant in the equation E = hf. Understanding frequency is crucial for determining the dimensions of Planck's constant, which plays a role in deriving the Planck length.
๐Ÿ’กFundamental constants
Fundamental constants are physical quantities that are universally consistent in nature, such as the speed of light, Planck's constant, and the gravitational constant. The video focuses on how these constants can be combined to derive a fundamental unit of length, the Planck length, demonstrating their importance in defining the structure of physical reality.
๐Ÿ’กQuantum gravity
Quantum gravity is a field of theoretical physics that seeks to describe gravity according to the principles of quantum mechanics. The video mentions that below the Planck length, a quantum theory of gravity is needed to understand the behavior of space and time, indicating that current classical and quantum theories break down at this scale.
Highlights

Derivation of Planck length using fundamental constants of physics.

Explanation of the dimensions of speed of light (c) in terms of length and time.

Use of Planck's constant (h-bar) to derive its dimensions involving mass, length, and time.

Introduction of Newton's law of gravitational attraction to find the dimensions of gravitational constant (G).

Calculation of the dimensions of G involving acceleration, length, and mass.

Max Planck's approach to combine constants into a length dimension.

Combination formula for Planck length: square root of h-bar times G divided by c cubed.

Dimensional analysis of the combination formula resulting in length dimension.

Cancellation of terms in the formula to simplify the calculation of Planck length.

Use of approximate values for constants to calculate Planck length in meters.

Result of Planck length calculation: approximately 1.61 x 10^-35 meters.

Implication of Planck length: lengths below this do not make physical sense due to quantum uncertainty.

Necessity of a quantum theory of gravity to understand scales below the Planck length.

Emphasis on the power of dimensional analysis in physics.

Recommendation for those interested in physics to learn dimensional analysis for problem-solving.

Invitation to watch another video on using dimensional analysis for complex physics problems.

Transcripts

Browse More Related Video

Cosmology Lecture 10

A Brief History of Quantum Mechanics (Part 1)

Lecture 5 | String Theory and M-Theory

Don't Miss This on Physics Olympiads (and Oxford PAT)

EASIEST question on the International Physics Olympiad?

Oxford Physics: How light bends around a star, PAT 2021

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Planck LengthPhysicsDimensional AnalysisFundamental ConstantsSpeed of LightQuantum TheoryGravitational ConstantReduced Planck ConstantBoltzmann ConstantEducationalScience