Derivatives of Inverse Functions

Chad Gilliland
20 Nov 201311:17
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the concept of finding derivatives of inverse functions, focusing on the relationship between exponential and logarithmic functions as inverses. It explains the process of determining inverse functions, the importance of domain and range, and the graphical representation of these functions as reflections across the line y=x. The script also explores the derivative of an inverse function, highlighting the reciprocal relationship between the slopes of inverse functions at their intersection points, and provides examples to illustrate the concept.

Takeaways
  • 🔄 Inverse functions, such as the natural log and exponential functions, reflect each other across the line y = x and essentially cancel each other out when composed.
  • 🔍 A function must pass the horizontal line test to have an inverse, meaning it must be one-to-one.
  • 🧩 When finding the inverse of a function, switch x and y and solve for y. Consider the domain and range to ensure correctness.
  • 📈 The domain and range of a function and its inverse swap places. For example, the domain of f(x) becomes the range of f<sup>-1</sup>(x).
  • 🤔 When graphing functions and their inverses, remember that they are reflections over the line y = x. This relationship is crucial for understanding their behavior.
  • 🔀 The slopes of inverse functions are reciprocals at corresponding points. This means if the slope of f(x) at a point is m, the slope of f<sup>-1</sup>(x) at the corresponding point is 1/m.
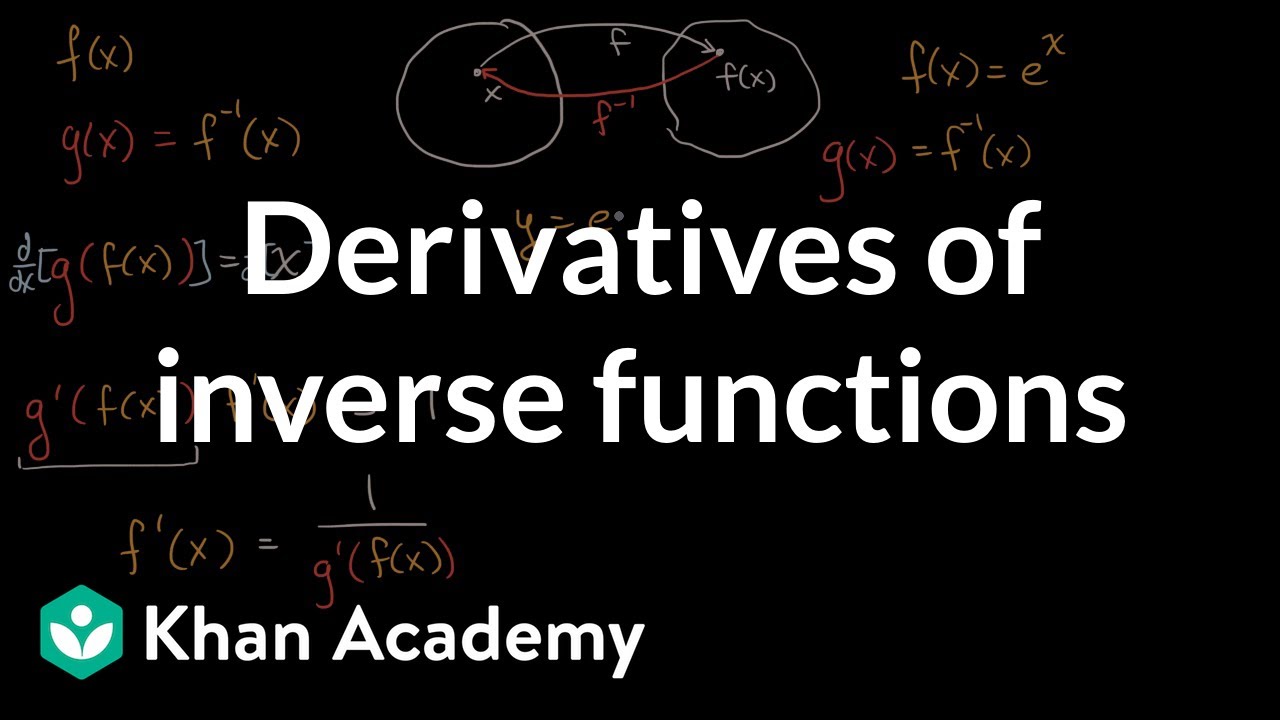
  • 🧮 The formula for the derivative of an inverse function, f<sup>-1</sup>'(a), is 1/f'(f<sup>-1</sup>(a)). This formula helps find slopes at specific points using given data.
  • 💡 To solve problems involving the derivative of an inverse function, use ordered pairs to find the 'flippy-floppy' points, which correspond between f(x) and f<sup>-1</sup>(x).
  • 🎯 Sometimes you need to find missing coordinates by solving the function equation, which helps establish the inverse relationship and slope calculations.
  • 📘 With practice, using these methods and understanding inverse functions in calculus becomes more intuitive, aiding in tackling complex problems efficiently.
Q & A

  • What are we discussing in the video?

    -The video discusses how to find derivatives of inverse functions, focusing on the relationship between exponential and logarithmic functions as inverses of each other.

  • What does it mean for two functions to be inverse functions of each other?

    -Two functions are inverse functions of each other if they are reflections across the line y=x and if you plug one function into the other, you get the original input value, effectively canceling each other out.

  • What is the notation used to denote the inverse of a function f?

    -The inverse of a function f is denoted as f^(-1), which means the inverse function, not 1 over f.

  • What is required for a function to have an inverse?

    -A function must pass the horizontal line test and be one-to-one to have an inverse. If it does have an inverse, it is unique.

  • How do you find the inverse of a function?

    -To find the inverse of a function, you start by rewriting it as y = f(x), then switch x and y, and solve for y to get the inverse function.

  • What is the relationship between the domain and range of a function and its inverse?

    -The domain of the inverse function is the range of the original function, and the range of the inverse function is the domain of the original function. They are 'flippy-floppy'.

  • How does the video demonstrate the relationship between the graphs of a function and its inverse?

    -The video shows that the graphs of a function and its inverse are reflections across the line y=x, not reciprocals.

  • What is a neat property of inverse functions in terms of their derivatives?

    -Inverse functions have reciprocal slopes at the points where their graphs intersect the line y=x, which are the 'flippy-floppy' points.

  • How does the video explain the derivative of the inverse function?

    -The video provides a formula for the derivative of the inverse function, which is the reciprocal of the derivative of the original function evaluated at the corresponding 'flippy-floppy' point.

  • What are some examples given in the video to illustrate the concept of derivatives of inverse functions?

    -Examples include the functions x^3 and the cube root of x, as well as functions involving square roots, cosine, and other operations, demonstrating how to find the derivative of their inverses.

  • What is the importance of practice in understanding the concept of derivatives of inverse functions as presented in the video?

    -Practice is emphasized in the video to help make the process of finding derivatives of inverse functions second nature, as it involves multiple steps and understanding the relationship between functions and their inverses.

Outlines
00:00
📚 Understanding Inverse Functions and Their Properties

This paragraph introduces the concept of inverse functions, specifically focusing on the exponential function e^x and the natural logarithm ln(x). It explains that these functions are inverses of each other, meaning they are reflections across the line y = x. The inverse function is denoted as f^-1, which is not to be confused with 1/f. The paragraph also discusses the conditions for a function to have an inverse, such as passing the horizontal line test and being one-to-one. The process of finding the inverse function is outlined, including rewriting the function in terms of y, switching x and y, and solving for y. Additionally, the relationship between the domain and range of a function and its inverse is explored, emphasizing the need to switch these when finding the inverse.

05:01
📈 Graphing Inverse Functions and Calculating Their Derivatives

This paragraph delves into the graphical representation of functions and their inverses, highlighting that they are reflections across the line y = x. It discusses the importance of understanding the domain and range of both the original function and its inverse when graphing. The paragraph also introduces the concept of calculating derivatives of inverse functions. It provides an example using the functions x^3 and ∛x, showing that the derivative of an inverse function at a point is the reciprocal of the derivative of the original function at the corresponding point. The relationship between the derivatives at these points is further illustrated with examples, emphasizing the reciprocal nature of their slopes.

10:04
🔍 Applying the Derivative Formula for Inverse Functions

This paragraph focuses on applying the formula for the derivative of an inverse function. It explains that the derivative of an inverse function at a point is the reciprocal of the derivative of the original function at the corresponding point. The paragraph provides several examples to demonstrate how to use this formula, including situations where all necessary information is given and others where additional steps are required to find the missing values. It also discusses the process of finding the derivative of an inverse function when the function is not explicitly given, such as in the case of cos(x) and arccos(x). The importance of identifying the correct points and using the reciprocal relationship between the derivatives is emphasized throughout.

Mindmap
Keywords
💡Derivative
A derivative in calculus represents the rate at which a function changes at a certain point. It is a fundamental concept used to analyze the behavior of functions, such as their slope at a given point. In the video, derivatives are discussed in the context of inverse functions, emphasizing the relationship between the derivative of a function and its inverse.
💡Inverse Functions
Inverse functions are a mathematical concept where one function 'undoes' the effect of another. If a function 'f' takes an input and produces an output, its inverse, denoted as 'f^-1', takes that output and returns the original input. The video script discusses finding derivatives of inverse functions and how they are reflections of the original function across the line y=x.
💡Natural Logarithm
The natural logarithm, often denoted as 'ln(x)', is the logarithm to the base 'e', where 'e' is an irrational constant approximately equal to 2.71828. It is used in various fields of mathematics and science. In the script, the natural logarithm is presented as an inverse function to the exponential function 'e^x'.
💡Exponential Function
An exponential function is a mathematical function of the form 'f(x) = a * b^x', where 'a' and 'b' are constants, and 'b' is not equal to zero or one. The video script mentions 'e^x' as an example of an exponential function, which is the inverse of the natural logarithm function.
💡Horizontal Line Test
The horizontal line test is a graphical method used to determine if a function has an inverse. If no horizontal line intersects the graph of the function more than once, the function passes the test and has an inverse. The video explains that not every function has an inverse and must pass this test to be one-to-one.
💡Domain and Range
In the context of functions, the domain refers to the set of all possible input values (x-values), while the range is the set of all possible output values (y-values). The video script discusses how the domain and range of a function and its inverse are related, with the domain of the inverse function being the range of the original function and vice versa.
💡Quadratic Function
A quadratic function is a polynomial function of degree two, standardly written as 'f(x) = ax^2 + bx + c'. The video script uses 'x^2 + 1' as an example of a quadratic function and discusses its graph, which is a parabola, and the importance of considering the domain when finding the inverse function.
💡Reciprocal
A reciprocal is a number which, when multiplied by the original number, results in one. In the context of the video, the reciprocal relationship is used to describe the slopes of inverse functions at corresponding points, where the slope of the inverse function is the reciprocal of the slope of the original function at the point of intersection.
💡Flippy-Floppy Points
This term, used colloquially in the script, refers to the points where the original function and its inverse intersect when graphed on the same coordinate plane. These points are reflections of each other across the line y=x, and the video uses this concept to illustrate the relationship between the derivatives of a function and its inverse at these points.
💡Derivative of an Inverse Function
The derivative of an inverse function is a concept discussed in the script, which involves finding the slope of the inverse function at a given point. The video explains that the derivative of the inverse function at a point is the reciprocal of the derivative of the original function at the corresponding point, which is a key insight in calculus for understanding the behavior of inverse functions.
Highlights

Introduction to the concept of finding derivatives of inverse functions.

Explanation of natural log of X and e to the X as inverse functions.

Geometric interpretation of inverse functions as reflections across the line y=x.

Condition for a function to have an inverse: it must pass the horizontal line test and be one-to-one.

Process of finding the inverse function by switching x and y and solving for the new y.

Importance of considering domain and range when finding inverse functions.

Graphing inverse functions and their relationship as reflections across y=x.

Example of finding the derivative of an inverse function using the relationship between the functions.

Derivation of the relationship between the derivatives of a function and its inverse at corresponding points.

Practical example of calculating the derivative of an inverse function using the formula.

Demonstration of how to find missing information to apply the derivative formula for inverse functions.

Explanation of the process to find the derivative of an inverse function when not all information is given.

Application of the derivative formula for inverse functions with the function G and its inverse.

Finding the derivative of an inverse trigonometric function and its relationship to the original function.

Final thoughts on the importance of practice in mastering the calculation of derivatives of inverse functions.

Transcripts

Browse More Related Video

Calculus 2 Lecture 6.2: Derivatives of Inverse Functions

Finding Inverse Functions (Precalculus - College Algebra 51)

Introduction to Inverse Functions

Introduction to function inverses | Functions and their graphs | Algebra II | Khan Academy

12 - What are Inverse Functions? (Part 1) - Find the Inverse of a Function & Graph

Derivatives of inverse functions | Advanced derivatives | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesInverse FunctionsExponentialLogarithmicCalculusMathematicsPrecalculusFunctionsDomain RangeGraphing