Taylor Polynomials from Taylor's Formula

turksvids
6 Jun 201507:25
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial demystifies the process of writing Taylor polynomials, which might seem daunting at first but are quite manageable. The presenter emphasizes the importance of memorizing the formula for the nth term of a Taylor polynomial, which involves the nth derivative evaluated at a specific center point. Through step-by-step examples, the video illustrates how to derive and simplify Taylor polynomials, including a special case for the Maclaurin polynomial when the center is zero. The key takeaway is the memorization of the formula and practice in taking derivatives and evaluating them at the center point.

Takeaways
  • 📚 The main topic of the video is teaching how to write a Taylor polynomial, which is a polynomial that approximates a function by using the derivatives of the function at a single point.
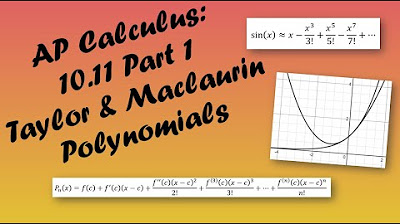
  • 🔍 The nth term of a Taylor polynomial has a specific form: the nth derivative evaluated at a point 'a', multiplied by (x - a) to the power of n, all divided by n factorial.
  • 📝 It's crucial to memorize the formula for the nth term of the Taylor polynomial as it's fundamental to solving problems related to Taylor series.
  • 🎯 If the Taylor polynomial is centered at zero, it is specifically called a Maclaurin polynomial.
  • 📋 The process involves creating a table with three columns for n, the nth derivative, and the evaluation of the nth derivative at the center point.
  • 🔑 The zeroth derivative is the original function, and the subsequent derivatives are calculated using standard differentiation rules.
  • 🔢 The table is used to evaluate each derivative at the center point, which is necessary for constructing the Taylor polynomial.
  • ✍️ The video provides an example of finding a fourth-degree Taylor polynomial centered at 0 for the function f(x) = x * sin(x), demonstrating the step-by-step process.
  • 📉 The video also shows a second example of finding a third-degree Taylor polynomial centered at 3 for the function f(x) = 1/(x + 2), emphasizing the importance of pattern recognition in derivatives.
  • 📝 The importance of simplifying the polynomial after writing out all terms, even if some coefficients are zero, is highlighted to avoid confusion and ensure clarity.
  • 💡 The video concludes by emphasizing the importance of memorizing the formula for the nth term and practicing taking derivatives and evaluating them to overcome the intimidation factor associated with Taylor polynomials.
Q & A

  • What is a Taylor polynomial?

    -A Taylor polynomial is a polynomial used to approximate a function by using the derivatives of the function at a single point, typically denoted as the center.

  • What is the formula for the nth term of a Taylor polynomial?

    -The nth term of a Taylor polynomial is given by \( \frac{f^{(n)}(a)}{n!}(x - a)^n \), where \( f^{(n)}(a) \) is the nth derivative of the function evaluated at the center \( a \).

  • What is the significance of the center 'a' in the Taylor polynomial formula?

    -The center 'a' is the point around which the polynomial is centered. It is the value at which the derivatives are evaluated to approximate the function.

  • What is a Maclaurin polynomial?

    -A Maclaurin polynomial is a special case of a Taylor polynomial where the center 'a' is zero. It is used to approximate functions centered at the origin.

  • How do you find the nth derivative of a function?

    -To find the nth derivative of a function, you repeatedly differentiate the function until you reach the nth derivative, applying differentiation rules such as the power rule, product rule, and chain rule as necessary.

  • What is the purpose of creating a table with three columns when finding a Taylor polynomial?

    -The table helps organize the information needed to construct the Taylor polynomial. It lists the values of n, the nth derivative of the function, and the nth derivative evaluated at the center.

  • Why is it important to memorize the formula for the nth term of a Taylor polynomial?

    -Memorizing the formula is crucial because it is the foundation for constructing the Taylor polynomial. Without it, you would not be able to approximate functions using Taylor series.

  • What is the zeroth derivative of a function?

    -The zeroth derivative of a function is the function itself. It is the starting point for finding higher order derivatives.

  • How do you evaluate the nth derivative at the center 'a'?

    -You substitute the center 'a' into the nth derivative to find its value at that point. This value is then used in the Taylor polynomial formula.

  • Can you provide an example of finding a Taylor polynomial using the method described in the script?

    -Yes, the script provides an example of finding a fourth-degree Taylor polynomial centered at zero for the function \( f(x) = x \sin(x) \). The process involves calculating the derivatives, evaluating them at zero, and using the formula to construct the polynomial.

Outlines
00:00
📚 Introduction to Taylor Polynomials

This paragraph introduces the concept of Taylor polynomials, emphasizing that they may seem intimidating but are manageable once understood. The key formula for the nth term of a Taylor polynomial is presented, which involves the nth derivative of a function evaluated at a specific point 'a', and then scaled by \( \frac{(x - a)^n}{n!} \). The importance of memorizing this formula for solving problems is stressed. An example is given to illustrate the process of finding a fourth-degree Taylor polynomial for a function centered at zero, which is also known as a Maclaurin polynomial. The process involves creating a table of derivatives and evaluating them at the center point, leading to the construction of the polynomial.

05:01
🔍 Constructing Taylor Polynomials with Examples

The second paragraph continues the discussion on Taylor polynomials with a focus on constructing them through examples. The first example involves simplifying the process of finding a Taylor polynomial by rewriting the function with a negative exponent, which streamlines the application of the power and chain rules. The derivatives are calculated and evaluated at the center point, which in this case is 3. The formula for the nth term is then used to construct the polynomial, with simplification steps provided. A second example is briefly mentioned, which involves finding a third-degree Taylor polynomial for a function centered at x=3, demonstrating the process of memorizing the formula, taking derivatives, evaluating them, and following the pattern to construct the polynomial. The paragraph concludes by encouraging memorization of the formula and practice in taking derivatives and evaluations.

Mindmap
Keywords
💡Taylor Polynomial
A Taylor Polynomial is an approximation of a function using a polynomial of degree n, where the coefficients are derived from the derivatives of the original function at a specific point, known as the center. In the video, the Taylor Polynomial is the main focus, with the script explaining how to construct it and its significance in approximating functions, especially evident in the examples provided.
💡nth Term
The nth term of a Taylor Polynomial refers to the specific part of the polynomial that corresponds to the nth derivative of the original function evaluated at the center. The script emphasizes the importance of understanding the nth term's formula, as it is crucial for constructing the Taylor Polynomial correctly.
💡Derivative
A derivative in calculus represents the rate of change of a function with respect to its variable. In the context of the video, derivatives are calculated for the given function to find the coefficients of the Taylor Polynomial. The script demonstrates the process of finding the first few derivatives of the provided functions as part of constructing the Taylor Polynomial.
💡Center
The center in the context of a Taylor Polynomial is the point around which the polynomial is centered. The script mentions that the value of the derivatives at this center is essential for determining the coefficients of the Taylor Polynomial. The concept is used in both examples to show how the polynomial is constructed around the point x=0 and x=3.
💡Factorial
Factorial, denoted by '!', is the product of all positive integers up to a given number. In the script, factorials are used in the formula for the nth term of the Taylor Polynomial, where the coefficient involves dividing by n factorial, illustrating the mathematical structure of the polynomial.
💡Mclaurin Polynomial
A McLaurin Polynomial is a special case of a Taylor Polynomial where the center is set at zero. The script introduces this term and explains that if the center is not specified, it is assumed to be zero, and the polynomial is referred to as a McLaurin Polynomial, as demonstrated in the first example with f(x) = x * sin(x).
💡Table of Derivatives
The script suggests creating a table to organize the derivatives of the function, which is a method to systematically find the coefficients for the Taylor Polynomial. This table includes the nth derivative and its evaluation at the center, which is instrumental in constructing the polynomial.
💡Product Rule
The product rule in calculus is used to find the derivative of a product of two functions. The script mentions the product rule in the context of finding the derivatives of the given function, which involves multiplying the derivative of one part by the other part of the function.
💡Chain Rule
The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. The script implies the use of the chain rule when dealing with functions like sin(x) or cos(x) within the context of the original function, which is necessary for deriving the function's derivatives.
💡Simplification
Simplification in mathematics refers to the process of making an expression more straightforward or easier to understand. The script demonstrates the simplification of the Taylor Polynomial after writing out all terms, including those with zero coefficients, to arrive at the final, more concise form of the polynomial.
💡Pattern Recognition
Pattern recognition is the ability to identify regularities or trends in data. In the script, the presenter notes a pattern in the derivatives, which helps in the process of constructing the Taylor Polynomial. Recognizing such patterns can simplify the process and make it more intuitive.
Highlights

The video discusses how to write a Taylor polynomial, which can be intimidating but is manageable.

The nth term of a Taylor polynomial has a specific form involving the nth derivative evaluated at a center point.

The formula for the nth term is the nth derivative at a times (x - a)^n divided by n factorial.

Memorizing the formula for the nth term is crucial for solving problems involving Taylor polynomials.

An example is given to find the fourth-degree Taylor polynomial centered at x = 0 for the function f(x) = x * sin(x).

Taylor polynomials centered at zero are specifically called Maclaurin polynomials.

A table with three columns (n, nth derivative, and nth derivative evaluated at the center) is used to solve the example.

The zeroth derivative is the original function, and subsequent derivatives are found using calculus rules.

The nth derivatives are evaluated at the center, which in the example is zero.

The formula is used to fill in the Taylor polynomial, starting with the zeroth derivative at the center.

The example simplifies to a polynomial of x^2 - 16x^4, demonstrating the process of filling in the formula.

A second example is provided to find the third-degree Taylor polynomial centered at x = 3 for f(x) = 1/(x + 2).

The function is rewritten with a negative exponent to simplify derivative calculations.

Derivatives are calculated and evaluated at the center, which in the second example is three.

The formula is applied again to write the polynomial, which simplifies to 1/5 - 5/3(x - 3) + 2/25(x - 3)^2 - 6/625(x - 3)^3.

The importance of memorizing the formula and practicing derivative calculations is emphasized for mastering Taylor polynomials.

The video concludes by encouraging viewers to memorize the formula and practice to overcome the intimidation of Taylor polynomials.

Transcripts

Browse More Related Video

AP Calculus BC Lesson 10.11 Part 1

Finding Taylor Series

Worked example: coefficient in Taylor polynomial | Series | AP Calculus BC | Khan Academy

Taylor Polynomials

Taylor polynomial remainder (part 1) | Series | AP Calculus BC | Khan Academy

Taylor Polynomials Day 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Taylor PolynomialsCalculus TutorialDerivative ExamplesMathematics EducationPolynomial ApproximationEducational ContentMath Problem SolvingTrigonometric FunctionsAlgebraic TechniquesVideo Script Summary