Worked example: coefficient in Taylor polynomial | Series | AP Calculus BC | Khan Academy

Khan Academy
17 Dec 201603:53
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script guides viewers through the process of finding the coefficient for a specific term in a Taylor polynomial. Centered at x = -2, the script explains the general form of the Taylor polynomial and emphasizes the importance of evaluating the function and its derivatives at the center point. The focus is on identifying the coefficient of the term containing x plus two to the fourth power. The process involves calculating the fourth derivative of the original function, evaluating it at x = -2, and dividing by four factorial, ultimately simplifying to a coefficient of 60.

Takeaways
  • 🔢 The goal is to find the coefficient of the term containing (x + 2)^4 in the Taylor polynomial centered at x = -2 for the function f(x).
  • 📝 The general form of the Taylor polynomial is established, with each term involving the function and its derivatives evaluated at the center point, x = -2.
  • 🧮 The constant term is derived from the function value at x = -2 divided by 0 factorial.
  • 🧑‍🏫 The linear term involves the first derivative evaluated at x = -2, divided by 1 factorial, and multiplied by (x + 2).
  • 🔢 The quadratic term includes the second derivative at x = -2, divided by 2 factorial, and multiplied by (x + 2)^2.
  • 🧮 The cubic term is derived from the third derivative at x = -2, divided by 3 factorial, and multiplied by (x + 2)^3.
  • 🔍 The term of interest, the fourth-degree term, involves the fourth derivative at x = -2, divided by 4 factorial, and multiplied by (x + 2)^4.
  • 🔢 The coefficient of the term (x + 2)^4 is the fourth derivative of the function evaluated at x = -2 divided by 4 factorial.
  • 🧮 Calculations show the first derivative as 6x^5 - 3x^2 and the second derivative as 30x^4 - 6x.
  • 🔢 The third derivative is 120x^3 - 6, and the fourth derivative is 360x^2.
  • 📉 Evaluating the fourth derivative at x = -2 gives 360 * 4.
  • 🧮 Dividing 360 * 4 by 4 factorial simplifies to 60.
  • ✅ The coefficient for the (x + 2)^4 term is 60.
Q & A

  • What is the general form of the Taylor polynomial centered at x = -2?

    -The general form of the Taylor polynomial p(x) centered at x = -2 is given by p(x) = f(-2) + f'(-2)/1! * (x+2) + f''(-2)/2! * (x+2)^2 + f'''(-2)/3! * (x+2)^3 + f''''(-2)/4! * (x+2)^4 + ...

  • What do we need to find to determine the coefficient for the term containing (x+2)^4?

    -We need to find the fourth derivative of the original function, evaluated at x = -2, and divide it by 4! (four factorial).

  • How is the first derivative of the given function f(x) determined?

    -The first derivative, f'(x), is determined using the power rule: f'(x) = 6x^5 - 3x^2.

  • What is the second derivative of the given function?

    -The second derivative, f''(x), is f''(x) = 30x^4 - 6x.

  • What is the third derivative of the given function?

    -The third derivative, f'''(x), is f'''(x) = 120x^3 - 6.

  • How is the fourth derivative of the function calculated?

    -The fourth derivative, f''''(x), is calculated as f''''(x) = 360x^2.

  • What is the value of the fourth derivative evaluated at x = -2?

    -The value of the fourth derivative evaluated at x = -2 is 360 * 4 = 1440.

  • How do we calculate the coefficient for the (x+2)^4 term?

    -We calculate the coefficient by dividing the value of the fourth derivative at x = -2 (1440) by 4!, which is 1440 / 24.

  • What is the simplified value of 1440 / 4!?

    -The simplified value of 1440 / 4! is 1440 / 24 = 60.

  • What is the final coefficient for the (x+2)^4 term in the Taylor polynomial?

    -The final coefficient for the (x+2)^4 term in the Taylor polynomial is 60.

Outlines
00:00
📚 Introduction to Taylor Polynomials

The script begins with a teacher introducing the concept of Taylor polynomials, specifically focusing on finding the coefficient for a term containing x to the power of four, in the polynomial centered at x equals negative two. The teacher encourages students to try solving the problem independently before guiding them through the process. The explanation starts by outlining the general form of the Taylor polynomial, emphasizing the importance of evaluating the function and its derivatives at the center point, which is x equals negative two in this case.

🔍 Deriving the Taylor Polynomial

The teacher proceeds to explain the process of deriving the Taylor polynomial by writing out the terms of the polynomial, including the zeroth, first, second, and third derivatives evaluated at x equals negative two. The focus is on identifying the term with x to the fourth power, which requires calculating the fourth derivative of the original function. The script provides a step-by-step breakdown of the derivatives, using the power rule to find each successive derivative of the given function.

📘 Calculating the Coefficient

The script then moves on to the calculation of the coefficient for the x to the fourth power term. The teacher demonstrates how to evaluate the fourth derivative at x equals negative two and divide it by four factorial to find the coefficient. The process involves simplifying the expression to find the numerical value of the coefficient, which is shown to be 60 after performing the division and simplification.

Mindmap
Keywords
💡Taylor Polynomial
A Taylor Polynomial is a mathematical expression used to approximate a function by a polynomial of a finite degree. In the video, the focus is on finding the Taylor polynomial centered at x equals negative two for a given function f(x). This is crucial for understanding how the function behaves near a specific point, in this case, x = -2.
💡Coefficient
In the context of polynomials, a coefficient is a numerical factor that multiplies a variable raised to a certain power. The video script discusses finding the coefficient for the term containing x plus two to the fourth power in the Taylor polynomial, which is essential for accurately approximating the function at x = -2.
💡Derivative
A derivative in calculus is a measure of how a function changes as its input changes. The script mentions taking the first, second, third, and fourth derivatives of the original function to construct the Taylor polynomial. These derivatives are evaluated at x = -2 to determine the coefficients of the polynomial.
💡Factorial
Factorial, denoted by n!, is the product of all positive integers less than or equal to n. In the script, factorials are used in the denominators of the terms in the Taylor polynomial formula, indicating the importance of factorials in calculating the coefficients of the polynomial.
💡Power Rule
The Power Rule is a basic differentiation rule that states the derivative of x^n (where n is a constant) is n times x to the power of n-1. The script uses the power rule to find the derivatives of the given function, which is a fundamental step in constructing the Taylor polynomial.
💡Centering
In the context of Taylor polynomials, centering refers to the point around which the polynomial is constructed. The script specifically discusses centering the polynomial at x = -2, which affects how the polynomial terms are evaluated and the coefficients are determined.
💡Fourth Degree Term
A fourth degree term in a polynomial refers to the term with the highest power of x, which in this case is x^4. The script focuses on finding the coefficient of this term in the Taylor polynomial, which is crucial for understanding the function's behavior at the center point.
💡Evaluation
Evaluation in calculus involves substituting a specific value into a function to find its value at that point. The script discusses evaluating the derivatives of the function at x = -2, which is necessary to determine the coefficients of the Taylor polynomial.
💡Polynomial Approximation
Polynomial approximation is a method used to represent a function as a polynomial, which can be easier to work with in certain contexts. The video script is centered around approximating the given function f(x) using a Taylor polynomial, which is a specific type of polynomial approximation.
💡Function Behavior
The behavior of a function refers to how the function changes as its input changes. The script aims to understand the behavior of the function f(x) near x = -2 by constructing a Taylor polynomial. This helps in predicting the function's values in the vicinity of the center point.
💡Differentiation
Differentiation is a process in calculus that involves finding the derivative of a function. The script involves differentiating the original function multiple times to construct the Taylor polynomial, which is a key step in approximating the function near x = -2.
Highlights

Introduction to finding the coefficient of a specific term in the Taylor polynomial centered at x=-2.

General form of the Taylor polynomial p(x) with the given center point.

Explanation of the pattern in the Taylor polynomial coefficients and powers of (x+2).

Focus on finding the coefficient for the term containing x^4 in the Taylor polynomial.

Derivation of the formula for the coefficient of the x^4 term using derivatives.

Calculation of the first derivative of the given function using the power rule.

Derivation of the second derivative and simplification.

Calculation of the third derivative and simplification steps.

Finding the fourth derivative, which is crucial for the coefficient calculation.

Evaluation of the fourth derivative at x=-2 to find the coefficient numerator.

Division of the evaluated fourth derivative by 4! to find the final coefficient.

Simplification of the coefficient calculation resulting in 60.

Emphasis on the importance of correctly evaluating derivatives for Taylor polynomial coefficients.

Illustration of the process to find the coefficient of any term in a Taylor polynomial.

Highlighting the consistency in the pattern of coefficients and powers in the Taylor polynomial.

Demonstration of the step-by-step approach to derive and calculate the coefficients.

Final conclusion with the coefficient for the x^4 term being 60.

Transcripts

Browse More Related Video

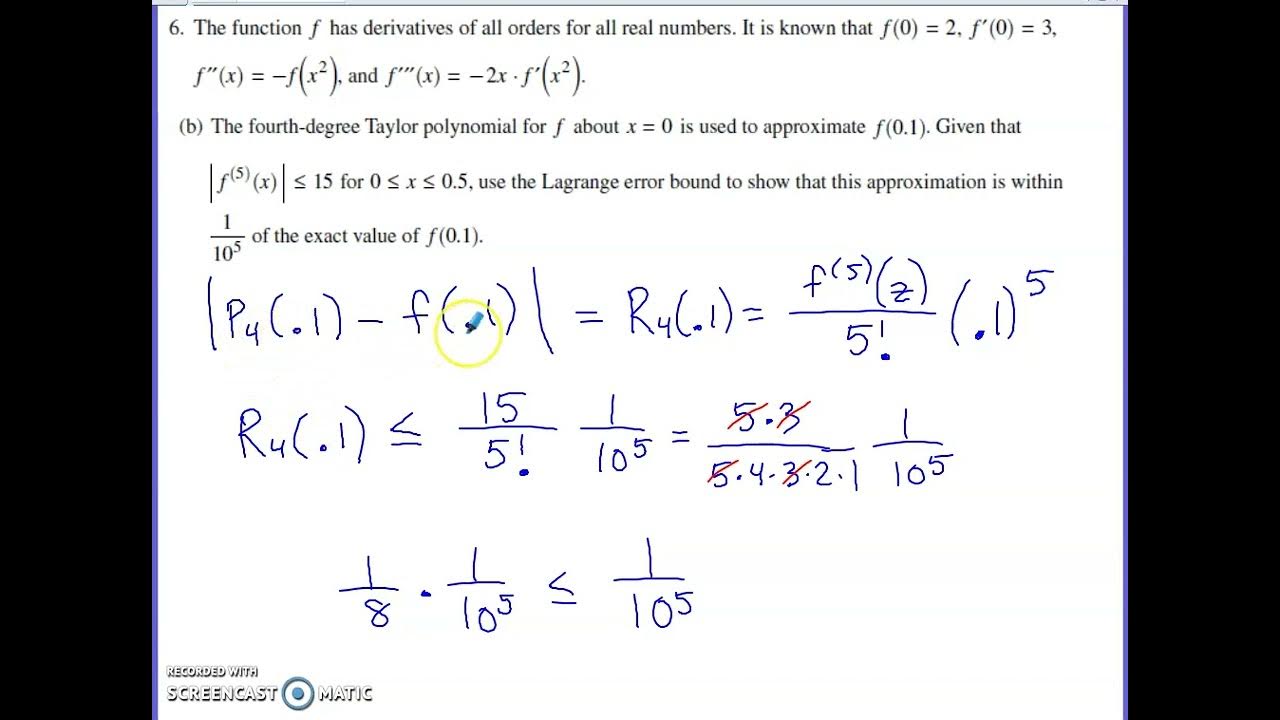
2023 AP Calculus BC Free Response Question #6

2023 AP Calculus BC FRQ #6

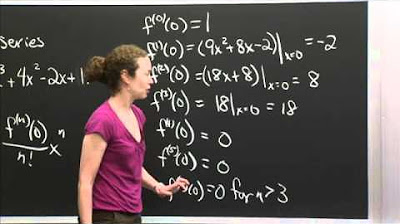
Taylor's Series of a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

Taylor Polynomials from Taylor's Formula

Worked example: coefficient in Maclaurin polynomial | Series | AP Calculus BC | Khan Academy

The Lagrange Error Bound for Taylor Polynomials

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Taylor PolynomialCoefficient CalculationMath EducationDerivative RulesPower RuleFactorial DivisionEducational ContentMathematics TutorialCalculus ConceptsVideo LessonMath Analysis