Integral of a Quotient

RH Mathematics

21 Jan 202218:33

EducationalLearning

32 Likes 10 Comments

TLDRThis educational video tutorial focuses on integral calculus, specifically the integration of quotients. The instructor demonstrates various techniques, such as rewriting expressions, using the power rule, and applying long division and synthetic division for complex fractions. The video also covers the u-substitution method for composite functions and introduces partial fraction decomposition for integrands with factorable denominators. The instructor provides step-by-step examples and encourages viewers to practice these methods, highlighting the importance of recognizing when to apply each technique for effective integration.

Takeaways

📚 The video discusses methods for integrating quotients, emphasizing the importance of recognizing the type of quotient to apply the correct technique.
🔍 When the quotient has a simpler numerator, algebraic manipulation and the power rule can be used to find the anti-derivative.
🌟 For quotients where the numerator is a composition of functions, the 'u-substitution' method is applicable, as demonstrated with the square root of sine theta example.
📉 In cases where the numerator is more complex and cannot be cleanly divided by the denominator, long division or synthetic division is used to simplify the integral.
🔢 Synthetic division is a quick method for dividing polynomials when the divisor is of the form x - a, and it's particularly useful in no-calculator environments.
📝 The script provides a step-by-step guide on how to perform synthetic division to simplify the integral of a complex fraction.
✂️ Long division is necessary when the numerator is larger than the denominator, and it's a precursor to finding the anti-derivative.
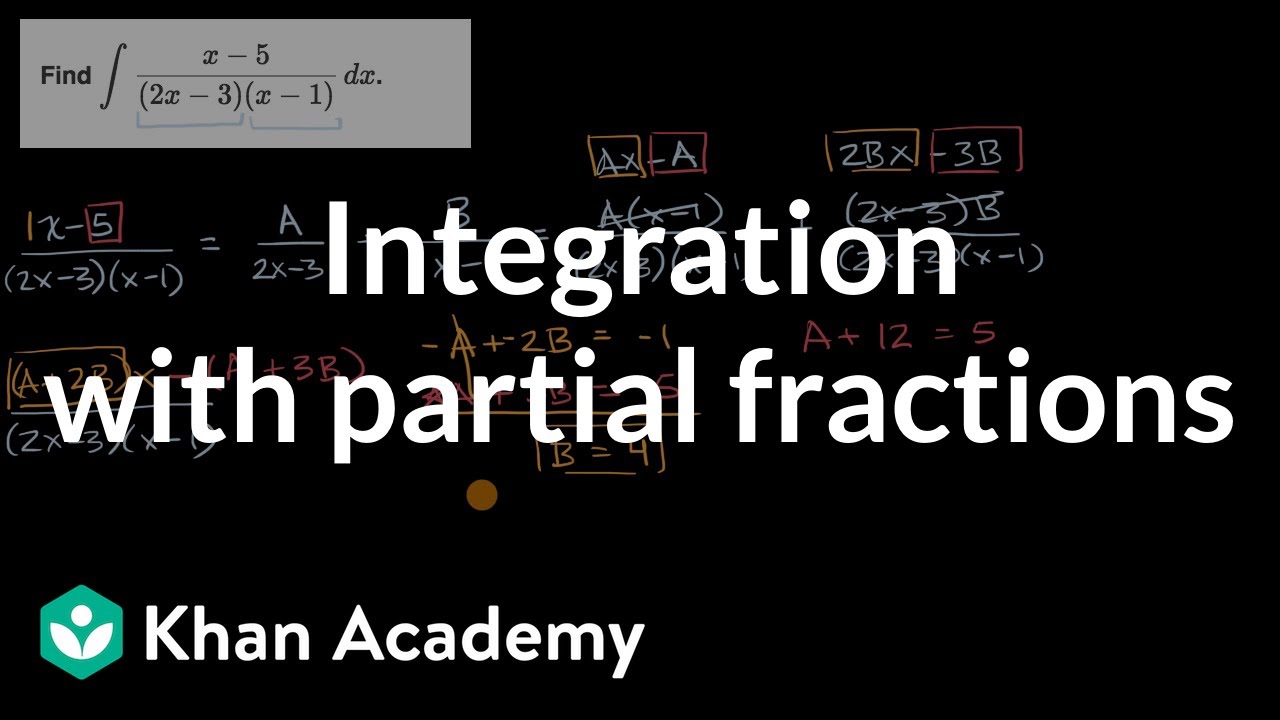
📈 Partial fraction decomposition is introduced as a technique for integrating fractions where the denominator factors into distinct linear factors.
📌 The video demonstrates how to decompose a complex fraction into simpler fractions by finding coefficients a and b, which are then integrated separately.
📚 The importance of recognizing when to apply partial fraction decomposition is highlighted, with the key indicator being a factorable denominator.
📘 The script also reminds viewers of the log properties that were once tested on the AP Calculus exam but are no longer emphasized, providing a brief overview for context.

Q & A

What is the first integral example discussed in the video?
-The first integral example discussed is the integral of 1/e^x, which can be rewritten as e^(-x) and integrated using the chain rule.
How is the integral of 1/x^2 + 4x^(-1) handled in the video?
-The integral is handled by using the power rule after rewriting the expression as x^(-1) + 4x^(-2) and then applying the logarithmic integral formula.
What is the u-substitution pattern used for the third integral example involving the square root of sine theta?
-The u-substitution pattern used is the composition of function pattern, where the derivative of the inside function appears on the outside with multiplication.
Why is long division necessary for the integral of x^4 + x^2 - 3x + 5 divided by x - 2?
-Long division is necessary because the numerator cannot be cleanly divided by the denominator, and the numerator is larger than the denominator, indicating the need for division before integration.
What is synthetic division used for in the video?
-Synthetic division is used as an alternative to long division for dividing polynomials, especially when the divisor is of the form x - a.
How does the video explain the process of partial fraction decomposition?
-The video explains partial fraction decomposition by showing how to break down a complex fraction into simpler fractions with common denominators, using algebraic techniques.
What is the key insight for recognizing when to use partial fraction decomposition?
-The key insight is that the denominator of the fraction factors nicely into two distinct linear factors, indicating the need for partial fraction decomposition.
How does the video handle the integral of 4/(x^2 - 1)?
-The video handles this integral by factoring x^2 - 1 into (x + 1)(x - 1) and then using partial fraction decomposition to break it down into simpler integrals.
What are the properties of logarithms mentioned in the video that are no longer emphasized in AP Calculus?
-The properties mentioned include the sum of logs being the log of the product, the difference of logs being the log of the quotient, and the power property of logs. These properties are no longer emphasized in AP Calculus as they are more algebraic in nature.
How does the video conclude the discussion on integrals?
-The video concludes by emphasizing the importance of recognizing different types of integrals and the techniques needed to solve them, such as algebraic manipulation, long division, and partial fraction decomposition.

Outlines

00:00

📚 Introduction to Integrating Quotients

The video begins with an introduction to the process of integrating quotients, a topic with which the audience is already familiar. The instructor demonstrates how to integrate simple quotients by rewriting expressions and applying the power rule and chain rule. The video also covers the integration of more complex quotients involving compositions of functions and the use of long division and synthetic division when the numerator does not factor cleanly over the denominator. The instructor encourages viewers to pause and reflect on the material before moving on to more advanced concepts.

05:01

🔍 Dealing with Complex Fractions Through Long Division

This paragraph delves into the integration of complex fractions where the numerator is larger than the denominator, necessitating the use of long division. The instructor illustrates how to apply synthetic division to a polynomial divided by a linear term, resulting in a quotient that can be more easily integrated using the power rule. The video emphasizes the importance of recognizing when long division is required before integration and provides a step-by-step guide to performing the division and subsequent integration.

10:02

📈 Partial Fraction Decomposition for Integrable Fractions

The instructor introduces partial fraction decomposition, a technique for integrating fractions with factorable denominators. The process involves breaking down the integrand into simpler fractions that can be individually integrated. The video provides a step-by-step example of how to decompose a fraction and integrate it, highlighting the importance of recognizing factorable denominators and the use of algebraic manipulation to simplify the integration process.

15:04

📘 Applying Logarithmic Properties and Recognizing Integration Techniques

In this section, the instructor discusses the application of logarithmic properties in integration, particularly in the context of AP Calculus exams. The video clarifies that while knowledge of logarithmic properties is useful, it is no longer a focus of recent AP exams. The instructor provides examples of how to integrate functions involving logarithms and emphasizes the importance of recognizing when to use partial fraction decomposition based on the factorability of the denominator. The video concludes with additional examples for practice and a reminder of the importance of knowing derivative and anti-derivative rules.

Mindmap

Keywords

💡Integral

An integral is a concept in calculus representing the area under the curve of a function, and it is the reverse process of differentiation. In the video, the theme revolves around teaching how to calculate integrals of various functions, particularly quotients, which is central to understanding the calculus of antiderivatives.

💡Quotient

A quotient is the result of division, expressed as one number divided by another. In the context of the video, the term refers to a fraction where the numerator is a function and the denominator is another function, and the video discusses methods for integrating such quotients.

💡Anti-derivative

An anti-derivative, or indefinite integral, is a function that represents the reverse operation of differentiation. The video explains how to find anti-derivatives for different types of functions, especially those involving quotients, showcasing the process of integration.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for differentiating composite functions. Although the video's focus is on integration, the chain rule is implicitly mentioned when preparing to integrate functions by rewriting them in a form that simplifies the integration process.

💡Power Rule

The power rule is a basic principle in calculus that allows for the easy differentiation and integration of polynomial functions. In the video, the power rule is used to integrate functions after they have been simplified or decomposed into simpler components.

💡Logarithm

A logarithm is the inverse operation to exponentiation, and it is used in both differentiation and integration. The video mentions the integration of functions that result in logarithmic terms, such as when integrating 1/x, which results in the natural logarithm of the absolute value of x.

💡Long Division

Long division is a method of dividing polynomials, which is relevant in the video when discussing the integration of functions where the numerator is a polynomial of a higher degree than the denominator. The video explains using long division to simplify the integral before finding the anti-derivative.

💡Synthetic Division

Synthetic division is a simplified form of polynomial division used when dividing by linear factors. The video script mentions synthetic division as an alternative to long division when integrating polynomial functions, particularly when the denominator is a simple linear term.

💡Partial Fraction Decomposition

Partial fraction decomposition is a technique used to break down a complex fraction into simpler fractions, which are easier to integrate. The video provides an example of how to use this technique to integrate a function with a factorable denominator, demonstrating its application in calculus.

💡Factorable

A factorable expression is one that can be expressed as the product of its factors. In the video, the term is used to describe denominators that can be broken down into simpler factors, which is a key step in the process of partial fraction decomposition for integration.

💡Log Properties

Log properties refer to the rules governing the manipulation of logarithmic expressions, such as the product, quotient, and power rules. The video mentions these properties in the context of evaluating logarithmic integrals, noting that while they are important, they are not the focus of recent AP calculus exams.

Highlights

Introduction to the method of integrating a quotient by rewriting 1 over e to the x as a negative power of e and applying the chain rule.

Explanation of rewriting complex fractions to simplify the integration process using the power rule.

Demonstration of the integration of a quotient with a logarithmic component, showcasing the use of properties of logarithms.

Introduction of the u-substitution pattern for integrating compositions of functions, like the square root of sine theta.

Presentation of an integral challenge involving a polynomial divided by a linear term, x - 2, and the approach of long division.

Explanation of synthetic division as an alternative to long division for certain types of integrals in AP Calculus.

Illustration of the process of synthetic division with coefficients and handling missing powers in the numerator.

Reveal of the result of synthetic division and the simplification of the integral for easier anti-differentiation.

Discussion on the importance of recognizing when an integral requires long division due to the numerator being larger than the denominator.

Introduction to partial fraction decomposition for integrating fractions with factorable denominators.

Tutorial on breaking down an integrand into separate fractions for easier integration using partial fraction decomposition.

Method of finding coefficients for partial fractions by plugging in convenient x values to simplify the equation.

Integration of a fraction using partial fraction decomposition resulting in a simplified form involving natural logarithms.

Practice example provided for integrating a fraction with a factorable denominator, x squared - 1, using partial fractions.

Explanation of the process for determining coefficients in partial fractions by setting up and solving equations.

Emphasis on the importance of recognizing when to use partial fraction decomposition based on the factorability of the denominator.

Review of log properties relevant to AP Calculus, including sum, difference, and power properties of logarithms.

Clarification that knowledge of log properties is not essential for current AP Calculus exams due to provided answer choices.

Conclusion summarizing the key techniques learned in the video: algebraic manipulation, long division, and partial fraction decomposition.

Presentation of additional examples for practice, including integrals that do not require partial fractions.

Explanation of anti-derivatives for certain functions, such as 1/(1 + x^2) and 5/(1 + x^2), and their application in integrals.

Transcripts

Browse More Related Video

Partial Fraction Decomposition - The Integration

7.5 WebAssign Discussion

Integration with partial fractions | AP Calculus BC | Khan Academy

BusCalc 13.2 Integration by Substitution

BusCalc 13.3 Integration by Parts

Integration Using u-Substitution