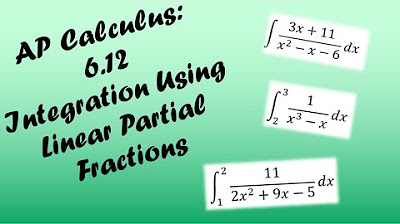
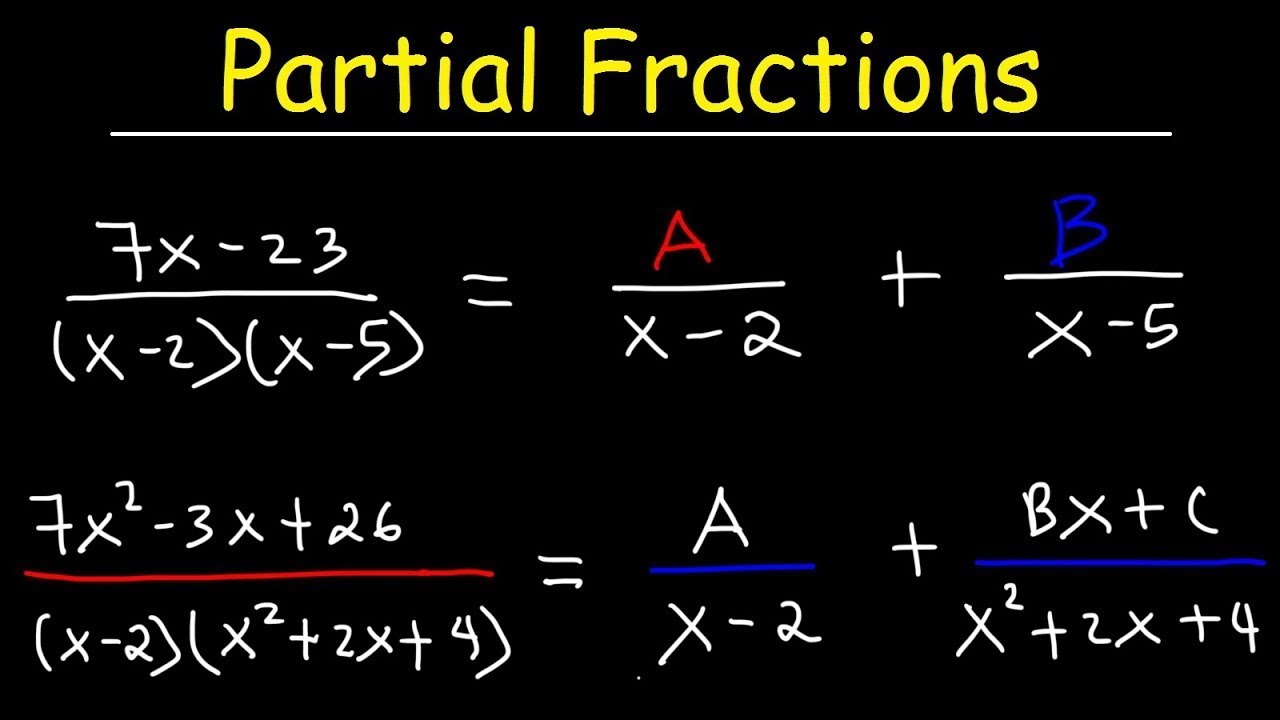
Partial Fraction Decomposition - The Integration
TLDRThis video tutorial delves into the technique of partial fraction decomposition, specifically from an integration perspective. It focuses on integrating rational functions where the numerator's degree is less than the denominator's. The instructor outlines four common integration forms that emerge from such decompositions, providing step-by-step methods for tackling each. The video simplifies the process by demonstrating how to apply these forms to decomposed rational expressions, making the complex task of integration more manageable and intuitive.
Takeaways
- 📚 The video focuses on partial fraction decomposition from an integration perspective rather than algebra.
- 🔍 It discusses the integration of polynomials over polynomials where the numerator's degree is less than the denominator's.
- 📉 The script assumes that the algebraic decomposition of the polynomial ratios has already been completed.
- 🔑 Four main forms of integration arise from partial fraction decomposition, which are key to solving integrals.
- 📌 The first form involves an irreducible quadratic factor with a constant term, which can be integrated using a substitution method.
- 📈 The second form is when there's a linear term over a quadratic denominator, which is straightforward for integration.
- 🔢 The third form deals with a linear factor over a higher degree polynomial, requiring a substitution to simplify the integral.
- 📐 The fourth and most common form is a first-degree factor with a constant term, which is ubiquitous in partial fraction decomposition.
- 🔄 The process involves substituting and simplifying each form to find the antiderivative of the original expression.
- 📝 The script emphasizes the importance of recognizing and applying these forms to the decomposed fractions for integration.
- 🔬 Partial fraction decomposition, despite being complex, is reliable and becomes more intuitive with practice.
Q & A
What is the main topic of the video?
-The main topic of the video is partial fraction decomposition, specifically focusing on its application in integration rather than algebra.
What is the prerequisite for the integration method discussed in the video?
-The prerequisite is that the polynomial in the numerator has a lesser degree than the polynomial in the denominator, and that the partial fraction decomposition has already been algebraically solved for the constants A, B, C, and D.
What are the four common forms that arise during the integration of partial fractions?
-The four common forms are: 1) irreducible quadratic factors with a constant term, 2) linear factors with a quadratic denominator, 3) linear factors with a higher degree term, and 4) degree one factors with a number upstairs and a quadratic term downstairs.
How does the video suggest dealing with an irreducible quadratic factor with just a number upstairs?
-The video suggests factoring out the constant term from the denominator and using a u-substitution where u = x/a, and then integrating 1/(u^2 + 1) with respect to u.
What is the result of integrating 1 over a quadratic expression with a constant term?
-The result is (1/a) * tan^(-1)(u) + C, which simplifies to (1/a) * tan^(-1)(x/a) + C after substituting back for u.
How is the integration of a linear factor with a quadratic denominator approached?
-By letting u equal the quadratic expression, using the derivative to solve for dx, and then integrating 1/u with respect to u.
What is the integral of 1 over a linear factor with a higher degree term?
-The integral is -1/m * (1/(u^2)) + C, which simplifies to -1/m * (1/(mx + b)^2) + C after substituting back for u.
How does the video handle the case of a degree one factor with a number upstairs and a quadratic term downstairs?
-By letting u equal mx + b, using the derivative to solve for dx, and then integrating 1/u with respect to u, resulting in ln|u| + C.
What is the significance of the 'plus C' in the integrals discussed in the video?
-The 'plus C' represents the constant of integration, which is less emphasized in the video because the focus is on the derivative expressions rather than the complete integral form.
Why is partial fraction decomposition considered reliable in the context of integration?
-Partial fraction decomposition is reliable because it consistently results in one of the four forms discussed, allowing for a systematic approach to integration after the algebraic decomposition is done.
Outlines
📚 Introduction to Partial Fraction Decomposition in Integration
The video begins with an introduction to partial fraction decomposition, specifically within the context of integration rather than algebra. It focuses on polynomials where the numerator's degree is less than the denominator's, which is a prerequisite for the method. The script assumes that the algebraic decomposition into simpler fractions has already been completed, and it's time to integrate these fractions. The presenter outlines four common forms that arise during the integration process and intends to demonstrate how to integrate each form individually, as understanding these forms is key to tackling the integration of any decomposed rational function.
🔍 Integration Techniques for Specific Fractional Forms
This paragraph delves into the integration techniques for four specific forms that emerge from partial fraction decomposition. The first form involves an irreducible quadratic factor with a constant term, where the presenter suggests a u-substitution to simplify the integral, resulting in an inverse trigonometric function. The second form features an x term over a quadratic expression, which is also amenable to u-substitution, leading to a natural logarithm function. The third form is characterized by a linear factor over a higher degree polynomial, which is integrated using a direct substitution method, yielding a logarithmic result. The final, most common form, involves a first-degree factor with a constant term, which also uses substitution to result in a natural logarithm. The presenter emphasizes the prevalence of this last form in partial fraction decomposition.
📝 Applying Integration Techniques to Decomposed Functions
The final paragraph illustrates how to apply the previously discussed integration techniques to the decomposed forms of rational functions. The presenter demonstrates how to integrate each term of the partial fraction decomposition by matching them to one of the four forms and then applying the corresponding integration method. This approach simplifies the process by breaking down complex integrals into more manageable parts. The video script provides specific examples of how to handle different cases, including those with linear factors and those that resemble inverse tangent functions. The presenter concludes by emphasizing the reliability of partial fraction decomposition as a method, despite its complexity, due to the consistency of the resulting integral forms.
Mindmap
Keywords
💡Partial Fraction Decomposition
💡Integration
💡Polynomial
💡Decomposition
💡U-Substitution
💡Irreducible
💡Inverse Trigonometric Functions
💡Algebraic Form
💡Derivative
💡Power Rule
Highlights
Introduction to partial fraction decomposition from an integration perspective.
Assumption that polynomials are already decomposed algebraically before integration.
Four common forms that arise during the integration of partial fractions.
Integration of irreducible quadratic factors with a constant term.
Use of u-substitution for integrals involving quadratic terms.
Transformation of integrals into inverse trigonometric functions.
Integration of linear factors with a quadratic denominator.
Application of u-substitution for linear factors in the denominator.
Derivation of the integral form involving natural logarithms.
Integration of linear factors with a higher degree term in the denominator.
Factoring out constants and applying power rules for integration.
Dealing with the most common case of degree one factors with a number upstairs.
U-substitution for linear factors leading to natural logarithm integrals.
Combining the four forms of integrals for partial fraction decomposition.
Practical application of the four forms to specific integral cases.
Detailed walkthrough of integrating a complex rational function.
Emphasis on the reliability of partial fraction decomposition in integration.
Encouragement for familiarity with the method for easier integration.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: