Integration with partial fractions | AP Calculus BC | Khan Academy
TLDRThis educational video script guides viewers through solving an indefinite integral using partial fraction decomposition, a technique recalled from precalculus or algebra classes. It begins with an attempt to identify a suitable substitution method, concluding that the numerator isn't a derivative of the denominator. The instructor demonstrates how to break the integral into simpler rational expressions with known denominators, leading to a system of equations to find unknown constants. The solution process unfolds with step-by-step explanations, culminating in integrating each fraction separately and emphasizing the role of u-substitution, concluding with the natural logarithm function and a constant of integration.
Takeaways
- 📚 The integral problem presented cannot be solved through simple u-substitution as the numerator is not a derivative or a constant multiple of the derivative of the denominator.
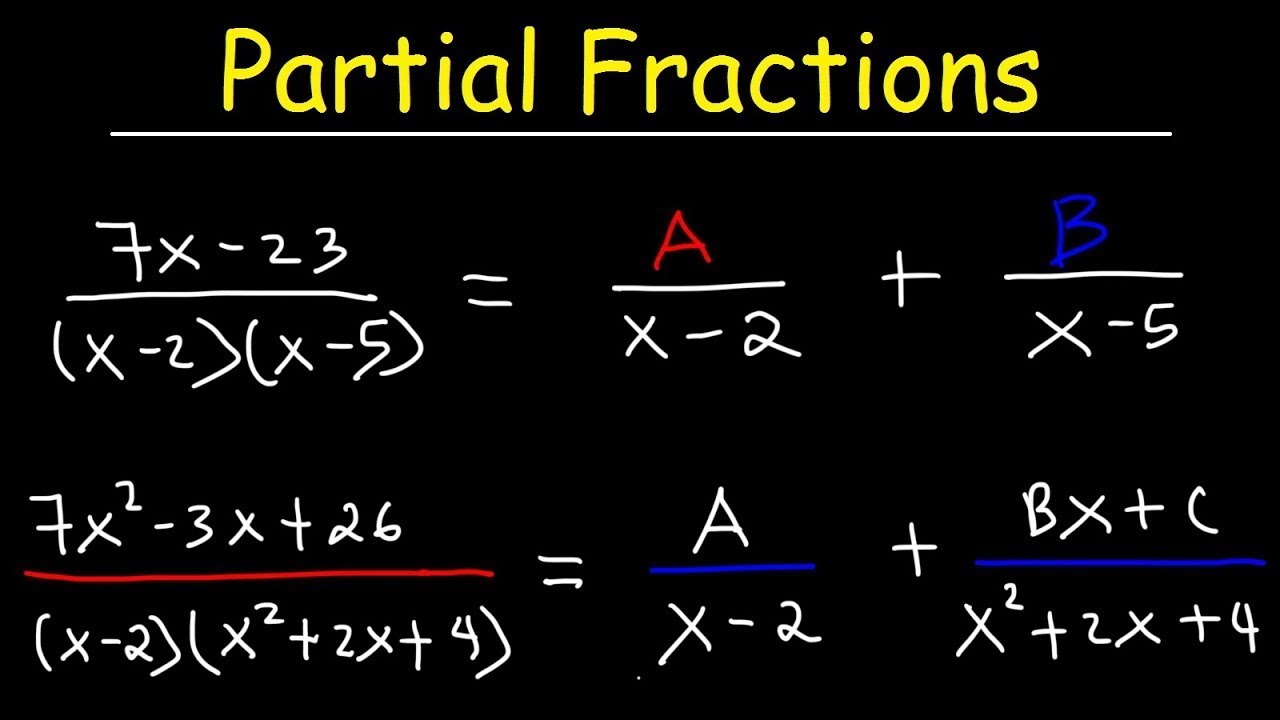
- 🔬 Partial fraction decomposition is recommended as a strategy to break down the given rational expression into simpler fractions, leveraging the fact that the denominator is factorable.
- 📈 The goal of partial fraction decomposition is to express the original fraction as a sum of two fractions with denominators being the factors of the original denominator.
- 📝 Numerators of the fractions in partial fraction decomposition are constants (zero degree) since the denominators are of the first degree.
- 🚡 Solving for the constants in the numerators involves finding a common denominator and equating the resulting expression to the original numerator.
- 🔧 Setting up and solving a system of equations based on the coefficients of the variables allows determination of the constants in the numerators.
- 🛫 Through substitution, the values of the constants are found, enabling the original integral to be expressed as the sum of two simpler integrals.
- 🎲 Simplification strategies, such as pulling constants out of the integral and ensuring the derivative of the denominator is in the numerator, facilitate easier integration.
- 📌 The antiderivatives of the simplified fractions involve natural logarithms, utilizing the fact that the derivative of ln(x) is 1/x.
- 💡 The final solution of the integral is presented as a linear combination of natural logarithms plus a constant of integration, illustrating the application of algebraic manipulation and calculus techniques.
Q & A
What is the main topic of the video?
-The main topic of the video is solving an indefinite integral using partial fraction decomposition.
Why can't u-substitution be directly applied to the given integral?
-U-substitution can't be directly applied because the numerator is not the derivative or a constant multiple of the derivative of the denominator.
What is the hint given by the instructor for solving the integral?
-The hint given by the instructor is to use partial fraction decomposition, which involves breaking up the rational expression into the sum of two simpler rational expressions.
How is the denominator of the given integral factorable?
-The denominator (2x-3)(x-1) is factorable because it can be expressed as the product of two binomials.
What are the general principles for partial fraction decomposition?
-The general principles for partial fraction decomposition include ensuring that the numerator of each term is one degree less than the denominator, resulting in the numerators being constants (A and B) that need to be solved for.
How are the equations for A and B set up in the partial fraction decomposition?
-The equations for A and B are set up by adding the numerators of the decomposed fractions and matching them with the original numerator (x-5). This results in two equations: A + 2B = 1 and A + 3B = 5.
What method is used to solve for the constants A and B?
-The method used to solve for the constants A and B is elimination, by manipulating the two equations obtained from the decomposition.
What are the values of A and B after solving the equations?
-After solving the equations, the values obtained are A = -7 and B = 4.
How is the indefinite integral rewritten after finding A and B?
-The indefinite integral is rewritten as the sum of two simpler integrals: -7/2 times the integral of 1/(2x-3) dx plus 4 times the integral of 1/(x-1) dx.
What antiderivative is used for the term 1/(2x-3)?
-The antiderivative used for the term 1/(2x-3) is the natural logarithm of the absolute value of (2x-3).
What is the final result of the indefinite integral after applying the antiderivative?
-The final result of the indefinite integral is -7/2 times the natural logarithm of the absolute value of (2x-3) plus 4 times the natural logarithm of the absolute value of (x-1), plus a constant (C).
Outlines
📚 Introduction to Partial Fraction Decomposition
The first paragraph introduces the concept of partial fraction decomposition as a technique to solve an indefinite integral. The instructor explains that the method involves breaking down a rational expression into the sum of two simpler rational expressions. The key hint given is that the denominator of the integral is factorable, suggesting the use of this technique. The instructor also advises that the numerators of the resulting expressions will be constants, as the original expression is of the first degree. The goal is to solve for these constants, denoted as A and B, using algebraic methods rather than calculus.
🧠 Solving the Integral Using Partial Fractions
In the second paragraph, the instructor walks through the process of solving the integral using the partial fraction decomposition technique. The steps involve setting up a system of equations based on the pattern that emerges from the numerators of the decomposed fractions. The equations are A+2B=1 and A+3B=5, which are then solved to find that A=-7 and B=4. The integral is then rewritten using these values, and the instructor proceeds to evaluate the resulting simpler integrals, applying the natural logarithm function where appropriate. The explanation concludes with the addition of the constant of integration, C, to complete the solution.
Mindmap
Keywords
💡indefinite integral
💡u-substitution
💡partial fraction decomposition
💡factorable
💡numerator
💡denominator
💡unknown constants
💡system of equations
💡natural log
💡antiderivative
Highlights
Introduction to solving an indefinite integral without direct u-substitution applicability.
Introduction of partial fraction decomposition as a technique for rational expression breakdown.
Highlighting the fact that the denominator of the given expression is factorable.
Setting the stage for expressing the given fraction as a sum of two rational expressions with simpler denominators.
Emphasis on the numerators of the decomposed fractions being constants due to the degree of the denominators.
Instructional guidance to consult additional resources for beginners in partial fraction decomposition.
Strategic approach to finding a common denominator for the expression simplification.
Derivation of a system of equations to solve for the constants in the numerators.
Application of the elimination method to solve for the unknown constants in the decomposed fractions.
Rewriting the original integral into an equivalent expression using identified constants.
Encouragement for self-directed problem solving using newly structured integral expression.
Explanation of integral solving through constant factor extraction and adjustment for u-substitution.
Simplification of the integral into a form that facilitates direct integration.
Conclusion of the integral solving process with natural logarithm functions representing the antiderivatives.
Final presentation of the solved indefinite integral with an emphasis on the inclusion of the constant of integration.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: