Calc Midterm review part 1
TLDRThis video script offers a comprehensive review of calculus concepts, focusing on limits and derivatives. It explains how to determine the left and right limits at specific points, and the implications when they differ. The script also covers the process of finding derivatives using the definition of derivative, including examples with polynomial functions, square roots, and exponential functions. Techniques such as the conjugate method for square roots and the chain rule for composite functions are discussed. The summary of derivatives for various functions, including those involving natural logarithms and quotients, provides a clear understanding of calculus principles.
Takeaways
- 📚 The script discusses review questions for a calculus midterm, focusing on limits and derivatives.
- 🔍 It explains how to determine limits as a function approaches certain values, including from the left and right sides, and the implications when they do not agree.
- 📉 The concept of limits approaching infinity and negative infinity is covered, with examples of how the graph's behavior influences the limit's value.
- 📈 The script provides a step-by-step process for finding the derivative of a function using the definition of a derivative, including expanding and simplifying expressions.
- 🔑 The importance of the limit process in derivatives is highlighted, showing how to take the limit as 'h' approaches zero to find the derivative.
- 🧩 The transcript includes examples of derivatives of polynomial functions, illustrating how terms often cancel out, leaving those with 'h' to simplify.
- 🌱 The process of differentiating functions with radicals involves multiplying by the conjugate to simplify the expression and find the derivative.
- 📐 The chain rule is applied when differentiating composite functions, such as the square root of an expression, and the transcript explains how to work through these steps.
- 📘 The transcript covers the derivative of exponential functions and how to bring down the exponent when applying the chain rule.
- 📌 The use of the quotient rule in differentiation is explained, showing how to handle the derivative of a function divided by another function.
- 📚 The final part of the script discusses the derivative of more complex functions, including those with nested roots and the application of the chain rule in these scenarios.
Q & A
What is the definition of a limit in calculus?
-A limit in calculus is a fundamental concept that describes the behavior of a function as its input approaches a certain value. It is denoted as \( \lim_{x \to a} f(x) \), which means the value that the function f(x) approaches as x gets arbitrarily close to a.
How does the left-hand limit differ from the right-hand limit?
-The left-hand limit is the value that the function approaches as x gets close to a from the left side, denoted as \( \lim_{x \to a^-} f(x) \). The right-hand limit is the value as x gets close to a from the right side, denoted as \( \lim_{x \to a^+} f(x) \). If both exist but are not equal, the overall limit does not exist.
What is the behavior of the function as x approaches 1 from the left?
-As x approaches 1 from the left, the function is described as approaching negative infinity, indicating that the function's value decreases without bound as it gets closer to 1.
What happens when the left-hand limit and right-hand limit of a function at a point do not agree?
-If the left-hand limit and right-hand limit of a function at a point do not agree, it means that the function does not have a limit at that point. The function's values are approaching different values from the left and right sides of the point.
What is the limit of the function as x approaches negative 3?
-The limit as x approaches negative 3 is 1. This is because both the left-hand limit and the right-hand limit are approaching 1, indicating that the function's value is 1 as x gets close to negative 3.
What is the limit of the function as x approaches 3?
-The limit as x approaches 3 is -1. Both the left-hand limit and the right-hand limit are approaching -1, which means the function's value is -1 as x gets close to 3.
How does the function behave as x approaches negative infinity?
-As x approaches negative infinity, the function is described as approaching positive infinity. This indicates that the function's value increases without bound as x moves far to the left on the number line.
What is the limit of the function as x approaches positive infinity?
-The limit as x approaches positive infinity is -2. This suggests that as x becomes very large, the function's value approaches -2, possibly asymptotically.
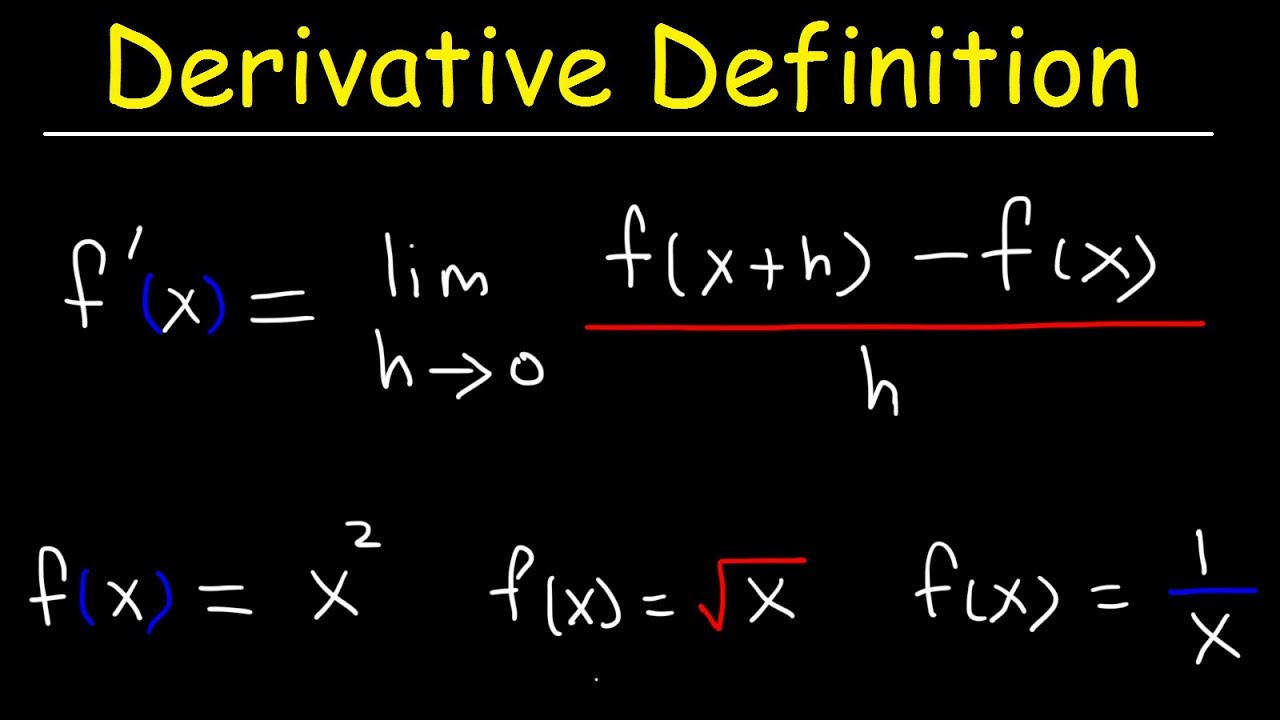
What is the process of finding the derivative of a function using the definition of the derivative?
-The derivative of a function f(x) at a point x is found using the definition of the derivative, which involves the limit \( \lim_{h \to 0} \frac{f(x+h) - f(x)}{h} \). This process involves substituting x+h into the function, expanding, and then simplifying the expression before taking the limit as h approaches 0.
How is the derivative of a function related to the slope of the tangent line to the graph of the function?
-The derivative of a function at a point gives the slope of the tangent line to the graph of the function at that point. It represents the instantaneous rate of change of the function at that specific point.
What is the derivative of the function f(x) = 2x^2 - 8x + 5?
-The derivative of the function f(x) = 2x^2 - 8x + 5 is found by applying the definition of the derivative. After simplifying, the derivative is 4x - 8.
How do you find the derivative of the function f(x) = √(3x + 1)?
-To find the derivative of f(x) = √(3x + 1), you would use the chain rule. The derivative is \( \frac{3}{2} \sqrt{3x + 1} \).
What is the derivative of the function f(x) = (5x + 2x^(1/3) - 3x^(-2))^2?
-The derivative of the function involves converting the cube root and fourth root into exponents, applying the chain rule, and simplifying. The derivative is \( 2 \cdot \frac{2}{3} \cdot (5x^{2/3} + x^{1/3} - 3x^{-5/3}) \).
How do you find the derivative of the function f(x) = sin^2(3x + 1)?
-The derivative of f(x) = sin^2(3x + 1) involves using the chain rule and the double angle formula for sine. The derivative is \( 6 \sin(3x + 1) \cos(3x + 1) \) or equivalently \( 6 \cot(x) \).
What is the derivative of the function f(x) = ln(sin(x))?
-The derivative of f(x) = ln(sin(x)) is found by applying the chain rule. The derivative is \( \cot(x) \).
How do you find the derivative of the function f(x) = (2x + 3)^(1/2)?
-The derivative of f(x) = (2x + 3)^(1/2) is found by applying the chain rule. The derivative is \( \frac{1}{2\sqrt{2x + 3}} \cdot 2 \), which simplifies to \( \frac{1}{\sqrt{2x + 3}} \).
What is the derivative of the function f(x) = (x^2)^(2x)?
-The derivative of f(x) = (x^2)^(2x) involves using the chain rule and the quotient rule. The derivative is \( \frac{2x \cdot e^{2x} - 2 \cdot e^{2x}}{x^3} \).
How do you find the derivative of the function f(x) = (x^3 + 5x)^(3/4)?
-The derivative of f(x) = (x^3 + 5x)^(3/4) involves using the chain rule. The derivative is \( \frac{3}{4} \cdot (x^3 + 5x)^{-1/4} \cdot (3x^2 + 5) \).
Outlines
📚 Calculus Midterm Review: Limits and Derivatives
This paragraph provides a review of limits in calculus, focusing on the behavior of functions as they approach certain points from the left and right. It discusses the concept of limits not existing when the left and right limits disagree, and gives examples with graphical interpretations. The speaker also introduces the topic of derivatives, explaining the process of finding the derivative using the definition of a derivative, including expanding and simplifying expressions to isolate terms involving the change in the variable 'h'.
🔍 Derivative Calculations: Polynomial and Radical Functions
The speaker explains the process of finding the derivative of a polynomial function, demonstrating the steps of expanding the function, simplifying, and then taking the limit as 'h' approaches zero. This is followed by an example involving the derivative of a radical function, where the conjugate is used to simplify the expression. The paragraph concludes with the application of the chain rule in derivatives, providing the final answers for both polynomial and radical functions.
📘 Advanced Derivatives: Exponential, Trigonometric, and Logarithmic Functions
This section delves into the derivatives of more complex functions, including exponential, trigonometric, and logarithmic forms. The speaker converts expressions with roots into exponential form to simplify the process, and then applies the rules of differentiation to find the derivatives. The paragraph covers the use of the chain rule for functions like sine squared and the natural logarithm, as well as the quotient rule for a function involving a quotient of exponentials.
📌 Derivative Applications: Combining Rules and Simplification
The final paragraph continues the theme of derivatives, focusing on the application of various derivative rules to different types of functions. It includes examples of combining cube and fourth roots into a single expression, and then differentiating the resulting function. The speaker also touches on the simplification of derivatives, such as factoring out common terms and using properties of exponents. The paragraph ends with an indication that the review will continue in a later session.
Mindmap
Keywords
💡Limit
💡Derivative
💡Conjugate
💡Chain Rule
💡Quotient Rule
💡Asymptote
💡Difference of Squares
💡Exponent
💡Natural Logarithm
💡Cotangent
Highlights
Introduction to reviewing calculus midterm questions.
Explanation of limits approaching a value from the left and right, and how they determine the existence of a limit.
Discussion on the behavior of a function as X approaches negative infinity and positive infinity.
Use of the definition of derivative to evaluate limits and find derivatives.
Expansion of a function and simplification to find the derivative.
Application of the limit process to find the derivative of a polynomial function.
Derivative of a function involving a square root, using conjugate multiplication.
Explanation of the derivative of a function involving a square root, including the chain rule.
Conversion of a function into numerical exponents to simplify derivative calculations.
Derivative of a function involving a sine squared, using the chain rule and double angle formula.
Derivative of a natural logarithm function, simplifying to cotangent.
Derivative of a function involving a square root, using the chain rule and exponent manipulation.
Use of the quotient rule to find the derivative of a function.
Derivative of a function involving a cube root and fourth root, using the chain rule.
Final summary of the calculus concepts discussed in the review.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: