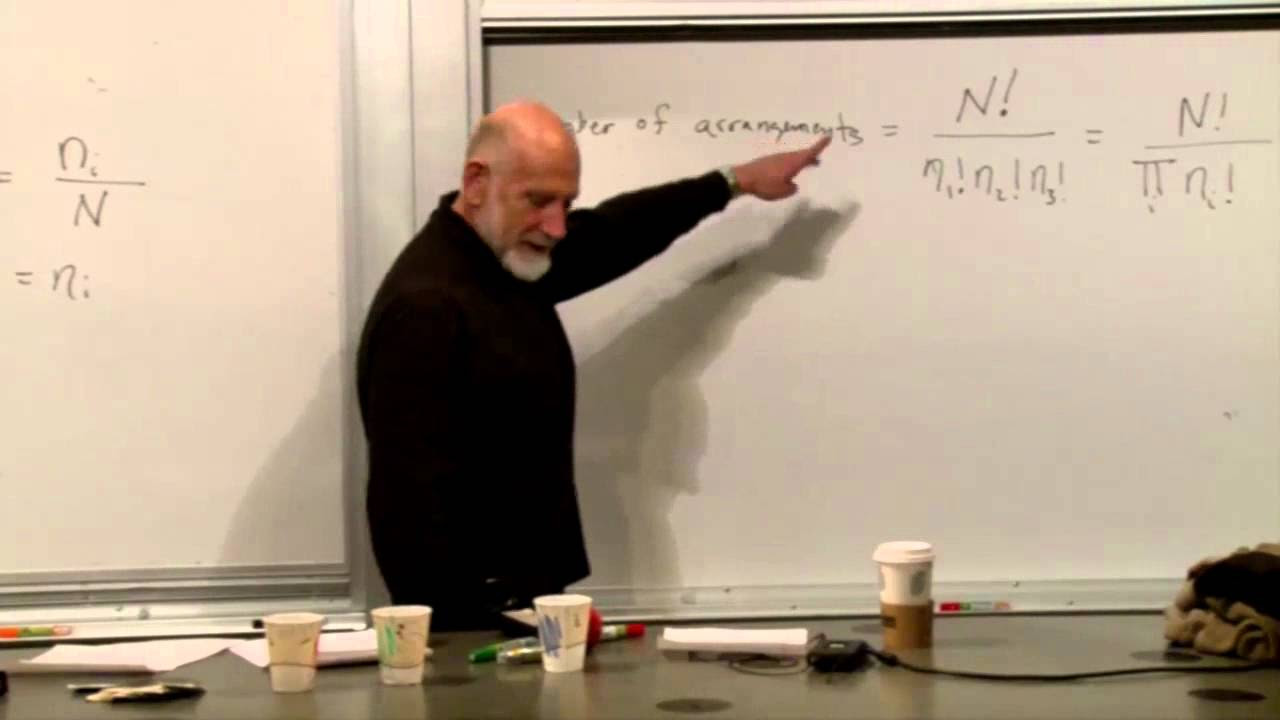Reconciling thermodynamic and state definitions of entropy | Physics | Khan Academy
TLDRThis script delves into the concept of entropy in thermodynamics, using a thought experiment involving gas particles in a container. It explains how the number of possible states for a system (microstates) relates to entropy, demonstrating that increasing the volume available to particles (by removing a wall) leads to an increase in entropy. The video connects this statistical mechanics perspective with the thermodynamic definition of entropy as heat added to a system divided by temperature. The script concludes by highlighting the equivalence of these definitions and the profound implications for understanding the second law of thermodynamics.
Takeaways
- 🌟 The video introduces the concept of entropy in thermodynamics, explaining it through both macroscopic and microscopic perspectives.
- 🔍 It starts by discussing a container with gas particles, emphasizing how each particle can be in various states, defined by its position and velocity.
- 📚 The total number of configurations for the system is calculated as x^n, where x is the number of states each particle can be in and n is the number of particles.
- 🧩 The concept of a 'state variable' is introduced, denoted as s for states, which is a macroscopic variable indicating the number of possible states a system can be in.
- 🔄 The video demonstrates how removing a wall between two containers doubles the volume and thus the number of states each particle can occupy, leading to an increase in entropy.
- 🔢 Entropy is defined as a logarithmic function of the number of states, using a constant k to scale the units, represented as k ln(x^n).
- ⚖️ The change in entropy (Δs) is calculated when the wall is removed, showing that the entropy increases as the system can now be in more states.
- 🔥 The video connects the statistical definition of entropy with the thermodynamic definition, showing that both lead to the same result for the change in entropy.
- 🔧 An isothermal process is used to illustrate how the work done by the system and the heat added to the system can be related to the change in entropy.
- 🔗 The final takeaway is that the thermodynamic definition of entropy as heat added divided by temperature is equivalent to the statistical definition involving the natural log of the number of states, when using Boltzmann's constant.
- 🌐 The video concludes by emphasizing that entropy is about the number of states a system can be in, not just disorder, and that a system with more potential states has higher entropy.
Q & A
What is the significance of the number of states (x) in the context of gas particles in a container?
-The number of states (x) represents the different positions and velocities that each gas particle can have. It is a measure of the possible configurations of the particle within the container, which is crucial for understanding the system's thermodynamic properties.
How does the total number of configurations for a system of n particles, each with x states, relate to the concept of combinatorics?
-The total number of configurations for the system is determined by multiplying the number of states (x) for each particle. This is a fundamental principle of combinatorics, where the total arrangements of a set of items (in this case, particle states) is the product of the number of choices for each item.
What is the macrostate variable 's' and why is it introduced in the script?
-The macrostate variable 's' is introduced to represent the number of states that the system can be in. It is a way to quantify the system's potential configurations, which is essential for understanding its thermodynamic behavior.
Why is the logarithm used to define the state variable 's' in terms of the number of states?
-The logarithm is used to define the state variable 's' because the number of states can grow extremely large. Taking the logarithm helps manage the scale and provides a more manageable measure that can be more easily related to other thermodynamic properties.
What happens when the wall between two containers is removed, and how does this affect the system's volume and pressure?
-When the wall is removed, the gas particles from one container can now occupy the volume of both containers. This doubles the volume, and as a result, the pressure decreases due to the increased space available for the particles to move.
Why does the temperature remain constant when the wall between two containers is removed?
-The temperature remains constant because no work is done by the particles to expand the volume. The wall is simply removed, and the particles continue their motion without expending kinetic energy, thus maintaining the average kinetic energy and hence the temperature.
How does the change in entropy (Δs) relate to the number of states when the wall is removed?
-The change in entropy (Δs) is calculated by comparing the final and initial state variables 's'. When the wall is removed, the number of states (2x^n) increases, leading to a positive change in entropy, indicating that the system can now be in more configurations.
What is the significance of the natural log of 2 in the calculation of the change in entropy when the wall is removed?
-The natural log of 2 is significant because it represents the factor by which the number of states increases when the volume is doubled. This increase in states directly translates to an increase in entropy, as measured by the natural log of the ratio of the final to initial number of states.
How does the script relate the statistical mechanics approach to the thermodynamic definition of entropy?
-The script shows that the change in entropy calculated from a statistical mechanics perspective (as a constant times the natural log of the number of states) matches the thermodynamic definition (heat added divided by temperature). This equivalence suggests that entropy can be understood both as a measure of heat transfer and as a measure of the system's state diversity.
What is the implication of the equivalence between the statistical mechanics and thermodynamic definitions of entropy?
-The equivalence implies that entropy can be universally understood as a measure of the system's potential states, regardless of the specific process or path taken. This unifies the concept of entropy across different thermodynamic processes and provides a deeper understanding of its role in the second law of thermodynamics.
Outlines
🌌 Introduction to Gas Particles and States
The script begins by setting the stage for a discussion on the behavior of gas particles within a container. It introduces the concept of particles existing in various states, defined by their position and velocity. The video aims to explain the mathematical representation of these states, emphasizing the combinatorial explosion of possible configurations as the number of particles increases. The focus is on understanding the fundamental principles that will lead to a surprising revelation about thermodynamics and state variables.
🔍 Expanding on Configurations and Macrostates
This paragraph delves deeper into the concept of configurations, explaining how the number of possible states for a system of particles (n), each with x potential states, is calculated by raising x to the power of n. It introduces the idea of a macrostate variable 's', representing the number of accessible microstates, and proposes taking the logarithm of this number to create a state variable that can be used to compare systems of varying complexity. The paragraph concludes with a thought experiment involving the removal of a wall between two containers, setting up a scenario that will be explored in subsequent paragraphs.
💥 The Instantaneous Expansion and its Effects
The script describes a thought experiment where a wall between two containers is removed, causing an instantaneous change in the system. It discusses the immediate effects on pressure and volume, noting that these variables are in flux as particles redistribute themselves in the new, larger volume. The temperature remains constant because the process is adiabatic, with no work done by the particles. The focus then shifts to recalculating the number of accessible states for the system after the expansion, leading to the realization that the system can now exist in twice as many states as before.
📈 Entropy Change and the Concept of Macrostates
This paragraph introduces the concept of entropy change, defined as the difference between the final and initial entropy values of a system. It uses the previous thought experiment to calculate the change in entropy when the wall is removed, showing that the entropy increases as the system can now occupy more states. The increase in entropy is quantified by the natural logarithm of the ratio of final to initial states, which in this case is 2^N, where N is the number of particles. The paragraph concludes by defining entropy as a measure of the number of accessible states in a system.
🔗 Relating Statistical Mechanics to Thermodynamics
The script explores the connection between the statistical mechanics approach to entropy and the thermodynamic definition, which relates entropy change to heat transfer and temperature. It uses a hypothetical isothermal process to illustrate how the work done by the system during an expansion can be equated to the heat added, leading to an expression for entropy change that mirrors the statistical definition. The paragraph highlights the equivalence of the two definitions of entropy and emphasizes the profound implications of this correspondence for understanding the second law of thermodynamics.
🔄 Entropy, Microstates, and the Second Law of Thermodynamics
The final paragraph synthesizes the concepts introduced throughout the script, reinforcing the idea that entropy is a measure of the number of microstates available to a system. It clarifies misconceptions about entropy as disorder, emphasizing instead that entropy is about the potential states of a system. The script concludes by setting the stage for further exploration of the second law of thermodynamics using the newly established understanding of entropy, hinting at the profound implications of these concepts for understanding the directionality of natural processes.
Mindmap
Keywords
💡Gas Particles
💡States
💡Combinatorics
💡Macrostate Variable
💡Logarithm
💡Adiabatic Process
💡Entropy
💡Boltzmann's Constant
💡Isothermal Process
💡PV Diagram
Highlights
Introduction to the concept of gas particles in a container and their states of motion, which create pressure.
Explanation of the term 'state' in relation to the position and velocity of individual gas particles.
The combinatorial calculation of total configurations for a system of gas particles.
Illustration of how increasing the number of particles increases the total configurations exponentially.
Introduction of a state variable 's' to represent the number of potential states a system can be in.
Use of logarithms to define the state variable 's' and its relation to the number of states.
The effect of removing a wall between two containers on the system's pressure, volume, and temperature.
The constant temperature in the system after the wall is removed, indicating no work done by the particles.
Calculation of the new number of states after the wall is removed, leading to an increase in entropy.
Definition of entropy as a measure of the number of states a system can occupy, and its increase upon system expansion.
Relating the change in entropy to the heat added to the system in an isothermal process.
Derivation of the work done by the system during an isothermal expansion using PV diagrams.
Equivalence of the thermodynamic definition of entropy with the statistical mechanics definition.
Demonstration that the change in entropy is a state function, independent of the process path.
The profound connection between the macroscopic and microscopic views of entropy, and its implications for the second law of thermodynamics.
Clarification of the misconception about entropy as disorder, emphasizing its true meaning as the number of accessible states.
Final thoughts on the significance of the entropy concept in understanding the second law of thermodynamics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: