Gas Stoichiometry: Equations Part 1

Tyler DeWitt
2 Dec 201109:42
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a step-by-step guide to solving a chemistry problem involving the combustion of natural gas, specifically methane (CH4). It begins with converting 58.2 liters of CH4 at standard temperature and pressure (STP) into moles, using the 22.4-liters-per-mole rule. Next, it calculates the moles of water (H2O) produced from the combustion of methane, based on the balanced chemical equation. Finally, it converts moles of H2O into grams using the molar mass of water (18 grams/mole). The script emphasizes the importance of using the 22.4-liters-per-mole rule only under STP conditions and provides a clear roadmap for solving stoichiometry problems in chemistry.

Takeaways
  • 🔍 The script discusses a chemistry problem involving the combustion of methane (CH4), a primary component of natural gas.
  • 📚 The problem requires calculating the amount of water (H2O) produced from a given volume of methane at standard temperature and pressure (STP).
  • 🔢 The chemical equation provided is CH4 + O2 → CO2 + 2H2O, indicating that one mole of methane produces two moles of water and carbon dioxide.
  • 📉 The problem assumes an excess of oxygen, meaning there is more than enough oxygen to react with the methane.
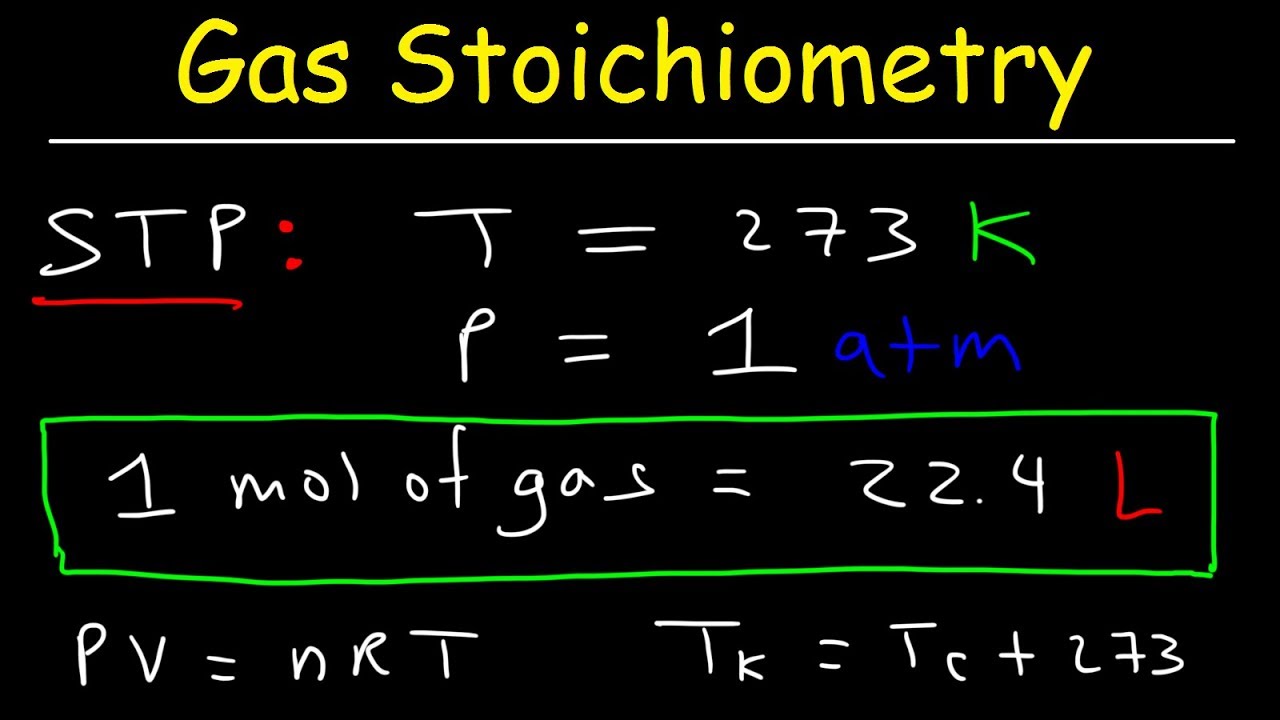
  • 📐 The first step in solving the problem is converting liters of CH4 to moles of CH4 using the molar volume at STP, which is 22.4 liters per mole.
  • 📝 After converting to moles, the next step is to determine the moles of H2O produced from the moles of CH4 using the stoichiometry of the chemical equation.
  • ⚖️ The final step is to convert moles of H2O to grams using the molar mass of water, which is 18 grams per mole.
  • 📉 The calculation shows that 58.2 liters of CH4 at STP is equivalent to 2.60 moles of CH4.
  • 📈 From the 2.60 moles of CH4, the stoichiometry of the reaction indicates that 5.20 moles of H2O will be produced.
  • 📊 Converting the 5.20 moles of H2O to grams yields a final answer of 93.6 grams of water.
  • ⚠️ It's emphasized that the 22.4-liter molar volume rule for gases at STP must be used only under specific conditions of 0°C and 1 atm pressure.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is solving a chemistry problem involving the combustion of natural gas, specifically methane (CH4), and calculating the amount of water (H2O) produced from a given volume of methane at standard temperature and pressure (STP).

  • What is the chemical equation for the combustion of methane mentioned in the script?

    -The chemical equation for the combustion of methane is CH4 + 2O2 → CO2 + 2H2O. This equation represents methane combining with oxygen to produce carbon dioxide and water, along with the release of a significant amount of heat.

  • Why is it important to convert liters of methane to moles for the problem?

    -It is important to convert liters of methane to moles because the chemical equation requires moles to calculate the reaction. The problem provides the volume of methane, so converting it to moles allows for the use of stoichiometry in the calculation.

  • What is the significance of the 22.4-liters rule at STP?

    -The 22.4-liters rule at STP signifies that one mole of any ideal gas occupies 22.4 liters at standard temperature and pressure (0 degrees Celsius and 1 atmosphere). This rule is used to convert the volume of a gas to the number of moles.

  • How many moles of CH4 are in 58.2 liters at STP?

    -Using the 22.4-liters rule at STP, 58.2 liters of CH4 is converted to moles by dividing by 22.4, resulting in approximately 2.60 moles of CH4.

  • What is the relationship between moles of CH4 and moles of H2O produced according to the script?

    -According to the script, for every mole of CH4 combusted, two moles of H2O are produced. This is derived from the balanced chemical equation for the combustion of methane.

  • How many moles of H2O are produced from 2.60 moles of CH4?

    -From 2.60 moles of CH4, 5.20 moles of H2O are produced, as the ratio from the balanced chemical equation is 1 mole of CH4 to 2 moles of H2O.

  • What is the molar mass of water (H2O) and how is it used in the calculation?

    -The molar mass of water is 18 grams per mole, which is calculated by adding the atomic masses of two hydrogen atoms (1 gram each) and one oxygen atom (16 grams). This molar mass is used to convert moles of H2O to grams.

  • How many grams of H2O are produced from 5.20 moles of H2O?

    -To find the mass in grams of 5.20 moles of H2O, multiply the moles by the molar mass of water (18 grams/mole), resulting in 93.6 grams of H2O.

  • Why is it crucial to use the correct conditions (STP) when applying the 22.4-liters rule?

    -The 22.4-liters rule is only applicable under specific conditions of STP (standard temperature and pressure). If the conditions differ, the volume occupied by a mole of gas will also differ, and the ideal gas law must be used for accurate calculations.

  • What is the final step in solving the problem according to the script?

    -The final step in solving the problem, as outlined in the script, is to convert the moles of H2O produced into grams using the molar mass of water.

Outlines
00:00
🔍 Combustion of Natural Gas and Water Production Calculation

The script begins by introducing a chemistry problem involving the combustion of natural gas, specifically methane (CH4). It explains the chemical equation for the combustion process, which produces carbon dioxide (CO2) and water (H2O) along with a significant amount of heat. The main focus is on calculating the amount of water produced by burning 58.2 liters of methane at standard temperature and pressure (STP), assuming an excess of oxygen. The solution involves several steps: converting liters of CH4 to moles, using the chemical equation to find the moles of H2O produced, and finally converting moles of H2O to grams using the molar mass of water. The script emphasizes the importance of using the correct conversion factors and the conditions under which the 22.4-liters-per-mole rule at STP applies.

05:00
📚 Step-by-Step Solution for Calculating Water from Methane Combustion

This paragraph continues the detailed explanation of the calculation process for determining the mass of water produced from the combustion of methane. It starts by converting the given volume of methane at STP to moles using the molar volume of a gas at STP, which is 22.4 liters per mole. The calculation results in 2.60 moles of CH4. Next, the script uses the chemical equation's stoichiometry to find the molar relationship between CH4 and H2O, revealing that one mole of CH4 yields two moles of H2O. Applying this ratio, the script calculates 5.20 moles of H2O from the 2.60 moles of CH4. Finally, to find the mass of water produced, the molar mass of water (18 grams per mole) is used to convert the moles of H2O to grams, resulting in 93.6 grams of H2O. The script concludes with a review of the steps and a reminder that the 22.4-liters-per-mole rule is only applicable under STP conditions, and for different conditions, the ideal gas law must be used.

Mindmap
Keywords
💡Combustion
Combustion is a chemical reaction that involves the reaction of a substance with oxygen, producing heat and light. In the context of the video, it specifically refers to the burning of natural gas, which is methane (CH4). The script discusses the combustion process to explain how methane reacts with oxygen to produce carbon dioxide (CO2) and water (H2O), along with a significant amount of heat.
💡Methane (CH4)
Methane is a hydrocarbon with the chemical formula CH4, consisting of one carbon atom bonded to four hydrogen atoms. It is the primary component of natural gas and is the focus of the chemical equation presented in the video. The script uses methane as an example to demonstrate the calculation of the amount of water produced during its combustion.
💡Oxygen (O2)
Oxygen is a chemical element with the symbol O and is a key reactant in the combustion process. In the script, oxygen is combined with methane to form carbon dioxide and water. The video mentions that an excess of oxygen is assumed, which simplifies the calculation by ensuring that methane is the limiting reactant.
💡Carbon Dioxide (CO2)
Carbon dioxide is a chemical compound with the formula CO2. It is one of the products of the combustion of methane, as described in the script. The formation of CO2 is a critical aspect of the chemical reaction, as it is a greenhouse gas and contributes to global warming.
💡Water (H2O)
Water is a chemical compound with the chemical formula H2O, consisting of two hydrogen atoms and one oxygen atom. In the script, water is highlighted as one of the products of methane combustion. The main goal of the problem presented in the video is to calculate the amount of water produced from a given volume of methane at standard temperature and pressure (STP).
💡Standard Temperature and Pressure (STP)
STP refers to standard temperature and pressure conditions, which are defined as 0 degrees Celsius (273.15 Kelvin) and 1 atmosphere of pressure. The script uses STP to establish a basis for converting volumes of gases to moles, as one mole of any gas at STP occupies 22.4 liters.
💡Molar Mass
Molar mass is the mass of one mole of a substance, typically expressed in grams per mole (g/mol). In the video, the molar mass of water (18 g/mol) is used to convert the number of moles of water produced to grams, which is the final answer to the problem presented.
💡Moles
Moles are a unit of measurement used in chemistry to express amounts of a chemical substance. The script demonstrates the conversion of liters of methane to moles, which is a necessary step in solving the problem of calculating the amount of water produced during combustion.
💡Conversion Factors
Conversion factors are used in chemistry to change the units of a measurement. In the script, the conversion factor of 22.4 liters per mole at STP is used to convert the volume of methane to moles, and the molar mass of water is used to convert moles of water to grams.
💡Chemical Equation
A chemical equation is a representation of a chemical reaction, showing the reactants and products along with their respective quantities. The script uses the chemical equation for the combustion of methane to illustrate the stoichiometric relationship between methane, oxygen, carbon dioxide, and water.
💡Stoichiometry
Stoichiometry is the calculation of the quantitative relationships between reactants and products in a chemical reaction. The script applies stoichiometry to determine the moles of water produced from a given amount of methane based on the balanced chemical equation.
Highlights

The problem involves calculating the amount of water produced by the combustion of natural gas (methane).

The chemical equation for the combustion of methane is provided, along with the requirement to assume an excess of oxygen.

The process begins with converting 58.2 liters of CH4 at STP to moles using the 22.4 liters per mole rule.

The conversion from liters to moles is essential as chemical equations require moles for calculations.

The calculation results in 2.60 moles of CH4 from 58.2 liters at STP.

The next step is to determine the moles of H2O produced from the moles of CH4 using the combustion equation.

The stoichiometry of the equation shows that one mole of CH4 yields two moles of H2O.

Calculating the moles of H2O results in 5.20 moles from 2.60 moles of CH4.

The final step is converting moles of H2O to grams using the molar mass of water (18 grams per mole).

The molar mass calculation gives 93.6 grams of H2O produced from the combustion of 58.2 liters of CH4.

The importance of using the 22.4 liters per mole rule is emphasized for STP conditions only.

For non-STP conditions, the ideal gas law must be used for accurate calculations.

The process is broken down into clear, logical steps for solving the problem.

The transcript provides a roadmap for solving chemical equations involving gas volumes and molar conversions.

The example illustrates practical applications of stoichiometry in chemical reactions.

The solution process is detailed, guiding the reader through each calculation step.

The final answer is 93.6 grams of H2O, showcasing the practical outcome of the combustion of natural gas.

Transcripts

Browse More Related Video

Converting Between Moles and Liters of a Gas at STP

Gas Stoichiometry Problems

Mole Concept

Step by Step Stoichiometry Practice Problems | How to Pass Chemistry

Ideal gas equation example 2 | Chemistry | Khan Academy

Unit 4.5 - Stoichiometry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ChemistryCombustionMethaneSTPMolar ConversionWater ProductionGas LawsEducationalScienceTutorial