Converting Between Moles and Liters of a Gas at STP

Tyler DeWitt
31 May 201412:43
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script teaches viewers how to convert between moles and liters of a gas at Standard Temperature and Pressure (STP), which is defined as 0°C and 1 atm. It walks through the process using the molar volume of a gas at STP, which is 22.4 liters per mole. The script includes step-by-step problem-solving techniques and conversion factors, and it emphasizes the importance of using these calculations only under STP conditions and for gases, not liquids or solids. It also highlights common mistakes, such as applying STP conversions outside of STP conditions or to non-gaseous substances, and provides additional practice problems to reinforce the concepts.

Takeaways
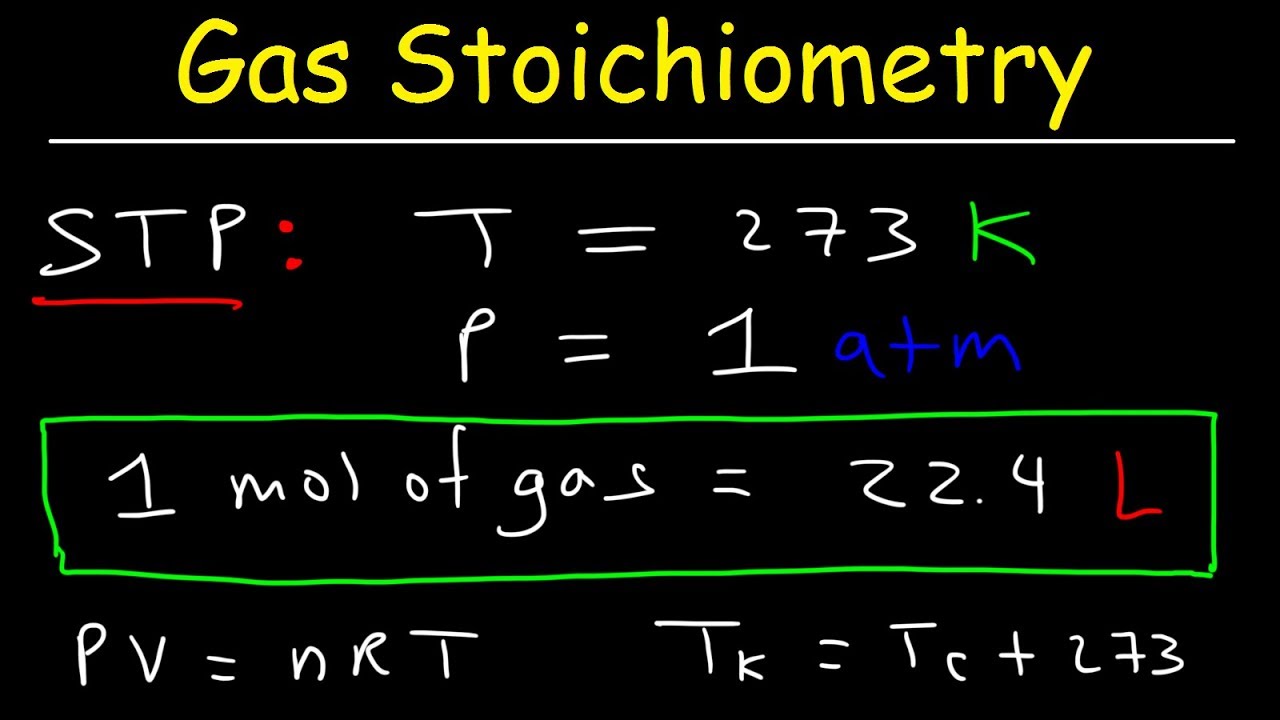
  • 🌡️ STP stands for Standard Temperature and Pressure, which is zero degrees Celsius and 1 atm.
  • 📏 At STP, one mole of any gas occupies 22.4 liters of volume.
  • 🔢 To convert moles to liters at STP, multiply the number of moles by 22.4 liters.
  • 🔄 Conversion factors can be used to convert between moles and liters, ensuring units cancel out appropriately.
  • 📉 To convert liters to moles at STP, divide the volume in liters by 22.4 liters.
  • ⚠️ The relationship of 22.4 liters per mole only applies to gases at STP and not to liquids or solids.
  • 🧩 Common mistake: Using the 22.4 liters per mole rule outside of STP conditions (e.g., different temperatures or pressures).
  • 💡 Another common mistake: Applying the gas volume relationship to liquids or solids instead of gases.
  • 🔍 Always double-check that the substance in question is a gas at STP before applying the 22.4 liters per mole rule.
  • 📚 For conditions other than STP, use the ideal gas law (PV=nRT) to solve problems involving gases.
  • 📉 Round answers to three significant figures, except when part of a definition or equation where precision is unlimited.
Q & A

  • What does STP stand for in the context of chemistry?

    -STP stands for Standard Temperature and Pressure, which are defined as 0 degrees Celsius and 1 atmosphere of pressure, respectively.

  • What is the volume occupied by one mole of any gas at STP?

    -At STP, one mole of any gas occupies 22.4 liters of volume.

  • How do you calculate the volume of 3.8 moles of CO2 gas at STP?

    -To calculate the volume of 3.8 moles of CO2 gas at STP, multiply the number of moles (3.8) by the volume occupied by one mole of gas at STP (22.4 liters), which gives 3.8 * 22.4 liters.

  • What is a conversion factor and how is it used in the context of moles and liters?

    -A conversion factor is a ratio that shows the equivalence between two quantities. In the context of moles and liters, it is used to convert from moles to liters or vice versa by multiplying the given quantity by the conversion factor that cancels out the unit you want to eliminate.

  • Why is it incorrect to use the 22.4 liters/mole relationship outside of STP conditions?

    -The 22.4 liters/mole relationship is specific to STP conditions (0 degrees Celsius and 1 atm pressure). Using this relationship outside of these conditions, such as at different temperatures or pressures, will yield incorrect results.

  • What is the common mistake made when calculating the volume of gases at non-STP conditions?

    -A common mistake is using the 22.4 liters/mole relationship for gases at non-STP conditions, such as different temperatures or pressures. The correct approach for non-STP conditions is to use the ideal gas law (PV=nRT).

  • How many moles are in 58.6 liters of nitrogen gas at STP?

    -To find the number of moles in 58.6 liters of nitrogen gas at STP, divide the volume (58.6 liters) by the volume occupied by one mole of gas at STP (22.4 liters), which gives 58.6 / 22.4 moles.

  • Why can't the 22.4 liters/mole relationship be used for liquids or solids?

    -The 22.4 liters/mole relationship is only applicable to gases at STP. For liquids or solids, different properties and equations must be used to calculate volume or moles, as the behavior of these states of matter differs significantly from that of gases.

  • What is the volume occupied by 0.735 moles of O2 gas at STP?

    -To find the volume occupied by 0.735 moles of O2 gas at STP, multiply the number of moles (0.735) by the volume occupied by one mole of gas at STP (22.4 liters), which gives 0.735 * 22.4 liters.

  • How many moles are in 13.0 liters of chlorine gas at STP?

    -To determine the number of moles in 13.0 liters of chlorine gas at STP, divide the volume (13.0 liters) by the volume occupied by one mole of gas at STP (22.4 liters), which gives 13.0 / 22.4 moles.

  • What are the two key considerations when converting between moles and liters of a gas at STP?

    -The two key considerations are: 1) Ensure that the conditions specified in the problem are indeed STP (0 degrees Celsius and 1 atm pressure), and 2) Confirm that the substance in question is a gas, as the 22.4 liters/mole relationship does not apply to liquids or solids.

Outlines
00:00
🧪 Converting Moles to Liters at STP

This paragraph introduces the concept of converting between moles and liters of a gas at Standard Temperature and Pressure (STP), defined as 0°C and 1 atm. The key information provided is that one mole of any gas occupies 22.4 liters at STP. The paragraph demonstrates how to calculate the volume of 3.8 moles of CO2 gas at STP, using both direct multiplication and conversion factors. It emphasizes the importance of using the correct conversion factor to cancel out the units and arrive at the volume in liters. The final answer is given as 85.1 liters, rounded to three significant figures.

05:02
📚 Calculating Moles from Liters and Common Mistakes

This section continues the theme of gas calculations at STP, focusing on determining the number of moles from a given volume. It illustrates the process using 58.6 liters of nitrogen gas, showing both the division method and the use of conversion factors to find the moles of gas. The result is 2.62 moles, rounded to three significant figures. The paragraph then discusses common mistakes made when calculating volumes and moles outside of STP conditions or when dealing with substances that are not gases, such as liquids or solids. It stresses the importance of adhering to STP conditions and the state of matter (gas) when using the 22.4 liters per mole relationship.

10:04
🔍 Further Practice with Moles and Liters Conversions

The final paragraph provides additional examples to practice converting between moles and liters of gas at STP. It includes calculating the volume occupied by 0.735 moles of O2 gas and determining the number of moles in 13.0 liters of chlorine gas at STP. The calculations are performed using the established relationship between moles and volume at STP, with conversion factors to simplify the process. The outcomes are 16.5 liters for the volume of O2 gas and 0.580 moles for the amount of chlorine gas, reinforcing the principles introduced earlier in the script. The paragraph also reiterates the importance of ensuring that the conditions are at STP and that the substance is a gas to apply the 22.4 liters per mole rule correctly.

Mindmap
Keywords
💡Moles
Moles in chemistry refer to the amount of a substance, measured in units called moles, which is a count of the number of atoms, molecules, or ions. In the video, moles are used to quantify the amount of gas, specifically CO2 and nitrogen, at standard temperature and pressure (STP). The script explains how to convert moles to liters, using the relationship that one mole of any gas occupies a specific volume at STP.
💡Liters
Liters are a unit of volume used to measure the space that a substance occupies. In the context of the video, liters are used to express the volume of gases at STP. The video script provides examples of how to calculate the volume of a gas given its number of moles, and vice versa, using the molar volume of a gas at STP.
💡STP (Standard Temperature and Pressure)
STP stands for standard temperature and pressure, which are defined as 0 degrees Celsius and 1 atmosphere of pressure, respectively. The video emphasizes that the relationship between moles and liters of a gas is specific to STP. The script uses STP as a condition to apply the molar volume of a gas in calculations.
💡Conversion Factors
Conversion factors are used in chemistry to convert between different units of measurement. In the video, conversion factors are derived from the relationship that one mole of gas equals 22.4 liters at STP. These factors are then used to perform calculations involving the conversion between moles and liters of gas.
💡Molar Volume
Molar volume is the volume occupied by one mole of any ideal gas at STP, which is 22.4 liters. The video script explains how to use molar volume to find the volume of a given number of moles of a gas, and how to calculate the number of moles in a given volume of gas at STP.
💡Ideal Gas Law
The ideal gas law is a mathematical equation that describes the relationship between pressure, volume, temperature, and the amount of gas. Although not the main focus of the video, the script mentions the ideal gas law (PV=NRT) as the appropriate method to use when conditions are not at STP, highlighting its relevance in different scenarios.
💡Significant Figures
Significant figures are the digits in a number that carry meaning contributing to its precision. The video script discusses rounding answers to three significant figures, which is a common practice in scientific calculations to ensure the precision of the results.
💡Equivalence
Equivalence in the context of the video refers to the relationship between one mole of gas and its volume at STP, which is 22.4 liters. The script uses this equivalence to create conversion factors that allow for the conversion between moles and liters.
💡Common Mistakes
The video script highlights common mistakes made when converting between moles and liters, such as using the molar volume of a gas at conditions other than STP or applying the concept to liquids or solids instead of gases. These mistakes are pointed out to help viewers avoid similar errors.
💡Gas
In the video, the term 'gas' is used to refer to the state of matter that is being measured in moles and liters. The script emphasizes that the molar volume concept applies only to gases at STP and not to liquids or solids, which is a crucial distinction for accurate calculations.
Highlights

The video teaches how to convert between moles and liters of gas at STP (Standard Temperature and Pressure).

STP is defined as zero degrees Celsius and 1 atm.

At STP, one mole of any gas occupies 22.4 liters.

Demonstration of converting 3.8 moles of CO2 gas to liters at STP.

Use of conversion factors to solve problems involving moles and liters.

The method to calculate the volume of 3.8 moles of CO2 gas at STP is shown.

Final answer for the volume of 3.8 moles of CO2 gas is 85.1 liters.

Explanation of how to find moles from liters of gas at STP.

Calculation of moles in 58.6 liters of nitrogen gas at STP.

Common mistakes when not adhering to STP conditions (e.g., different temperatures and pressures).

Clarification that the 22.4 liters per mole rule only applies to gases at STP.

Highlighting the incorrect application of the 22.4 liters per mole rule to non-gaseous states.

Two additional problems are solved to practice mole and liter conversions at STP.

Conversion of 0.735 moles of O2 gas to liters at STP.

Conversion of 13.0 liters of chlorine gas to moles at STP.

Emphasis on ensuring the problem specifies STP conditions and involves a gas.

Transcripts

Browse More Related Video

Gas Stoichiometry: Equations Part 1

Gas Stoichiometry Problems

Ideal gas equation example 2 | Chemistry | Khan Academy

Mole Concept

Know This For Your Chemistry Final Exam - Stoichiometry Review

9.3 Additional Gas Laws | Dalton's Law and Graham's Law | High School Chemistry

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Chemistry TutorialSTP ConversionGas LawsMole CalculationVolume to MolesMoles to VolumeIdeal Gas LawEducational ContentScience EducationChemistry MistakesProblem Solving