Ideal gas equation example 2 | Chemistry | Khan Academy
TLDRThis educational video script explores the ideal gas equation, demonstrating how pressure changes with volume while temperature is held constant. It illustrates the concept with an example of a gas in a container, showing that reducing volume by two-thirds increases pressure threefold. The script further explains the relationship between pressure, volume, and temperature using the formula PV = nRT, and provides an example of calculating temperature changes. It concludes with a discussion on standard temperature and pressure (STP), revealing that one mole of an ideal gas occupies 22.4 liters at STP, a valuable concept in chemistry and physics.
Takeaways
- 🌡️ The ideal gas equation is \( PV = nRT \), where \( P \) is pressure, \( V \) is volume, \( n \) is the number of moles, \( R \) is the gas constant, and \( T \) is temperature.
- 🔍 When the temperature and the number of moles of a gas are held constant, the product of pressure and volume (PV) remains constant.
- 📏 If the volume of a container is reduced while holding temperature constant, the pressure of the gas inside increases proportionally.
- 🔎 The initial pressure of 3 atmospheres and volume of 9 liters can be used to calculate the new pressure when the volume is reduced to 3 liters by using the relationship \( P_1V_1 = P_2V_2 \).
- 🔄 When the volume is decreased to one-third of its original size, the pressure increases by a factor of three, resulting in a new pressure of 9 atmospheres.
- 🌡️ The concept of \( \frac{PV}{T} \) being a constant for a given number of moles of gas is crucial, indicating that changes in pressure and volume are inversely proportional to changes in temperature.
- ⚖️ The ideal gas equation can be rearranged to \( \frac{PV}{T} = nR \), showing that the ratio of pressure and volume to temperature is constant for a fixed number of moles.
- 🌡️ Converting Celsius to Kelvin is essential for calculations involving temperature, as Kelvin is the standard unit in the ideal gas equation.
- 🔢 Example calculations in the script demonstrate how changes in pressure and volume affect temperature, and vice versa, under constant conditions.
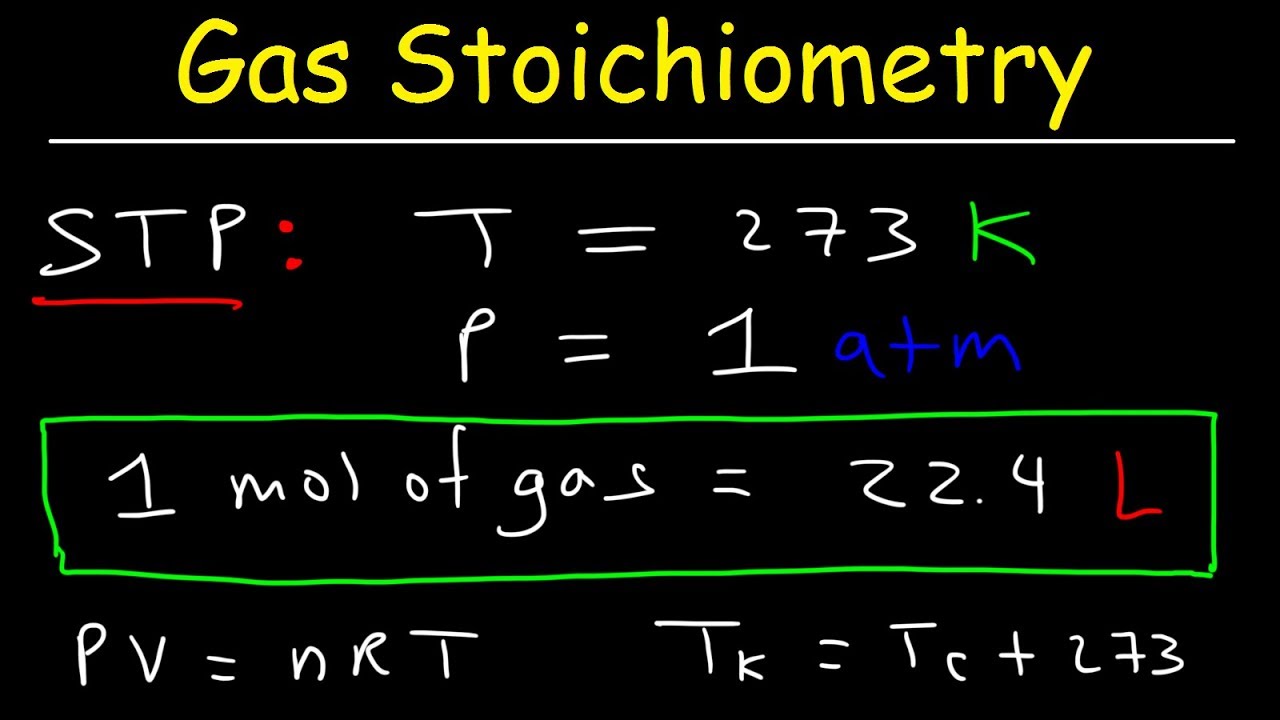
- 📚 The script discusses the concept of standard temperature and pressure (STP), which is often defined as 0°C (273 Kelvin) and 1 atmosphere, although there are variations in definitions.
- 📦 At STP, one mole of an ideal gas occupies 22.4 liters, which is a useful constant for calculations involving moles and volumes of gases.
Q & A
What is the ideal gas equation?
-The ideal gas equation is PV = nRT, where P is the pressure, V is the volume, n is the number of moles of the gas, R is the ideal gas constant, and T is the temperature in Kelvin.
What happens to the pressure of a gas when the volume of its container is reduced, assuming the temperature is constant?
-When the volume of a container is reduced while the temperature is held constant, the pressure of the gas increases. This is because the same number of gas particles are now exerting force over a smaller area.
If the initial pressure of a gas is 3 atmospheres and the volume is 9 liters, what is the new pressure if the volume is reduced to 3 liters?
-If the volume is reduced to one-third of its original size while the temperature is constant, the pressure will increase by a factor of three. So the new pressure will be 9 atmospheres.
What does it mean to hold the temperature constant in the context of the ideal gas equation?
-Holding the temperature constant means that the kinetic energy of the gas particles does not change. This is a key assumption in the ideal gas equation, as it allows for predictable relationships between pressure, volume, and the number of moles of the gas.
What is the significance of the ratio PV/T in the context of the ideal gas law?
-The ratio PV/T is a constant for a given amount of gas at constant temperature. This means that if you change the pressure, volume, or temperature, the product of pressure and volume divided by temperature will remain the same, assuming the number of moles of gas does not change.
How do you convert Celsius to Kelvin?
-To convert a temperature from Celsius to Kelvin, you add 273 to the Celsius temperature. For example, 0 degrees Celsius is equal to 273 Kelvin.
What is the standard temperature and pressure (STP) according to the script?
-According to the script, the standard temperature and pressure (STP) is defined as a temperature of 0 degrees Celsius (273 Kelvin) and a pressure of 1 atmosphere, which can also be expressed as 101.325 kilopascals.
How many liters will 1 mole of an ideal gas occupy at STP?
-At standard temperature and pressure, 1 mole of an ideal gas will occupy exactly 22.4 liters.
What is the relationship between the number of moles of gas and the volume it occupies at STP?
-The volume occupied by a gas at STP is directly proportional to the number of moles of the gas. If you double the number of moles, the volume will also double, assuming the temperature and pressure remain constant.
What is the volume in meters cubed for 1 mole of an ideal gas at STP?
-The volume of 1 mole of an ideal gas at STP is 22.4 liters, which is equivalent to 0.0224 meters cubed (since 1 meter cubed is equal to 1000 liters).
Why is it important to convert units when working with the ideal gas equation?
-It is important to convert units when working with the ideal gas equation to ensure consistency and accuracy in calculations. For example, the temperature must be in Kelvin, and the pressure and volume must be in compatible units, such as atmospheres and liters or kilopascals and cubic meters.
Outlines
🧪 Ideal Gas Law Application
This paragraph introduces the application of the ideal gas law in a scenario where a gas's pressure changes due to a change in volume while the temperature remains constant. The initial conditions of the gas are given as 3 atmospheres of pressure and 9 liters of volume. The problem posed is to determine the new pressure if the volume is reduced to 3 liters. The explanation uses the ideal gas law formula PV = nRT to show that the pressure will triple when the volume is reduced to one-third of its original size, assuming the number of particles (n) and the gas constant (R) remain constant and the temperature (T) does not change.
🔍 Exploring Gas Behavior Under Variable Conditions
The second paragraph delves into how the behavior of an ideal gas can be predicted under different conditions of pressure, volume, and temperature. It uses the ideal gas law to demonstrate the relationship between these variables, particularly focusing on how changes in pressure and volume affect temperature. The example given starts with a pressure of 1 atmosphere, a volume of 2 meters cubed, and a temperature of 27 degrees Celsius (converted to 300 Kelvin). The scenario explores increasing the pressure to 5 atmospheres and decreasing the volume to 1 meter cubed, leading to a new temperature calculation of 750 Kelvin. The paragraph also touches on the concept of standard temperature and pressure (STP), noting the discrepancies in definitions but ultimately using 0 degrees Celsius (273 Kelvin) and 1 atmosphere as the standard for further calculations.
📏 Calculating Molar Volume at Standard Temperature and Pressure
The final paragraph focuses on calculating the volume occupied by one mole of an ideal gas at standard temperature and pressure (STP), defined as 0 degrees Celsius (273 Kelvin) and 1 atmosphere. Using the ideal gas law PV = nRT, the paragraph demonstrates the calculation with R being the specific gas constant for use with atmospheres and liters. The result shows that one mole of an ideal gas at STP occupies 22.4 liters. The paragraph also provides a conversion to cubic meters, illustrating that 22.4 liters is equivalent to 0.0224 cubic meters. It concludes by emphasizing the direct proportionality between the number of moles of gas and the volume it occupies at STP, a useful concept for understanding gas behavior under standard conditions.
Mindmap
Keywords
💡Ideal Gas Equation
💡Pressure
💡Volume
💡Temperature
💡Moles
💡Kinetic Energy
💡Standard Temperature and Pressure (STP)
💡Avogadro's Number
💡Pascal
💡Kelvin
💡Constant
Highlights
The ideal gas equation is used to solve problems involving changes in gas properties under constant temperature.
When the volume of a container decreases, the pressure of the gas increases, assuming the temperature and the number of particles remain constant.
The ideal gas equation is PV = nRT, where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature.
The number of particles (n) and the gas constant (R) do not change when the volume is altered, maintaining the product of pressure and volume constant at constant temperature.
An example calculation shows that if the volume is reduced to one-third, the pressure increases by a factor of three, assuming constant temperature.
The relationship PV/T = nR can be derived from the ideal gas equation, indicating that the ratio of pressure and volume to temperature is constant for a given number of moles.
An example demonstrates how changes in pressure and volume can be used to calculate the new temperature of a gas, given the initial conditions.
The concept of standard temperature and pressure (STP) is discussed, noting that there is no universal agreement on the exact values.
STP is commonly defined as 0 degrees Celsius (273 Kelvin) and 1 atmosphere, but variations exist.
At STP, one mole of an ideal gas occupies 22.4 liters, which is a useful constant for calculations involving moles and volumes.
The volume occupied by a gas at STP can be converted to cubic meters, with 1 mole occupying 0.0224 cubic meters.
The relationship between moles, volume, and pressure at STP is linear; doubling the moles doubles the volume, and halving the moles halves the volume.
The importance of unit consistency in calculations involving the ideal gas equation is emphasized.
The practical application of the ideal gas equation in understanding the behavior of gases under various conditions is highlighted.
The video provides a step-by-step approach to solving problems using the ideal gas equation, making it accessible for educational purposes.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: