Algebra Basics: What Are Polynomials? - Math Antics
TLDRIn this Math Antics video, Rob introduces the concept of polynomials in Algebra, starting with the fundamental building blocks called 'terms'. Terms consist of a coefficient (number part) and a variable part (which may include variables raised to powers). Polynomials are chains of these terms linked by addition or subtraction, with specific names like 'monomial', 'binomial', and 'trinomial' based on the number of terms. The video also covers the concept of 'degree', which is determined by the highest power of the variable in a term, and explains how polynomials are arranged from the highest to the lowest degree. Rob simplifies the understanding of polynomials by discussing coefficients, including negative ones, and emphasizes the importance of arranging terms correctly for polynomial simplification.
Takeaways
- 📚 A polynomial is a mathematical expression consisting of multiple terms linked by addition or subtraction.
- 🔢 Terms are made up of two parts: a coefficient (number part) and a variable part, which can include variables raised to various powers.
- 💡 The order of a term is conventionally written with the coefficient first, followed by the variable part.
- 🎓 A monomial has one term, a binomial has two terms, and a trinomial has three terms, with more terms simply referred to as a polynomial.
- 🤔 The absence of a number part in a term implies a coefficient of 1, and the absence of a variable part can be thought of as the variable raised to the 0th power, which equals 1.
- 📉 The degree of a term is determined by the highest power of the variable within it, and for terms with multiple variables, the degrees are summed.
- 📈 Polynomials are often classified by the degree of their highest term, which influences their behavior and the number of solutions they have.
- 🔄 Polynomials should be arranged with terms in descending order of degree, from highest to lowest.
- 🔄 Missing terms in a polynomial can be considered as having coefficients of zero, which do not affect the polynomial's value.
- 📝 It's crucial to consider the sign in front of each term as part of its coefficient when rearranging or simplifying polynomials.
- 🔑 Understanding polynomials and their properties is foundational for further study in algebra and solving more complex mathematical problems.
Q & A
What is the basic concept of a term in Algebra?
-A term in Algebra is a mathematical expression made up of two parts: a number part, known as the coefficient, and a variable part, which can be one or more variables raised to a power. The two parts are usually written next to each other without a multiplication symbol.
What is the coefficient in a term?
-The coefficient is the number part of a term, which is simply a number like 2, 5, or 1.4. It is multiplied by the variable part of the term.
How is a variable part of a term represented?
-The variable part of a term can be a single variable raised to a power, such as 'x squared', or a combination of variables raised to various powers, like 'x squared times y squared'.
What is the significance of the order of the number and variable parts in a term?
-It is conventional to write the number part of the term first, followed by the variable part. This order is important for understanding and simplifying expressions.
What is a polynomial?
-A polynomial is a combination of many terms linked together using addition or subtraction. Each term within the polynomial must be joined by either addition or subtraction.
What are the specific names for polynomials with one, two, or three terms?
-A polynomial with one term is called a 'monomial', with two terms it's a 'binomial', and with three terms, it's a 'trinomial'.
How is the degree of a term determined?
-The degree of a term is determined by the power of the variable part. If a term has multiple variables, the degrees of each variable are added together to get the overall degree of the term.
Why is the degree of terms important in polynomials?
-The degree of terms is important because polynomials are often referred to by the degree of their highest term. It also helps in arranging the terms of a polynomial from highest to lowest degree.
What is a constant term in a polynomial?
-A constant term in a polynomial is a term without a variable part, or a term where the variable is raised to the 0th power, which simplifies to 1 and does not affect the value of the term.
How should terms in a polynomial be arranged?
-Terms in a polynomial should be arranged in order from the highest degree to the lowest degree. This organization helps in simplifying and solving polynomial equations.
How should you think about the signs in front of terms in a polynomial?
-In polynomials, it's best to think of all terms as being added together, with each term having either a positive or a negative coefficient, which is indicated by the sign directly in front of the term.
Outlines
📚 Introduction to Polynomials and Terms
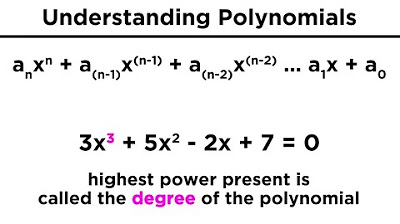
In the first paragraph, Rob introduces the concept of polynomials as a significant topic in Algebra. He explains that polynomials are made up of 'terms,' which are mathematical expressions consisting of a coefficient (number part) and a variable part. The variable part can be a single variable raised to a power or a combination of variables multiplied together and raised to various powers. Rob emphasizes the conventional order of writing terms with the coefficient first, followed by the variable part. He also introduces the special case of terms with implied coefficients or variables, such as 'x' being equivalent to 'x' to the first power or a constant term representing a term with a variable raised to the zero power.
🔢 Understanding Polynomial Structure and Degree
The second paragraph delves deeper into the structure of polynomials, explaining how they are arranged from the highest to the lowest degree terms. Rob discusses the concept of 'degree,' which refers to the power of the variable part in a term, and how it is crucial in naming polynomials based on their highest degree term. He illustrates this with examples, showing how to identify and arrange terms within a polynomial. Additionally, he touches on the idea of constant terms, which do not contain variables and thus have a degree of zero. The paragraph highlights the importance of recognizing coefficients, both positive and negative, as they indicate the value and arrangement of terms within a polynomial.
🎓 Wrapping Up Polynomial Concepts
In the concluding paragraph, Rob summarizes the key points covered in the video about polynomials. He advises viewers that it's normal to feel overwhelmed by the amount of new information and encourages them to revisit the video and practice problems to solidify their understanding. The paragraph ends with a reminder to check out Math Antics' website for more learning resources, and a friendly sign-off until the next video.
Mindmap
Keywords
💡Polynomials
💡Terms
💡Coefficient
💡Variable
💡Exponent
💡Monomial
💡Binomial
💡Trinomial
💡Degree
💡Constant Term
Highlights
Introduction to the concept of Polynomials in Algebra.
Definition of 'terms' in Algebra as mathematical expressions with a number part and a variable part.
Explanation of the term's number part being called the 'coefficient'.
Description of the variable part of a term, which can include variables raised to powers.
Clarification that 'y' is equivalent to 'y' raised to the 1st power, with the exponent 1 typically omitted.
Convention of writing the number part of a term before the variable part.
Introduction to polynomials as a combination of many terms linked by addition or subtraction.
Classification of polynomials based on the number of terms: monomial, binomial, trinomial, and general polynomial.
Example of a polynomial and explanation of terms missing number or variable parts.
Understanding that a term with only a variable part has an implicit coefficient of 1.
Concept that any number or variable to the 0th power equals 1, making constant terms without variables.
Identification of the constant term in a polynomial that always retains the same value.
Introduction to the 'degree' of terms and polynomials, determined by the power of the variable part.
Explanation of how to calculate the degree of a term with multiple variables by summing their powers.
Importance of the degree in naming polynomials and arranging terms from highest to lowest degree.
Guidance on rearranging polynomials with consideration of coefficients and their signs.
Emphasis on treating all terms in a polynomial as added together, with positive or negative coefficients.
Encouragement for re-watching the video and practicing to better understand polynomials.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: