Binomial theorem combinatorics connection | Algebra II | Khan Academy

Khan Academy

12 May 200811:59

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the combinatorial nature of multiplying binomials, specifically focusing on the expansion of (a + b)^3. The presenter uses a colorful, step-by-step approach to illustrate the process, emphasizing the importance of each term in the expansion. By meticulously multiplying each combination of 'a' and 'b', the video reveals how binomial coefficients emerge naturally from the process. The script aims to provide an intuitive understanding of why these coefficients are present and how they relate to the concept of combinations, ultimately offering a deeper insight into the binomial theorem.

Takeaways

📚 The video aims to provide an intuitive understanding of why multiplying binomials involves combinatorics and the role of binomial coefficients.
🎨 The use of multiple colors in the explanation is intentional to help illustrate the process and make it easier to follow.
🔢 The script demonstrates the multiplication of (a + b) to the third power using the distributive property and emphasizes the importance of the order of operations.
📉 The process involves breaking down the multiplication into smaller parts and multiplying each term step by step.
🌈 The colors used for each term help to visually distinguish between the different parts of the binomial being multiplied.
🔍 Each term in the expansion is a product of three numbers, with one from each of the three binomial expressions (a + b).
🧩 The expansion shows every possible combination of choosing 'a' or 'b' from each of the three binomial expressions.
📈 The script explains how the coefficients in the binomial expansion, such as 3a^2b, relate to the number of combinations for choosing 'a's and 'b's.
🤔 The video encourages viewers to think about the process as picking terms from each binomial expression, highlighting the connection to permutations and combinations.
📝 The explanation concludes by relating the coefficients in the binomial expansion to the concept of 'choose' from combinatorics, such as 3 choose 2 for the a^2b term.
🌟 The overall goal is to provide a deep intuition for why combinations are involved in the binomial theorem and how they relate to the expansion of a binomial to a certain power.

Q & A

What is the main topic of the video?
-The main topic of the video is to explain the intuition behind why multiplying binomials involves combinatorics and the role of binomial coefficients in the process.
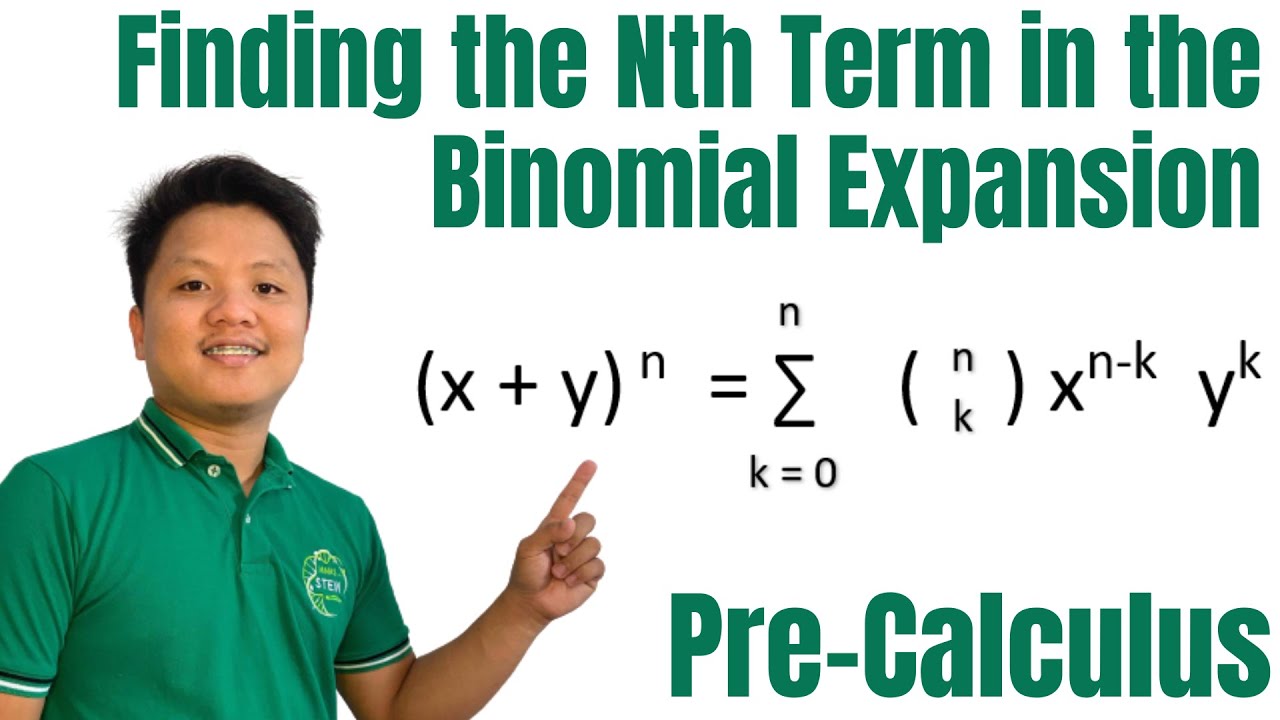
What is the binomial being multiplied in the video?
-The binomial being multiplied in the video is (a + b) to the third power.
How does the video use colors to aid understanding?
-The video uses colors to distinguish between different terms and factors in the binomial expansion, making it easier to track and understand the process.
What is the purpose of using the distributive property in the video?
-The distributive property is used to expand the binomial (a + b)^3 step by step, showing each multiplication and how the terms are generated.
How many terms are there in the expansion of (a + b)^3 before simplification?
-There are eight terms in the expansion of (a + b)^3 before any simplification.
What does the video suggest about the relationship between the number of terms and the binomial coefficients?
-The video suggests that the number of terms in the expansion corresponds to the binomial coefficients, which represent the number of ways to choose 'a' or 'b' from each set of terms.
How does the video explain the term 'a squared b' in the expansion?
-The video explains that 'a squared b' can be obtained in three different ways by choosing two 'a's and one 'b' from the three sets of (a + b), which corresponds to the binomial coefficient '3 choose 2'.
What is the significance of the binomial coefficient '3 choose 1' in the video?
-The binomial coefficient '3 choose 1' signifies the number of ways to choose one 'a' and two 'b's from the three sets of (a + b), which is used to determine the coefficient for the 'ab squared' term.
How does the video relate the binomial expansion to permutations and combinations?
-The video relates the binomial expansion to permutations and combinations by showing that each term in the expansion represents a unique way of choosing 'a' or 'b' from each set of (a + b), which is a combinatorial problem.
What is the final simplified form of the binomial expansion shown in the video?
-The final simplified form of the binomial expansion is not explicitly shown in the transcript, but it is implied that it would follow the pattern of the binomial theorem, with terms like a^3, a^2b, ab^2, and b^3, each multiplied by the appropriate binomial coefficient.

Outlines

00:00

📚 Introduction to Binomial Multiplication and Combinatorics

The video begins with an introduction to the concept of multiplying binomials and the role of combinatorics in the process. The speaker uses colors to visually distinguish between different terms and to illustrate the multiplication of (a + b)^3 step by step. The goal is to show why binomial coefficients appear in the expansion and to provide an intuitive understanding of the process.

05:02

🌈 Detailed Expansion of (a + b)^3

In this section, the speaker meticulously expands the expression (a + b)^3 using the distributive property, emphasizing the importance of each term and how they are derived. By multiplying the binomial by itself three times and carefully tracking the colors, the speaker demonstrates that each term in the expansion results from choosing either 'a' or 'b' from each instance of the binomial, resulting in a total of eight terms.

10:04

🔍 Understanding the Role of Combinations in Binomial Expansion

The speaker delves into the combinatorial aspect of the binomial expansion, explaining how each term in the expansion of (a + b)^3 corresponds to a specific combination of 'a's and 'b's. The explanation focuses on the number of ways to choose 'a' or 'b' from the three instances of the binomial, leading to the realization that the coefficients in the expansion are the same as those found in the binomial theorem. This part of the video aims to clarify why the binomial coefficients appear as they do and to provide a deeper understanding of the underlying combinatorial principles.

🎯 Conclusion and Summary of Binomial Theorem's Combinatorial Connection

The final paragraph wraps up the video by summarizing the insights gained from the detailed expansion of (a + b)^3. The speaker reiterates the connection between the binomial theorem and combinatorics, highlighting how the coefficients in the expansion are derived from combinations of 'a's and 'b's chosen from the three instances of the binomial. The video concludes with a reaffirmation of the importance of understanding these combinatorial principles to grasp the full intuition behind the binomial theorem.

Mindmap

Keywords

💡Binomials

Binomials are algebraic expressions with two terms, typically in the form of (a + b). In the video, the focus is on expanding binomials, specifically (a + b) to the third power, to illustrate the concept of binomial coefficients and combinatorics involved in the expansion.

💡Combinatorics

Combinatorics is a branch of mathematics concerned with counting, arrangement, and combination of objects. The video uses combinatorics to explain the process of multiplying binomials and determining the coefficients that appear in the expansion, such as '3 choose 2'.

💡Binomial Coefficients

Binomial coefficients are numerical values that appear in the expansion of binomials, represented as 'n choose k', where 'n' is the power of the binomial and 'k' is the term in the expansion. The video demonstrates how these coefficients are derived from the process of choosing terms from the binomial expression.

💡Distributive Property

The distributive property is a fundamental principle in algebra that allows for the expansion of products, such as (a + b)(c + d) = ac + ad + bc + bd. In the video, this property is used to expand (a + b) to the third power step by step.

💡Permutations and Combinations

Permutations and combinations are concepts within combinatorics that deal with the arrangement and selection of items, respectively. The video connects these concepts to the expansion of binomials, showing how the coefficients arise from the number of ways to choose certain terms from the binomial expression.

💡Color Coding

The video uses color coding as a visual aid to help the viewer track the terms being multiplied during the expansion of (a + b) to the third power. Each color represents a different instance of (a + b), aiding in understanding the combinatorial process.

💡Expansion

The expansion of an algebraic expression involves breaking it down into its simplest terms. In the context of the video, the expansion of (a + b) to the third power is carried out to show how each term is derived and how the binomial coefficients come into play.

💡Coefficients

In algebra, coefficients are the numerical factors that multiply the variables in an expression. The video discusses how coefficients in the binomial expansion are determined by the number of ways to select terms from the binomial, as illustrated by the binomial coefficients.

💡Binomial Theorem

The binomial theorem provides a formula for the expansion of powers of a binomial. It is central to the video's discussion, as it is used to explain why the coefficients in the expansion of (a + b)^n follow a specific pattern that can be predicted by combinatorics.

💡Intuition

The video aims to provide intuition behind the mathematical concepts being discussed. Intuition refers to the ability to understand or know something instinctively, without the need for conscious reasoning. The presenter uses the process of expanding binomials and color coding to build an intuitive understanding of binomial coefficients and combinatorics.

💡Simplification

Simplification in mathematics involves reducing an expression to a more straightforward or standard form. The video mentions that the expansion of (a + b) to the third power is not simplified initially to clearly demonstrate the combinatorial process before reaching the simplified form of the expansion.

Highlights

The video aims to provide intuition behind the binomial theorem and combinatorics in multiplying binomials.

The presenter uses multiple colors to illustrate the process, emphasizing their significance in the explanation.

The multiplication of (a + b) to the third power is demonstrated using the distributive property.

Each color represents a different instance of the binomial (a + b), aiding in visualizing the multiplication.

The process involves tedious multiplication, but it is crucial for understanding the outcome.

The expansion of (a + b)^3 is shown step by step, with each term being carefully calculated.

The multiplication results in terms with three numbers, each from a different color group.

The presenter explains that each term in the expansion is a product of choosing either 'a' or 'b' from each group.

The total number of terms in the expansion equals the number of ways to choose 'a' or 'b' from the three groups.

The simplification of the expansion reveals terms like a^3, a^2b, and ab^2, each with specific coefficients.

The coefficient '3' in front of a^2b is explained as 3 choose 2, linking to the concept of combinations.

The presenter explores the symmetry and the combinatorial aspect of the binomial expansion.

The binomial theorem's definition is related to the process of choosing 'a' or 'b' in the expansion.

The concept of '3 choose 1' and '3 choose 2' is used to explain the coefficients of the expanded terms.

The presenter concludes by summarizing the binomial expansion in terms of combinations.

The video provides a deep intuition for why combinations are involved in the binomial theorem.

The final message is that the binomial expansion can be viewed as choosing 'a' or 'b' in different combinations.

Transcripts

Browse More Related Video

Binomial Theorem (part 2)

Binomial Theorem (part 1)

Binomial Expansion Using Pascal's Triangle | Pre-Calculus

Finding the Nth Term in the Binomial Expansion | Binomial Theorem | Pre-Calculus

Multiplying Binomials by the FOIL Method

BINOMIAL THEOREM || PRE-CALCULUS