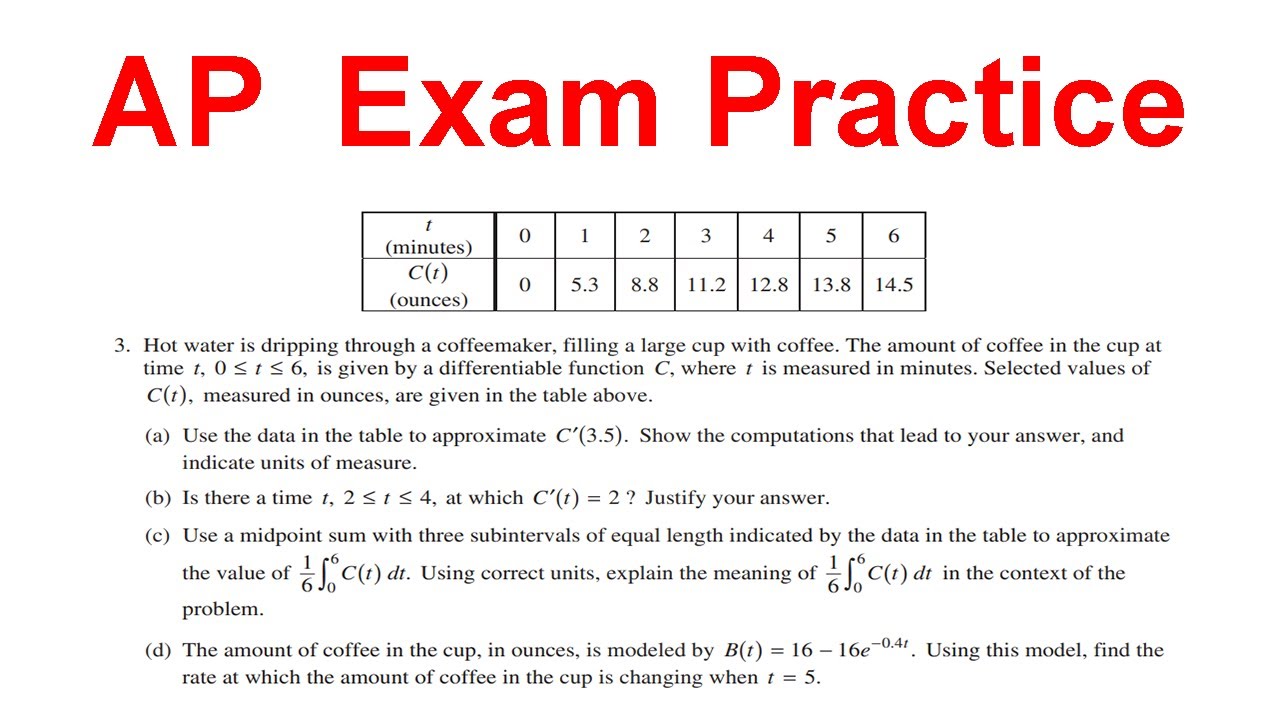
2013 AP Calculus AB Free Response #3
TLDRIn this video, Alan from Bothell Stem Coach dives into AP Calculus 2013 free response question number three, focusing on the non-calculator portion. The problem involves hot water dripping through a coffee maker to fill a cup, with the coffee amount at time T given by a specific function. Alan uses a table of selected values to approximate the derivative of the coffee amount function at 3.5 minutes, employing the secant line slope method. He then discusses the mean value theorem, which guarantees the existence of a time 'T' where the instantaneous rate of change equals the average rate over an interval. Alan proceeds to approximate the value of the integral using the midpoint sum with 3 subintervals, which provides an average value of coffee in the cup over a 6-minute period. Finally, he models the rate of change of coffee in the cup using a derivative function and calculates its value at T equals 5. The video concludes with a review of the scoring guidelines for the question, highlighting minor mistakes and emphasizing the importance of catching errors during the problem-solving process.
Takeaways
- 📚 The video is a continuation of a series on AP Calculus 2013 free response questions, focusing on the non-calculator portion.
- 🕒 The problem involves hot water dripping through a coffee maker to fill a cup with coffee, and the amount of coffee in the cup at time T=0 is given by a specific function.
- 📊 A table of selected values for the coffee amount function is provided, which is used to approximate the derivative of the function at T=3.5 minutes.
- 🔍 The secant line method is used to approximate the derivative, which is the rate of change of the coffee amount at T=3.5 minutes.
- 📌 The mean value theorem is mentioned, which guarantees the existence of a time T between 2 and 4 minutes where the instantaneous rate of change equals the average rate over that interval.
- 🔢 The midpoint sum rule with 3 subintervals of equal length is used to approximate the value of the integral of the coffee amount function over a 6-minute interval.
- ⏱ The integral represents the total amount of coffee added to the cup over the 6-minute interval, and the average value is found by dividing by the interval's width.
- 📐 The average amount of coffee in the cup over the 6-minute interval is calculated to be 10.1 ounces.
- 🔧 The rate of change of the amount of coffee in the cup when T=5 minutes is found by differentiating the given function and substituting T=5.
- 📉 The derivative of the exponential part of the function is found using the chain rule, leading to the final expression for the rate of change at T=5.
- 🎯 The final answer for the rate of change at T=5 is 6.4 divided by e squared, which is a correct application of the derivative and substitution.
- 🏁 The video concludes with a review of the scoring guidelines for the question, highlighting the importance of catching and correcting minor mistakes during the problem-solving process.
Q & A
What is the context of the video transcript?
-The video transcript is about a session with Alan from Bothell Stem Coach, where he is working through AP Calculus 2013 free response questions, specifically focusing on a problem involving the rate at which coffee fills a cup.
What is the mathematical concept being discussed in the beginning of the transcript?
-The mathematical concept being discussed is the use of secant line slopes to approximate the derivative of a function at a certain point, specifically C'(3.5), where C(T) represents the amount of coffee in the cup at time T.
What is the formula used to approximate C'(3.5)?
-The formula used to approximate C'(3.5) is (C(4) - C(3)) / (4 - 3), which simplifies to C(4) - C(3).
What is the mean value theorem mentioned in the transcript?
-The mean value theorem states that if a function is differentiable and continuous on a closed interval, then there exists a point 'c' in the open interval where the derivative of the function at 'c' is equal to the average rate of change of the function over the closed interval.
How is the average rate of change of coffee in the cup between times 2 and 4 found?
-The average rate of change is found using the secant line slope between the points corresponding to times 2 and 4, which is calculated as (C(4) - C(2)) / (4 - 2).
What is the midpoint sum method used for in the problem?
-The midpoint sum method is used to approximate the value of a definite integral. In the context of the problem, it is used to approximate the total amount of coffee in the cup over a 6-minute interval.
What is the integral that Alan is trying to approximate?
-Alan is trying to approximate the integral of the rate of coffee accumulation over a 6-minute interval, which represents the total amount of coffee in the cup during that time.
What is the average amount of coffee in the cup during the 6-minute interval?
-The average amount of coffee in the cup during the 6-minute interval is approximately 10.1 ounces, as calculated using the midpoint sum method.
What is the rate of change of the amount of coffee in the cup when T equals 5?
-The rate of change of the amount of coffee in the cup when T equals 5 is found by evaluating the derivative of the given function at T=5, which results in 6.4 / e^2 ounces per minute.
What is the scoring guidelines reference in the transcript?
-The scoring guidelines reference is likely a set of criteria or standards used to evaluate the correctness and completeness of a student's answers to the AP Calculus free response questions.
What is the significance of the scoring guidelines in the context of the video?
-The scoring guidelines are significant because they help the viewer understand what is expected in terms of correct answers and the minor mistakes that may be acceptable or penalized in an actual AP Calculus exam.
What mathematical tool is used to find the rate of change of the coffee amount in the cup?
-The derivative is used to find the rate of change of the coffee amount in the cup. Specifically, the derivative of the function modeling the amount of coffee in the cup with respect to time is calculated.
Outlines
📚 AP Calculus FRQ 2013 Question 3 Overview
This paragraph introduces the video content, which is focused on AP Calculus 2013 free response question number three. The discussion is about the non-calculator portion of the exam, specifically the problem involving the dripping of hot water through a coffee maker into a cup. The problem statement provides a formula for the amount of coffee in the cup at time T, measured in minutes, and a table of selected values for C(T). The video aims to approximate the derivative of C at T=3.5 using the secant line method and to show the computations involved. The presenter also touches on the mean value theorem and the concept of differentiability and continuity in functions.
🧮 Secant Line Method and Mean Value Theorem Application
The presenter calculates the derivative of C at T=3.5 using the secant line method, which involves finding the slope between two points. After a minor correction, the presenter finds that the derivative C'(3.5) is approximately 1.6 ounces per minute. The video then discusses the mean value theorem, which guarantees the existence of a time T where the instantaneous rate of change (C') equals the average rate of change between two points, in this case, between T=2 and T=4. The presenter uses the midpoint sum with three subintervals of equal length to approximate the integral of the function over the interval from 0 to 6 minutes. The midpoint sum is calculated, and the presenter simplifies the expression to find an average value of 10.1 ounces of coffee over the six-minute interval. The presenter also explains the concept of the average value of a function and how it relates to the volume under the curve divided by the width of the interval.
Mindmap
Keywords
💡AP Calculus
💡Free Response Questions
💡Secant Line Slope
💡Mean Value Theorem
💡Midpoint Sum
💡Derivative
💡Integral
💡Average Value
💡Coffee Maker
💡Rate of Change
💡E to the Power of
Highlights
Alan from Bothell stem coach continues with AP Calculus 2013 free response questions, focusing on question number three in the non-calculator portion.
The problem involves hot water dripping through a coffee maker, filling a large coffee cup, with the amount of coffee given by a function C(T) where T is measured in minutes.
Selected values of C(T) are provided in a table to approximate the derivative C'(3.5), representing the rate of coffee filling the cup at 3.5 minutes.
The secant line slope method is used to approximate C'(3.5), which involves calculating the difference in coffee levels between 3 and 4 minutes divided by the time difference.
The computation for C'(3.5) is shown to be (12.8 - 11.2) / (4 - 3), resulting in a rate of 1.6 ounces per minute.
Differentiability and continuity of C are discussed, with the mean value theorem implying the existence of a time T where C'(T) equals the secant line slope within the interval [2, 4].
The midpoint sum with 3 subintervals of equal length is used to approximate the integral of C(T) over the interval [0, 6], representing the total amount of coffee in ounces.
The average value of C over the interval [0, 6] is calculated to be 10.1 ounces, indicating the average amount of coffee in the cup during this time.
The rate at which the amount of coffee in the cup is changing when T equals 5 is found by differentiating the given model and evaluating it at T=5.
The derivative of the model at T=5 is calculated to be 6.4e^(-2), representing the rate of change of coffee in the cup at that time.
The scoring guidelines for question three are reviewed, with minor mistakes corrected during the process.
The importance of recognizing the average value in the context of the integral and its relation to the volume and width of the interval is emphasized.
The video concludes with a summary of the progress made in the AP Calculus test so far, highlighting the minor corrections made and the overall understanding of the problem.
Alan reassures viewers that catching minor mistakes while solving problems is a good practice for understanding and learning.
The video ends with a teaser for the next video, encouraging viewers to continue following the AP Calculus series.
The use of the midpoint sum and the trapezoid rule in approximating integrals is explained, providing a clear method for solving such problems.
The application of the mean value theorem in finding a specific time T where the instantaneous rate of change matches the average rate over an interval is demonstrated.
The process of differentiating the given function to find the rate of change at a specific time T is shown, illustrating the use of calculus in real-world problems.
The concept of the derivative as the rate of change and its calculation using the chain rule is explained, enhancing understanding of fundamental calculus principles.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: