What is Calculus used for? | How to use calculus in real life
TLDRCalculus, derived from the Latin word for pebble, is a fundamental mathematical language for describing the dynamic nature of the universe. It encompasses two branches: differential calculus, which deals with the rate of change and is used to predict behavior, and integral calculus, which focuses on areas and volumes under curves. The script illustrates the application of calculus in various fields, including aerospace, where it models rocket motion and thrust; economics, where it maximizes profits and revenue; and medicine, where it analyzes tumor growth rates. The fundamental theorem of calculus connects these branches, showcasing calculus as a versatile tool for solving problems involving change or motion.
Takeaways
- 📚 Calculus is a branch of mathematics that deals with rates of change and motion, including integration and differentiation.
- 🚀 Calculus is used in various fields such as aerospace, economics, and medicine to model and predict dynamic systems.
- 📈 In economics, calculus helps in maximizing profits and revenue by analyzing marginal revenue and costs.
- 🔬 In medicine, calculus can model the growth of tumors to determine the effectiveness of treatments and predict outcomes.
- ✈️ In aerospace engineering, calculus is essential for calculating thrust and modeling the motion of rockets.
- 🔢 The word 'calculus' originates from Latin, initially referring to the use of pebbles for computation, much like an abacus.
- 🔄 The two main branches of calculus are differential calculus, which involves derivatives, and integral calculus, which involves integration.
- 🔁 The Fundamental Theorem of Calculus, developed by Newton and Leibniz, connects differentiation and integration as inverse operations.
- 📉 Differentiation allows us to find the instantaneous rate of change of a quantity, which is crucial for predicting behavior in dynamic systems.
- 📈 Integration, the reverse of differentiation, helps in calculating areas and volumes, especially those with curved boundaries.
- 🔧 Calculus provides a powerful tool for analyzing and controlling systems in the physical world, from electricity and heat to light and sound.
- 📊 By using calculus, we can model phenomena such as stock market fluctuations, weather patterns, and the decay of radioactive materials.
Q & A
What is the origin of the word 'calculus'?
-The word 'calculus' originates from the Latin word for pebble, which the Romans used to perform calculations on an abacus. It became associated with computation, similar to the word 'calculator'.
How is calculus described as the language of?
-Calculus is described as the language of motion and change, as it is used to describe the dynamic nature of our universe.
What are the two main branches of calculus?
-The two main branches of calculus are differential calculus, which involves the concept of the derivative of a function, and integral calculus, which is the reverse process of differentiation.
What is the fundamental theorem of calculus?
-The fundamental theorem of calculus, created by Newton and Leibnitz, states that differentiation and integration are inverse operations. It connects these two branches and shows that if you differentiate a function and then integrate it, you will return to the original function.
How does calculus help in predicting weather?
-Calculus helps in predicting weather by using differential equations to analyze and predict the effects of changing conditions in the atmosphere, such as temperature and pressure.
What is the connection between rocket propulsion and calculus?
-Rocket propulsion uses calculus to model the thrust of a rocket into space, which is based on the calculus of motion. The thrust is essentially the rate of change of momentum, which can be calculated using the first derivative of momentum with respect to time.
How can calculus be used to maximize business profits?
-Calculus can be used to maximize business profits by finding the optimal quantity of products to produce and sell. This is done by differentiating the revenue function to find the marginal revenue and setting it equal to marginal cost to find the production level that maximizes profit.
What is the role of calculus in modeling the growth of a tumor in medicine?
-In medicine, calculus is used to model the growth of a tumor using an exponential function with respect to time. The first derivative of this function, known as the specific growth rate (SGR), provides information about the rate at which the tumor is growing or shrinking.
How does Newton's third law relate to rocket propulsion?
-Newton's third law, also known as the conservation of momentum, states that for every action, there is an equal and opposite reaction. In rocket propulsion, this means that as the exhaust is ejected backwards at high velocity, the rocket gains forward momentum, allowing it to accelerate upwards.
What is the significance of the first derivative in calculus?
-The first derivative in calculus represents the rate of change of a quantity with respect to another. It is used to find the instantaneous rate of change at any point, which is crucial in modeling and predicting behavior in various fields such as physics, economics, and medicine.
How does calculus help in determining the optimal solution in a system?
-Calculus helps in determining the optimal solution in a system by analyzing the behavior of the system under changing conditions. By using mathematical modeling of calculus, one can find the point at which a certain quantity, such as profit or efficiency, is maximized or minimized.
What is the process of integration in calculus?
-Integration in calculus is the process of finding the area under a curve or the volume of an object with a curved boundary. It is achieved by breaking the region into thin, infinite rectangles of equal width and taking a limit as the width of the rectangles approaches zero.
Outlines
📚 Calculus: The Language of Motion and Change
This paragraph introduces calculus as a fundamental tool in modern science and technology. It explains that calculus is used for modeling dynamic systems, such as the stock market or space travel, and is essential for predicting changes in various fields. The origin of the word 'calculus' is traced back to Latin, highlighting its historical significance in computation. The paragraph also emphasizes the beauty of calculus in forming relationships and describing the world's dynamics. It outlines the two branches of calculus: differential calculus, which involves derivatives and the rate of change, and integral calculus, which is about areas and volumes under curves. The fundamental theorem of calculus, linking differentiation and integration, is mentioned, showcasing the interconnectedness of these operations. Finally, the application of calculus in aerospace engineering is discussed, particularly in rocket propulsion and Newton's laws of motion.
🚀 Calculus in Aerospace and Economics
The second paragraph delves into the application of calculus in aerospace, specifically in rocket propulsion. It describes how Newton's laws of motion are applied to model the thrust of a rocket during launch, with calculus providing the mathematical framework to calculate momentum and force. The principle of conservation of momentum, as per Newton's third law, is also discussed in the context of rocket stages and the need to minimize mass for achieving desired velocities. The paragraph then transitions to the field of economics, illustrating how calculus can be used to maximize business profits. It explains the concept of marginal revenue and how differentiation can help find the optimal quantity of products to produce for maximum revenue. An example is provided using a hypothetical video game business to demonstrate the process of using calculus to determine the number of video games that should be sold to achieve maximum revenue.
🏥 Calculus in Medicine: Modeling Tumor Growth
The final paragraph explores the use of calculus in the medical field, particularly in modeling the growth of tumors over time. It presents an exponential function to represent the volume of a tumor and explains how differentiation can reveal the specific growth rate (SGR) of the tumor. This rate is crucial for doctors to determine the effectiveness of a treatment and to make informed decisions about therapy. The example of a doctor using calculus to analyze the progression of a patient's tumor is given to illustrate the practical application of calculus in medicine. The paragraph concludes by emphasizing the versatility of calculus as a tool for addressing problems involving change or motion across various disciplines.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Integration
💡Fundamental Theorem of Calculus
💡Motion
💡Change
💡Differential Equations
💡Optimization
💡Newton's Laws
💡Exponential Function
💡Specific Growth Rate (SGR)
Highlights
Calculus is used to model the dynamic nature of our universe, including motion and change.
The word 'calculus' originates from the Latin word for pebble, used in ancient Rome for computation.
Calculus has unlimited uses and benefits in various fields such as meteorology, physics, engineering, and economics.
Differential calculus involves the concept of the derivative, analyzing the rate of change of quantities.
Integral calculus, or anti-differentiation, allows for the calculation of areas and volumes with curved boundaries.
The Fundamental Theorem of Calculus connects differentiation and integration as inverse operations.
In aerospace, calculus models the thrust of a rocket and its momentum, applying Newton's laws of motion.
Rocket propulsion uses calculus to optimize the mass and achieve desired orbital velocity.
Calculus is essential in microeconomics for modeling financial problems and maximizing profits and revenue.
The marginal revenue, derived from the revenue function, helps determine the optimal quantity for maximum profit.
In medicine, calculus is used to model the growth of tumors and analyze the effectiveness of treatments.
The specific growth rate (SGR) of a tumor, found using calculus, provides insights into its progression or regression.
Calculus can predict future motion and analyze systems in fields like electricity, heat, light, harmonics, and acoustics.
The application of calculus in economics can help businesses maximize revenue by finding the optimal pricing and production levels.
Calculus is a powerful tool for modeling and predicting in any dynamic situation involving change or motion.
The use of calculus in various scientific and technological domains highlights its versatility and importance.
By differentiating and integrating functions, calculus provides precise mathematical models for a wide range of phenomena.
Transcripts
Browse More Related Video
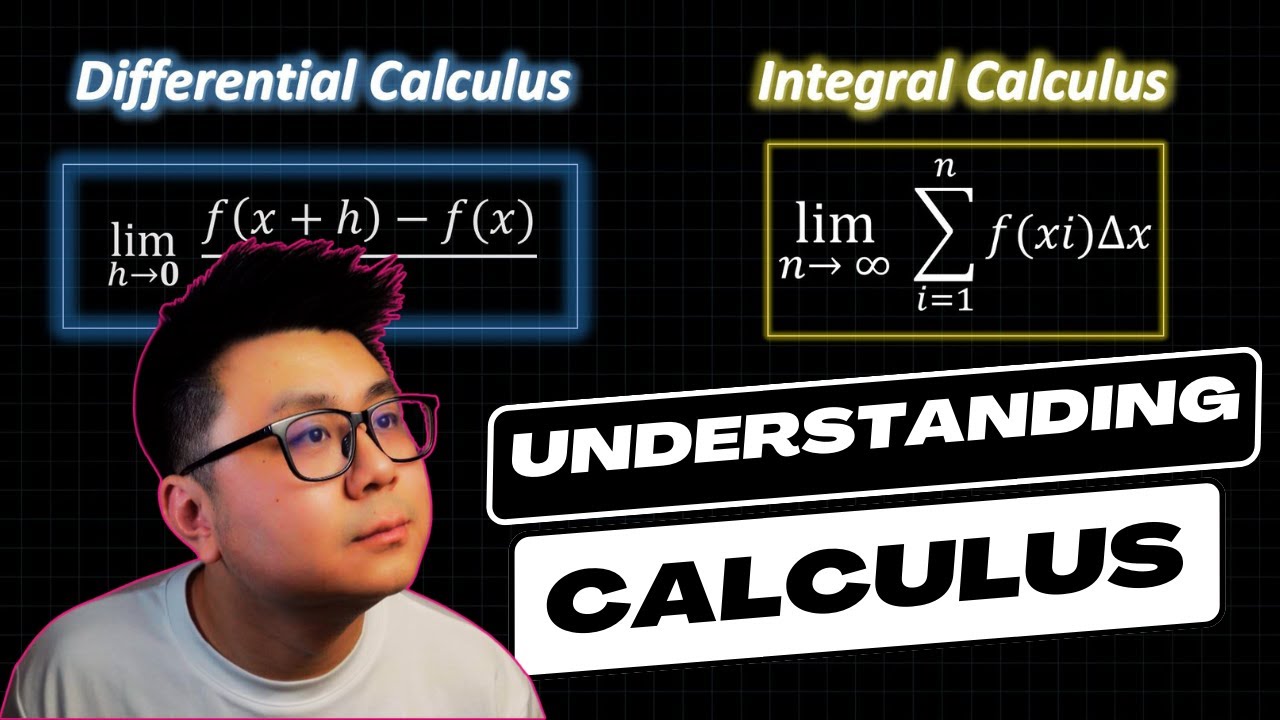
Calculus Made EASY! Learning Calculus
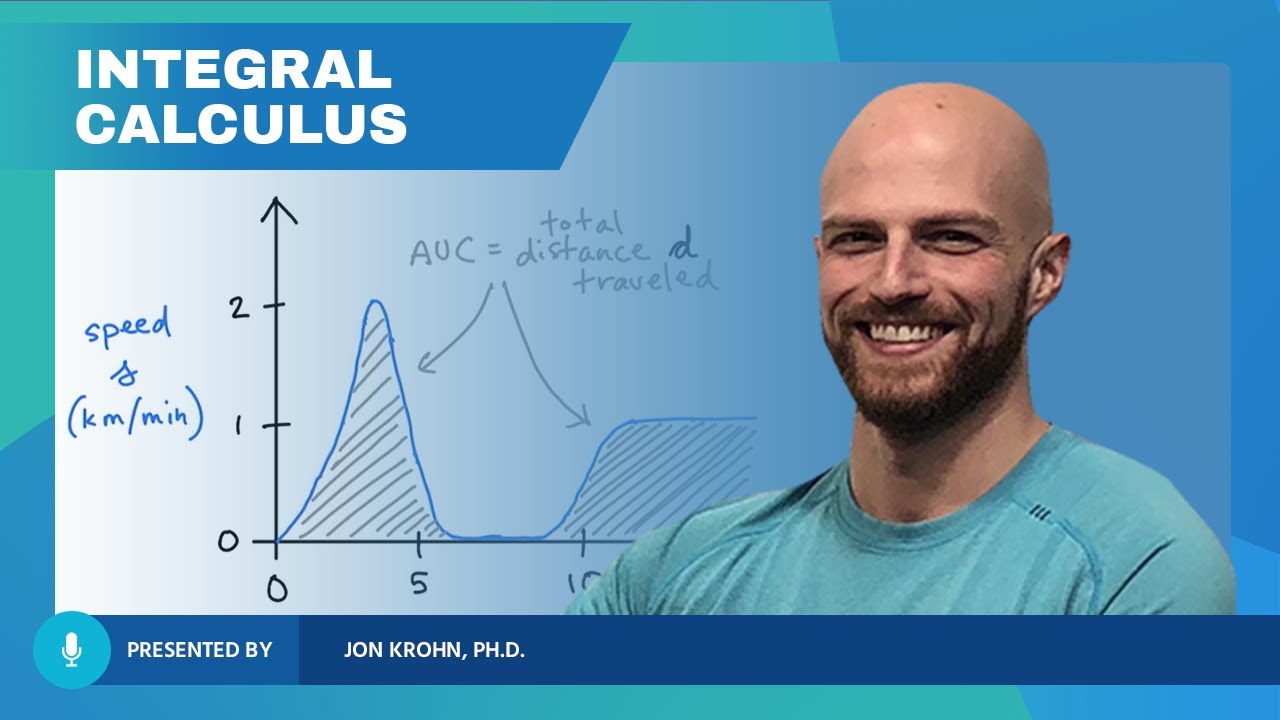
What Integral Calculus Is — Topic 85 of Machine Learning Foundations

Fundamental Theorem of Calculus 1 | Geometric Idea + Chain Rule Example
How to Solve Related Rates Problems in 5 Steps :: Calculus

Calculus in 20 Minutes with Professor Edward Burger
Lec 20 | MIT 18.01 Single Variable Calculus, Fall 2007
5.0 / 5 (0 votes)
Thanks for rating: