Properties of Definite Integrals - Basic Overview

The Organic Chemistry Tutor
20 Dec 201610:44
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into the fundamental properties of integrals, using various examples to illustrate key concepts such as anti-derivatives, the impact of interval reversal on integral values, and the additive nature of integrals over different segments of a function. It emphasizes understanding the geometric interpretation of integrals as areas under curves and the algebraic relationships between different integral expressions, ultimately aiming to strengthen the viewer's grasp of calculus principles.

Takeaways
  • 📚 The fundamental concept of integrals as anti-derivatives allows us to calculate the area under a curve.
  • 🔢 Multiplying an integral by a constant scales the result proportionally; for example, 2 times an integral of 5 equals 10.
  • 🔄 When the limits of integration are reversed, the result becomes the negative of the original integral, such as from 3 to 1 being -5.
  • 🕒 The value of an integral from a point to itself is zero, as the antiderivative values cancel each other out.
  • 📈 The sum of integrals over consecutive intervals equals the integral over the entire range, as demonstrated from 2 to 5 being the sum of 2 to 3 and 3 to 5.
  • 🌐 Graphical analysis of integrals can help visualize the area calculations, aiding in understanding the principles behind the calculations.
  • 🔄 The integral of a function over an interval can be found by subtracting the antiderivative at the lower limit from the antiderivative at the upper limit.
  • 🔢 The relationship between integrals of non-overlapping intervals can be used to determine the value of an integral over a larger interval.
  • 🔄 Understanding the algebraic nature of integrals helps in solving problems where intervals are reversed or combined.
  • 📈 The concept of net change is key to understanding integrals; the integral from one point to another represents the change in the antiderivative value.
  • 🔢 By combining given integral values and using the principles of integral calculus, complex problems can be solved step by step.
Q & A

  • What is the integral of f(x)dx from 1 to 3 if it equals 5?

    -The integral of f(x)dx from 1 to 3 is the anti-derivative of the function f(x) evaluated from 1 to 3, and according to the script, it is given that this value is 5.

  • If the integral of f(x)dx is 5 from 1 to 3, what is the value of 2 times this integral?

    -If the integral of f(x)dx from 1 to 3 equals 5, then 2 times this integral would be 2 * 5, which equals 10.

  • What happens to the integral value if the limits are reversed from the example given?

    -If the limits are reversed, so instead of from 1 to 3 it becomes 3 to 1, the answer becomes the negative of the original integral value. Thus, the integral from 3 to 1 would be -5.

  • What is the result of the integral of f(x)dx from 3 to 3?

    -The integral of f(x)dx from 3 to 3 is the anti-derivative evaluated at the same point, which means f(3) - f(3), and since they are the same, they cancel out, resulting in a value of 0.

  • How can we find the integral from 2 to 5 if the integral from 2 to 3 is 4 and from 3 to 5 is 7?

    -The integral from 2 to 5 can be found by summing the areas of the individual intervals. So, it is the integral from 2 to 3 (which is 4) plus the integral from 3 to 5 (which is 7), resulting in a total of 11.

  • What does the integral of a function represent in a graphical context?

    -In a graphical context, the integral of a function represents the area of the shaded region between the curve and the x-axis over the given interval.

  • How can we express the relationship between integrals from different intervals, as shown in the example with f(x)dx from 2 to 3 and f(x)dx from 3 to 5?

    -The relationship between integrals from different intervals can be expressed as the net change in the function's value over the intervals. For example, the integral from 2 to 3 is f(3) - f(2), and from 3 to 5 is f(5) - f(3). When combined, the positive and negative values cancel each other out, resulting in f(5) - f(2).

  • What is the integral of g(x)dx from 6 to 9 if the integral from 3 to 9 is -8 and from 3 to 6 is 5?

    -To find the integral from 6 to 9, we can relate the three integrals over the intervals 3 to 9, 3 to 6, and 6 to 9. The total area from 3 to 9 is the sum of the areas from 3 to 6 and 6 to 9. Given the integral from 3 to 6 is -8 and from 3 to 9 is 5, we solve for the unknown integral, x, by setting up the equation -8 + x + 5 = 0. Adding 8 to both sides gives us x = 13, so the integral from 6 to 9 is 13.

  • How does the integral from 4 to 7 relate to the integrals from 1 to 4 and from 7 to 9, given the values are -9 and -6 respectively?

    -The integral from 4 to 7 can be found by considering the net change in the function's value over the intervals from 1 to 4 and from 7 to 9. The integral from 1 to 4 is -9 and from 7 to 9 is -6. To find the value for the integral from 4 to 7, we add 9 (the absolute value of the integral from 1 to 4) to both sides of the equation -9 + x = -6. Solving for x gives us x = 3, indicating that the integral from 4 to 7 is +3.

  • Given the integral from 5 to 2 is 8 and from -1 to 5 is 12, how do we find the integral from 2 to -1?

    -To find the integral from 2 to -1, we first consider the total area from -1 to 5, which is 12. We know the integral from 5 to 2 is 8, so the remaining area from 2 to 5 must be 12 - 8 = 4. Since the integral from 2 to 5 is the sum of the integrals from 2 to -1 and -1 to 5, we can set up the equation 5 times the integral from 2 to -1 plus the integral from -1 to 5 equals 12. We know the integral from -1 to 5 is 12, so the equation simplifies to 5 * (integral from 2 to -1) + 12 = 12. Solving for the integral from 2 to -1 gives us -100.

Outlines
00:00
📚 Basic Properties of Integrals

This paragraph discusses the fundamental properties of integrals, focusing on the calculation of anti-derivatives and the evaluation of definite integrals. It begins with an example where the integral of a function from 1 to 3 is given as 5, and explores how to find the value of expressions with different constants or reversed limits. The concept of antiderivatives being equal to function values at the limits of integration is emphasized, as well as the property that integrals over identical intervals will always yield zero. The paragraph then introduces the idea of using graphical analysis to understand the sum of integrals over different intervals, ultimately highlighting the principle that the integral from a lower limit to an upper limit is the sum of the integrals over any subintervals.

05:01
🔢 Solving Integral Equations

This section delves into solving equations involving integrals by establishing relationships between different integrals over various intervals. It presents a scenario where the integral of a function g from 3 to 9 is known, and the goal is to find the value of the integral from 6 to 9. The explanation involves setting up an equation that relates the integrals and solving for the unknown value. The paragraph continues with more examples, each time using the given integral values to find missing integrals, reinforcing the concept of using known integrals to deduce unknown ones and the importance of understanding the net change represented by integrals.

10:02
📈 Evaluating Complex Integral Expressions

The final paragraph focuses on evaluating complex integral expressions using the information provided in previous integral values. It presents a series of problems where the integral of a function f from different intervals is given, and the task is to find the value of a new integral expression. The explanation involves combining integrals from various intervals and using the properties of integrals to simplify and solve for the unknown integral. The paragraph concludes with a methodical approach to finding the value of an integral with a negative lower limit by transforming the interval into a positive one and using the known total integral to solve for the unknown.

Mindmap
Keywords
💡Integrals
Integrals are a fundamental concept in calculus that represent the accumulation of a quantity, often visualized as the area under a curve. In the context of the video, integrals are used to calculate the antiderivatives of functions, which helps in understanding the net change or accumulation of a variable over a given interval. For example, the video explains how to find the integral of a function from one to three and demonstrates how this relates to the area under the curve of the function in that interval.
💡Antiderivatives
Antiderivatives, also known as indefinite integrals, are functions that represent the reverse process of differentiation. They are used to find the original function from its derivative. In the video, the concept of antiderivatives is crucial in determining the value of integrals, as it allows the calculation of the accumulated change over an interval. The video demonstrates this by showing how to find the antiderivatives of a function and use them to compute the value of integral expressions.
💡Net Change
Net change refers to the difference in a particular quantity after a process or over a period. In the context of the video, it is used to describe the difference in the value of a function at two points, which is calculated through integration. The net change is the core concept that integrals represent, as they measure the accumulated change over an interval. The video explains this by showing how the integral of a function from one point to another is equivalent to the difference in the function's values at those points.
💡Area Under the Curve
The area under the curve of a function is a geometric interpretation of integrals, representing the sum of the values that the function takes over a certain interval. In the video, this concept is used to visualize and understand the results of integrals, as the area under the curve between two points corresponds to the value of the integral for that interval. This helps in understanding how integrals can be used to calculate quantities such as volume, work, or probability in various contexts.
💡Additivity
Additivity is a property of integrals that states the integral of a function over a sum of intervals is equal to the sum of the integrals over each interval. This property is crucial for breaking down complex integration problems into simpler parts and solving them individually. The video emphasizes this by explaining how the integral from 2 to 5 can be found by adding the integrals from 2 to 3 and from 3 to 5.
💡Limits of Integration
The limits of integration are the boundaries that define the interval over which an integral is calculated. They are crucial in determining the specific region under the curve that is being summed or accumulated. In the video, the limits of integration are consistently used to set up the problem and calculate the area or net change that the integral represents.
💡Function Evaluation
Function evaluation refers to the process of determining the value of a function at specific points or for specific inputs. In the context of the video, function evaluation is essential for calculating the value of integrals, as it often involves finding the function's value at the limits of integration. This helps in determining the accumulated change or area under the curve for a given interval.
💡Graphical Analysis
Graphical analysis is a method of solving mathematical problems by visualizing them on a graph or diagram. In the video, graphical analysis is used to understand the concept of integrals and their additivity property by visualizing the area under the curve of a function. This approach helps in intuitively understanding how integrals can be added or subtracted when the intervals overlap or are consecutive.
💡Integration by Parts
Integration by parts is a technique used in calculus to evaluate integrals by breaking down a single integral into a sum of simpler integrals. It is based on the product rule for derivatives and is particularly useful when the integral involves a product of two functions. Although not explicitly mentioned in the video, the concept is related to the additivity property discussed and could be used in more complex integration problems.
💡Negative Integrals
Negative integrals occur when the interval of integration is reversed, meaning the upper limit is less than the lower limit. This results in a negative value for the integral, as it represents the opposite direction of accumulation or a decrease in the function's value over the interval. The video explains this by showing that reversing the limits of integration results in the negative of the integral value.
💡Integration Intervals
Integration intervals refer to the specific ranges over which an integral is calculated. These intervals are important in defining the scope of the integration problem and determining the limits within which the function's values are accumulated or summed. The video script consistently uses integration intervals to set up and solve various integral problems.
Highlights

The video reviews basic properties of integrals, providing foundational knowledge for understanding calculus.

The integral of a function from one to three is given as an example with a value of 5.

Doubling the integral 'f of x dx from one to three' results in a value of 10, showcasing the linearity of integrals.

Reversing the limits of integration (three to one) results in a negative value, illustrating how limits affect the sign of the integral.

Evaluating the antiderivative at a single point (from 3 to 3) results in a zero value, as the function values cancel each other out.

The graphical interpretation of integrals as areas under the curve is introduced, aiding in visualizing the concept.

The principle of additivity of integrals is explained, where the integral from two to five is the sum of integrals from two to three and three to five.

The concept of net change is used to explain the difference in function values at different points of integration.

An example is given to demonstrate how to find the value of an integral when related integrals are known, using the principle of additivity.

The method of finding the value of an integral by relating it to known integrals and solving for the unknown is illustrated.

The video emphasizes the importance of understanding the relationship between different parts of an integral and their corresponding areas.

An example is provided to show how to calculate the integral over a complex interval by breaking it down into simpler parts.

The concept of integrating over a reverse interval is explored, and how it relates to the original integral.

The video concludes with a comprehensive example that ties together the concepts of integral properties, additivity, and net change.

Transcripts

Browse More Related Video

Calculus 1 Lecture 4.5: The Fundamental Theorem of Calculus

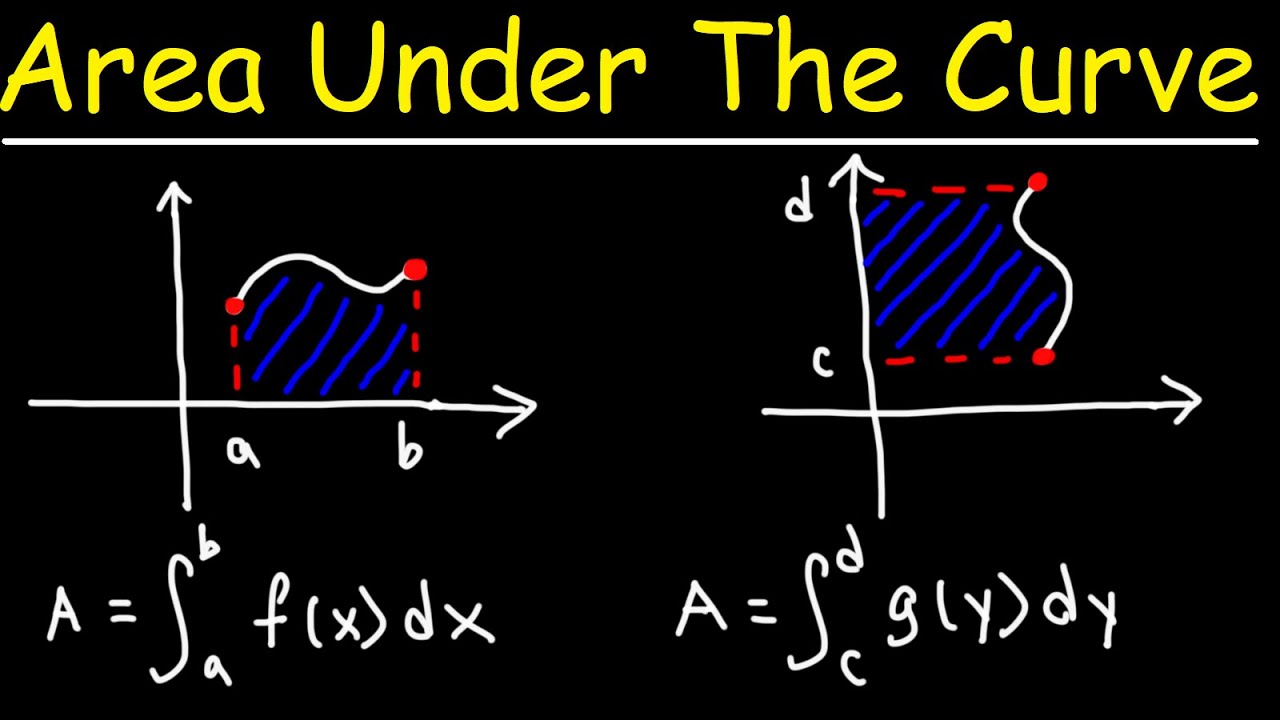
Finding The Area Under The Curve Using Definite Integrals - Calculus

Math 1325 Lecture 13 2

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Applications of Integrals Review (All of AP Calculus Unit 8)

Lec 20 | MIT 18.01 Single Variable Calculus, Fall 2007

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegral PropertiesAntiderivativesFunction AnalysisMathematical ConceptsEducational ContentMath TutorialArea Under CurveNet ChangeFundamental Theorems