Understanding Integral Notation

Tommea Analytics
29 May 201704:10
EducationalLearning
32 Likes 10 Comments

TLDRThis video from Tommy Analytics introduces integral notation, focusing on calculating the area under the curve of a function, specifically x^2 between points A and B. It explains the use of rectangles to approximate the area, transitioning from rectangles to infinitesimally small widths represented by Δx, and finally to the summation notation, ∑, to accurately capture the area. The video also touches on the concept of Δy for areas along the y-axis. It encourages viewers to engage with questions or comments.

Takeaways
  • 💡 The function under consideration, f(x) = x^2, represents a simple parabola.
  • 🗣 Integral notation is used to approximate the area under the curve of f(x) between two points, A and B.
  • 🖥 The area under the curve is approximated using rectangles, with the area of each rectangle being the product of its width (Δx) and height (f(x)).
  • 👉 The width of these rectangles is referred to as Δx, and the height is determined by the function value f(x), or the y-value at the left endpoint.
  • 🔍 A major challenge in using rectangles is that they do not account for the error (represented as blue area) underneath the curve.
  • 🤖 To minimize the error, the widths of the rectangles are made infinitesimally small, transforming Δx into dx, which represents a very tiny change in x.
  • 📈 As the widths shrink to almost zero, more rectangles fit under the curve, greatly reducing the approximation error.
  • 📚 The elongated 's' symbol in integral notation stands for summation, indicating the sum of areas of all rectangles to approximate the area under f(x) = x^2 between A and B.
  • 📌 Additionally, dy might be seen when rectangles are oriented along the y-axis, with dy representing an infinitesimally small change in y, similar to dx for the x-axis.
  • 📰 The script concludes by encouraging questions or comments to be posted below the video, fostering viewer engagement.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is integral notation, specifically focusing on understanding the area under a curve, such as the function x^2 between points A and B.

  • What does the 'F' inside the integral symbol represent?

    -The 'F' inside the integral symbol represents the function that is being integrated. In this case, the function is x^2, which is a simple parabolic function.

  • How is the area under the curve approximated in the initial explanation?

    -The area under the curve is approximated by using rectangles, where the area of each rectangle is calculated as the width times the height.

  • What does the term 'width' refer to in the context of approximating the area under a curve?

    -In the context of approximating the area under a curve, 'width' refers to the change in X, which is also known as Δx.

  • What does the term 'height' refer to in the context of approximating the area under a curve?

    -In the context of approximating the area under a curve, 'height' refers to the value of the function F(x) at a particular x value, which is the y-value of the function at that point.

  • Why is it necessary to shrink the rectangles to infinitesimally small widths?

    -It is necessary to shrink the rectangles to infinitesimally small widths to eliminate the error between the rectangles and the actual curve, providing a more accurate approximation of the area under the curve.

  • What does the symbol 'dx' represent?

    -The symbol 'dx' represents an infinitesimally small change in x, which is used when the widths of the rectangles are shrunk to very small values in the process of finding the area under the curve.

  • What is the elongated 'S' symbol used to represent in the integral notation?

    -The elongated 'S' symbol, known as the summation symbol, is used to represent the process of adding up all the infinitesimally small rectangles' areas to approximate the area under the curve.

  • What does 'Δy' represent in the context of integrals?

    -'Δy' represents the change in y when the rectangles are considered along the y-axis. It is used when the widths of the rectangles are with respect to y, as opposed to Δx which is used when the widths are along the x-axis.

  • How can one improve their understanding of integrals?

    -To improve understanding of integrals, one should practice visualizing the process of shrinking rectangles and summing their areas, as well as solving problems involving different functions and intervals.

  • What is the significance of the points A and B in the integral?

    -The points A and B in the integral define the interval over which the area under the curve is being calculated. The integral will sum up the areas of infinitesimally small rectangles between these two points.

Outlines
00:00
📚 Introduction to Integral Notation

The video begins with an introduction to integral notation, focusing on the concept of the function F(x) which is x² in this case, representing a simple parabolic function. The main objective is to calculate the area under the curve between two points, A and B. The method involves approximating this area with rectangles, where the width represents the change in X (∆X) and the height is the function value F(x). The video explains the process of refining this approximation by shrinking the rectangles' widths to infinitesimal sizes, symbolized as ∆X, to better fit under the curve and reduce error. Additionally, the summation symbol (∑) is introduced to represent the sum of the areas of all rectangles, providing a closer estimate of the actual area under the curve. The video also mentions the alternative notation ∆y for when rectangles are aligned along the y-axis, with widths representing the change in y.

Mindmap
Keywords
💡Integral
Integral is a fundamental concept in calculus that represents the accumulation of a quantity over a given interval. In the context of this video, it is used to calculate the area under the curve of a function, specifically the function f(x) = x^2 between points A and B. Integrals are essential for solving a variety of real-world problems, such as determining the displacement of an object or the total volume of a substance.
💡Function
A function is a mathematical relation that couples each element from a given set (the domain) with exactly one element from another set (the range). In this video, the function of interest is f(x) = x^2, which represents a simple parabolic curve. Functions are central to many areas of mathematics and science, as they describe relationships between different variables and can be used to model a wide range of phenomena.
💡Area
Area refers to the amount of space enclosed within a two-dimensional shape. In mathematical terms, it is a measure of the size of a surface. In the video, the area is used to describe the region under the curve of the function f(x) = x^2 between points A and B. Calculating areas is crucial in various fields, including geometry, physics, and engineering, for determining sizes and volumes.
💡Approximation
Approximation is the process of estimating a value or quantity that is nearly equal to another value or quantity. In mathematics, approximations are often used when exact values are difficult or impossible to determine. In the video, rectangles are initially used to approximate the area under the curve, but as the widths of the rectangles become infinitesimally small, the approximation becomes more accurate, leading to the concept of an integral.
💡Rectangles
In the context of the video, rectangles are used as a tool for approximating the area under a curve. By using the width of the rectangles (change in X) and the height (function value at a point), one can estimate the area. This method is a visual and intuitive way to introduce the concept of integration, where the area under the curve is divided into small segments, each represented by a rectangle.
💡Change in X (ΔX)
Change in X, often denoted as ΔX, refers to the difference in the X-values between two points on the X-axis. In the process of approximating areas under a curve, ΔX represents the width of the rectangles used in the approximation. As the rectangles become smaller and more numerous, the approximation of the area becomes more accurate, eventually leading to the precise calculation of the integral.
💡Summation
Summation is the process of adding together a sequence of numbers or terms. In the context of this video, summation is used to combine the areas of all the rectangles to approximate the total area under the curve. The summation symbol (Σ) is used to denote this process, which is a key concept in understanding how integration works to find the area under a curve.
💡Error
Error, in the context of mathematical approximations, refers to the difference between the true value and the approximate value. In the video, the error is associated with the initial approximation of the area under the curve using rectangles. As the rectangles become smaller, the error decreases, and the approximation becomes more accurate. The goal is to minimize this error to get a precise estimate of the area.
💡Differential (dx)
Differential, often represented by 'dx', is a mathematical term used to describe an infinitesimally small change in a variable. In the context of the video, 'dx' represents the infinitely small width of the rectangles used in the approximation of the area under the curve. The concept of the differential is crucial in calculus as it allows for the precise calculation of derivatives and integrals.
💡Dy
In the context of the video, 'Dy' represents an infinitesimally small change in the Y direction, which is perpendicular to the X-axis. It is used when considering rectangles that are aligned along the Y-axis, as opposed to 'dx' which is used for changes along the X-axis. The concept of 'Dy' is important in understanding how to set up integrals when the area of interest is oriented with respect to the Y-axis.
💡Curve
A curve is a continuous, smooth shape formed by connecting a series of points with a line. In mathematics, curves are often represented by functions and can be analyzed in terms of their geometric and algebraic properties. In the video, the curve of interest is the graph of the function f(x) = x^2, which is a parabola. Understanding curves is essential in various fields, including calculus, where they are used to study the behavior of functions.
Highlights

Introduction to integral notation and its application in calculating areas under a curve.

Explanation of the function F(x) in the context of the integral, specifically F(x) = x^2 representing a simple parabola.

Discussion on the area under the curve x^2 between two points A and B and how to approximate it using rectangles.

The concept of width and height in approximating area, where width is the change in X (∆X) and height is the function value (F(x))

The transition from using rectangles to infinitesimally small widths to reduce error in area approximation.

The change in notation from ∆X to dx, symbolizing the infinitesimally small change in X.

The summation notation (∑) used to represent the addition of an infinite number of rectangles to approximate the area under the curve.

Clarification on the use of dy when dealing with rectangles along the y-axis, as opposed to dx along the x-axis.

The importance of shrinking the widths of rectangles to fit more underneath the curve and eliminate approximation error.

The practical application of integral calculus in approximating complex shapes and areas, such as those in physics and engineering.

The video's aim to provide a clear and engaging explanation of integral notation, making it accessible to viewers.

The use of visual aids, such as shading and diagrams, to enhance understanding of integral concepts.

The encouragement for viewers to ask questions and engage in discussions to deepen their understanding of the material.

The acknowledgment of the limitations of using rectangles for approximation and the transition to more advanced methods.

The potential for this foundational knowledge of integrals to be applied in various fields, highlighting the versatility of mathematical concepts.

Transcripts

Browse More Related Video

Lec 18 | MIT 18.01 Single Variable Calculus, Fall 2007

AP Calculus AB: Lesson 6.2 Part 2 (Limit Definition of Definite Integral)

Riemann approximation introduction | Accumulation and Riemann sums | AP Calculus AB | Khan Academy

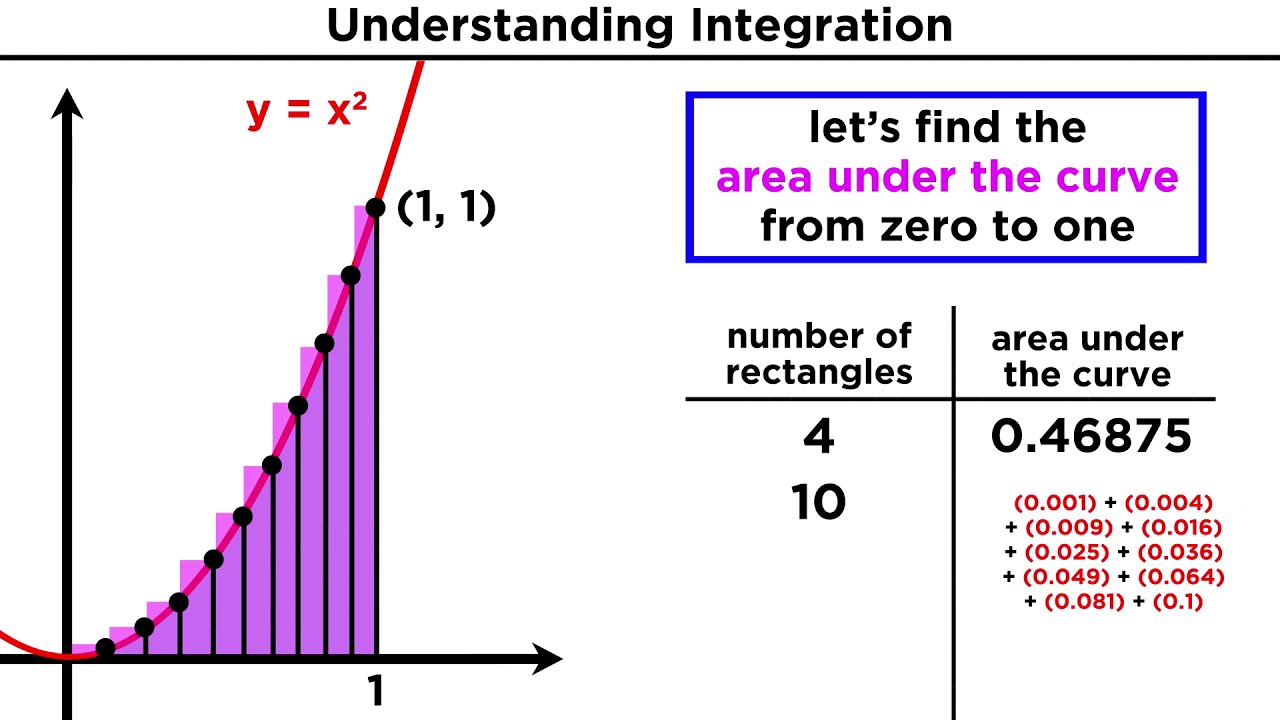
What is Integration? Finding the Area Under a Curve

The Definite Integral Part II: Using Summation Notation to Define the Definite Integral

The Definite Integral Part I: Approximating Areas with rectangles

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Integral NotationMathematicsCalculusEducationalTutorialArea CalculationFunction AnalysisSummationMath GuideLearning Resource