Calculus 5.2e - Notation and Terminology

Derek Owens
12 Apr 201104:35
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the fundamentals of calculus, focusing on notation and terminology related to differentiation and integration. It explains the concept of 'D over DX' as an operator representing the derivative, and similarly introduces the integral sign as an operator acting on functions. The script emphasizes the inverse relationship between differentiation and integration, likening it to undoing operations such as squaring a number and then finding its square root. An example is provided to illustrate finding the indefinite integral of a function, introducing terms like 'integrand' and various synonyms for the process of integration, including 'anti-differentiation' and 'evaluation of integral'.

Takeaways
  • 📌 The notation D/DX represents the derivative of a function, which can be thought of as an operator acting on the function.
  • 📈 The meaning of dy/dx is the derivative of y with respect to x, which can be interpreted not only as a slope but also as the rate of change of the function.
  • 🌟 The integral symbol can also be viewed as an operator, with the function to be integrated placed between the integral sign and the differential (dx).
  • 🔄 Differentiation and integration are inverse operations, meaning that applying one after the other will return the original function.
  • 🔢 The process of finding the integral of a function is sometimes referred to as 'anti-differentiation', 'integrating', 'evaluating the integral', or 'doing the integration'.
  • 📊 The term 'integrand' is used to describe the function that is being integrated within the integral expression.
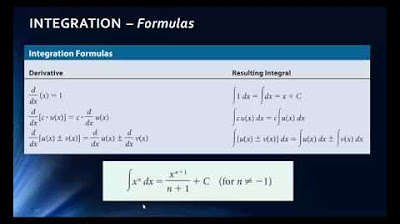
  • 🎓 The integral of a function, such as x to the power of 4, is found by applying the appropriate anti-derivative rules, resulting in x to the power of 5 divided by 5, plus a constant (C).
  • 🌐 The concept of an operator in calculus is crucial as it simplifies the understanding and application of differentiation and integration.
  • 📚 The script emphasizes the importance of understanding the notation and terminology in calculus to effectively perform operations on functions.
  • 🔍 The process of integration essentially reverses the process of differentiation, highlighting the connection between these fundamental calculus concepts.
  • 📈 The script uses the example of integrating x^4 to demonstrate the steps and terminology involved in finding the anti-derivative of a given function.
Q & A

  • What does the notation 'D over DX' represent in calculus?

    -In calculus, 'D over DX' represents the derivative operator. It is used to denote the derivative of a function with respect to the variable x, similar to how 'dy/dx' is used. The 'D' stands for differential, and it is applied to a function to find its rate of change or slope at any given point.

  • How can the derivative operator be thought of in terms of algebra?

    -In algebraic terms, the derivative operator 'D' can be thought of as a multiplication with the variable 'y'. So, 'D * y' would represent the derivative of 'y' with respect to 'x'. This operator is applied to a function to yield its derivative, just as other algebraic operations like squaring or taking a square root are applied.

  • What is the meaning of the integral symbol in calculus?

    -The integral symbol in calculus represents the integral operator. It is used to denote the process of integration, which is the reverse operation of differentiation. The integral of a function involves finding the antiderivative, or the function from which the original function could have been derived through differentiation.

  • How do differentiation and integration act as inverse operations?

    -Differentiation and integration are inverse operations in the sense that applying one after the other returns the original function. If you differentiate a function and then integrate the result, you should get back the original function, assuming the function is continuous and integrable. This property is fundamental in calculus and is analogous to taking a number, squaring it, and then taking the square root to get back the original number.

  • What is the term used to describe the function that is being integrated in an integral expression?

    -The term used to describe the function that is being integrated in an integral expression is called the 'integrand'. It is the part of the integral expression that sits inside the integral symbol and with respect to which the integration is performed.

  • What is the result of integrating a function called?

    -The result of integrating a function is called the 'indefinite integral' or 'antiderivative'. It represents a family of functions that differ by a constant (denoted by 'C'), which accounts for the arbitrary constant that can be added to any antiderivative without changing the derivative.

  • What are the synonyms for the process of finding the integral of a function?

    -The process of finding the integral of a function can be referred to as 'integrating', 'evaluating the integral', or 'doing the integration'. All these terms describe the process of finding the antiderivative of a given function.

  • How do you represent the integral of a function mathematically?

    -The integral of a function is mathematically represented by the integral symbol (∫), followed by the function and the differential of the variable with respect to which the integration is performed (usually 'dx'). For example, the integral of a function f(x) with respect to x would be written as ∫f(x)dx.

  • What is the relationship between the derivative and the integral of a function?

    -The derivative of a function gives the rate of change or slope at any point of the function, while the integral provides a way to find the original function from which the derivative could have been obtained. The process of finding the integral of a function is also known as anti-differentiation, highlighting that it is the reverse process of differentiation.

  • What is the result of differentiating and then integrating a function?

    -If you differentiate a function and then integrate the result, you should get back the original function, provided that the function is integrable. This is due to the inverse relationship between differentiation and integration, where integration can 'undo' the process of differentiation.

  • How does the concept of an 'indefinite integral' relate to the concept of an 'antiderivative'?

    -The indefinite integral and the antiderivative refer to the same concept in calculus. They both describe the result of integrating a function, which is a family of functions that differ by a constant. The term 'antiderivative' emphasizes the inverse relationship to differentiation, while 'indefinite integral' highlights the fact that the constant of integration is not specified.

Outlines
00:00
📚 Introduction to Calculus Notation and Terminology

This paragraph introduces the basics of calculus notation and terminology, focusing on the representation of derivatives and integrals. It explains the meaning of 'D over DX' as an operator acting on a function, similar to how a square root operates on a variable. The paragraph emphasizes the inverse relationship between differentiation and integration, likening it to the process of undoing an operation to return to the original value. It also provides a clear example of finding the indefinite integral of a function, highlighting the terms 'integral' and 'integrand', and explains the process of anti-differentiation or integration.

Mindmap
Keywords
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. In the video, calculus is the main subject, focusing on two fundamental operations: differentiation and integration. These operations are inverse to each other, meaning that they can 'undo' each other's effects, similar to how taking a square root and then squaring a number will return the original number.
💡Notation
Notation refers to the symbols and abbreviations used in a particular field, such as mathematics, to represent concepts and operations. In the context of the video, notation is important for understanding the symbols used to represent derivatives (dy/dx) and integrals (∫f(x)dx). The video explains the meaning behind these symbols and how they relate to the functions they act upon.
💡Derivative
A derivative is a concept in calculus that represents the rate of change or the slope of a function at a particular point. In the video, the derivative is symbolized as dy/dx and can be thought of as an operator acting on a function to find its rate of change. The derivative is a fundamental concept used to analyze how a function changes and is an essential tool in various mathematical and real-world applications.
💡Integral
An integral is a mathematical concept used to find the accumulated quantity or area under a curve of a function. In the video, integrals are symbolized using the integral sign (∫) and act as an operator on a function, indicating the process of accumulation. Integrals are used to solve problems involving accumulation, such as calculating the total distance traveled by an object or the area of a shape.
💡Operator
In mathematics, an operator is a symbol or a set of rules that act on one or more operands (numbers, variables, or functions) to produce a new value or expression. In the video, both the derivative (dy/dx) and integral (∫) are described as operators that act on functions to yield new functions or values. This concept helps to understand how these operations modify or transform the input functions.
💡Inverse Operations
Inverse operations are mathematical operations that, when applied in sequence, will return the original value or state. In the video, differentiation and integration are described as inverse operations because applying them in reverse order to a function will yield the original function. For example, integrating a function and then differentiating the result will return the original function.
💡Terminology
Terminology refers to the specific words and phrases used within a particular discipline or field. In the context of the video, terminology is important for understanding the language of calculus, including terms like 'integral', 'integrand', and 'anti-derivative'. The video clarifies these terms to help viewers better understand the concepts being discussed.
💡Anti-Derivative
An anti-derivative, also known as an indefinite integral, is a function whose derivative is the given function. In the video, finding the anti-derivative of a function involves integrating the function to find a new function whose derivative will be the original function. This process is a key part of understanding calculus and its applications.
💡Indefinite Integral
The indefinite integral, represented by the symbol ∫, is the process of finding a function whose derivative is a given function, without specifying the constant of integration. It is called 'indefinite' because it does not have a specific value; instead, it represents a family of functions that differ by a constant. In the video, the indefinite integral is discussed as a way to find the anti-derivative of a function.
💡Integrand
The integrand is the function that is being integrated in the process of finding an integral. It is the part of the integral expression that lies inside the integral symbol and is being 'operated on' by the integral operator. In the video, the integrand is emphasized as the function that will contribute to the final result of the integration.
💡Integration
Integration is the process of finding the integral or the anti-derivative of a function. It is the inverse operation of differentiation and is used to find the accumulated quantity or area under a curve. In the video, integration is described as the action of the integral operator on the integrand, resulting in the integral expression.
Highlights

Introduction to the concept of notation and terminology in calculus.

Explanation of the meaning of D/DX for a function y = f(x).

Derivative as an operator acting on a function.

Integral sign as an operator and its algebraic representation.

Integration as the inverse operation of differentiation.

Derivative of an integral being the original function.

The process of finding the anti-derivative or indefinite integral of a function.

The integral expression and its components, including the integrand.

Terminology associated with integrals: integrand, integrating, evaluating, and anti-differentiating.

Example provided: finding the indefinite integral of x^4.

Result of the example: integral of x^4 is x^(5/5) + C.

Differentiation and integration as inverse operations that can undo each other.

The concept of an operator in calculus and its application to functions.

The role of the integral sign in representing the operation of integration.

Understanding the relationship between differentiation and integration as fundamental operations in calculus.

The practical application of calculus in solving problems involving rates of change and accumulation.

Transcripts

Browse More Related Video

The Indefinite Integral or Anti-derivative

Math 1325 Lecture 12 1

Antiderivatives

The Fundamental Theorem of Calculus: Redefining Integration

4.1 - Antidifferentiation

Fundamental Theorem of Calculus - Part I

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus BasicsDerivativesIntegrationInverse OperationsMath EducationFunction AnalysisAnti-DerivativesIntegral CalculusMath TerminologyEducational Content